Implicit Differentiation | Basic Calculus

Prof D
26 Jan 202114:31
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, the host Prof D demonstrates the process of implicit differentiation to find the derivative of functions where 'y' is not explicitly given as a function of 'x'. The tutorial covers four examples, each illustrating how to differentiate complex expressions involving 'x' and 'y', and then isolate 'y' to find its derivative with respect to 'x'. The examples range from simple quadratic expressions to more complex cubic and exponential terms, showcasing the application of power rules, product rule, and chain rule in implicit differentiation. The video is designed to help viewers understand and apply implicit differentiation techniques effectively.

Takeaways
  • 📚 The video is a tutorial on differentiating functions of y with respect to x using implicit differentiation.
  • 🔍 The first example involves differentiating the equation ( x^2 + y^2 - 2x + 3y = 4 ).
  • 📝 The process includes differentiating each term with respect to x and isolating ( y' ) to find the derivative.
  • 🧩 The second example is differentiating ( x^3 - 3x + y^2 = 16 ), where the chain rule is not needed.
  • 📉 The third example features the differentiation of ( y + (2x - 5)^3 = 3 ), which requires the chain rule.
  • 🔢 In the third example, the derivative is simplified to ( y' = rac{-9(2x - 5)^2}{1} ).
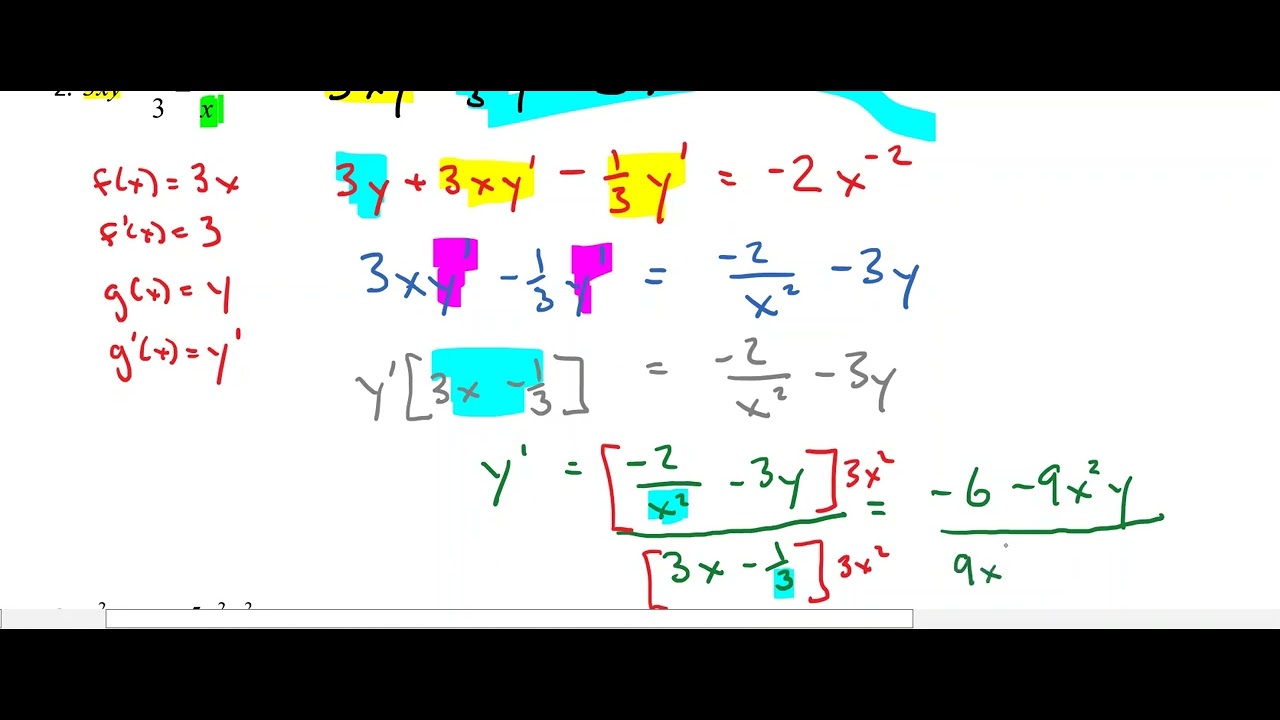
  • 🌐 The fourth and final example is the differentiation of ( x^2 + 3x^4y^2 + y^2 = -4x ).
  • 📐 The fourth example uses both the power rule and the product rule to differentiate the terms.
  • 📘 The final derivative for the fourth example is ( y' = rac{-2 - 6x^3y^2}{3x^4y + y} ).
  • 👨‍🏫 The presenter, Prof D, provides step-by-step instructions for each differentiation example.
  • 👋 The video concludes with an invitation for viewers to ask questions in the comments section.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is how to differentiate functions of y with respect to x using implicit differentiation.

  • Why is it difficult to isolate y in the given equation x squared plus y squared minus two x plus three y equals four?

    -It is difficult to isolate y in the given equation because the equation is not defined explicitly as a function of x, meaning y cannot be expressed directly in terms of x.

  • What is the process called when finding the derivatives of implicit functions?

    -The process is called implicit differentiation.

  • What is the first step in differentiating each term with respect to x according to the video?

    -The first step is to apply the power rule to differentiate each term, including x^2, y^2, and any other terms involving x or y.

  • How does the video demonstrate the differentiation of y squared in the first example?

    -The video demonstrates the differentiation of y squared by applying the power rule, resulting in 2y, and since y is a function of x, it is written as 2y with y' (the derivative of y with respect to x).

  • What is the derivative of a constant like the number four in the equation?

    -The derivative of a constant is zero, as constants do not change with respect to the variable.

  • How does the video isolate y' (the derivative of y with respect to x) in the first example?

    -The video isolates y' by rearranging the terms and factoring out y' from the left side of the equation, then dividing both sides by the terms involving y' to solve for y'.

  • What is the final expression for the first derivative of the implicit function in the first example?

    -The final expression for the first derivative in the first example is y' = (-2x + 2) / (2y + 3).

  • Can you provide an example from the video where the chain rule is applied?

    -An example from the video where the chain rule is applied is in the third example, where the derivative of (2x - 5)^3 involves multiplying the derivative of the inner function (2x - 5) by the power to which it is raised.

  • What is the final answer for the derivative of the implicit function in the third example?

    -The final answer for the derivative in the third example is y' = (3/2) * (2x - 5)^(-2).

  • How does the video handle the differentiation of a term involving both x and y, such as 3x^4y^2, in the fourth example?

    -The video uses the product rule to differentiate the term 3x^4y^2, treating x^4 as u and y^2 as v, and then applying the product rule to find the derivative.

  • What is the final expression for the first derivative of the implicit function in the fourth example?

    -The final expression for the first derivative in the fourth example is y' = (-2 - x - 6x^3y^2) / (3x^4y + y).

Outlines
00:00
📚 Introduction to Implicit Differentiation

This paragraph introduces the concept of implicit differentiation, a method used to differentiate functions that are not explicitly defined in terms of y(x). The video starts with a greeting and an overview of the topic. The presenter explains that when an equation is difficult to isolate y, implicit differentiation can be used to find the derivative of y with respect to x. The first example provided is an equation involving x^2, y^2, and linear terms in x and y, which is differentiated term by term with respect to x. The process involves applying the power rule and the chain rule where necessary, and then isolating y' (the derivative of y with respect to x) to find the first derivative of the implicit function.

05:02
🔍 Example 2: Differentiating a Cubic Function

The second paragraph presents the second example, which involves a cubic function in x and a squared function in y, equated to a constant. The presenter demonstrates the differentiation process by applying the power rule to x^3 and the constant differentiation to the rest of the terms. After differentiating, the presenter isolates y' and simplifies the equation to find the first derivative of the implicit function. The final answer is given as a simplified expression for the derivative of y with respect to x.

10:05
📈 Example 3: Chain Rule Application

In this paragraph, the presenter tackles a more complex example involving a cubed term with a chain rule application. The equation given has y and a cubic polynomial in x. The differentiation process is explained step by step, with a focus on the chain rule for the cubic term. The presenter multiplies the derivative of the outer function by the derivative of the inner function and simplifies the resulting expression. After isolating y', the final derivative of the implicit function is presented.

🧩 Example 4: Advanced Implicit Differentiation

The final example in the script is a more advanced case of implicit differentiation, involving a term with x raised to the fourth power multiplied by y squared. The presenter differentiates each term using the power rule and the product rule, carefully explaining the steps. After differentiating, the equation is simplified, and y' is isolated. The final answer is presented as a simplified expression for the derivative, showcasing the application of implicit differentiation in more complex scenarios.

Mindmap
Keywords
💡Implicit Differentiation
Implicit differentiation is a mathematical technique used to differentiate equations where the variable of interest is not explicitly expressed as a function of the independent variable. In the video's theme, this concept is central to finding the derivative of y with respect to x when y is given implicitly. The script provides examples of equations that are differentiated implicitly, such as 'x squared plus y squared minus 2x plus 3y equals four', where y is not isolated and thus requires implicit differentiation to find dy/dx.
💡Derivative
A derivative in calculus represents the rate at which a function changes with respect to its variable. In the context of the video, the derivative is used to find the rate of change of y with respect to x for various functions. The script mentions finding derivatives through implicit differentiation, such as 'differentiate x squared' resulting in '2x', which is part of the process to solve for dy/dx.
💡Power Rule
The power rule is a basic principle in calculus that allows for the differentiation of polynomial terms. It states that if you have a term of the form f(x) = x^n, where n is a constant, then its derivative f'(x) = n*x^(n-1). The video script applies the power rule when differentiating 'x squared' to get '2x' and 'x cubed' to get '3x squared', demonstrating its use in implicit differentiation.
💡Chain Rule
The chain rule is a fundamental theorem in calculus used to differentiate composite functions. It states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function. In the script, the chain rule is applied when differentiating '(2x - 5) cubed', where the derivative involves multiplying the derivative of the outer function (cubed) by the derivative of the inner function (2x - 5).
💡Product Rule
The product rule is a calculus theorem that allows for the differentiation of a product of two functions. It states that the derivative of a product uv is u'v + uv'. The video script uses the product rule when differentiating '3x to the fourth times y squared', where u = 3x to the fourth and v = y squared, resulting in '3x to the fourth times 2y plus y squared times 12x cubed'.
💡Isolation
Isolation in the context of differentiation refers to the process of rearranging an equation to solve for the derivative of the variable of interest. In the video, isolation is used to find dy/dx by rearranging the differentiated equation to get 'y prime' (dy/dx) by itself on one side of the equation, as seen when the script states 'isolate y prime' in various examples.
💡Differentiate
To differentiate, in mathematical terms, means to find the derivative of a function. The script repeatedly uses this term to describe the process of applying differentiation rules to each term in an equation to eventually find the derivative of y with respect to x. It is a key action in the script's examples, such as 'differentiate x squared' and 'differentiate y squared'.
💡Variable
In mathematics, a variable represents a value that can change. In the video, variables are used in equations to represent unknowns or quantities that can take on different values. The script deals with variables x and y, where y is the dependent variable whose derivative with respect to the independent variable x is being sought.
💡Constant
A constant is a value that does not change. In the context of differentiation, the derivative of a constant is zero. The script mentions constants when differentiating equations, such as 'the derivative of 16 is zero', indicating that constants do not contribute to the rate of change of the function.
💡Equation
An equation is a mathematical statement that asserts the equality of two expressions. In the video, equations are used to represent relationships between variables, and the process of implicit differentiation is applied to these equations to find the derivative of one variable with respect to another. Examples from the script include 'x squared plus y squared minus 2x plus 3y equals four'.
💡Example
The script provides several examples to illustrate the process of implicit differentiation. Each example is a different equation that requires differentiation with respect to x to find dy/dx. The term 'example' is used to introduce each new scenario, such as 'example number one', 'example number two', etc., where a unique equation is presented and differentiated.
Highlights

Introduction to the process of differentiating functions of y with respect to x.

Explanation of the difficulty in isolating y when the equation is not explicitly defined as a function of x.

Introduction of implicit differentiation as a method to find derivatives of implicit functions.

Example 1: Differentiating the equation x^2 + y^2 - 2x + 3y = 4.

Application of the power rule for differentiation in the first example.

Differentiating terms involving y with respect to x, including the use of y'.

Isolating y' in the first example to find the first derivative.

Example 2: Differentiating the equation x^3 - 3x + y^2 = 16.

Use of the power rule for x^3 and differentiation of constant terms in the second example.

Isolating y' in the second example to derive the first derivative.

Example 3: Differentiating the equation y + (2x - 5)^3 = 3.

Use of the chain rule for differentiating the term (2x - 5)^3 in the third example.

Isolating y' in the third example to find the first derivative.

Example 4: Differentiating the equation x^2 + 3x^4y^2 + y^2 = -4x.

Application of the product rule for differentiating 3x^4y^2 in the fourth example.

Isolating y' in the fourth example to derive the first derivative.

Final answer for the fourth example, simplifying the expression for y'.

Conclusion of the video with a summary of the process and an invitation for questions.

Transcripts

Browse More Related Video

Implicit Differentiation - More Examples

Implicit Differentiation for Calculus - More Examples, #1

Implicit Differentiation

Introduction to Implicit Differentiation

Implicit Differentiation Second Derivative Trig Functions & Examples- Calculus

Implicit Differentiation

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Implicit DifferentiationMath EducationDerivative CalculationProf DAlgebra TechniquesCalculus TutorialFunction AnalysisEducational ContentMath ChannelLearning Resource