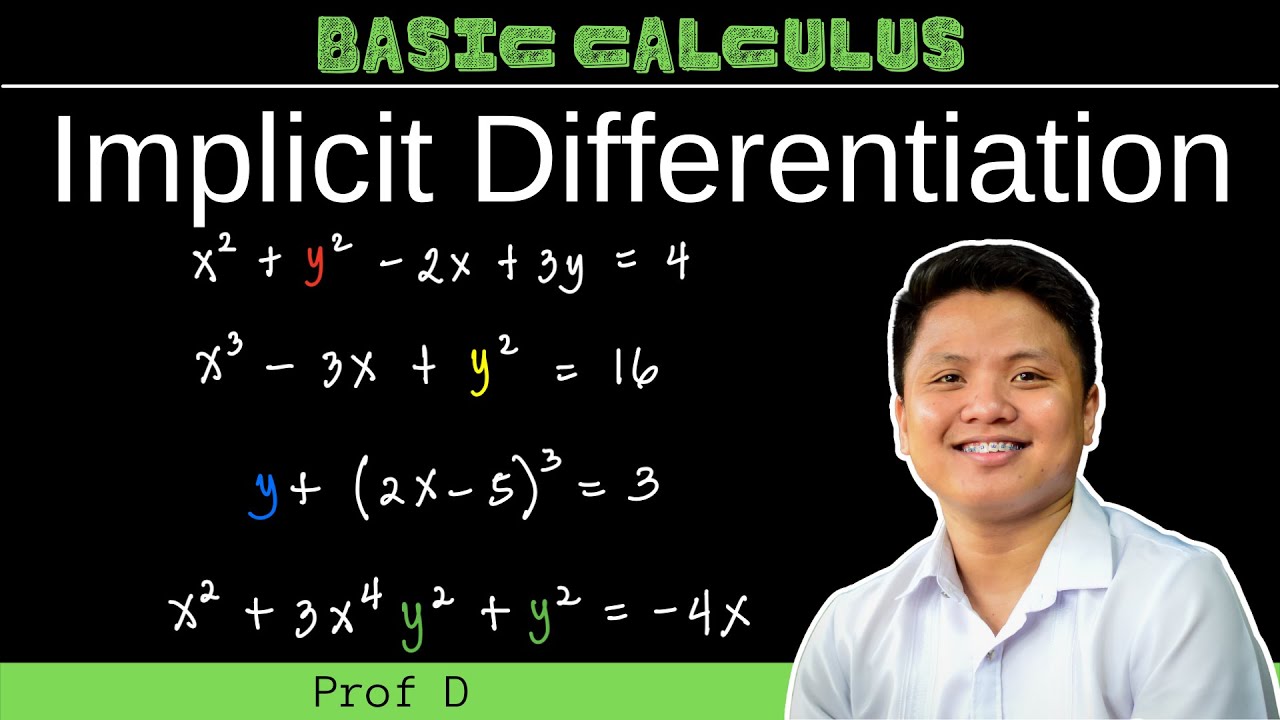
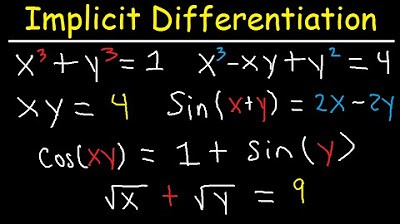Implicit Differentiation - More Examples

Sun Surfer Math
27 Feb 202209:44
EducationalLearning
32 Likes 10 Comments

TLDRThis video script focuses on the process of implicit differentiation, a method used to find the derivatives of functions where x and y are not explicitly separated. The speaker provides step-by-step examples, starting with a simple case where the derivative is found by applying basic differentiation rules and then multiplying by y' (the derivative of y with respect to x). The second example involves a more complex scenario with a product rule, which requires the use of f(x) and g(x) to represent parts of the equation, followed by a clean-up and simplification process to isolate y'. The third example also uses the product rule twice and demonstrates how to combine like terms and factor out y' to solve for the derivative. The script is an educational walkthrough that clarifies the often confusing concept of implicit differentiation, making it accessible to learners.

Takeaways
  • 📚 Implicit differentiation is used when x's and y's are on the same side of the equation, requiring us to differentiate both sides with respect to x.
  • 🔑 When differentiating y^2, we use the chain rule, resulting in 2y*(dy/dx), where dy/dx is the derivative of y with respect to x.
  • 🧮 The derivative of a constant, like 5 in the example, is 0, as constants do not change with respect to the variable.
  • ⚖️ To solve for dy/dx or y', we often need to rearrange the equation to isolate y' on one side.
  • 🔍 In the first example, y' simplifies to x/y after applying implicit differentiation and rearranging terms.
  • 🤔 For more complex equations, like products or quotients, we use specific rules such as the product rule, which is applied in the second example.
  • 🔄 When applying the product rule, we differentiate each part of the product separately and then combine them, considering the derivatives of both parts.
  • 📉 In the second example, after applying the product rule and combining like terms, we factor out y' to isolate and solve for it.
  • 📝 Simplifying complex fractions is a key step in the process, often achieved by multiplying the numerator and denominator by a common factor to simplify the expression.
  • 🔗 In the third example, we apply the product rule twice due to the nature of the equation, first to the term xy and then to the term y^2.
  • 🧹 Cleaning up the equation by distributing and combining like terms is crucial to solving for dy/dx or y'.
  • 🏁 The final step is to express the derivative in its simplest form, ensuring that all terms are properly factored and simplified.
Q & A

  • What is the derivative of x squared with respect to x?

    -The derivative of x squared (x^2) with respect to x is 2x.

  • How do you find the derivative of a dependent variable like y in an implicit function?

    -To find the derivative of a dependent variable like y, you multiply the derivative of y with respect to x (denoted as y' or dy/dx) by the derivative of the independent variable (x), which is 1 in this case.

  • What is the process to solve for y prime (dy/dx) in the given script?

    -To solve for y prime, you first take the derivative of both sides of the equation with respect to x, then isolate y prime on one side of the equation, and finally simplify the expression to find its value.

  • What is the role of the product rule in implicit differentiation?

    -The product rule is used when differentiating terms that are multiplied together. It states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function.

  • How is the derivative of 5 treated in differentiation?

    -The derivative of a constant, such as 5, is 0 because a constant does not change with respect to the variable being differentiated.

  • What is the simplified form of the derivative dy/dx for the equation x^2 - y^2 = 5?

    -After simplifying, the derivative dy/dx for the equation x^2 - y^2 = 5 is x/y.

  • How do you rewrite the equation xy - (1/3)x = 2x^(-1) to make it more appealing for differentiation?

    -The equation can be rewritten as x*y - x/3 = 2/x by moving x to the numerator and writing y/3 as y/(3x).

  • What is the derivative of the equation xy - (1/3)x = 2x^(-1) after applying the product rule?

    -The derivative involves applying the product rule to the term xy and then simplifying the expression, which results in a complex fraction that needs to be further simplified to isolate y prime.

  • What is the final simplified form of the derivative for the equation involving the product rule twice?

    -The final simplified form of the derivative is (2x - y - 10xy^2) / (10x^2y + x).

  • How do you factor out the common factor of y prime in a derivative expression?

    -You factor out y prime by dividing each term in the numerator and denominator by y prime, which consolidates the y prime terms and simplifies the expression.

  • What is the purpose of multiplying the numerator and denominator by 3x^2 in the derivative expression?

    -Multiplying by 3x^2 helps to eliminate the x^2 term in the denominator and simplify the expression, making it easier to solve for y prime.

  • What is the importance of simplifying the derivative expression after applying differentiation rules?

    -Simplifying the derivative expression is crucial for clarity and to express the derivative in its most understandable and usable form, which is essential for further analysis or application.

Outlines
00:00
📚 Implicit Differentiation Basics

This paragraph introduces the concept of implicit differentiation, explaining that it's used when the variables x and y are on the same side of the equation. The process involves taking the derivative of both sides of the equation and then solving for dy/dx, which represents the derivative of the dependent variable y with respect to the independent variable x. An example is given where the derivative of x^2 + y^2 = 5 is found to be dy/dx = x/y. The importance of multiplying by dy/dx when differentiating the dependent variable is emphasized.

05:00
🔍 Advanced Implicit Differentiation with Product Rule

The second paragraph delves into more complex examples of implicit differentiation, specifically using the product rule for terms that are multiplied together. The video script rewrites the equation xy - (1/3)x = 2x^(-1) to simplify the differentiation process. It applies the product rule by differentiating each part of the equation separately, combining terms with dy/dx, and then solving for dy/dx. The final simplified derivative is given as dy/dx = (-2/x^2 - 3y)/(3x - 1/3). Further simplification of the complex fraction is then demonstrated, resulting in a cleaner expression for the derivative.

Mindmap
Keywords
💡Implicit Differentiation
Implicit differentiation is a method used in calculus to find the derivative of an equation that does not explicitly express one variable in terms of another. It is central to the video's theme as it is the technique demonstrated throughout the transcript. For instance, the video begins with the statement 'these are implicit functions the x's and the y's are all on the same side of the equal sign,' illustrating the starting point for implicit differentiation.
💡Derivative
A derivative in calculus represents the rate at which a function changes with respect to a variable. It is a fundamental concept in the video, as the process of finding derivatives of given functions is the primary focus. For example, 'the derivative of x squared is simply, 2x' is a straightforward calculation of a derivative that is explained in the transcript.
💡Dependent Function
A dependent function is one that is defined in terms of another function or variable. In the context of the video, 'y' is referred to as the dependent function, as it is the function whose derivative is being sought. The concept is important as it is the subject of differentiation, as seen where 'y prime' is used to denote the derivative of the dependent variable 'y'.
💡Product Rule
The product rule is a fundamental rule in calculus for differentiating a product of two functions. It is used multiple times in the video to handle terms where two functions are multiplied together. For example, the script describes using the product rule for 'x y' and explains the steps to differentiate this product, which is a key part of the implicit differentiation process.
💡Chain Rule
The chain rule is a method for finding the derivative of a composition of functions. Although not explicitly named in the transcript, the concept is implicitly used when differentiating composite functions like 'y squared'. The chain rule is essential for handling nested functions within the broader process of implicit differentiation.
💡Differentiating with Respect to x
Differentiating with respect to x means finding the derivative of a function in terms of the variable x. This is a common phrase in calculus and is repeatedly emphasized in the video as the method for finding the derivatives of the given functions. It is exemplified in the script where it is stated 'we're looking for dy/dx', which means the derivative of y with respect to x.
💡Simplifying Expressions
Simplifying expressions is a process used to make complex mathematical expressions more manageable and easier to understand. It is a recurring theme in the video, as the speaker simplifies the results of differentiation to make them clearer. For instance, after applying the product rule, the speaker simplifies 'three x y prime minus one third y prime' to a more understandable form.
💡Factoring Out
Factoring out is a mathematical technique used to simplify expressions by extracting common factors. It is a key step in the process of solving for 'y prime' in the video. The script demonstrates factoring out 'y prime' from an equation to isolate it and solve for the derivative, as seen when the speaker factors out 'y prime' from 'three x y prime minus one third y prime'.
💡Complex Fraction
A complex fraction is a fraction where the numerator or the denominator contains another fraction. The video deals with complex fractions as part of the simplification process after differentiating. The script mentions simplifying a complex fraction 'negative two over x squared minus three y' by multiplying the numerator and denominator by '3x squared' to simplify the expression.
💡Distributing
Distributing is a mathematical operation that involves multiplying each term in a set by a given number or expression. It is used in the video when the speaker multiplies terms through brackets, such as 'multiplying the 5 through we have 10 x y squared, plus another 10 x squared y, y prime'. This operation is crucial for combining like terms and simplifying expressions.
💡Solving for y prime
Solving for y prime is the goal of the differentiation process demonstrated in the video. It involves isolating the derivative of the dependent variable 'y' with respect to 'x'. The script outlines this process multiple times, showing how to manipulate and simplify expressions to find 'y prime', which is the central objective of the implicit differentiation examples provided.
Highlights

Introduction to implicit differentiation and its application to functions where x's and y's are on the same side of the equation.

Derivative of x squared is 2x, and for y squared, it involves multiplying by y prime (dy/dx).

The process of solving for y prime by manipulating the equation and simplifying.

For the next example, a product rule is applied to a more complex function involving x and y.

Rewriting the function to make the application of the product rule clearer.

Using f(x) and g(x) to represent parts of the function for easier application of the product rule.

Combining like terms involving y prime and moving non-y prime terms to the other side of the equation.

Factoring out y prime to isolate and solve for it.

Simplifying the complex fraction by multiplying the numerator and denominator by 3x squared.

Cancelling out terms to simplify the derivative further.

Applying the product rule twice to another function involving x squared and a product of x and y.

Distributing terms and combining like terms with y prime on one side of the equation.

Factoring out y prime on the right-hand side to prepare for dividing both sides by it.

Dividing both sides of the equation by the expression involving x and y to isolate y prime.

Final expression for the derivative of the function after simplification.

Emphasis on the importance of multiplying the derivative of the dependent variable by y prime.

Explanation of how to handle the derivative of constants and the application of the power rule.

Guidelines for cleaning up and simplifying the derivative expressions after applying differentiation rules.

Transcripts

Browse More Related Video

Implicit Differentiation | Basic Calculus

Implicit Differentiation Second Derivative Trig Functions & Examples- Calculus

Implicit Differentiation

Implicit Differentiation

Implicit Differentiation for Calculus - More Examples, #1

Implicit Differentiation for Calculus - More Examples #2

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Implicit DifferentiationMath TutorialDerivative CalculationProduct RuleComplex FractionsAdvanced MathEducational ContentMathematical AnalysisCalculus TechniquesSolving EquationsLearning Math