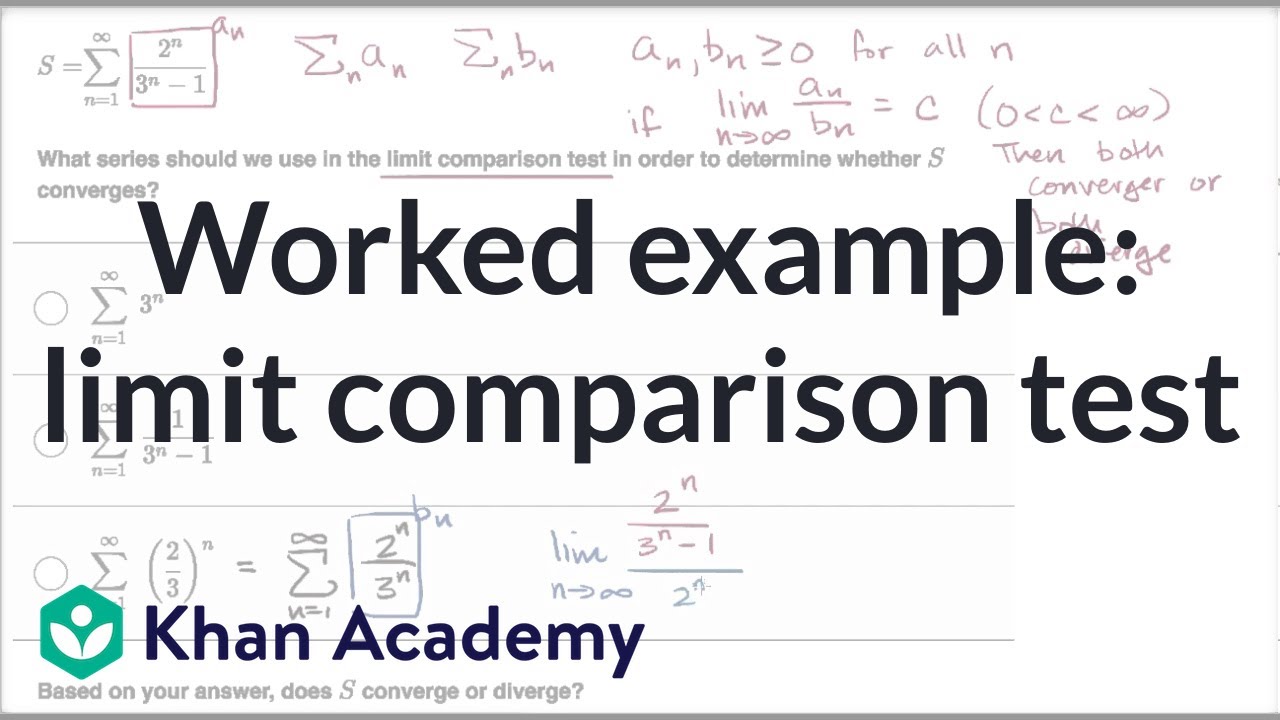
Lesson 19 - Comparison Tests (Calculus 2 Tutor)

Math and Science
18 Aug 201604:00
EducationalLearning
32 Likes 10 Comments

TLDRThis tutorial segment focuses on enhancing understanding of series convergence through the comparison test. After introducing the integral test in the previous section, which involves integrating the function equivalent to the series terms to determine convergence, the instructor acknowledges the difficulty of finding integrals by hand for some functions. The comparison test offers an alternative approach by comparing an unknown series to a known series with similar behavior. If the known series converges and the terms of the unknown series are less than or equal to the known series' terms for all n, then the original series also converges. This method is simple yet powerful for determining convergence when integrals are challenging to compute.

Takeaways
  • 📚 The video is part of an 'Advanced Calculus' tutorial focusing on understanding series convergence.
  • 🔍 The Integral Test is introduced as a method to determine if a series converges by integrating the function that represents the terms of the series.
  • 🚫 The Integral Test has limitations as some integrals are difficult to compute by hand and may require computer assistance.
  • 🆚 The Comparison Test is the main topic of the section, which involves comparing an unknown series to a known series to determine convergence.
  • 📉 The Comparison Test involves two series with positive terms, where one series is used to study and compare with the other.
  • 📈 Part A of the Comparison Test states that if a series \( b_n \) is convergent and \( a_n \leq b_n \) for all \( n \), then the series \( a_n \) also converges.
  • 📝 The terms \( a_n \) and \( b_n \) are underlined as important in the script, indicating their significance in the Comparison Test.
  • 📈 The script suggests using a visual representation, like a graph, to better understand the behavior of the series being compared.
  • 🤔 The Comparison Test requires specific conditions to be met, and if these can be shown, the test can be a powerful and simple tool.
  • 📚 The tutorial aims to develop skills in determining series convergence and provides a step-by-step approach to using the Comparison Test.
Q & A

  • What is the main focus of this section of the advanced calculus tutorial?

    -The main focus of this section is to develop skills in understanding when series converge and when they do not, specifically discussing the comparison test for series convergence.

  • What is the Integral Test mentioned in the script, and how does it relate to series convergence?

    -The Integral Test is a method where you take the integral of the function that resembles the terms in a series. If the integral converges, then the series also converges. It's a reliable way to determine convergence, although it may not always be practical if the integral is difficult to find by hand.

  • Why might the Integral Test not be helpful in some cases?

    -The Integral Test might not be helpful if the integrals are hard to find by hand or if they require a computer to calculate, making it impractical for determining series convergence when manual integration is not feasible.

  • What is the Comparison Test, and how does it work?

    -The Comparison Test involves comparing a series of interest (subscript n) with another related series (B subscript n) to determine if the original series converges. By comparing the behavior of the two series under certain conditions, one can infer the convergence of the original series.

  • What are the conditions for using the Comparison Test effectively?

    -The conditions for using the Comparison Test effectively include having both series with positive terms and ensuring that for all n, the terms of the series of interest (a subscript n) are less than or equal to the terms of the series used for comparison (B subscript n).

  • If the series B subscript n is convergent and the terms a subscript n are less than or equal to B subscript n for all n, what can be concluded about the series a subscript n?

    -If B subscript n is convergent and a subscript n is less than or equal to B subscript n for all n, then the series a subscript n also converges.

  • What is the significance of the term 'positive terms' in the context of the Comparison Test?

    -The term 'positive terms' is significant because it is a prerequisite for the Comparison Test. Both the series of interest and the series used for comparison must consist of positive terms to apply the test.

  • Can the Comparison Test be used if the series have negative terms?

    -No, the Comparison Test requires that both series have positive terms. Negative terms would not allow for a valid comparison under the conditions of this test.

  • What is the purpose of creating a related series (B subscript n) for comparison?

    -The purpose of creating a related series (B subscript n) is to have a reference series whose convergence is known or can be easily determined. This allows for a comparison with the series of interest (a subscript n) to infer its convergence.

  • How does the Comparison Test help in situations where the Integral Test is not practical?

    -The Comparison Test provides an alternative method to determine the convergence of a series when the Integral Test is not practical due to the difficulty of finding the integral by hand or the need for computational assistance.

Outlines
00:00
📚 Introduction to Series Convergence and Comparison Test

This paragraph introduces the topic of series convergence, focusing on when series converge and when they do not. It discusses the integral test as a method to determine convergence by integrating the equivalent function of the series terms. The speaker explains that while the integral test is reliable, it can be impractical if the integrals are difficult to calculate by hand. The paragraph then transitions into discussing the comparison test, which involves comparing an unknown series to a known series to determine convergence under certain conditions. The comparison test is presented as a simpler and more powerful alternative when direct integration is not feasible.

Mindmap
Keywords
💡Advanced Calculus
Advanced Calculus is a higher-level branch of mathematics that deals with the study of functions, limits, derivatives, and integrals. In the context of the video, it is the subject matter being taught, focusing on the convergence of series, which is a fundamental concept in understanding the behavior of infinite series. The script mentions 'advanced calculus to tutor,' indicating that the content is aimed at students who have a basic understanding of calculus and are looking to deepen their knowledge.
💡Series Convergence
Series Convergence refers to the property of an infinite series where the sum of its terms approaches a finite limit as the number of terms increases. The video script discusses methods to determine whether a given series converges or not, which is a central theme of the tutorial. The script mentions the comparison test as a means to understand convergence, indicating that it is a key concept in the study of series.
💡Integral Test
The Integral Test is a method used to determine the convergence of an infinite series by comparing it to the integral of its general term. The script introduces the integral test, stating that if the integral of the function converges, then the series does as well. This test is highlighted as a 'bulletproof' way to check for convergence, although it may not always be practical due to the difficulty of finding integrals by hand.
💡Comparison Test
The Comparison Test is a technique for assessing the convergence of a series by comparing it with another series whose convergence is already known. The script explains that by comparing the terms of the series we are interested in (a_sub_n) with those of a related series (b_sub_n), we can infer the convergence of the original series under certain conditions. This test is presented as a simple yet powerful tool in the analysis of series.
💡Positive Terms
Positive Terms refer to the elements of a series that are greater than zero. The script specifies that the comparison test is applicable when both series being compared have positive terms. This is an important condition because it ensures that the comparison between the series is meaningful and that the behavior of the series is not affected by negative values.
💡Convergent Series
A Convergent Series is an infinite series whose sum approaches a finite value as more terms are added. In the script, it is mentioned that if the series b_sub_n is convergent and the terms of the series we are studying (a_sub_n) are less than or equal to those of b_sub_n, then the original series also converges. This illustrates the use of a known convergent series to determine the convergence of another.
💡Related Series
Related Series are two or more series that share some common characteristics or are constructed in a way that allows for meaningful comparison. The script suggests creating a series (b_sub_n) that is related to the original series (a_sub_n) we want to study. By examining the behavior of the related series, we can gain insights into the convergence of the original series.
💡Behavior of Series
The Behavior of Series refers to how the terms of a series change as more terms are added, and how the partial sums approach a limit. The script discusses analyzing the behavior of a related series to understand the convergence of the series of interest. It implies that by observing the trends and limits of the related series, we can make inferences about the original series.
💡Underline
In the script, 'underline' is used as a directive to emphasize a particular point or condition. Specifically, it is used to highlight the condition that for all n, the terms a_sub_n must be less than or equal to b_sub_n for the comparison test to be valid. This visual emphasis in the script is meant to draw attention to a crucial aspect of the test.
💡XY Graph
An XY Graph, also known as a Cartesian coordinate graph, is a visual representation of data where values along one axis are plotted against values on another axis. In the script, the instructor mentions using an XY graph to illustrate the terms of the series and their behavior over time. This visual aid helps in understanding the comparison between the series and how their terms relate to each other.
Highlights

Introduction to the advanced calculus tutorial section on series convergence.

Continuation from the integral test for series convergence, which involves integrating the equivalent function of the series terms.

Limitations of the integral test due to the difficulty of finding integrals by hand for some functions.

Introduction of the comparison test as an alternative method to determine series convergence.

Explanation of the comparison test's premise: comparing an unknown series to a known series to infer convergence.

Requirement for both series in the comparison to have positive terms.

Part A of the comparison test: if a made-up series (B sub n) converges and is greater than or equal to the original series (a sub n) for all n, then the original series also converges.

Emphasis on the importance of the condition that B sub n must be convergent.

Illustration of the comparison test with a visual example on an XY graph.

The simplicity and power of the comparison test when the conditions are met.

The comparison test's utility in situations where integrals are hard to find by hand.

The process of making up a related series (B sub n) to study the behavior of the original series (a sub n).

The comparison test's reliance on specific conditions to be proven true for its application.

The significance of the comparison test in advanced calculus for determining the convergence of series.

The practical application of the comparison test in mathematical analysis.

The potential for the comparison test to simplify the process of determining series convergence compared to other methods.

Transcripts

Browse More Related Video

Direct comparison test | Series | AP Calculus BC | Khan Academy

Lesson 18 - Integral Test (Calculus 2 Tutor)

Worked example: limit comparison test | Series | AP Calculus BC | Khan Academy

Limit comparison test | Series | AP Calculus BC | Khan Academy

Series Tests - Limit Comparison Test

The Root Test

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Advanced CalculusSeries ConvergenceComparison TestIntegral TestMathematics TutorEducational ContentMath SkillsSeries AnalysisCalculus TechniquesEducational Video