Limit comparison test | Series | AP Calculus BC | Khan Academy

Khan Academy
2 Sept 201406:53
EducationalLearning
32 Likes 10 Comments

TLDRThis script delves into the Comparison Test for infinite series, illustrating its utility and limitations. It demonstrates how to prove convergence using a known convergent series as an upper bound. However, when the Comparison Test falls short, the Limit Comparison Test offers a solution for series with similar behaviors. The script provides a step-by-step application of the Limit Comparison Test, showing how to determine convergence by comparing the limit of terms from two series, ultimately concluding convergence based on a positive and finite limit.

Takeaways
  • 📚 The Comparison Test is a method used to determine the convergence of an infinite series by comparing it to another series with known convergence properties.
  • 🔍 To apply the Comparison Test, construct a series where each term is greater than the corresponding term in the original series.
  • 🔑 The series \( \sum_{n=1}^{\infty} \frac{1}{2^{n+1}} \) is used as an example, where it is shown that \( \frac{1}{2^n} \) is a suitable comparison series since it converges.
  • 🚫 The Comparison Test cannot be applied directly to the series \( \sum_{n=1}^{\infty} \frac{1}{2^n - 1} \) because \( \frac{1}{2^n} \) is not greater than \( \frac{1}{2^n - 1} \).
  • 🔎 As \( n \) becomes large, the behavior of the series is dominated by the term \( 2^n \), making the Comparison Test less applicable.
  • 🌐 The Limit Comparison Test is introduced as a broader tool that can be used when the Comparison Test is not applicable.
  • 📈 The Limit Comparison Test states that if the limit \( \lim_{n \to \infty} \frac{a_n}{b_n} \) is positive and finite, then both series converge or diverge together.
  • 🧩 Applying the Limit Comparison Test to the series \( \sum_{n=1}^{\infty} \frac{1}{2^n - 1} \) and \( \sum_{n=1}^{\infty} \frac{1}{2^n} \) shows that the limit is positive and finite, indicating convergence.
  • 📉 The limit calculation involves simplifying \( \frac{1}{2^n - 1} \) over \( \frac{1}{2^n} \) to show that it approaches 1 as \( n \) approaches infinity.
  • 📚 The geometric series \( \sum_{n=1}^{\infty} \frac{1}{2^n} \) is known to converge, and thus by the Limit Comparison Test, the series \( \sum_{n=1}^{\infty} \frac{1}{2^n - 1} \) also converges.
Q & A

  • What is the Comparison Test used for in the context of infinite series?

    -The Comparison Test is used to determine the convergence of an infinite series by comparing it to another series where the terms of the latter are greater than or equal to the terms of the former. If the latter series converges, the original series also converges.

  • Why can't the Comparison Test be applied directly to the series ∑(1/(2^n - 1))?

    -The Comparison Test cannot be applied directly to the series ∑(1/(2^n - 1)) because the terms of the series ∑(1/2^n) are not greater than or equal to the terms of ∑(1/(2^n - 1)). The terms of ∑(1/2^n) are actually less than the terms of ∑(1/(2^n - 1)), which means they cannot provide an upper bound.

  • What is the significance of the terms being positive in the context of the Comparison Test?

    -The terms being positive is significant because it ensures that the series is non-negative, which is a requirement for applying the Comparison Test. This allows for a valid comparison between the series terms.

  • What is the Limit Comparison Test and how does it differ from the Comparison Test?

    -The Limit Comparison Test is a method used to determine the convergence of two series by comparing their terms' ratio as n approaches infinity. It differs from the Comparison Test in that it does not require the terms of one series to be strictly greater than the other; instead, it focuses on the limit of their ratio.

  • What is the condition for the Limit Comparison Test to be applicable?

    -The Limit Comparison Test is applicable if the limit of the ratio of the terms of two series (a_n/b_n) as n approaches infinity is positive and finite. This indicates that either both series converge or both diverge.

  • How does the Limit Comparison Test help in determining the convergence of the series ∑(1/(2^n - 1))?

    -The Limit Comparison Test helps by comparing the series ∑(1/(2^n - 1)) with a known convergent series, such as ∑(1/2^n). By calculating the limit of the ratio of their terms as n approaches infinity, if the limit is positive and finite, it indicates that both series converge.

  • What is the limit of (2^n)/(2^n - 1) as n approaches infinity?

    -The limit of (2^n)/(2^n - 1) as n approaches infinity is 1. This is because the highest power of 2 in the denominator approaches 2^n, making the fraction approach 1.

  • Why is the limit of the ratio of the terms in the Limit Comparison Test important?

    -The limit of the ratio of the terms is important because it indicates the behavior of the series as n approaches infinity. A positive and finite limit suggests that the series have similar behaviors, and thus, they either both converge or both diverge.

  • What is a geometric series and why does it converge?

    -A geometric series is an infinite series where each term is a constant multiple of the previous term. It converges if the common ratio (r) is between -1 and 1 (excluding -1 and 1), ensuring that the terms approach zero.

  • How does the convergence of the geometric series ∑(1/2^n) relate to the convergence of ∑(1/(2^n - 1))?

    -Since the geometric series ∑(1/2^n) converges (because the common ratio 1/2 is less than 1), and the Limit Comparison Test shows that the limit of the ratio of the terms of ∑(1/(2^n - 1)) and ∑(1/2^n) is positive and finite, it implies that ∑(1/(2^n - 1)) also converges.

Outlines
00:00
📚 Introduction to the Comparison Test

This paragraph introduces the Comparison Test, a mathematical method used to determine the convergence of an infinite series. It explains that if a series can be compared to another series with known convergence properties, and if the terms of the unknown series are greater than or equal to the terms of the known series, then the unknown series also converges. The example given is the series '1/(2^(n+1))', which is compared to the convergent series '1/2^n'. The explanation highlights the importance of term comparison and the conditions under which the Comparison Test is applicable.

05:02
🔍 Limit Comparison Test and Its Application

The second paragraph delves into the Limit Comparison Test, which is an extension of the Comparison Test for cases where direct term comparison isn't feasible. The speaker discusses the conditions under which the Limit Comparison Test can be used, emphasizing that both series must have positive terms and that the limit of the ratio of corresponding terms must be positive and finite. The example provided involves comparing the series '1/(2^n - 1)' with '1/2^n', showing that as 'n' approaches infinity, the ratio of the terms converges to a positive finite value, indicating that both series converge. The explanation clarifies the mathematical process and the rationale behind the Limit Comparison Test.

Mindmap
Keywords
💡Comparison Test
The Comparison Test is a method used in mathematics to determine the convergence of an infinite series by comparing it to another series whose convergence is already known. In the video, the Comparison Test is initially used to prove the convergence of the series \( \sum_{n=1}^{\infty} \frac{1}{2^{n+1}} \) by comparing it to the known converging series \( \sum_{n=1}^{\infty} \frac{1}{2^n} \). The test is applicable when each term of the series being tested is greater than or equal to the corresponding term of the known series.
💡Infinite Series
An infinite series is the sum of the terms of an infinite sequence. The video discusses the convergence of such series, specifically focusing on series where terms are fractions with powers of two in the denominator. The series \( \sum_{n=1}^{\infty} \frac{1}{2^{n+1}} \) and \( \sum_{n=1}^{\infty} \frac{1}{2^n} \) are examples of infinite series discussed, where the behavior of the terms as \( n \) approaches infinity is crucial in determining their convergence.
💡Convergence
Convergence in the context of an infinite series refers to the property where the sum of the terms approaches a finite limit as the number of terms increases indefinitely. The video script discusses proving convergence using the Comparison Test and the Limit Comparison Test, highlighting that if a series converges, its sum can be thought of as approaching a certain value.
💡Limit Comparison Test
The Limit Comparison Test is a more general method for determining the convergence of an infinite series. It involves taking the limit of the ratio of corresponding terms from two series as \( n \) approaches infinity. If this limit is positive and finite, the series either both converge or both diverge. The video script applies this test to the series \( \sum_{n=1}^{\infty} \frac{1}{2^n} \) and \( \sum_{n=1}^{\infty} \frac{1}{2^{n-1}} \), showing that the limit of their ratio as \( n \) approaches infinity is finite and positive.
💡Terms
In the context of an infinite series, terms refer to the individual elements that are being summed. The video discusses how the terms of the series \( \sum_{n=1}^{\infty} \frac{1}{2^{n+1}} \) are greater than the terms of the series \( \sum_{n=1}^{\infty} \frac{1}{2^n} \), which is crucial for applying the Comparison Test.
💡Denominator
The denominator in a fraction is the number or expression below the line in a fraction. In the video, the behavior of the denominator in the series terms is analyzed, particularly how a larger denominator results in a smaller fraction, which is key to understanding the Comparison Test.
💡Geometric Series
A geometric series is a series where each term is a constant multiple of the previous term. The video mentions a geometric series with a common ratio less than one, which is known to converge. This is used as a reference series in the Limit Comparison Test to determine the convergence of another series.
💡Diverge
Divergent in the context of an infinite series means that the sum of the terms does not approach a finite limit as the number of terms increases indefinitely. The video script discusses the possibility of a series diverging based on the Limit Comparison Test, indicating that if the limit of the ratio of terms is not positive and finite, the series may not converge.
💡Limit
In mathematics, a limit refers to the value that a function or sequence approaches as the input approaches some value. The video script uses the concept of limits to discuss the Limit Comparison Test, where the limit of the ratio of terms from two series as \( n \) approaches infinity is crucial in determining their convergence or divergence.
💡Positive and Finite
Positive and finite in this context means that the value is greater than zero and not infinite. The video script emphasizes that for the Limit Comparison Test to be applicable, the limit of the ratio of terms must be positive and finite, indicating that both series being compared behave similarly as \( n \) approaches infinity.
Highlights

Introduction to the Comparison Test and its utility in determining the convergence of infinite series.

Explanation of when the Comparison Test might not be applicable.

Introduction of the Limit Comparison Test for broader applicability.

Demonstration of proving convergence using the Comparison Test with a series of 1/(2^(n+1)) terms.

Construction of a series with terms greater than the given series for comparison.

Use of the Comparison Test to infer convergence based on a known converging series.

Attempt to apply the Comparison Test to a different series with 1/(2^(n-1)) terms and the challenges faced.

Discussion on the dominance of exponential terms in series as n approaches infinity.

Introduction to the Limit Comparison Test with a formal definition.

Criteria for the Limit Comparison Test, including the positivity and finiteness of the limit.

Application of the Limit Comparison Test to the series with 1/(2^(n-1)) terms.

Calculation of the limit of the ratio of terms from the two series as n approaches infinity.

Conclusion that the series converges based on the Limit Comparison Test and the known convergence of a geometric series.

Explanation of the significance of a positive and finite limit in the Limit Comparison Test.

Clarification of the behavior of the series as n approaches infinity and the role of the exponential term.

Final conclusion that the series with 1/(2^(n-1)) terms also converges.

Transcripts

Browse More Related Video

Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test

Series Tests - Limit Comparison Test

Tests for Convergence

AP Calculus BC Lesson 10.6 Part 2

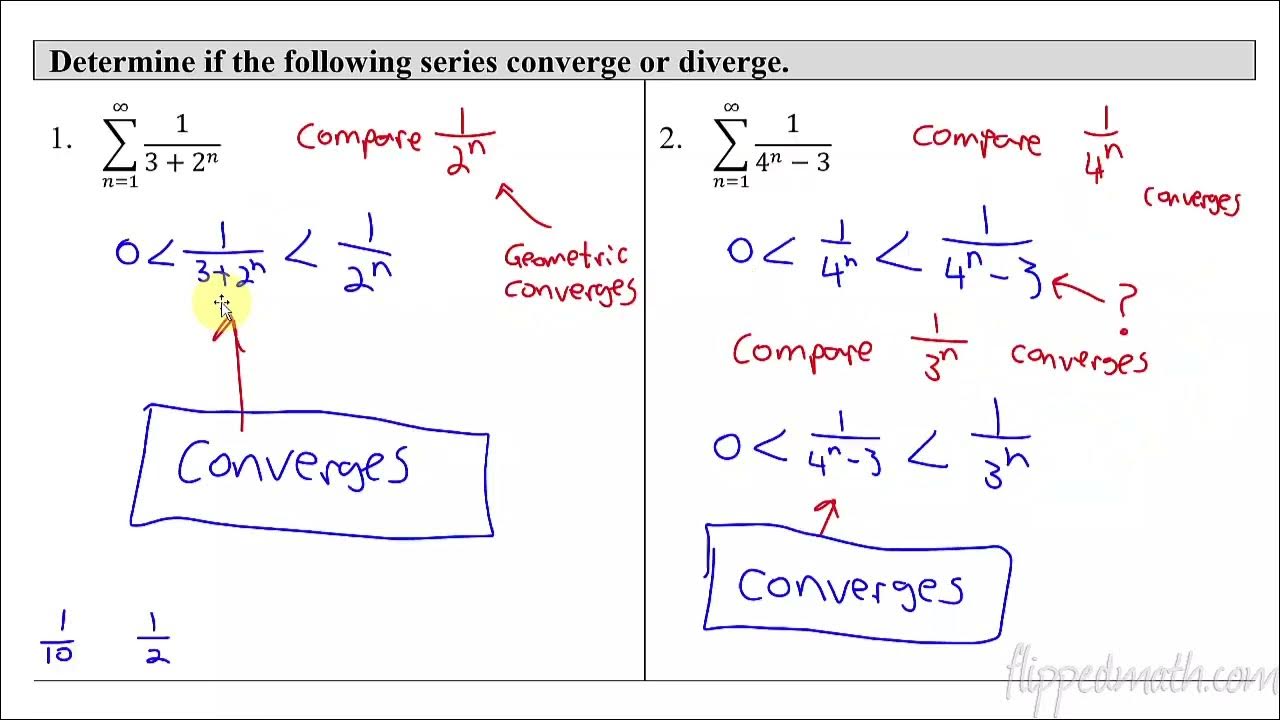
Calculus BC – 10.6 Comparison Tests for Convergence

Worked example: limit comparison test | Series | AP Calculus BC | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Comparison TestSeries ConvergenceLimit ComparisonMathematicsInfinite SeriesGeometric SeriesUpper BoundConvergence AnalysisEducational ContentMath Tutorial