Lesson 18 - Integral Test (Calculus 2 Tutor)

Math and Science
18 Aug 201604:00
EducationalLearning
32 Likes 10 Comments

TLDRIn this advanced calculus tutorial, the focus is on series convergence, specifically introducing the integral test. The instructor explains the concept of sequences and their convergence, which is essential for understanding series. Series are the sum of an infinite sequence of numbers, and this section aims to define tests that can determine if a series converges or diverges. The analogy between sequences and functions is highlighted, suggesting that calculus knowledge can be applied to assess series convergence. The integral test is presented as a method to evaluate the convergence of a series by comparing it to the integral of its terms, leveraging the summation nature of integrals.

Takeaways
  • 📚 The lecture is part of an advanced calculus course focusing on series and their convergence.
  • 🔍 The series convergence is a critical topic, discussing methods to determine if a series converges or diverges.
  • 📈 The integral test is introduced as a specific method to examine the convergence of a series in the upcoming sections.
  • 🔑 A series is defined as the sum of an infinite sequence of numbers, which is the main focus of the section.
  • 📉 The goal is to determine if the infinite sum of a series converges to a number or diverges to infinity.
  • 📝 The analogy between sequences and functions is highlighted, suggesting that calculus concepts can be applied to series convergence.
  • 📊 The integral is likened to a summation, summing an infinite number of infinitesimal parts, which is a key concept in understanding series convergence.
  • 📐 The integral is related to the area under a curve, which is a visual representation of summing up infinitesimally small parts of a function.
  • 📈 The integral test will be used to apply calculus knowledge to determine the convergence of a series, even though a series is not a function.
  • 🔬 The lecture suggests that while series and functions are not the same, the concepts from calculus of functions can be used by analogy to analyze series.
Q & A

  • What is the main focus of the section on advanced calculus?

    -The main focus of the section is to discuss series, specifically how they converge, and to introduce various tests or methods to determine the convergence of a series.

  • What is a sequence in the context of calculus?

    -A sequence in calculus is a function whose domain is the set of natural numbers. It is a series of numbers that can be plotted on a graph as discrete points corresponding to each element in the sequence.

  • What is the difference between a sequence and a series?

    -A sequence is a function that maps each natural number to a real number, while a series is the sum of the terms of a sequence. A series involves adding an infinite number of terms from the sequence.

  • What is the purpose of defining tests for series in calculus?

    -The purpose of defining tests for series is to provide tools to determine whether a given series converges to a finite sum or diverges to infinity.

  • How does the concept of a function relate to a sequence or series?

    -Although a series is not a function, it can be visualized on a graph similar to a function, with points representing the terms of the sequence. This analogy allows us to apply calculus concepts to analyze the convergence of a series.

  • What is the integral test mentioned in the script?

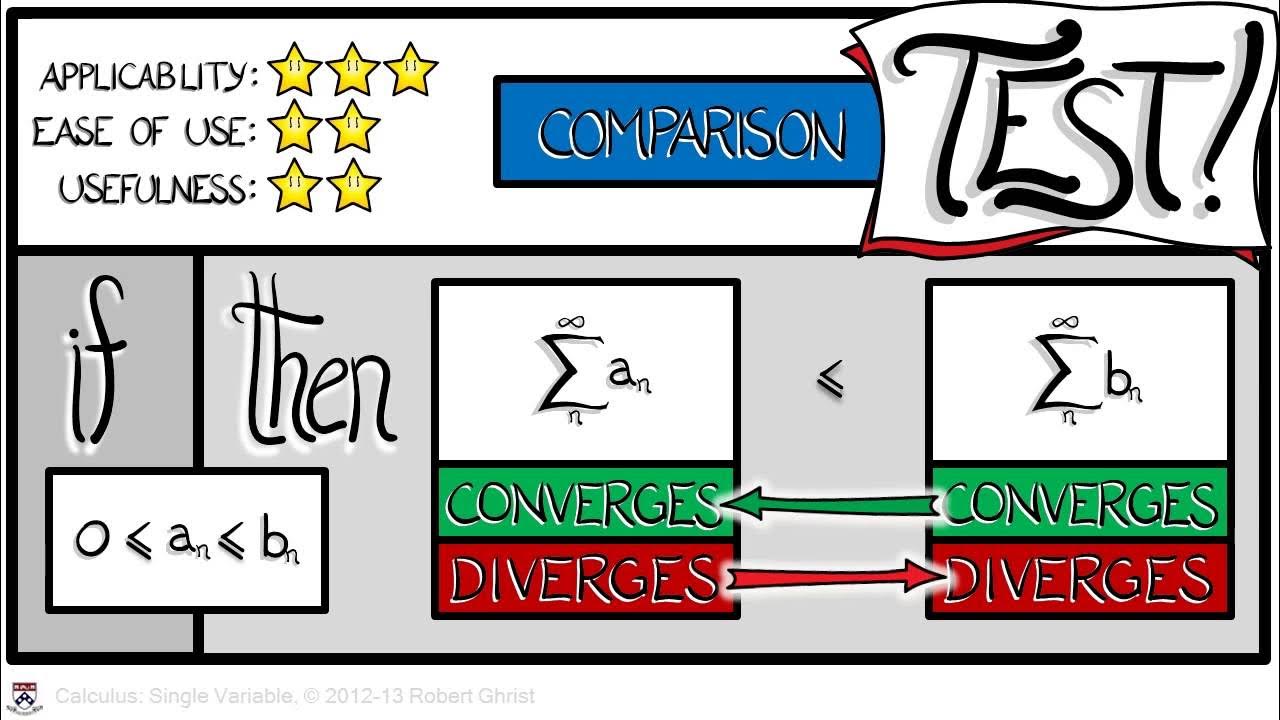
    -The integral test is a method used to determine the convergence of an infinite series by comparing it to an improper integral. If the integral converges, under certain conditions, the series also converges.

  • What is the significance of the integral in calculus?

    -The integral in calculus is a fundamental concept representing the summation of an infinite number of infinitesimally small quantities, often used to calculate areas, volumes, and total quantities.

  • How does the concept of an improper integral relate to the convergence of a series?

    -An improper integral can be used to test for the convergence of a series by comparing the series to the integral of its general term. If the integral converges, it provides evidence that the series may also converge.

  • What is the role of the differential 'dx' in the context of an integral?

    -The differential 'dx' represents an infinitesimally small change in the variable of integration. It is used in the integral to denote the small portions of the function that are being summed up.

  • How does the area under a curve relate to the concept of an integral?

    -The area under a curve can be thought of as the sum of an infinite number of infinitesimally thin vertical strips. This is analogous to the integral, which sums up the function values over infinitesimally small intervals of x.

  • What is the analogy made between a series and a function in the script?

    -The analogy made is that when you plot the terms of a sequence as discrete points on a graph, it resembles a function. However, it's important to note that a series is not a function because it is composed of discrete points rather than a continuous curve.

Outlines
00:00
📚 Introduction to Series Convergence and the Integral Test

This paragraph introduces the topic of advanced calculus, specifically focusing on series and their convergence. The speaker explains the concept of a sequence and how it relates to a series, which is the sum of an infinite number of terms. The main goal of the section is to define various tests to determine whether a series converges or diverges. The analogy between a sequence plotted on a graph and a function is made, emphasizing that while they are not the same, the calculus of functions can be applied to understand the convergence of a series. The integral test is introduced as the first method to be explored in the subsequent sections.

Mindmap
Keywords
💡Advanced Calculus
Advanced Calculus refers to the study of mathematical analysis that extends beyond the basic concepts taught in introductory calculus courses. It typically includes topics such as series, sequences, functions of several variables, and more complex integration techniques. In the context of the video, Advanced Calculus is the overarching subject, with the focus on understanding the convergence of series, which is a fundamental concept in this field.
💡Series
A series in mathematics is the sum of the terms of a sequence. It is represented as an infinite sum, where each term is added to the previous ones. In the video, the concept of series is central to the discussion, as the tutor is explaining how to determine whether a series converges to a finite value or diverges to infinity.
💡Convergence
Convergence, in the context of sequences and series, refers to the property of approaching a certain value or behavior as the terms of the sequence or series increase indefinitely. The video discusses various tests to determine the convergence of a series, which is key to understanding if the sum of an infinite series will result in a finite number.
💡Integral Test
The Integral Test is a method used to determine the convergence of an infinite series by comparing it to an improper integral. The video introduces the Integral Test as a specific technique to be explored in the subsequent sections, which allows one to use calculus to assess the convergence of a series.
💡Sequence
A sequence is an ordered list of numbers, which can be finite or infinite. In the video, sequences are mentioned as a precursor to series, where each term of the sequence is added together to form a series. The behavior of a sequence as it progresses is crucial in understanding the convergence of the series it forms.
💡Limit
In mathematics, a limit is the value that a function or sequence approaches as the input or index approaches some value. The concept of a limit is fundamental when discussing convergence, as it helps to determine whether a sequence or series approaches a finite value. The video script mentions limits in the context of sequences marching towards a limit.
💡Function
A function is a mathematical relationship between two sets that assigns exactly one output to each input. While a series is not a function, the video script draws an analogy between the two, suggesting that the behavior of a sequence can be visualized similarly to a function, which helps in understanding the convergence of a series.
💡Infinitesimal
An infinitesimal is a quantity that is closer to zero than any standard real number. In calculus, infinitesimals are used to describe the infinitesimally small changes in variables, such as dx in an integral. The video script uses the concept of infinitesimals to explain how integrals represent the summation of an infinite number of infinitesimally small quantities.
💡Area Under the Curve
The area under the curve is a geometric interpretation of the definite integral. It represents the total accumulated value over a specified interval. In the video, the area under the curve is mentioned as a way to visualize the summation process in integrals, which is analogous to summing terms in a series.
💡Differential
A differential, often denoted by 'dx' or 'dy', is a mathematical concept representing an infinitesimally small change in a variable. In the context of the integral test discussed in the video, differentials are used to express the small increments of the variable over which the function is being integrated.
Highlights

Introduction to the advanced calculus section focusing on series convergence.

Explanation of the concept of series as the sum of an infinite number of terms in a sequence.

The goal of defining tests to determine if a series converges or diverges.

Recap on the concept of sequences and their convergence towards a limit.

Series convergence as adding up the elements of a sequence to see if it approaches a finite value.

Introduction of the integral test as a method to assess series convergence.

Analogous relationship between sequences plotted on a graph and functions.

The integral as a summation of an infinite number of infinitesimal parts, represented by DX.

Integral's equivalence to the area under a curve due to the summation of infinitesimal areas.

The integral test's relevance to series convergence despite the difference between series and functions.

The integral as a summation process over differential values of x in calculus.

The importance of using calculus knowledge to apply to the convergence of a series.

The integral test's big picture approach to understanding series convergence.

The integral test's foundation on the definition of the integral from calculus 1.

The integral test's application to determine if a series converges by summing function values over differential x.

The integral test's utility in the tool bag of methods to assess series convergence.

Transcripts

Browse More Related Video

Lesson 19 - Comparison Tests (Calculus 2 Tutor)

Calculus 2 Lecture 9.3: Using the Integral Test for Convergence/Divergence of Series, P-Series

Lesson 21 - Ratio And Root Test (Calculus 2 Tutor)

Infinite Series Multiple Choice Practice for Calc BC (Part 6)

AP Calculus BC Lesson 10.1

Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Advanced CalculusSeries ConvergenceIntegral TestMathematics TutorSequence AnalysisInfinite SumsCalculus TechniquesFunction AnalogyConvergence TestsEducational Content