Derivative By First Principle Method | Derivative Formula Trick

mathmontrealmath
28 Jul 202207:17
EducationalLearning
32 Likes 10 Comments

TLDRThe video script appears to be an educational discussion about limits in calculus, specifically focusing on the concept of limits as a variable 's' approaches zero. The speaker guides the audience through a step-by-step process of evaluating a limit involving a function with 'x' and 's'. The explanation covers substituting values into the function, handling terms with 'x' and 's', and simplifying expressions by canceling out common factors. Emphasis is placed on the importance of correctly substituting 'x + s' into the function and understanding how the change in 'x' affects the result. The presenter also touches on verifying the result by taking the derivative of the given function, highlighting the practical application of the concept in calculus. The script is instructional and seems aimed at demystifying a potentially confusing topic for learners.

Takeaways
  • 📘 The process involves plugging in 's' for every 'x' in a given mathematical expression to find the limit as 's' approaches zero.
  • 🔍 When faced with an expression like 'x + s', it's important to recognize that 's' represents a small change in 'x'.
  • 📌 The 'h' in the expression stands for a small change, and it should be copied exactly as it appears in the original expression.
  • 🔢 In the context of the script, 'h7' would mean that the number '7' is directly placed in the expression where 'h' is.
  • 🎓 For terms involving 'x', if there's no specific number, use 'x + s' or 'x' depending on the context of the problem.
  • 🚫 It's crucial not to simplify or combine terms prematurely, especially when dealing with a cube term that requires special attention.
  • 🧐 Understanding the origin of each term in the expression is key to correctly substituting and simplifying the equation.
  • 🛄 After substituting, the next step is often to remove brackets and simplify the equation step by step.
  • ✅ Cancellations occur when like terms are present on either side of a subtraction or addition operation.
  • 🔧 The final answer is obtained by simplifying the expression until all 's' terms cancel out, leaving behind the desired value.
  • ➗ At the end, when 's' approaches zero, any term with 's' in the denominator becomes undefined, so 's' is replaced with zero.
  • 🔗 The result can be verified by taking the derivative of the original function, which should match the derived expression.
Q & A

  • What is the process described in the transcript?

    -The process described in the transcript is a step-by-step explanation of how to calculate a limit as 's' approaches zero in a mathematical expression involving 'x' and 's'.

  • What does the term 'limit' in the context of this transcript refer to?

    -In the context of this transcript, 'limit' refers to a fundamental concept in calculus where one evaluates the value that a function approaches as the input (in this case 's') approaches a certain value, here zero.

  • What is the significance of the term 'h' in the transcript?

    -The term 'h' in the transcript represents a small change in the variable 'x'. It is used to illustrate how the function behaves as 'x' changes by a small amount 'h'.

  • How does the speaker address the confusion regarding the 's' in the expression?

    -The speaker clarifies that 's' should be substituted with 'x + s' because it is related to the first 'h', and both terms involving 'x' are affected by this change.

  • What is the rule mentioned for handling the cube term in the expression?

    -The rule mentioned for handling the cube term is to not interfere with it until the last step, implying that it should be treated separately during the initial stages of the calculation.

  • What does the speaker mean by 'remove the brackets'?

    -The speaker is referring to the process of simplifying the expression by removing parentheses, which allows for easier manipulation and calculation of the limit.

  • How does the speaker simplify the expression after removing the brackets?

    -The speaker simplifies the expression by distributing the negative sign and canceling out terms, such as the negative 'x' canceling out the positive 'x' in the expression.

  • What is the final step in finding the limit as described in the transcript?

    -The final step is to substitute 's' with zero, as the limit is approached as 's' goes to zero, and then simplify the expression to find the final answer.

  • How can one verify the result of the limit calculation?

    -The result of the limit calculation can be verified by taking the derivative of the original function and checking if it matches the derived limit expression.

  • What does the term 'sns' in the transcript refer to?

    -The term 'sns' in the transcript refers to the process of canceling out the 's' terms in the expression, as every term has an 's' that can be simplified or canceled out.

  • Why is it important to follow the order of operations when calculating the limit?

    -Following the order of operations is important to ensure the accuracy of the limit calculation, as it dictates the sequence in which mathematical operations should be performed.

  • What does the speaker emphasize about the process of calculating the limit?

    -The speaker emphasizes the importance of understanding the process and the reasoning behind each step, rather than just memorizing the steps, to truly grasp the concept of limits in calculus.

Outlines
00:00
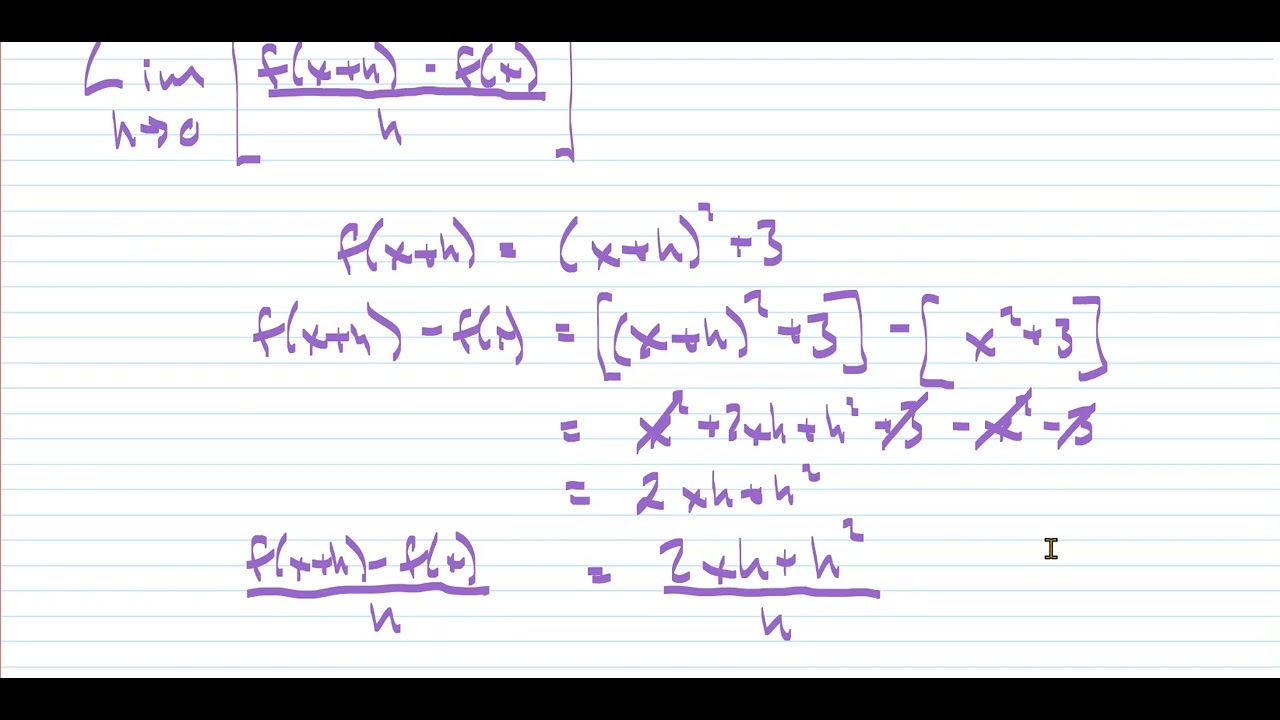
🧮 Calculating Limits with Variables

The first paragraph discusses the process of calculating limits in a mathematical context, specifically when the variable 's' approaches zero. The speaker guides through substituting 's' into an expression involving 'x' and 's', emphasizing the importance of correctly identifying the terms that involve 's' for substitution. The paragraph also touches on the concept of derivatives and how they can be used to verify the result of the limit calculation. The process involves simplifying expressions and understanding how terms change as 's' approaches zero.

05:05
📉 Simplifying and Solving Limits

The second paragraph continues the mathematical theme, focusing on simplifying expressions after substituting variables to solve a limit problem. The discussion involves the cancellation of terms and the transformation of expressions, such as a cube becoming a square, as 's' approaches zero. The speaker emphasizes the final step of substituting 's' with zero to find the limit's value. Additionally, the paragraph suggests verifying the result by taking the derivative of the original expression and comparing it to the simplified form obtained from the limit calculation.

Mindmap
Keywords
💡Limit
In the context of the video, 'limit' refers to a fundamental concept in calculus that describes the value that a function or sequence approaches as the input (often denoted as 's' or 'h' in the script) approaches a particular point. The script discusses 'limit, s goes to zero', which is a common scenario in calculus when finding the derivative of a function, illustrating the process of evaluating the limit as the change in the input (s) approaches zero.
💡Cube
The term 'cube' in the video is used to describe the mathematical operation of raising a number to the power of three. It is part of the expressions being manipulated, such as 'minus this cube', indicating the process of dealing with polynomial terms where an unknown quantity is raised to the third power.
💡Derivative
The 'derivative' is a concept from calculus that represents the rate at which a function changes with respect to a change in its input variable. The video script alludes to the process of taking the derivative of a function, which is central to the discussion. For example, the script mentions 'if you do the derivative of this', highlighting the application of differentiation in finding the instantaneous rate of change.
💡Plug in
'Plug in' is a common mathematical phrase used to substitute a specific value into an expression to evaluate it. In the script, phrases like 'so plug this in every x you see' refer to the process of substituting the variable 'x' with particular values to simplify the expression or to find a specific limit.
💡Simplify
Simplification in mathematics involves reducing a complex expression to a more straightforward form. The script emphasizes the importance of simplification with phrases like 'simplification happens', indicating the process of making the mathematical expression easier to understand or work with by combining like terms or reducing it to its most basic components.
💡Brackets
In the context of the video, 'brackets' likely refer to parentheses used in mathematical expressions to group terms together. The script discusses 'remove the brackets', which is a step in simplifying an expression by eliminating the grouping of terms that the parentheses create.
💡Cancel
The term 'cancel' in mathematics is used when two opposite terms (like +x and -x) in an expression are combined to result in zero. The script uses the phrase 'that one cancels' to describe this process, which is a common simplification technique where terms are eliminated from the expression because they sum to zero.
💡Direct derivative
A 'direct derivative' refers to the straightforward application of differentiation rules to find the derivative of a function without resorting to more complex techniques. The script suggests 'verify by deriving, by direct derivative', which means to confirm the result of a limit or a derivative by applying the basic rules of differentiation directly.
💡Verify
Verification in mathematics is the process of confirming that a result or solution is correct. In the script, the speaker talks about 'verifying' the result of a derivative by comparing it with the expected outcome, which is a crucial step in mathematical problem-solving to ensure accuracy.
💡Change
The concept of 'change' in the video is related to the idea of how a function's value changes as its input variable changes. It is particularly relevant when discussing limits, as the script mentions 'the change happens only in x', referring to the process of evaluating how the function behaves as the input variable approaches a certain value.
💡Zero
In the context of limits, 'zero' often represents the value that a sequence or function approaches. The script uses 'zero' in the phrase 'limit, s goes to zero', which is a common way to describe the behavior of a function as the input gets arbitrarily close to zero without actually being zero.
Highlights

The process begins with plugging in 's' for every 'x' to evaluate the limit as 's' approaches zero.

When faced with an expression like 'x plus s', it's important to recognize that 's' represents a change in 'x'.

The concept of 'h' is introduced as a specific value that should be copied exactly from the expression.

The term 'h7' is used to illustrate that if 'h' were 7, it would be placed directly in the expression.

The discussion emphasizes the importance of understanding the origin of terms in the expression, such as 'x plus s'.

The transcript explains that the change in the expression occurs due to the variable 'x' and its relation to 'h'.

The process of removing brackets is detailed to simplify the expression for further manipulation.

The rule of not interfering until the last step is mentioned, emphasizing the importance of following a structured approach.

The concept of '3n' is mentioned, suggesting a memorized rule or formula that aids in the simplification process.

The transcript illustrates how to cancel out terms in the expression by correctly applying the rules of algebra.

The importance of verifying the final answer by taking the derivative of the original expression is highlighted.

The final answer is obtained by simplifying the expression to a form where 's' cancels out, leaving a function of 'x'.

The process concludes with the evaluation of the limit as 's' approaches zero, resulting in a simplified expression.

The transcript demonstrates the practical application of mathematical concepts in deriving and simplifying expressions.

The use of color coding in the explanation helps to distinguish different parts of the expression and their manipulation.

The discussion emphasizes the need to understand the relationship between variables and how they change within the expression.

The transcript provides a step-by-step guide on how to approach and solve complex mathematical problems involving limits.

The concept of verifying the solution by direct derivative is introduced as a method to ensure the correctness of the solution.

Transcripts

Browse More Related Video

Limit examples (part 1) | Limits | Differential Calculus | Khan Academy

Applying the Definition of the Derivative to 1/x

Building up to computing limits of rational functions

Definition of the Derivative Review

Limits of functions | Calculus

Finding Derivative Using Limit Definition

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusLimitDerivativeMathematicsEducationDiscussionLearningSolvingConceptualInteractive