Calculus - Lesson 16 | Indefinite and Definite Integrals | Don't Memorise
TLDRThis educational video script explores the concept of integration as the reverse process of differentiation. It demonstrates how to find the integral of a function by identifying an antiderivative, which is a function whose derivative matches the original function. The script uses the example of finding the integral of 'x squared' by deriving 'x cubed over 3', and explains that adding a constant does not change the derivative, thus any function of the form 'x cubed over 3 + C' is an antiderivative. It distinguishes between indefinite integrals, which include a constant, and definite integrals, which provide a numerical value by evaluating the antiderivative at specific limits. The script challenges viewers to find the indefinite integral of a new function 'H of X' equal to 'x squared plus 6', encouraging active learning and engagement.
Takeaways
- 📚 The fundamental concept of integration is that it is the reverse process of differentiation.
- 🔍 The integral of the derivative of a function f(x) is equal to the difference in the function f(x) evaluated at the limits of integration.
- 🤔 The video aims to teach how to apply this principle to find the integral of a function.
- 📈 The process involves finding a function G(x) such that its derivative is the function g(x) for which we want to find the integral.
- 🧩 By finding G(x), the integral of g(x) is the difference between G(x) evaluated at the limits of integration.
- 🔑 The derivative of a function f_1(x) = x is 1, and f_2(x) = x^2 has a derivative of 2x, indicating a pattern where the power of x decreases by 1 with each derivative.
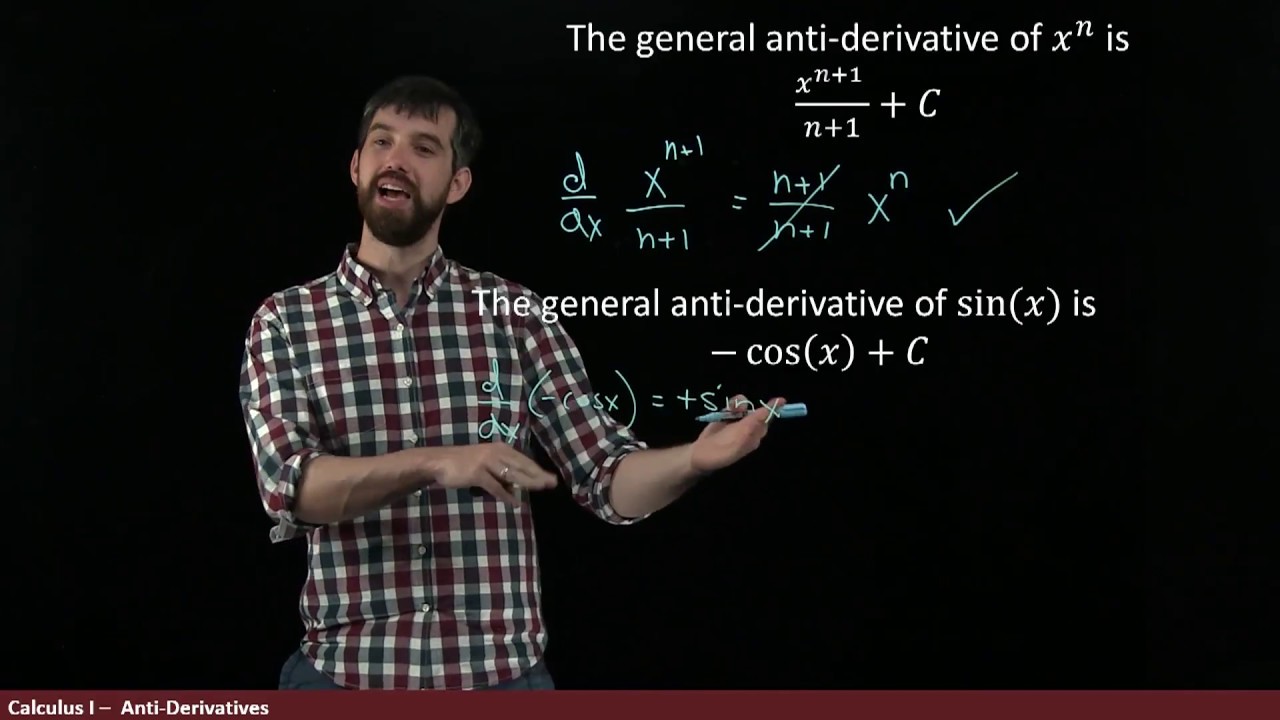
- 🎯 To find a function whose derivative is x^2, consider f_3(x) = x^3 and adjust it to G(x) = x^3 / 3 to get the desired derivative.
- 🤹 Adding a constant to an antiderivative does not change its derivative, thus any function G(x) + C is also an antiderivative of g(x).
- 📉 The indefinite integral of a function is represented by the integral symbol without limits and includes a constant C.
- 📊 The definite integral is found by evaluating the indefinite integral at the specified limits of integration, yielding a numerical answer.
- 📝 The process of finding the definite integral involves first finding the indefinite integral, then applying the limits to get the specific value.
Q & A
What is the fundamental relationship between integration and differentiation?
-Integration is the reverse process of differentiation. The integral of the derivative of a function f(x) is equal to the function f(x) itself, evaluated at the limits of integration.
How can we find the integral of a function G(x) from A to B?
-To find the integral of G(x) from A to B, we first find a function G(x) such that its derivative is G(x), and then evaluate the difference between the values of G(x) at the limits A and B.
What is the process of finding a function whose derivative is a given function G(x)?
-The process involves finding an antiderivative of G(x), which is a function G(x) such that its derivative is equal to G(x).
Why do we need to consider a function G(x) when finding the integral of G(x)?
-We need to consider a function G(x) because it represents the antiderivative of G(x), which is necessary to evaluate the definite integral from A to B.
How does the power of x change when we take the derivative of a function?
-Each time we take the derivative of a function, the power of x is reduced by 1.
What function should we consider if we want the derivative to be equal to 'x squared'?
-We should consider the function 'x cubed over 3' because its derivative is 'x squared'.
What is the effect of adding a constant to an antiderivative function on its derivative?
-Adding a constant to an antiderivative function does not affect its derivative, as the derivative of a constant is zero.
Why can we use any antiderivative of G(x) to find the value of the integral?
-We can use any antiderivative of G(x) because the constant added to the antiderivative does not affect the value of the definite integral, which is the difference between the function values at the limits of integration.
What is the general form of the indefinite integral of a function G(x)?
-The general form of the indefinite integral of a function G(x) is G(x) plus a constant C, denoted as ∫G(x)dx = G(x) + C.
How is the definite integral different from the indefinite integral?
-The definite integral involves evaluating the antiderivative at specific limits of integration and results in a numerical value, whereas the indefinite integral is a function and does not have specific limits.
What is the process to find the indefinite integral of a function H(x) = x squared plus 6?
-To find the indefinite integral of H(x) = x squared plus 6, we find a function H(x) such that its derivative is equal to H(x), and then add a constant C to it, resulting in H(x) + C.
Outlines
📚 Understanding Integrals and Antiderivatives
The script introduces the concept of integrals and their relationship with derivatives. It explains that the integral of a derivative of a function is equal to the function itself, evaluated at the limits of integration. The video aims to show how to find the integral of a function 'G of X' by finding a function 'capital G of X' whose derivative is 'G of X'. The process involves identifying a function that, when differentiated, yields 'x squared', which is found to be 'X cubed over 3'. The video also discusses the impact of adding a constant to the antiderivative and how it does not change the value of the integral, highlighting the concept of indefinite integrals and their notation.
🔍 The Process of Finding Indefinite and Definite Integrals
This paragraph delves deeper into the process of finding integrals, focusing on the difference between indefinite and definite integrals. It clarifies that any function which is 'G of X' plus some constant is an antiderivative of 'G of X'. The indefinite integral is represented by 'capital G of X plus a constant C' and is denoted without limits of integration. The definite integral, on the other hand, is found by evaluating the indefinite integral at the limits of integration, yielding a numerical value. The paragraph also reviews the steps to find the definite integral of a function, emphasizing the importance of first finding its indefinite integral. Finally, the script challenges the viewer to find the indefinite integral of a new function 'H of X' equal to 'x squared plus 6' and encourages engagement by asking for answers in the comments section.
Mindmap
Keywords
💡Integral
💡Differentiation
💡Antiderivative
💡Definite Integral
💡Indefinite Integral
💡Limits of Integration
💡Derivative
💡Rate of Change
💡Function
💡Constant of Integration
Highlights
Integration is the reverse process of differentiation.
Integral of the derivative of a function f(x) equals the function evaluated at the limits of integration.
The process of finding the integral of a function G(x) from 'A' to 'B' is discussed.
Finding a function G(x) such that its derivative is the given function G(x) is key to integration.
The integral of G(x) is equal to the integral of the derivative of G(x).
The derivative of a function reduces the power of x by 1.
The function f3(x) = x^3 is hypothesized to have a derivative of x^2.
The derivative of x^3 is calculated to be 3x^2, not x^2 as desired.
The function G(x) = x^3/3 is found to have a derivative of x^2.
Adding a constant to an antiderivative does not change its derivative.
Any function G(x) + C where C is a constant is an antiderivative of G(x).
Indefinite integrals are represented by the integral symbol without limits of integration.
The definite integral is found by evaluating the indefinite integral at the limits of integration.
The indefinite integral of x^2 is x^3/3 + C.
The definite integral yields a numerical answer, unlike the indefinite integral which yields a function.
The process of finding the indefinite integral of a function involves finding a function whose derivative equals the original function.
The indefinite integral is also referred to as simply the integral of the function.
The video challenges viewers to find the indefinite integral of the function H(x) = x^2 + 6.
The importance of subscribing to the channel for more educational content is highlighted.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: