Antiderivatives and indefinite integrals | AP Calculus AB | Khan Academy

Khan Academy
25 Jan 201303:43
EducationalLearning
32 Likes 10 Comments

TLDRThe video script explains the concept of derivatives and antiderivatives in calculus. It illustrates how the derivative of x squared is 2x and that adding constants like 1 or pi does not change this derivative. The script then introduces the concept of the antiderivative, which is essentially the reverse process of differentiation. The antiderivative of 2x is x squared plus a constant, and the notation for this is introduced as ∫(2x)dx = x^2 + C, known as the indefinite integral. This notation becomes particularly useful when studying definite integrals and calculating areas under curves.

Takeaways
  • 📌 The derivative of x squared (∂/∂x of x^2) is 2x.
  • 📌 Applying the derivative operator to x squared plus a constant yields the same result, 2x.
  • 📌 The derivative of a constant with respect to x is 0, as constants do not change with x.
  • 📌 The general rule for the derivative of x squared plus any constant is 2x, maintaining the constant nature.
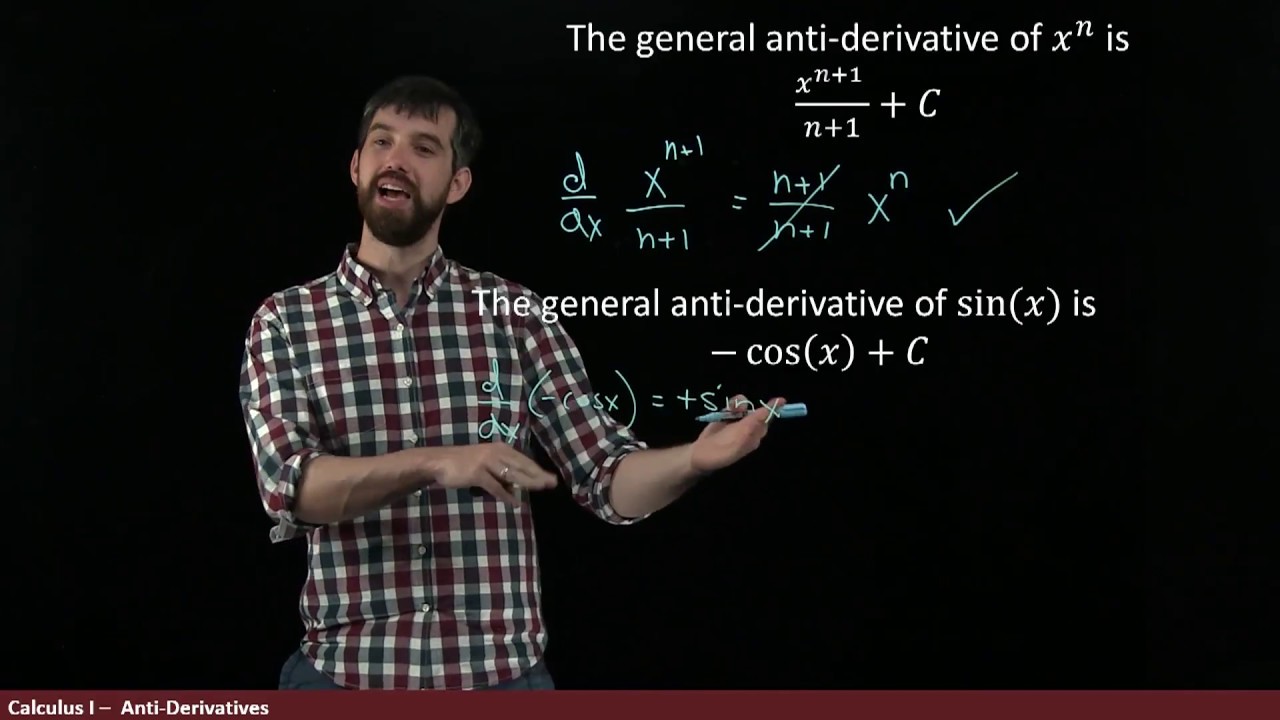
  • 📌 The antiderivative is the inverse operation of the derivative, aiming to find the original function given its derivative.
  • 📌 The antiderivative of 2x is x squared plus a constant, denoted as x^2 + C.
  • 📌 The notation for the antiderivative involves an elongated 'S' symbol (∫) and 'dx' indicating the variable of integration.
  • 📌 The indefinite integral symbol (∫) represents the antiderivative in a concise form.
  • 📌 The concept of the indefinite integral is crucial for understanding definite integrals and calculating areas under curves.
  • 📌 The use of ∫ and dx in the notation for antiderivative is a convention that simplifies the expression and prepares for further integration applications.
Q & A

  • What is the derivative of x squared with respect to x?

    -The derivative of x squared with respect to x is 2x.

  • What happens when you apply the derivative operator to a constant like 1?

    -The derivative of a constant with respect to x is 0, as constants do not change with respect to x.

  • What is the relationship between the derivative and the antiderivative?

    -The derivative and the antiderivative are inverse operations. The derivative finds the rate of change of a function, while the antiderivative finds a function whose derivative is the given function.

  • What does the notation ∫(2x)dx represent?

    -The notation ∫(2x)dx represents the antiderivative of 2x with respect to x, which is x squared plus a constant.

  • How can you express the antiderivative of 2x in the most general sense?

    -In the most general sense, the antiderivative of 2x can be expressed as x squared plus some constant, or x^2 + C.

  • What is the purpose of the indefinite integral notation?

    -The indefinite integral notation is used to denote the antiderivative of a function. It is particularly useful when studying definite integrals and calculating areas under curves.

  • Why is the constant 'pi' in the derivative of x squared plus pi also resulting in 2x?

    -The constant 'pi' is a numerical constant and when added to x squared, it does not affect the derivative with respect to x, which is still 2x. The derivative of a constant is zero, so it does not contribute to the derivative of the function.

  • What is the sum rule in differentiation?

    -The sum rule in differentiation states that the derivative of a sum of functions is the sum of the derivatives of each function individually. So, the derivative of (f(x) + g(x)) is (f'(x) + g'(x)).

  • What is the product rule in differentiation?

    -The product rule in differentiation states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function, expressed as (f(x) * g(x))' = f'(x) * g(x) + f(x) * g'(x).

  • What is the quotient rule in differentiation?

    -The quotient rule in differentiation states that the derivative of a quotient of two functions is the derivative of the numerator times the denominator minus the numerator times the derivative of the denominator, all divided by the square of the denominator, expressed as (f(x) / g(x))' = (f'(x) * g(x) - f(x) * g'(x)) / g(x)^2.

  • How can the chain rule be applied in differentiation?

    -The chain rule in differentiation is used when differentiating a composite function. It states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function, times the derivative of the inner function, expressed as (f(g(x)))' = f'(g(x)) * g'(x).

Outlines
00:00
📚 Understanding Derivatives

This paragraph introduces the concept of derivatives and their application to various functions. It explains how the derivative of x squared is consistently 2x, regardless of additional constants or symbols like pi and 1. The explanation emphasizes that the derivative of a constant is zero, as it does not change with respect to the variable x. The paragraph establishes that the general rule for the derivative of x squared with respect to x is 2x and that this applies even when x squared is combined with any constant.

🔄 Exploring Antiderivatives

The paragraph delves into the concept of antiderivatives, which are essentially the inverse operations of derivatives. It describes the process of finding an antiderivative as determining what function could have been differentiated to yield a given expression. The discussion uses the example of the derivative 2x to illustrate that it could correspond to the antiderivative of x squared alone, or x squared plus any constant. The paragraph introduces the notation for antiderivatives, using an elongated 's' (integral symbol) followed by dx around the function. It explains that this notation becomes particularly useful when studying definite integrals and approximating areas under curves.

Mindmap
Keywords
💡Derivative
The derivative is a fundamental concept in calculus that represents the rate of change of a function with respect to its variable. In the context of the video, it is used to find the slope of a curve at any given point, which can be thought of as the tangent line to the graph of the function. For example, the derivative of x squared is 2x, indicating that the rate of change of the function x^2 with respect to x is 2 times the value of x.
💡Antiderivative
The antiderivative, also known as the indefinite integral, is the reverse process of finding a derivative. It is used to find the original function whose derivative is given. The antiderivative is crucial in solving problems that involve finding the area under a curve or determining the original function from its rate of change. In the video, the antiderivative of 2x is x squared plus a constant, illustrating that there are multiple functions that can have the same derivative.
💡Constant
A constant is a value that does not change. In the context of the video, when dealing with derivatives, the derivative of a constant is always zero because a constant does not vary with the variable. This is a fundamental property of constants in calculus. On the other hand, when finding antiderivatives, constants are included as an additional term, known as the constant of integration, to account for the infinite number of functions that could have the same derivative.
💡Integration
Integration is the process of finding the antiderivative of a given function. It is the counterpart to differentiation and is used to calculate areas under curves, volumes, and other quantities that can be described by summing smaller pieces. The concept of integration is essential for understanding the accumulation of change, as it allows us to reverse the process of finding derivatives to find the original function.
💡Rate of Change
The rate of change is a fundamental concept in calculus that describes how a quantity changes with respect to another quantity. In the context of the video, it is used to describe the slope of a curve at any point, which is found by taking the derivative of a function. The rate of change is a key concept in understanding how differentiable functions behave and how they can be analyzed.
💡Slope
The slope of a curve at a particular point is a measure of how steep the graph is at that point. In calculus, the slope is found by taking the derivative of the function at the point of interest. The concept of slope is crucial for understanding the tangent line to a curve and for analyzing the behavior of functions.
💡Tangent Line
A tangent line is a straight line that touches a curve at a single point without crossing it. The slope of the tangent line is equal to the derivative of the function at that point. Tangent lines are used to approximate the behavior of a function near a specific point and are a fundamental concept in calculus for understanding the local properties of functions.
💡Indefinite Integral
The indefinite integral is a mathematical operation that is the inverse of differentiation. It is used to find a function whose derivative is a given function, up to a constant. The indefinite integral is represented by the symbol ∫ followed by the function and dx, indicating the integration with respect to the variable x. In the video, the indefinite integral of 2x is x squared plus a constant, which is denoted by ∫(2x)dx = x^2 + C, where C is the constant of integration.
💡Constant of Integration
The constant of integration, often denoted by 'c', is an additional term that is included when finding the antiderivative or indefinite integral of a function. It accounts for the fact that there are infinitely many functions that can have the same derivative. The constant of integration represents the vertical shift that can occur among these functions without affecting their derivatives.
💡Area Under a Curve
The area under a curve refers to the region enclosed by the graph of a function and the x-axis over a specified interval. This area can be calculated using the concept of integration. In the video, the mention of areas under curves and the use of rectangles to approximate these areas foreshadow the use of integration to find the exact area, which is a crucial application in various fields such as physics and engineering.
💡Differentiation
Differentiation is the process of finding the derivative of a function. It involves determining the rate at which a function changes with respect to its variable. Differentiation is a fundamental operation in calculus and is used to study the behavior of functions, including their maxima, minima, and inflection points.
Highlights

Derivative of x squared is 2x, a fundamental concept in calculus.

The derivative of x squared plus 1 is also 2x, demonstrating the property of constants in differentiation.

The derivative of any constant with respect to x, such as pi or 1, is 0, a key rule in differentiating constants.

Applying the derivative operator to expressions involving x squared and constants always yields 2x.

The antiderivative is the inverse operation of the derivative, used to find the original function given its derivative.

2x is the derivative of x squared, which is a basic relationship in calculus.

2x can also be the derivative of x squared plus any constant, illustrating the antiderivative's generality.

The antiderivative notation involves an elongated S and dx, representing the process of finding the antiderivative.

The antiderivative of 2x is x squared plus c, where c represents an arbitrary constant.

The indefinite integral of 2x is expressed as ∫(2x)dx, which is another way of denoting the antiderivative.

The notation for the antiderivative is crucial for understanding definite integrals and calculating areas under curves.

The process of finding antiderivatives is simplified by using the established notation, making it easier to work with calculus problems.

The concept of the antiderivative is foundational for more advanced topics in calculus, such as integration and summing areas.

Differentiation and antiderivative operations are essential for solving a wide range of mathematical and real-world problems.

Understanding the relationship between derivatives and antiderivatives is key to mastering calculus.

The properties of constants and their role in differentiation are important for accurate mathematical analysis.

The process of finding the antiderivative of an expression is fundamental to the study of calculus and its applications.

Transcripts

Browse More Related Video

Calculus - Lesson 16 | Indefinite and Definite Integrals | Don't Memorise

The Indefinite Integral or Anti-derivative

Integrals AKA Antiderivatives EXPLAINED with Examples

Derivatives of Integrals (w/ Chain Rule)

What's an anti-derivative?

Derivative of absolute value function

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus ConceptsDerivativesAntiderivativesIntegrationMath EducationIndefinite Integrals2x FunctionNotation ExplanationArea Under CurvesMathematical Analysis