The Indefinite Integral or Anti-derivative

Khan Academy
18 Oct 200709:28
EducationalLearning
32 Likes 10 Comments

TLDRThe video script provides an insightful overview of the concept of indefinite integrals, also known as antiderivatives, in calculus. It begins with a review of derivatives, using the example of the derivative of x squared to illustrate the process. The script then delves into the reverse process of finding the antiderivative, using the example of 2x and explaining that the antiderivative of x to the power of n is x to the power of n+1 divided by n+1, plus a constant. The general rule for finding the indefinite integral of a function is clearly outlined, and the concept is reinforced with examples, highlighting the inverse relationship between differentiation and integration. The script concludes by teasing future topics, such as integration by parts.

Takeaways
  • 📚 The presentation introduces the concept of the indefinite integral, also known as the antiderivative.
  • 🔄 The process of finding the indefinite integral is the reverse of taking the derivative.
  • 🎓 Reviewing derivatives is important to understand the relationship between derivatives and indefinite integrals.
  • 🌟 The derivative of x squared is 2x, which is a fundamental concept in calculus.
  • 🔢 For an expression like 2x, the indefinite integral is x squared plus a constant (C).
  • 📈 The general rule for finding the indefinite integral of a function is to increase the exponent by one and multiply by the coefficient divided by the new exponent.
  • 🌐 The notation ∫ (integral sign) represents the operation of finding the antiderivative.
  • 📊 The derivative of a constant is zero, which is a key property used in solving indefinite integrals.
  • 🧩 The process of solving indefinite integrals can be generalized for any power function by following the rule of increasing the exponent and adjusting the coefficient.
  • 🔄 The concept of the chain rule and product rule in derivatives has a counterpart in integration, which will be explored in further presentations.
  • 🚀 The presentation concludes with a teaser for upcoming content, which will delve deeper into more complex integration techniques.
Q & A

  • What is the main topic of the presentation?

    -The main topic of the presentation is the indefinite integral, also known as the antiderivative.

  • How is the derivative of x squared calculated?

    -The derivative of x squared is calculated by taking the exponent (which is 2), making it the new coefficient, and reducing the exponent by one, resulting in 2x to the power of 1, or simply 2x.

  • What does it mean to find the antiderivative of a function?

    -To find the antiderivative of a function is to determine a function whose derivative is equal to the original function. It is the reverse process of taking a derivative.

  • What is the notation used for the indefinite integral?

    -The notation used for the indefinite integral is the squiggly line (∫), followed by the function and dx. For example, the indefinite integral of 2x dx is written as ∫2x dx.

  • Why is it important to include a constant 'c' when expressing the result of an indefinite integral?

    -Including a constant 'c' is important because it accounts for all possible antiderivatives of the function. The derivative of a constant is zero, so adding or subtracting a constant does not change the derivative, making the 'plus c' essential for the general solution.

  • How does the process of finding the indefinite integral of x cubed illustrate the general rule for integration?

    -The process of finding the indefinite integral of x cubed demonstrates the general rule for integration by showing that you increase the exponent by one and multiply by the reciprocal of the new exponent, while also accounting for the original coefficient.

  • What happens to the exponent when applying the general rule for integration?

    -When applying the general rule for integration, the exponent on the function is increased by one.

  • How is the coefficient 'b' treated during the integration process?

    -The coefficient 'b' remains unchanged during the integration process. It is multiplied by the new function after the exponent has been increased and the reciprocal has been taken.

  • What is the result of the indefinite integral of 5x to the seventh power?

    -The result of the indefinite integral of 5x to the seventh power is (5/8)x to the eighth power plus a constant 'c'.

  • What is the relationship between the derivative and the indefinite integral in calculus?

    -The derivative and the indefinite integral are inverse operations in calculus. The derivative finds the rate of change or slope of a function, while the indefinite integral finds the original function of which the given function could be the derivative.

Outlines
00:00
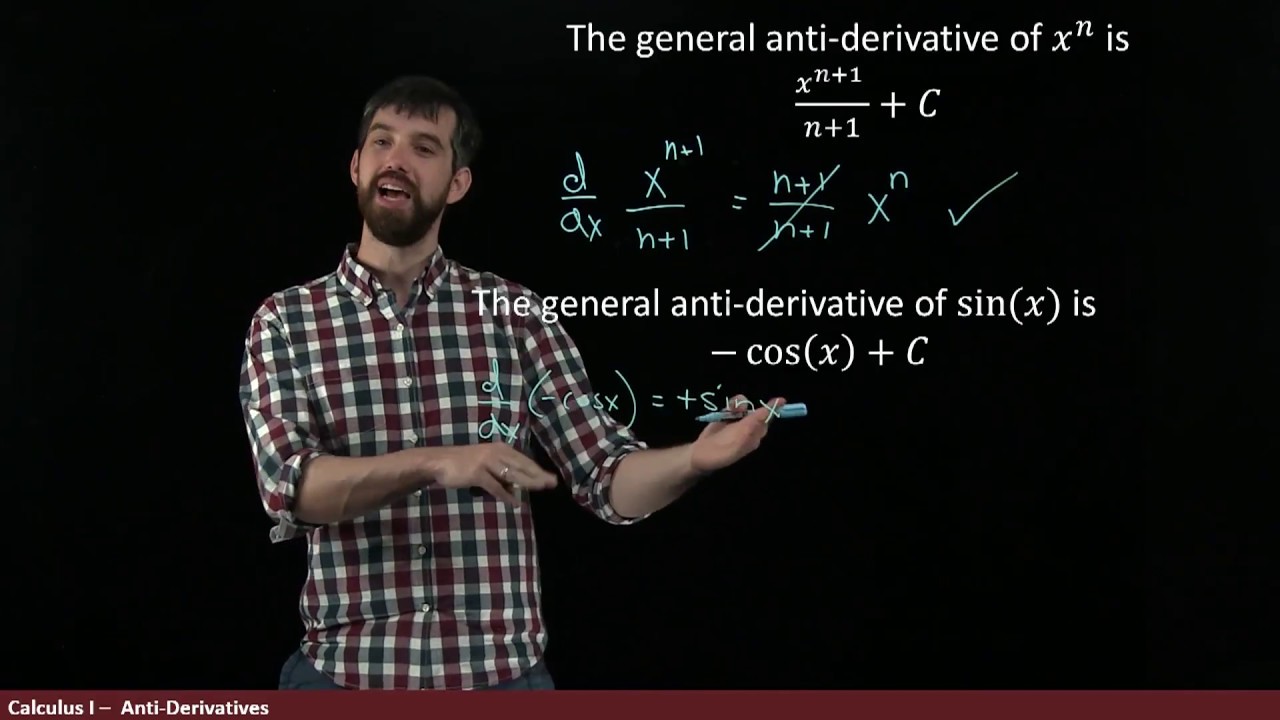
📚 Introduction to Indefinite Integrals

This paragraph begins with a review of the concept of derivatives, emphasizing the process of finding the derivative of an expression, such as x squared, which results in 2x. The speaker then transitions into the main topic, the indefinite integral, or antiderivative, and explains the process of finding it by reversing the operation of differentiation. The paragraph highlights the notation used for indefinite integrals, including the squiggly line and dx, and introduces the idea of adding a constant (c) to the result, as the derivative of a constant is zero. The speaker encourages an intuitive understanding of the process and sets the stage for further exploration of indefinite integrals in the following sections.

05:01
🧠 Systematic Approach to Solving Indefinite Integrals

In this paragraph, the speaker delves deeper into the method of solving indefinite integrals by using a systematic approach. Starting with an intuitive guess based on the form of the derivative, the speaker guides the audience through the process of finding the indefinite integral of x cubed. By applying the derivative rule in reverse, the speaker increases the exponent by one and multiplies by the reciprocal of the new exponent, resulting in 1/4 x to the fourth. The paragraph establishes a general rule for finding the indefinite integral of any function of the form b times x to the n, and emphasizes the importance of including the constant (c) in the answer. The speaker also provides a quick example to reinforce the rule and promises a more detailed exploration in the next presentation.

Mindmap
Keywords
💡indefinite integral
The indefinite integral, also known as the antiderivative, is a fundamental concept in calculus that represents a family of functions that could have a given function as its derivative. In the context of the video, the indefinite integral is used to find the original function from which a given derivative can be obtained. For example, the derivative of x squared is 2x, and the indefinite integral of 2x dx is x squared plus a constant (c), illustrating that the indefinite integral 'undoes' the derivative operation.
💡derivative
A derivative is a mathematical concept that represents the rate of change or the slope of a function at a particular point. In the video, the derivative is used to understand how a function changes and to find the relationship between a function and its derivative. The process of finding the derivative is demonstrated with the example of x squared, where the derivative is 2x.
💡antiderivative
An antiderivative is a function that, when differentiated, yields the original function. It is the inverse operation of differentiation and is used to find the original function from which a given derivative can be obtained. The antiderivative is also known as the indefinite integral. In the video, the process of finding the antiderivative is explained, showing that it can be used to 'reverse' the operation of differentiation.
💡constant
In the context of the video, a constant refers to a value that does not change. It is important to note that the derivative of any constant is zero, which is a fundamental property of constants in calculus. Additionally, when finding the indefinite integral, a constant term is added to the result, known as the constant of integration (c), to account for the family of functions that could have the same derivative.
💡exponent
An exponent is a mathematical notation that indicates the number of times a base is multiplied by itself. In the context of the video, exponents are used to express functions like x squared or x cubed, and understanding how to manipulate exponents is crucial for both differentiation and integration.
💡integration
Integration is a mathematical process that is the inverse of differentiation. It is used to find the original function from which a given derivative can be obtained. In the video, integration is discussed in the context of the indefinite integral, which is a function plus an arbitrary constant (c).
💡rule of integration
The rule of integration is a general method for finding the indefinite integral of a function. According to the rule, to find the integral of a function b times x to the n, you increase the exponent on x by one to get x to the n plus 1, and then multiply by the coefficient b times the inverse of the new exponent (1 over n plus 1). This rule is demonstrated in the video through examples like the indefinite integral of x cubed and 5 x to the seventh.
💡chain rule
The chain rule is a fundamental principle in calculus that is used to find the derivative of a composite function. It involves differentiating the outer function and then multiplying by the derivative of the inner function. Although not explicitly discussed in the video, the concept of reversing the chain rule is mentioned as a topic for a future presentation, implying its relevance to understanding integration techniques.
💡product rule
The product rule is a calculus formula used to find the derivative of a product of two functions. It states that the derivative of u times v is u times the derivative of v plus v times the derivative of u. In the context of the video, the product rule is not directly discussed, but the mention of integration by parts suggests that it is relevant since integration by parts is essentially reversing the product rule.
💡slope
Slope is a measure of the steepness of a line, or in the context of a curve, the rate of change of the function at a particular point. In the video, the concept of slope is used to describe the derivative of a function, which gives the slope of the tangent line to the curve at any point on the function.
💡notation
In the context of the video, notation refers to the symbols and conventions used to represent mathematical operations, such as the ∫ symbol for integration and the dx for differentials. Proper notation is crucial for expressing and communicating mathematical ideas accurately.
Highlights

Introduction to the concept of the indefinite integral or antiderivative.

Review of the derivative concept and its operation on the function x squared.

Explanation of the process to find the antiderivative of an expression, using 2x as an example.

Discussion on the relationship between the derivative and antiderivative, and the importance of the plus c in indefinite integrals.

Illustration of how to reverse the process of differentiation to find the antiderivative, emphasizing the inverse operation.

Presentation of a general rule for finding the indefinite integral of a function of the form b times x to the n.

Example calculation of the indefinite integral of x cubed and the explanation of the resulting function.

Explanation of the pattern observed when converting from a function to its antiderivative, involving increasing the exponent and adjusting the coefficient.

Demonstration of how to find the indefinite integral of 5x to the seventh power using the general rule.

Confirmation of the correctness of the calculated indefinite integral by differentiating it back to the original function.

Introduction to the concept of the definite integral and its relation to the indefinite integral.

预告 of upcoming presentations covering more examples and the reverse of the chain rule in integration.

Emphasis on the practical applications and theoretical significance of understanding the process of finding antiderivatives.

Explanation of the integration by parts as a method essentially reversing the product rule.

The importance of the coefficient b in the indefinite integral and its effect on the resulting function.

Transcripts

Browse More Related Video

Antiderivatives and indefinite integrals | AP Calculus AB | Khan Academy

Calculus - Lesson 16 | Indefinite and Definite Integrals | Don't Memorise

Antiderivatives!

What's an anti-derivative?

Integrals AKA Antiderivatives EXPLAINED with Examples

Calculus - Integration By Parts

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusIntegrationAntiderivativeMathematicsEducationOnline LearningMath TutorialIndefinite IntegralsDerivative RulesMathematics Education