LEPT Math Majorship 2024 | Trigonometry Calculator Techniques

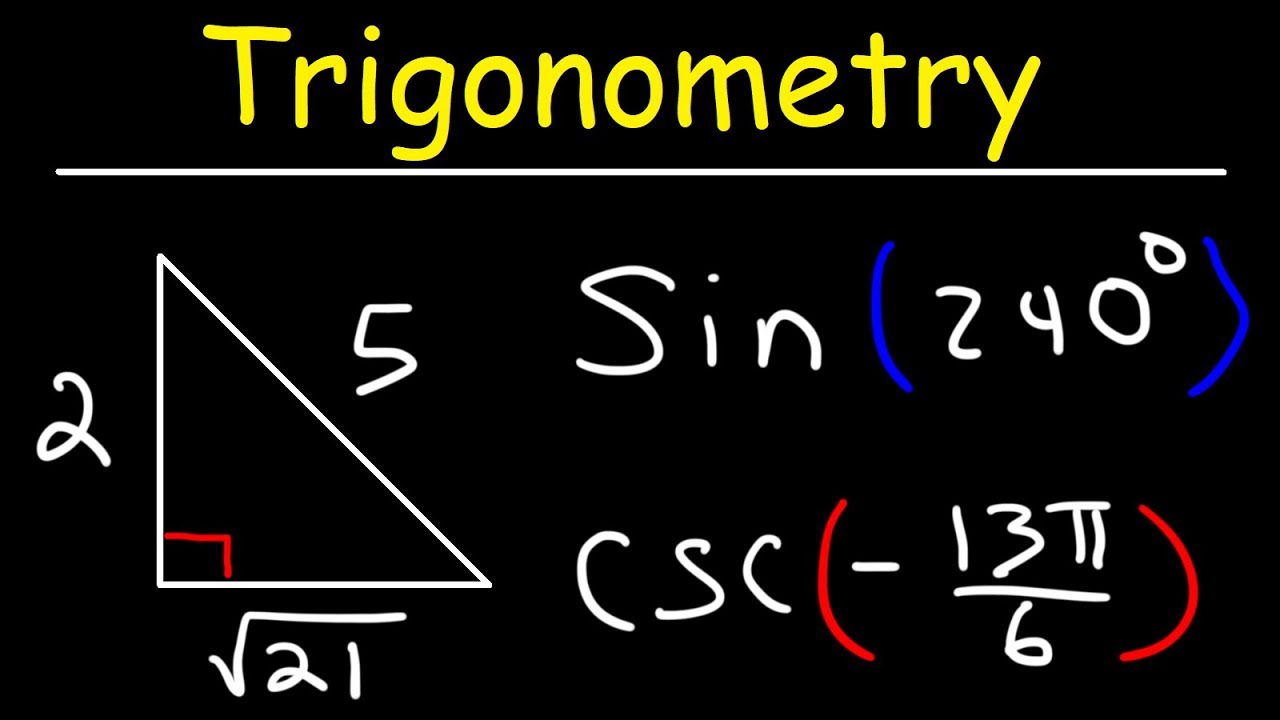
The video begins with an introduction to trigonometry, a mathematical branch that studies the relationships between the sides and angles of right-angled triangles. The speaker mentions the use of a calculator, specifically the 'cash fex 570 es Plus,' for solving trigonometric problems. The focus is on finding missing angles or sides using trigonometric formulas, with angles measured in either degrees or radians. The video promises to cover basic trigonometric functions—sine, cosine, and tangent—but will not delve into trigonometric identities. An overview of these functions is provided, with sine represented as the ratio of the opposite side to the hypotenuse.

This paragraph delves deeper into the basic trigonometric functions: sine (sin), cosine (cos), and tangent (tan). It explains the meaning of each letter in the function names and provides the formulas for each. The reciprocals of these functions are also introduced: cosecant (csc) for sine, secant (sec) for cosine, and cotangent (cot) for tangent. The speaker emphasizes the importance of understanding these reciprocals for solving problems, especially when using a calculator, and provides examples of how to input these functions into the calculator.

The speaker presents a series of problems to solve using the calculator techniques introduced earlier. The first problem involves finding the value of an angle between 270° and 360° given a trigonometric equation. The solution process is demonstrated step by step, including how to input the equation into the calculator and interpret the results. The correct answer is identified, and the speaker encourages viewers to practice similar problems to improve their skills.

The video continues with more complex problems, such as finding the value of an angle when given the sum of cosines of two other angles. The process involves using the calculator to input and solve the equation. The speaker also presents a problem that requires simplifying a trigonometric expression, demonstrating how to use the calculator to find the simplest form of the expression. The correct answers are revealed, and the speaker provides a brief analysis of each problem.

A real-world problem is introduced where a tree breaks due to a storm, and the top touches the ground at a 30° angle with the horizontal. The distance from the base of the tree to the point where it touches the ground is given as 18 meters. The speaker uses trigonometric functions to solve for the height of the tree, demonstrating the practical application of trigonometry. The solution involves calculating the opposite and adjacent sides relative to the given angle and then combining these to find the tree's height.

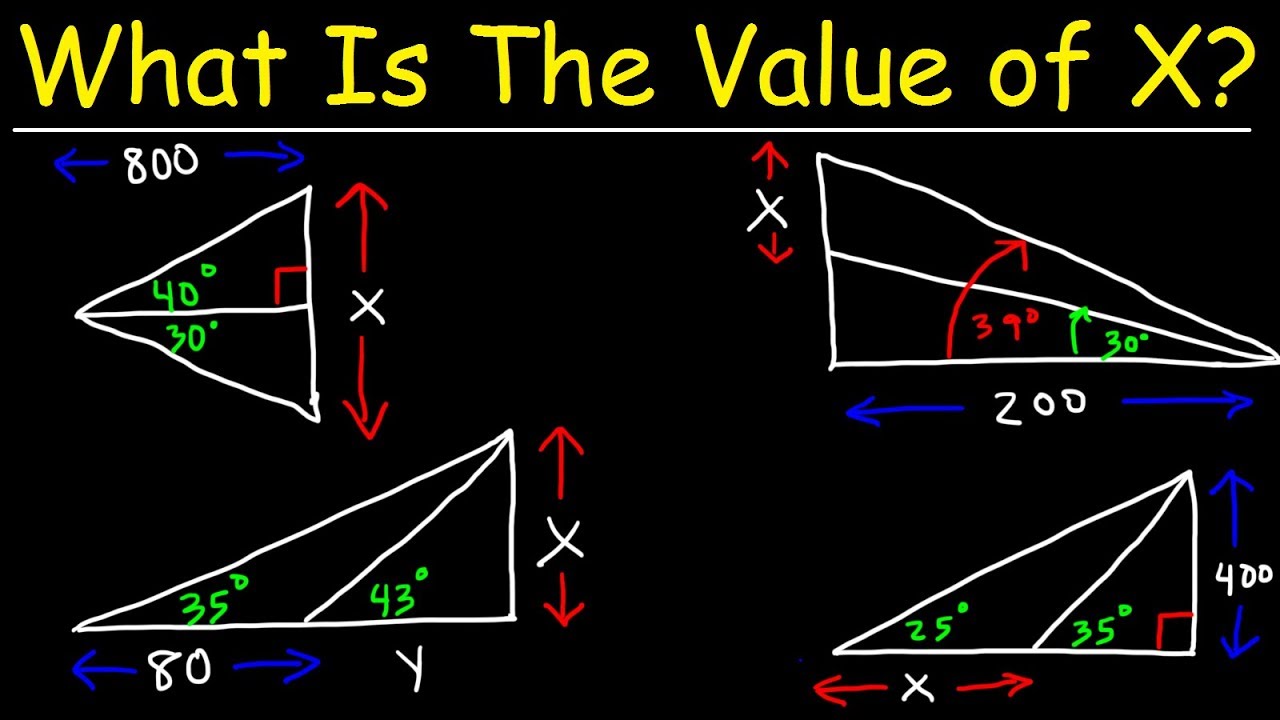
The video presents a problem involving a triangle with two known sides and an angle opposite one of those sides. The speaker explains how to use the sine law to find the third side of the triangle. The process involves setting up an equation based on the ratio of the sides to the sines of their opposite angles. The solution is found using a calculator, and the correct answer is identified.

The speaker introduces a problem where a man finds the angle of elevation of the top of a tower to be 30° and then walks 85 meters closer to the tower, finding the angle of elevation to be 60°. The goal is to find the height of the tower. The solution involves using trigonometric functions to relate the angles of elevation to the height of the tower and the distance the man walked. The correct height is calculated and presented.

A problem involving a pole casting a 15-meter shadow with the sun at an angle of elevation of 61° is presented. The pole is also inclined 15° from the vertical towards the sun. The speaker uses trigonometric principles to determine the length of the pole. The solution process involves using the sine and cosine laws to relate the given angles and side lengths, leading to the calculation of the pole's length.

The final problem involves calculating the height of a monument when the angles of depression from the top of a 50-meter tall PLT tower are given as 13° and 35°. The speaker explains how to use trigonometric functions to relate these angles to the heights of the tower and the monument. The solution involves setting up equations based on the tangent of the angles and solving for the unknown height of the monument.

The video concludes with a review of the key trigonometric concepts and laws covered, including the three basic trigonometric functions (sine, cosine, tangent), their reciprocals, and the use of calculator techniques for solving problems. The speaker also recaps the sine law and cosine law, explaining when to apply each law based on the given sides and angles of triangles. The importance of understanding angles of elevation and depression is emphasized, as well as the practical applications of these concepts in solving real-world problems.

In the closing segment, the speaker offers prayers for blessings and success in the upcoming licensure exam in 2024. They encourage viewers to share the video and express gratitude to the teachers involved in the video's creation. The speaker also includes a musical element, adding a positive and hopeful tone to the conclusion of the video.