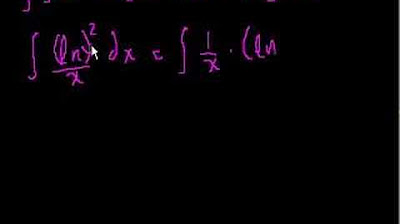Watch this before your calculus 2 final! Top 4 integration mistakes students often make!
TLDRThis educational video script addresses common mistakes made while solving calculus integrals. It begins by correcting the oversight of the constant 'C' in the integral of x^2 dx. It then refutes the incorrect application of the reverse power rule to sin^2(x), demonstrating the correct method using trigonometric identities. The script further explains the proper integration of inverse tangent functions using integration by parts and the substitution method. It concludes with a reminder to avoid similar errors, emphasizing the importance of understanding calculus concepts for efficient problem-solving.
Takeaways
- 📚 Always remember to include the constant of integration, 'C', when solving indefinite integrals.
- ❌ Incorrectly applying the reverse power rule to functions like sin^x instead of x^n can lead to mistakes.
- 🔄 The derivative of sin^x is not sin^(3x), but rather 3sin^2(x)cos(x), highlighting the importance of the chain rule.
- 🧩 To integrate sin^2(x), use the power reduction identity sin^2(x) = (1 - cos(2x))/2, which simplifies the integration process.
- 🔑 The integral of 1/(1 + x^2) is arctan(x) + C, not ln(1 + x^2), which is a common mistake.
- 🚫 Forgetting to use the chain rule when differentiating can lead to incorrect results.
- 🔠 When integrating functions like arctan(x), consider using integration by parts or recognizing the standard integral form.
- 📐 For the integral of 1/(1 + x^2), a substitution method with u = 1 + x^2 and du = 2x dx can simplify the integration.
- 🔁 Using the substitution method (u-sub) can be a powerful tool for integrating products of functions.
- 📐 The integral of 1/u du is the natural logarithm ln|u| + C, which is key for integrating rational functions.
- 🔍 When faced with an integral like ∫1/(1 + x^2), recognize it as a standard form and use the known antiderivative, arctan(x) + C.
- ⛓ The mistake of omitting the chain rule or the constant 'C' can be costly in calculus, leading to incorrect solutions.
Q & A
What is the mistake in the initial integral calculation of x^2 dx using the reverse power rule?
-The mistake is that the constant of integration, denoted by 'C', was omitted from the final answer. The correct integral should include 'C' as part of the result.
Why is the integral of sin^2(x) incorrect when using the reverse power rule directly?
-The reverse power rule can only be applied when the base is a simple 'x', not a trigonometric function like sin(x). The derivative of sin^2(x) is not the same as the integral of sin^2(x), which requires a power reduction identity to be correctly integrated.
What is the power reduction identity used to integrate sin^2(x)?
-The power reduction identity used is sin^2(x) = (1 - cos(2x))/2. This identity allows the integration of sin^2(x) by reducing the power of the trigonometric function.
How does one integrate the expression 1/(1 + x^2)?
-The integral of 1/(1 + x^2) is the inverse tangent of x, denoted as arctan(x) or tan^(-1)(x), plus a constant of integration 'C'.
What is the mistake in the script regarding the integral of arctan(x)?
-The mistake is that the script initially presents the derivative of arctan(x) instead of its integral. The correct integral of arctan(x) is x * arctan(x) - 1/2 * ln(1 + x^2) plus a constant 'C'.
What is the purpose of the chain rule in differentiation and integration?
-The chain rule is used in differentiation when the function is composed of another function, allowing us to differentiate the outer function times the derivative of the inner function. In integration, it is used to integrate composite functions by reversing the process of differentiation.
What is the method used to integrate the function 1/(1 + x^2)?
-The method used to integrate 1/(1 + x^2) is the direct application of the known integral formula for rational functions of the form 1/(ax + b), which yields the inverse tangent of x as the result.
Why can't we use the identity 1 - cos^2(x) to integrate sin^2(x)?
-While 1 - cos^2(x) is equal to sin^2(x), using this identity would lead to a situation where we would have to integrate -cos^2(x), which is not as straightforward as integrating sin^2(x). The identity does not simplify the integration process.
What is the correct way to integrate sin^2(x) using the given identity?
-The correct way is to use the identity sin^2(x) = (1 - cos(2x))/2, which allows us to integrate each term separately after applying the identity, leading to a simpler integral to solve.
What is the method of integration by parts and when is it used?
-Integration by parts is a technique used to integrate a product of two functions, where one function is chosen to be integrated directly and the other to be differentiated. It is used when the direct integration of the product is difficult, and it involves reversing the order of differentiation.
How does the method of substitution (u-substitution) help in integrating more complex expressions?
-U-substitution simplifies the integral by letting 'u' be a function of 'x' and 'du' be its derivative with respect to 'x'. This changes the integral into a new form that is easier to integrate, particularly useful for integrals involving products and compositions.
What is the final answer for the integral of 1/(1 + x^2) using the method of substitution?
-After applying u-substitution with u = 1 + x^2, the integral becomes 1/2 * integral of 1/u du, which simplifies to 1/2 * ln|u| + C. Substituting back, we get 1/2 * ln|1 + x^2| + C.
Outlines
🤓 Correcting Common Calculus Mistakes
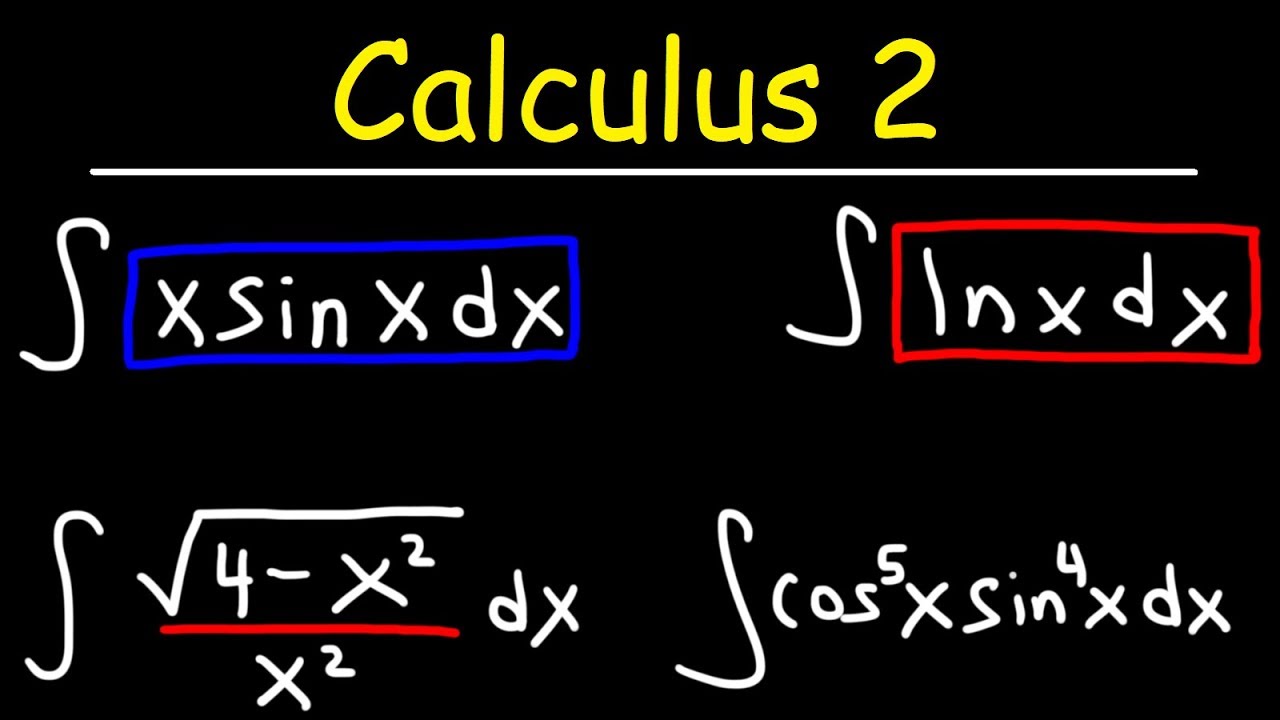
This paragraph addresses common mistakes made while solving calculus problems. It starts by pointing out the omission of the constant of integration 'C' in the integral of x^3 dx, which should be 1/3 x^3 + C. The speaker then critiques the incorrect application of the reverse power rule to sin^2(x), demonstrating the correct method by using the identity sin^2(x) = 1/2 (1 - cos(2x)) and integrating each part. The paragraph emphasizes the importance of the chain rule and the correct use of trigonometric identities in integration.
📚 Mastering Integration Techniques in Calculus
This section focuses on the integration of inverse tangent functions. It clarifies that the integral of arctan(x) is not simply 1/(1+x^2) and proceeds to show the correct integration by parts method. The process involves differentiating 1/(1+x^2) and integrating x, leading to the use of substitution with u = 1+x^2 to simplify the integral to a natural logarithm. The summary concludes with the correct integral of arctan(x) being x * arctan(x) - 1/2 ln|1+x^2| + C.
🧐 The Art of Correct Integration: Tricks and Techniques
The final paragraph discusses the integration of 1/(1+x^2), correcting the common misconception that it results in a natural logarithm. It introduces a trick by setting x = tan(θ) to transform the integral into a simpler form, which then cancels out to leave an integral in terms of θ. After integrating and converting back to the original x variable, the correct answer is found to be arctan(x) + C. This paragraph serves as a review of calculus integration techniques and emphasizes avoiding common errors.
Mindmap
Keywords
💡Integral
💡Reverse Power Rule
💡Constant of Integration (+C)
💡Chain Rule
💡Power Reduction Identity
💡Integration by Parts
💡Trigonometric Functions
💡Differentiation
💡U-Substitution
💡Natural Logarithm
💡Inverse Trigonometric Functions
Highlights
The integral of x^2 dx can be solved using the reverse power rule by adding 1 to the power and dividing by the new power.
The integral of sin^2(x) dx requires using an identity to reduce the power. The identity sin^2(x) = (1 - cos(2x))/2 is useful.
When integrating sin^2(x), the power reduction identity allows converting the base from sin(x) to x, making the integral easier to solve.
The integral of arctan(x) dx can be solved using integration by parts. Differentiate one part and integrate the other.
Integration by parts involves multiplying the derivative of one part by the integral of the other part, and subtracting.
To integrate 1/(1+x^2), use the substitution x = tan(θ) to simplify the integral to θ + C, where θ = arctan(x).
Forgetting the constant of integration C is a common mistake when solving integrals. Always include +C in the final answer.
Forgetting to apply the chain rule when differentiating is another common mistake, which can lead to incorrect solutions.
When using the reverse power rule, ensure the base is just x, not a function like sin(x).
For sin^2(x), using the identity sin^2(x) = (1 - cos(2x))/2 allows applying the reverse power rule to both terms separately.
When integrating a product of a constant and a function, you can treat the constant as a coefficient outside the integral.
The anti-derivative of -cos(2x) involves using the chain rule in reverse to divide by the power of 2.
Integration by parts is a powerful technique for integrating inverse functions like arctan(x).
When integrating 1/(1+x^2), the substitution x = tan(θ) simplifies the integral by converting it to an integral in θ.
Always check your work by differentiating the result of an integral to ensure it matches the original function.
Mastering common integral techniques like power rule, substitution, and integration by parts can save a lot of time.
Practicing a variety of integral problems helps solidify understanding and avoid common mistakes.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: