Calculus 2 - Basic Integration
TLDRThis video delves into advanced integration techniques in calculus, focusing on integration by parts, trigonometric integrals, and trigonometric substitution. It demonstrates how to integrate complex functions like x*sine(x) and natural log(x), and covers the use of trigonometric identities and substitutions for solving integrals involving trigonometric functions. The video serves as a comprehensive guide for students tackling calculus 2, offering insights into problem-solving strategies and encouraging further exploration through additional video content.
Takeaways
- 📚 Integration by parts is a technique used for integrating functions of the form u dv, where u and dv are chosen according to the problem at hand.
- 🌟 The formula for integration by parts is ∫u dv = u*v - ∫v du, which requires selecting appropriate functions for u and dv.
- 📈 When integrating a product of a linear function and a trigonometric function, such as x*sin(x), use integration by parts with u = x and dv = sin(x)dx.
- 🧠 For the integral of ln(x)dx, use integration by parts with u = ln(x) and dv = dx, resulting in x*ln(x) - ∫x*(1/x)dx, which simplifies to x*ln(x) - x + C.
- 🌐 In dealing with trigonometric integrals, use identities such as Pythagorean identities and double angle formulas to simplify the integrand before applying techniques like u-substitution.
- 🔄 The integral of a function like cos^3(x)dx can be approached by using u-substitution with u = sin(x), transforming the problem into a more manageable form.
- 📊 For integrals involving powers of trig functions, like cos^5(x)*sin^4(x),, separate the integrand into components that can be handled with u-substitution and those that can be simplified with trigonometric identities.
- 🤔 The integral of sin^2(x)dx can be solved using the half-angle identity, which states that sin^2(x) = (1 - cos(2x))/2, simplifying the integration process.
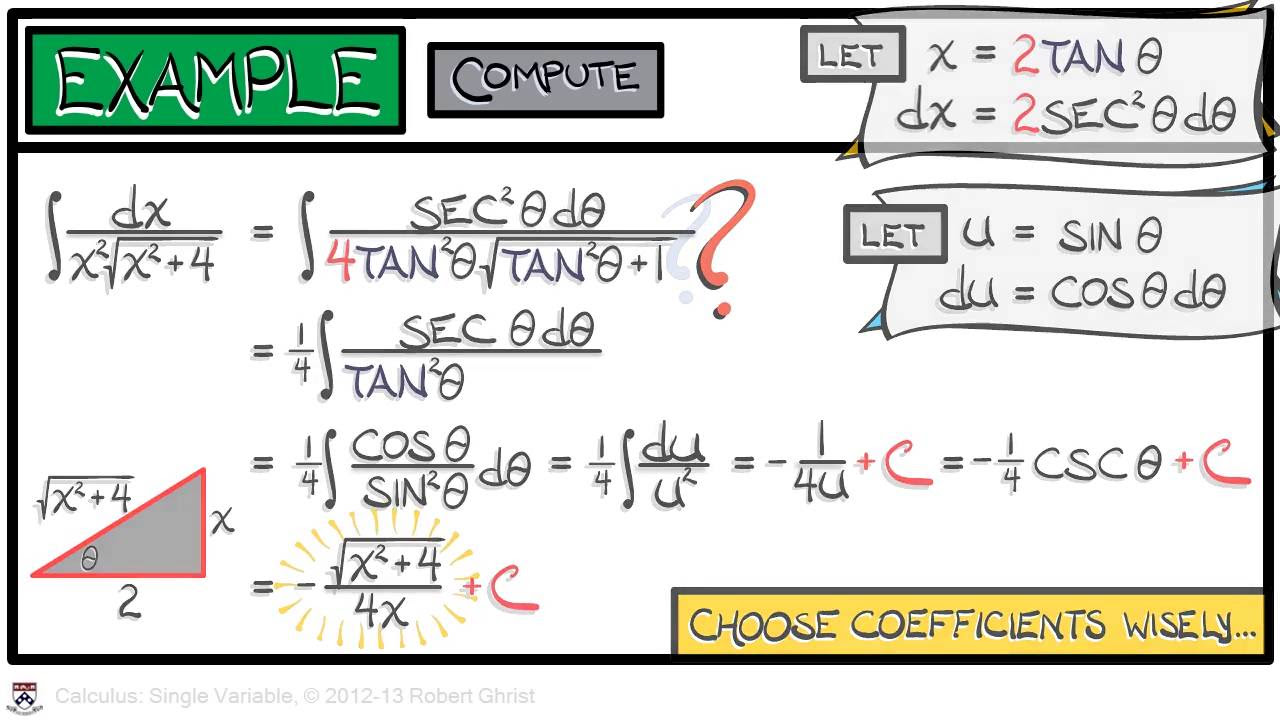
- 📐 Trigonometric substitution is a powerful technique for integrating expressions of the form √(a^2 - x^2) or √(a^2 + x^2) by setting x = a*sin(θ) or x = a*tan(θ), respectively.
- 🌟 The antiderivative of functions involving powers of tangent and secant, such as ∫tan^6(x)sec^4(x)dx, can be found by applying integration by parts and using the relevant trigonometric identities.
- 🔍 The process of integration can often be simplified by recognizing and applying relevant trigonometric identities and substitution techniques to transform the integrand into a more familiar form.
Q & A
What is the main topic of the video?
-The main topic of the video is different integration techniques in Calculus 2, including integration by parts, trigonometric integrals, and trigonometric substitution.
How is the integration by parts method applied in the video?
-Integration by parts is applied to the function x * sin(x). The video demonstrates selecting u as x (with du = dx) and dv as sin(x) (with v as -cos(x)). The method is then used to find the antiderivative, resulting in -x * cos(x) + sin(x) + C.
What is the significance of the Pythagorean identity used in the video?
-The Pythagorean identity (sin^2(x) + cos^2(x) = 1) is used to rewrite the integral of cosine cubed x as 1 - sin^2(x) * cos(x), allowing the use of u-substitution for easier integration.
How does the video approach the integral of a function with an odd and even power of trigonometric functions?
-The video breaks down the function into components, separating the odd and even powers. It then uses u-substitution and trigonometric identities to simplify and integrate the expression, resulting in a final antiderivative involving powers of sine and cosine.
What trigonometric identity is used to solve the integral of sine squared x?
-The video uses the half-angle identity, which states that sine squared x is equal to (1 - cos(2x))/2, to solve the integral of sine squared x.
How does the video introduce the concept of trigonometric substitution?
-The video introduces trigonometric substitution by explaining the conditions under which to set x equal to sine theta, tangent theta, or secant theta based on the form of the integral expression, and demonstrates its application by solving the integral of the square root of (4 - x^2) / x^2.
What is the role of the power rule in the video's examples?
-The power rule is used in the video to find the antiderivative of terms involving powers of the variable u, such as in the example where the integral of u^6 and u^8 is solved using the power rule to find u^(6/7) and u^(8/9) respectively.
How does the video handle the integration of products of trigonometric functions?
-The video handles the integration of products of trigonometric functions by using u-substitution and trigonometric identities to simplify the expression, breaking it down into manageable parts, and then integrating each part separately.
What is the significance of the identity 1 + tan^2(x) = sec^2(x) in the video?
-The identity 1 + tan^2(x) = sec^2(x) is used in the video to simplify the expression for the integral of tan^6(x) * sec^4(x), allowing the application of u-substitution and the subsequent integration of the terms.
How does the video explain the process of finding the antiderivative of a function with a complex integrand?
-The video explains the process by first identifying the appropriate substitution method, then applying the necessary algebraic and trigonometric identities to simplify the integrand, and finally integrating the terms to find the antiderivative.
What additional resources does the video recommend for learning more about integration techniques?
-The video recommends checking out the new Calculus video playlist in the description section or subscribing to the channel for more examples and content on integration techniques.
Outlines
📚 Integration by Parts
This paragraph introduces integration by parts, a technique used to integrate functions that are the product of two different types of functions, such as a linear function and a trigonometric function. The formula for integration by parts is ∫(uv')dx = uv - ∫(vdu), where u and v' are chosen based on the function to be integrated. The process is demonstrated by integrating x*sin(x)dx, resulting in -x*cos(x) + ∫(-cos(x)dx), which simplifies to -x*cos(x) + sin(x) + C. Another example involves integrating ln(x)dx, leading to x*ln(x) - ∫(x*(1/x)dx), simplifying to x*ln(x) - x + C.
📐 Trigonometric Integrals
This paragraph delves into techniques for integrating trigonometric functions, such as cosine cubed x and cosine to the fifth power times sine to the fourth power. The approach involves using trigonometric identities and u-substitution to simplify the integral. For instance, cosine cubed x is integrated by replacing it with (1 - sin^2(x))*cos(x) and using u-substitution with u = sin(x), resulting in sin(x) - (1/3)*sin^3(x) + C. The paragraph also introduces the concept of trigonometric substitution for certain types of integrals.
🔢 Integration of Trigonometric Functions
The focus of this paragraph is on integrating more complex trigonometric functions, such as sine squared x and tangent to the sixth power times secant to the fourth power. The half-angle identity for sine squared is used to integrate sine squared x, resulting in (1/2)x - (1/4)*sin(2x) + C. The paragraph also covers the integration of tangent and secant functions, using the identity 1 + tan^2(x) = sec^2(x) to simplify the integral and resulting in 1/7*tan^7(x) + 1/9*tan^9(x) + C. The process involves substituting trigonometric functions with variables, applying the power rule, and simplifying the expression to find the antiderivative.
🔍 Trigonometric Substitution
This paragraph explains the concept of trigonometric substitution, a technique used for integrating expressions of the form a^2 - x^2 under a square root. The substitution x = a*sin(θ) is used, with dx = a*cos(θ)dθ, to transform the integral into a more manageable form. The example provided involves integrating the square root of 4 - x^2 divided by x^2, which after substitution becomes an integral involving cosine and cotangent functions. The final result is derived by using the relationships between trigonometric functions and their derivatives, and the constant of integration is included.
🎓 Advanced Integration Techniques
The final paragraph of the script wraps up the discussion on integration techniques, emphasizing the importance of understanding these methods for success in calculus 2. It provides a brief overview of the main integration techniques covered in the video, such as integration by parts and trigonometric integrals, and encourages viewers to explore additional examples through a linked playlist. The paragraph also poses a final integration problem involving sine squared x, prompting viewers to apply the half-angle identity to find the antiderivative.
Mindmap
Keywords
💡Integration by Parts
💡Trigonometric Integrals
💡U-Substitution
💡Trigonometric Identities
💡Antiderivatives
💡Natural Logarithm
💡Power Rule
💡Constant of Integration
💡Trigonometric Substitution
💡Half-Angle Identity
💡Foil Method
💡Secant and Tangent
Highlights
The video focuses on different integration techniques in Calculus 2.
Integration by parts is introduced as a method for integrating functions with different components.
The formula for integration by parts is ∫(uv')dx = uv - ∫(vdu).
An example of integrating x*sin(x) dx is provided using integration by parts.
Integration by parts is applied to find the anti-derivative of ln(x) dx.
Trigonometric integrals are discussed, specifically focusing on cosine cubed x.
The Pythagorean identity for trigonometry is used to simplify the integration of cosine cubed x.
Another example is given for integrating a complex trigonometric function involving cosine and sine.
Integration by parts and trigonometric identities are used to simplify the given complex integral.
The video also covers the topic of trigonometric substitution for integration problems.
An example of using trigonometric substitution to find the antiderivative of √(4-x^2)/x^2 dx is provided.
The video explains the use of half-angle identities in integration, specifically for sine squared x.
The antiderivative of cosine 2x is derived using u-substitution.
The video presents a problem involving the integration of tangent and secant functions, showcasing the use of trigonometric identities.
The process of using trigonometric substitution is detailed in solving a specific integration problem.
The final answer for the integration by trigonometric substitution is given, involving the use of cotangent and arc sine.
The video encourages viewers to check out the new Calculus video playlist for more examples on integration techniques.
Transcripts
Browse More Related Video
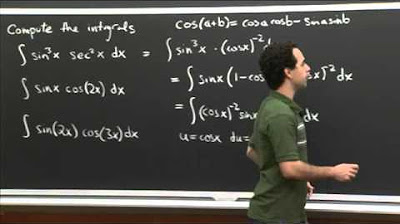
Trig Integral Practice | MIT 18.01SC Single Variable Calculus, Fall 2010
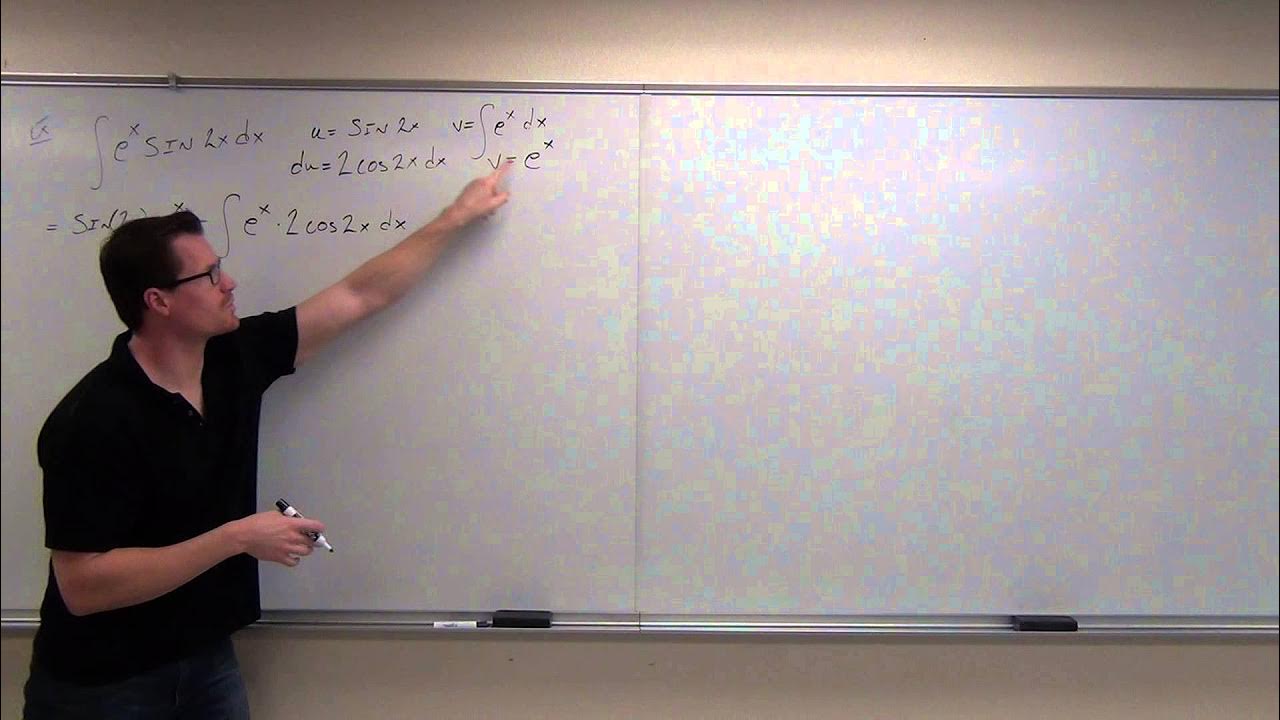
Calculus 2 Lecture 7.1: Integration By Parts
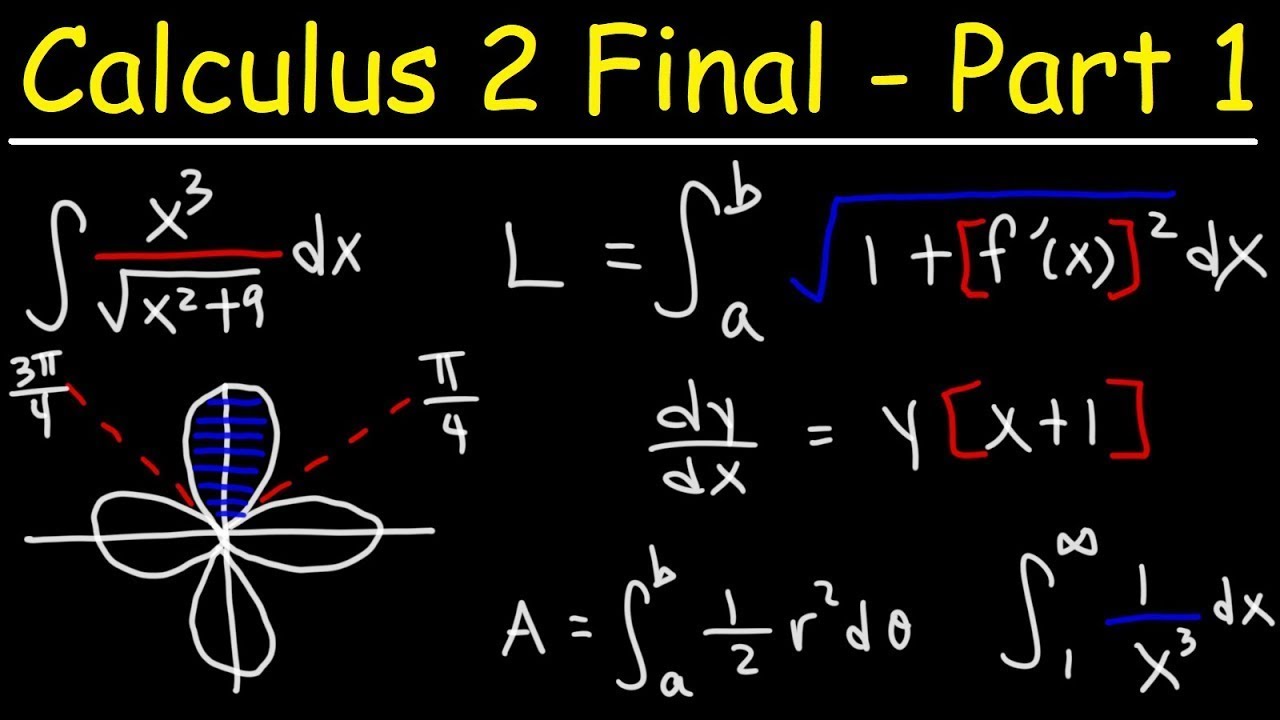
Calculus 2 Final Exam Review -
Lec 28 | MIT 18.01 Single Variable Calculus, Fall 2007

What Integration Technique Should I Use? (trig sub, u sub, DI method, partial fractions) calculus 2
Calculus Chapter 3 Lecture 23 Trig Substitution
5.0 / 5 (0 votes)
Thanks for rating: