Total consumer surplus as area | Microeconomics | Khan Academy
TLDRThe script explains the concept of consumer surplus in the context of an orange stand. It illustrates how the surplus is calculated by the difference between what consumers are willing to pay and the actual price they pay, using the example of 300 oranges sold at $2 each. The total consumer surplus is determined by summing the surplus for each pound, ultimately finding it to be $300, which is the area under the demand curve above the price line.
Takeaways
- 🍊 The script discusses the concept of a demand curve, also known as the marginal benefit curve or willingness to pay for a product, in this case, oranges.
- 📉 The willingness to pay decreases as more units of the product are purchased, indicating a downward-sloping demand curve.
- 💰 The consumer surplus is the difference between what consumers are willing to pay and what they actually pay for a product.
- 🛒 If the price is set at $2 and 300 oranges are sold, the consumer surplus can be calculated for each unit sold.
- 📝 The consumer surplus for an individual unit is the vertical distance between the demand curve and the price line, multiplied by the quantity.
- 📊 To find the total consumer surplus, one can sum up the surplus for each unit or estimate the area under the demand curve above the price line.
- 🔍 For a linear demand curve, the total consumer surplus can be found by calculating the area of a triangle formed by the price line and the demand curve.
- 📚 The area of the triangle is calculated using the formula: 1/2 * base * height, where the base is the quantity sold and the height is the difference between the demand curve and the price.
- 📈 In this scenario, the base is 300 pounds and the height is the $2 difference between the demand curve and the price, resulting in a total consumer surplus of $300.
- 📚 For non-linear demand curves, calculus would be required to find an accurate measure of the total consumer surplus.
- 📝 The script emphasizes the importance of understanding consumer surplus as the additional value consumers receive beyond the price they pay.
Q & A
What is the concept of 'willingness to pay' as mentioned in the script?
-The 'willingness to pay' refers to the maximum amount a consumer is willing to pay for a good or service. In the context of the script, it is the price someone would be willing to pay per pound of oranges at different quantities.
How is the demand curve related to the marginal benefit curve in the script?
-In the script, the demand curve is synonymous with the marginal benefit curve. It represents the value or benefit that consumers derive from each additional unit of the product, which in this case is a pound of oranges.
What is the significance of setting the price at $2 in the script's example?
-Setting the price at $2 is significant because it is below the willingness to pay for the first 100 pounds of oranges. This allows the seller to sell more oranges and also creates consumer surplus for the buyers.
What is consumer surplus and how is it calculated in the script?
-Consumer surplus is the difference between what a consumer is willing to pay for a good and what they actually pay. In the script, it is calculated by finding the area between the demand curve and the price line, which represents the price consumers pay.
How does the script describe the process of calculating the consumer surplus for the 100th pound of oranges?
-The script describes the process by stating that the consumer who bought the 100th pound had a benefit of $3.30 but paid only $2, resulting in a consumer surplus of $1.30 for that pound.
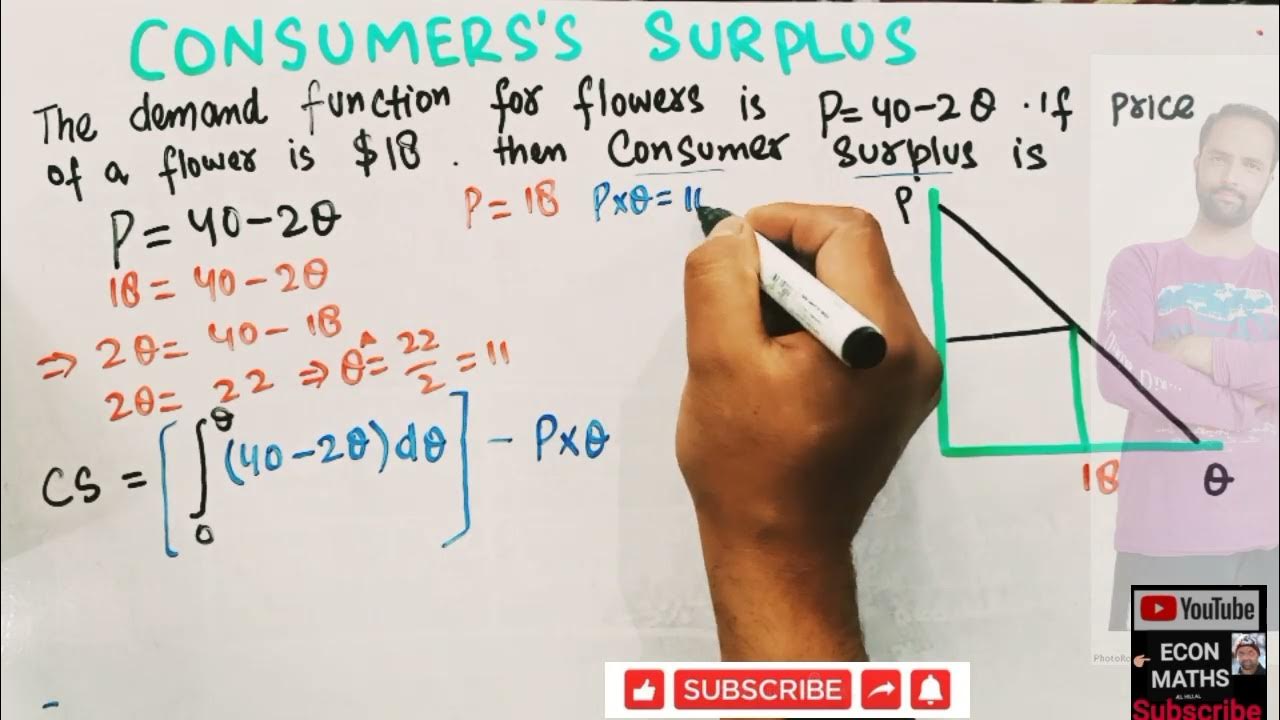
What is the total consumer surplus for the 300 pounds of oranges sold at $2 per pound as per the script?
-The total consumer surplus is $300, which is calculated by finding the area of the triangle formed by the demand curve and the price line at $2, using the formula for the area of a triangle (1/2 * base * height).
Why is the area under the demand curve and above the price line important in the script?
-This area is important because it represents the total consumer surplus, which is the collective benefit consumers gain from the transaction beyond what they paid for the product.
How does the script suggest improving the approximation of consumer surplus for non-linear demand curves?
-The script suggests using smaller rectangles to approximate the area under a non-linear demand curve, which would provide a better estimate of the consumer surplus as the rectangles become thinner and more numerous.
What is the base and height of the triangle used to calculate the total consumer surplus in the script?
-The base of the triangle is the quantity of oranges sold, which is 300 pounds, and the height is the difference between the willingness to pay and the price, which is $1 per pound in this case.
How does the script illustrate the concept of consumer surplus using the example of an orange stand?
-The script uses the example of an orange stand to illustrate consumer surplus by showing how the price set by the seller affects the quantity sold and the surplus gained by consumers, which is the value they receive beyond the price they paid.
What is the implication of the consumer surplus for both consumers and producers in the script?
-The implication is that consumer surplus is a measure of the welfare gained by consumers, and for producers, understanding consumer surplus can help in setting prices that maximize sales and consumer satisfaction.
Outlines
🍊 Understanding Consumer Surplus at an Orange Stand
This paragraph explains the concept of consumer surplus using an orange stand as an example. It introduces the idea of a demand curve, which represents the willingness to pay for oranges, and how it decreases with each additional pound. The script describes a scenario where the price is set at $2, and 300 oranges are sold. The consumer surplus is calculated by determining the difference between what consumers are willing to pay and what they actually pay. The example of the 100th pound of oranges is used to illustrate this, where the consumer benefits from paying $2 but values the orange at $3.30, resulting in a surplus of $1.30. The total consumer surplus is found by summing the surplus for each pound sold, which is visualized as the area between the demand curve and the price line. The explanation simplifies the process by breaking it down into small rectangles under the demand curve, emphasizing the importance of the area calculation for understanding consumer surplus.
📊 Calculating Total Consumer Surplus Using the Triangle Area Formula
The second paragraph continues the discussion on consumer surplus but focuses on calculating the total surplus using the area of a triangle. It explains that the total consumer surplus is the area between the demand curve and the price line set at $2 per pound. The calculation is simplified by considering the linear nature of the demand curve, which allows for a straightforward application of the triangle area formula (1/2 * base * height). The base is the quantity of 300 pounds sold, and the height is the difference between the maximum willingness to pay and the price, which is $2 in this case. The calculation results in a total consumer surplus of $300, emphasizing the straightforward method of estimating the area under the demand curve when the demand curve is linear.
Mindmap
Keywords
💡Demand Curve
💡Marginal Benefit
💡Willingness to Pay
💡Consumer Surplus
💡Price Setting
💡Linear Demand Curve
💡Area Under the Curve
💡Calculus
💡Non-linear Demand Curve
💡Rectangles in Integration
💡Base and Height of a Triangle
Highlights
Introduction of the concept of demand curve and its relation to marginal benefit or willingness to pay for oranges.
Explanation of how the price of $2 is set for selling oranges and the resulting quantity sold, which is 300 oranges.
Definition and calculation of consumer surplus as the difference between what consumers are willing to pay and what they actually pay.
Illustration of consumer surplus for the 100th pound of oranges with a specific example.
Visual representation of consumer surplus through the area between the demand curve and the price line.
Technique of breaking down the demand curve into small rectangles to approximate the area of consumer surplus.
Importance of using smaller rectangles for more accurate approximations, especially with non-linear demand curves.
Calculation of total consumer surplus by summing the areas of rectangles under the demand curve.
Introduction of calculus as a method for estimating consumer surplus with infinitely small rectangles.
Explanation of how consumer surplus can be viewed as the area under the demand curve above the price line.
Demonstration of calculating the total consumer surplus as the area of a triangle using the formula for the area of a triangle.
Use of the base and height of the triangle to calculate the total consumer surplus, resulting in $300.
Emphasis on understanding consumer surplus as the value gained by consumers relative to what they paid for each pound of oranges.
Clarification that the total consumer surplus is the sum of the value consumers gain across all pounds of oranges sold.
Discussion on the practical application of understanding consumer surplus in pricing strategies for businesses.
Highlight of the significance of consumer surplus in economic analysis and its impact on consumer welfare.
Final summary of the process of calculating consumer surplus and its importance in economic decision-making.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: