Integration by Substitution

Professor Monte
5 May 202106:35
EducationalLearning
32 Likes 10 Comments

TLDRIn this informative video, Professor Lonnie dives into the concept of integration by substitution, a technique akin to the reverse of the chain rule in calculus. He illustrates the method with two examples, highlighting how to identify the inside function that would require the chain rule for differentiation. The first example involves integrating (x^2 + 3) to the fifth power, where he demonstrates the substitution process, replacing 2x dx with du, and then solving the integral. The second example, integrating (x^3 e^{x^4}), showcases a more complex scenario where the inside function is (x^4), leading to the substitution u = x^4, and simplification using a constant multiplier. Professor Lonnie emphasizes the importance of checking the solution by differentiating it back and reminds viewers to include the constant C for indefinite integrals. The video is a valuable resource for those looking to understand and practice integration by substitution.

Takeaways
  • ๐Ÿ“š Integration by substitution is the integral equivalent to the chain rule, used to simplify complex integrals.
  • ๐Ÿ” Look for an inside function that would require the chain rule to differentiate, and let a new variable (commonly u) equal to that function.
  • ๐Ÿงฎ To perform the substitution, take the derivative of the inside function with respect to x and include dx, then replace the corresponding dx term in the integral.
  • ๐Ÿ”‘ After substitution, integrate the new expression, remembering to increase the exponent by one and multiply by the reciprocal when dealing with polynomials.
  • ๐Ÿ”„ Always resubstitute the original function back into the integral to express the final answer in terms of the original variable.
  • ๐Ÿง Check your work by differentiating the result to ensure it matches the original integrand, using the chain rule if necessary.
  • ๐Ÿšซ Be aware that not all integrals will be solvable by substitution; if the dx term doesn't match after substitution, a different method may be required.
  • ๐Ÿ“‰ When integrating functions involving x's multiplied by another function, consider either substitution or integration by parts.
  • ๐Ÿค” For the integral of x^3 * e^(x^4), u-substitution is applicable by setting u = x^4, which simplifies the integralๅคงๅคง.
  • ๐Ÿ“ˆ The antiderivative of e^u is simply u, and after integration, resubstitute x^4 for u to find the final answer.
  • ๐Ÿ’ก Remember to include the constant of integration (+C) in indefinite integrals, as it represents the family of all functions that could be the antiderivative.
  • ๐Ÿ‘ Engage with the content by liking, subscribing, and requesting future topics in the comments for further assistance.
Q & A

  • What is the main concept behind integration by substitution?

    -Integration by substitution is an integral technique that is the reverse of the chain rule for differentiation. It involves finding an inside function where the derivative would require the chain rule, and then substituting it with a new variable to simplify the integral.

  • What is the purpose of the substitution variable, often denoted as 'u' in integration?

    -The substitution variable 'u' is used to replace a complex function or an expression within the integral to simplify the integral. It is chosen in a way that its derivative is simpler and can be easily canceled out when the integral is transformed.

  • How do you determine the inside function for substitution?

    -The inside function for substitution is typically determined by looking for a function within the integral that, if you were to take its derivative, would require the application of the chain rule.

  • What is the process to perform integration by substitution?

    -The process involves: (1) choosing the inside function and setting it equal to 'u', (2) finding the derivative of 'u' with respect to 'x' (du/dx), (3) replacing the part of the integral that includes 'u' with 'du', (4) simplifying the integral, (5) integrating the new expression, and (6) substituting 'u' back with the original function to get the final answer.

  • Why is it important to include the 'dx' when taking the derivative of 'u'?

    -Including 'dx' is important because it signifies that 'u' is a function of 'x', and the derivative du/dx is with respect to the variable 'x', not 'u'. It ensures the correct application of the chain rule when transforming the integral.

  • What is the purpose of multiplying by a constant, like 1/4, in the second example provided in the script?

    -Multiplying by a constant, such as 1/4, is done to make the derivative of 'u' (du) match the form of the expression in the integral so that it can be canceled out, simplifying the integral and making it easier to solve.

  • How do you check if your answer to an integral is correct?

    -You can check the correctness of your integral by differentiating the result and comparing it with the original integrand. If the derivative of the result matches the original integrand, then the integral is correctly solved.

  • Why is it necessary to include a 'plus c' when you have an indefinite integral?

    -The 'plus c' is included because indefinite integrals represent a family of functions that are derivatives of the original function. The constant 'c' accounts for the unknown constant of integration that would be present in any function that has been differentiated.

  • What is the significance of the limits of integration in an integral?

    -The limits of integration specify the range over which the integral is evaluated. They are crucial when calculating a definite integral, which gives a specific numerical value as opposed to an indefinite integral, which gives a general function.

  • What is the role of the chain rule in the context of integration by substitution?

    -The chain rule is implicitly used in the reverse process during integration by substitution. It helps in identifying the inside function that requires the chain rule for its derivative, which is then replaced by a new variable to simplify the integral.

  • Why might integration by parts be considered instead of substitution in certain integrals?

    -Integration by parts is considered when the integral involves products of functions and substitution does not yield a straightforward simplification. It is particularly useful for integrals of the form โˆซu dv, where 'u' and 'dv' are chosen based on certain criteria to make the resulting integrals easier to solve.

  • How does the script illustrate the process of integration by substitution with the example of x squared plus 3?

    -The script illustrates the process by setting u = x^2 + 3, finding du/dx = 2x dx, and then replacing 2x dx with du in the integral. It then integrates u to the power of 5, resubstitutes x^2 + 3 for u, and finally checks the result by differentiating.

Outlines
00:00
๐Ÿ“š Introduction to Integration by Substitution

Professor Lonnie begins the video with an introduction to the concept of integration by substitution, which is essentially the integral counterpart to the chain rule in differentiation. He explains that the method is used to find integrals of composite functions by looking for an 'inside function' that would require the application of the chain rule when differentiating. Lonnie provides an example where the inside function is x squared plus 3, and demonstrates the substitution process by letting u equal this expression. He then shows how to take the derivative of u, and how to rewrite the integral in terms of u, before integrating and substituting back to get the final answer in terms of x. The importance of checking the solution by differentiating is also emphasized.

05:03
๐Ÿ” Applying Integration by Substitution with e^x

The second paragraph delves into another example of integration by substitution, this time involving the function x cubed times e to the power of x to the fourth. Lonnie illustrates how to identify the inside function, which in this case is x to the fourth, and how to set up the substitution with u. He then guides through the process of differentiating u with respect to x, multiplying by a constant to cancel out terms, and simplifying the integral to a form that can be easily integrated. The anti-derivative of e to the u is found, and the constant of integration is included. After integrating, Lonnie resubstitutes to express the final answer in terms of x. He also demonstrates the verification process by differentiating the result to confirm the correctness of the solution. The video concludes with a reminder to include the constant of integration for indefinite integrals and an invitation for viewers to like, subscribe, and request future video topics.

Mindmap
Keywords
๐Ÿ’กIntegration by substitution
Integration by substitution is a method used to evaluate integrals by treating them as a composition of functions. It is analogous to the chain rule in differentiation. In the video, Professor Lonnie uses this technique to solve complex integrals by finding a suitable inside function (u), differentiating it to find du, and then substituting back to the original variable (x) after integrating with respect to u.
๐Ÿ’กChain rule
The chain rule is a fundamental theorem in calculus for differentiating composite functions. In the context of the video, it is mentioned to illustrate the reverse process of integration by substitution. When integrating a complex function, one might need to apply the chain rule in reverse to find a suitable substitution variable (u).
๐Ÿ’กInside function
An inside function is the part of the integrand that, if being differentiated, would require the application of the chain rule. In the video, Professor Lonnie identifies the inside function as a critical step in the substitution method. For example, in the integral of x^2 + 3, x^2 + 3 is the inside function that is chosen as u for substitution.
๐Ÿ’กDerivative
A derivative in calculus represents the rate of change of a function with respect to its variable. In the video, derivatives are used to find the substitution variable (du) from the inside function. For instance, when u is set to x^2 + 3, the derivative du is found to be 2x dx, which is then used in the substitution process.
๐Ÿ’กLeibniz notation
Leibniz notation is a form of notation used in calculus, named after the mathematician Gottfried Wilhelm Leibniz. It is often used to express the derivative of a function. In the video, Professor Lonnie briefly mentions this notation as a way to represent the derivative du/dx when explaining the substitution process.
๐Ÿ’กExponential function
An exponential function is a mathematical function of the form f(x) = a^x, where a is a constant. In the video, exponential functions are used in the context of integrals that can be solved using substitution. For example, e^(x^4) is an exponential function that is integrated using the substitution method with u = x^4.
๐Ÿ’กAnti-derivative
An anti-derivative, also known as an integral, is a function whose derivative is equal to the original function. In the context of the video, finding the anti-derivative is the goal of the integration process. For example, after substituting u for x^4, the anti-derivative of e^u is found to be (1/4)e^u + C.
๐Ÿ’กResubstitution
Resubstitution is the process of replacing the substitution variable (u) back with the original function in the integral after integrating with respect to u. In the video, resubstitution is used to express the final answer in terms of the original variable (x) after the integration process is complete.
๐Ÿ’กConstant of integration (C)
The constant of integration (C) is added to the result of an indefinite integral to account for the possibility of an infinite number of original functions that could have been differentiated to produce the given integrand. In the video, Professor Lonnie reminds viewers to include the constant of integration in their final answers.
๐Ÿ’กIntegration by parts
Integration by parts is another technique used in calculus for integrating products of functions. It is mentioned in the video as an alternative method to substitution when dealing with integrals involving x's multiplied by something else with x's. It is not demonstrated in the video but is acknowledged as a potential approach for certain types of integrals.
๐Ÿ’กLimits of integration
Limits of integration refer to the boundaries within which an integral is evaluated. When an integral has limits, it is considered a definite integral, and the constant of integration is not included in the final answer. In the video, Professor Lonnie notes that if there are limits of integration, the plus C is not necessary.
Highlights

Integration by substitution is the integral equivalent to the chain rule.

The process involves finding an inside function where the chain rule would be applied when taking the derivative.

An example is given where the inside function is x squared plus 3, and u is set to this expression.

Derivative of u (du) is found to be 2x dx, which is then used to simplify the integral.

Leibniz notation is mentioned as an alternative way to express the derivative du/dx.

The integral is simplified by replacing 2x dx with du.

After integrating, the result is expressed in terms of u, which is then resubstituted back to x for the final answer.

Checking the solution by differentiating and confirming it matches the original integral expression is advised.

A second example is presented where the integral does not work out as nicely due to the absence of a direct 2x dx term.

The importance of having the x to the first power in the integral for the substitution method to work is emphasized.

An alternative problem of integrating x cubed times e to the x to the fourth is solved using substitution, with u set to x to the fourth.

A trick of multiplying by 1/4 is used to cancel out terms and simplify the integral.

The anti-derivative of e to the u is found, and then resubstituted to provide the final answer.

Derivatives are used to check the correctness of the integral solutions.

The necessity of including a plus c in indefinite integrals to account for the family of functions is explained.

The video encourages practice and provides guidance on when to use integration by substitution versus other methods like integration by parts.

The key to successful integration by substitution is identifying the inside function that would require the chain rule for differentiation.

The video concludes with an invitation for viewers to like, subscribe, and comment for more content.

Transcripts

Browse More Related Video

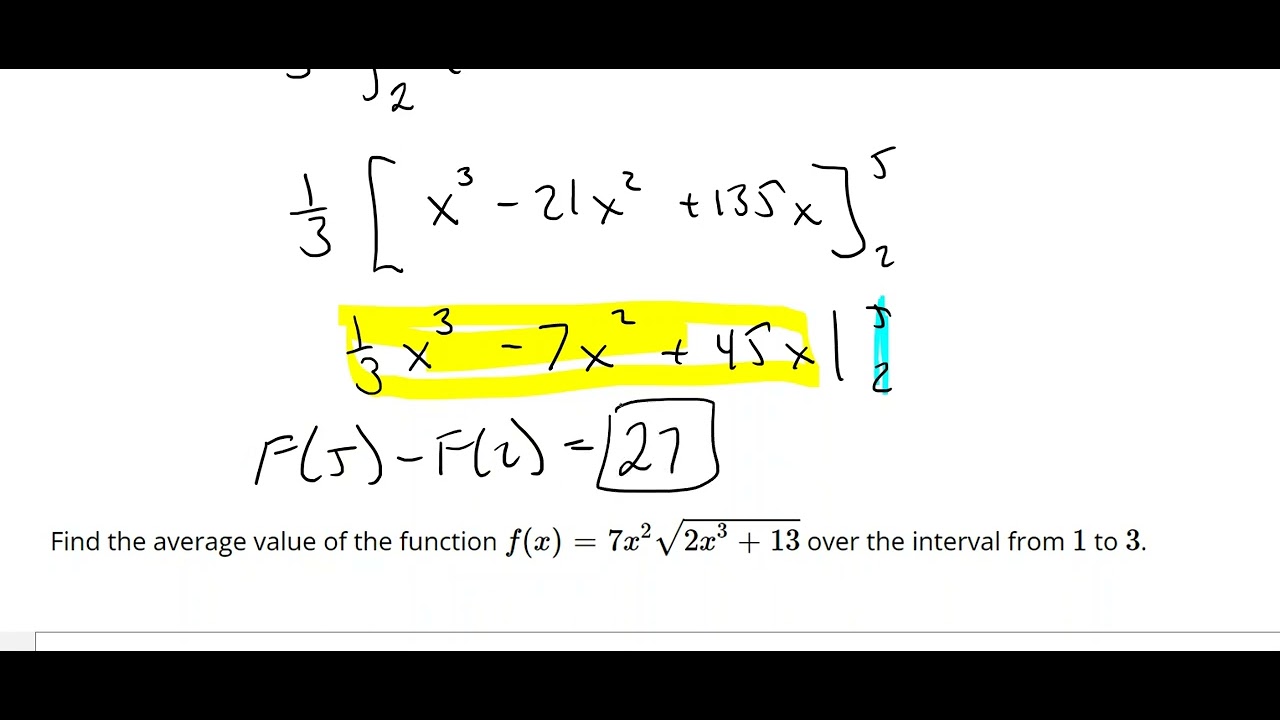
Average Value of a Function

Fundamental Theorem of Calculus: Changing the Bounds with u-Substitution

Integration Using The Substitution Rule

Find The Definite Integral Of x3 Into 3 Plus x Power 4 All Power -2 [Evaluate The Definite Integral]

Calculus Divide and Integrate

Calculus AB Homework 6.7 U-Substitution

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
IntegrationSubstitutionMathematicsEducationChain RuleCalculusProfessor LonnieDerivativeIntegralLearningMath Tutorial