BusCalc 13.6 Integral Applications
TLDRThe transcript appears to be a detailed calculus lesson focusing on the concepts of definite integrals, antiderivatives, and their applications in calculating areas between curves and solving real-world problems such as business revenue and profit calculations. The instructor explains the process of evaluating definite integrals by finding antiderivatives, emphasizing the cancellation of the constant 'C' in definite integrals. They also cover how to handle areas below the x-axis by considering them as negative contributions to the integral. The lesson further explores techniques like u-substitution and integration by parts for more complex integrals. Practical examples, including calculating the total profit for a company over a five-year period, are used to illustrate the application of these concepts. The instructor also discusses the use of graphing tools like Desmos to visualize the integrals and areas, which can be particularly helpful for understanding the subject matter.
Takeaways
- 📚 The definite integral of a function can be evaluated as the antiderivative of the function at the upper bound minus the antiderivative at the lower bound.
- 🔢 When evaluating definite integrals, the constant 'C' from the antiderivative is canceled out, so it's not necessary to include it.
- 🏞️ To find the area between a curve and the x-axis, you may need to split the integral into parts to account for areas above and below the x-axis.
- 📈 The process of finding areas between curves involves determining which curve is on top, finding intersection points, and setting up the integral with the top curve minus the bottom curve.
- 🧮 For a definite integral, the antiderivative is evaluated at the upper and lower bounds, and the results are subtracted from each other to find the area.
- 🤔 When dealing with complex antiderivatives that cannot be found exactly, numerical methods or approximations can be used to estimate the integral.
- 📝 The antiderivative power rule states that if you have a function f(x) = x^n, then its antiderivative F(x) is (x^(n+1))/(n+1) + C.
- 🔑 U-substitution is a technique used in integration where a new variable 'u' is set equal to a function of 'x', and the integral is then solved in terms of 'u'.
- 🛠️ Integration by parts is a method used for integrating products of functions, and it involves using a table to organize the integration process.
- 📉 The Fundamental Theorem of Calculus connects the concept of the definite integral to the antiderivative, allowing for the calculation of areas under curves.
- 📊 In business calculus, the focus is on teaching the most important and simplest cases of calculus that are likely to be useful in practical applications.
- 💡 For word problems involving revenue and costs, the total profit over a period can be found by integrating the rate of profit, which is the revenue rate minus the cost rate.
Q & A
What is the process to evaluate a definite integral with an antiderivative?
-To evaluate a definite integral with an antiderivative, you find the antiderivative (capital F) of the integrand function (lowercase f), then evaluate this antiderivative at the upper bound and subtract the evaluation of the antiderivative at the lower bound. The antiderivative represents the accumulated area under the curve from one point to another.
Why do we not need to include the constant 'C' when evaluating a definite integral?
-The constant 'C' represents an arbitrary constant that can be added to any antiderivative. However, in the case of a definite integral, the 'C' terms cancel out when you subtract the antiderivative evaluated at the lower bound from the antiderivative evaluated at the upper bound, so it's not necessary to include it.
How does the process of finding the area under a curve change when the curve dips below the x-axis?
-When a curve dips below the x-axis, the area under the curve in that region is considered negative. To find the total positive area, you would calculate the definite integral from the lower to the upper bound and take the absolute value of the result if the integral is negative.
What is the Fundamental Theorem of Calculus, and how does it relate to definite integrals?
-The Fundamental Theorem of Calculus connects differentiation and integration. It states that if you have a continuous function and its antiderivative (also known as an indefinite integral or primitive), the definite integral from a to b of the function is equal to the antiderivative evaluated at b minus the antiderivative evaluated at a.
How do you find the area between two curves?
-To find the area between two curves, you first determine which curve is above the other in the region of interest. Then, you find the points of intersection of the two curves. The area between the curves is given by the definite integral of the difference of the top curve's function minus the bottom curve's function, evaluated from the lower intersection point to the upper intersection point.
What is the antiderivative of x squared?
-The antiderivative of x squared is (1/3)x cubed. When finding antiderivatives, you apply the power rule which involves increasing the exponent by 1 and then dividing by the new exponent.
How do you find the total profit earned by a company over a period of time, given the rate of revenue and rate of cost?
-To find the total profit, you first determine the rate of profit by subtracting the rate of cost from the rate of revenue. Then, you find the antiderivative of the rate of profit function, which gives you the profit function. Finally, you evaluate the profit function at the endpoints of the time period and subtract the lower bound evaluation from the upper bound evaluation.
What is the derivative power rule, and how does it differ from the antiderivative power rule?
-The derivative power rule states that the derivative of x to the power n is n times x to the power (n-1). The antiderivative power rule is the reverse: the antiderivative of x to the power n is (1/(n+1)) times x to the power (n+1). Essentially, the derivative decreases the exponent by 1 and multiplies by that exponent, while the antiderivative increases the exponent by 1 and divides by the new exponent.
Why is it important to graph the curves when finding the area between them?
-Graphing the curves helps visualize which curve is above the other in the region of interest and assists in identifying the points of intersection accurately. It also aids in understanding the shape of the area between the curves, which is crucial for setting up the correct integral to calculate the area.
What is the role of the constant 'C' in antiderivatives and definite integrals?
-The constant 'C' is used in antiderivatives to represent the constant of integration, which accounts for an infinite number of original functions that could have been differentiated to produce the given function. However, in definite integrals, the 'C' cancels out because the definite integral is a difference of two antiderivative evaluations, and the constants subtract to zero.
How does the process of finding the area between curves relate to the concept of integration by parts or u-substitution?
-Integration by parts and u-substitution are methods used to find the antiderivative of complex functions when the antiderivative cannot be found using basic rules. These methods are crucial before applying the Fundamental Theorem of Calculus to find areas between curves, as they allow you to find the necessary antiderivative to set up the definite integral.
Outlines
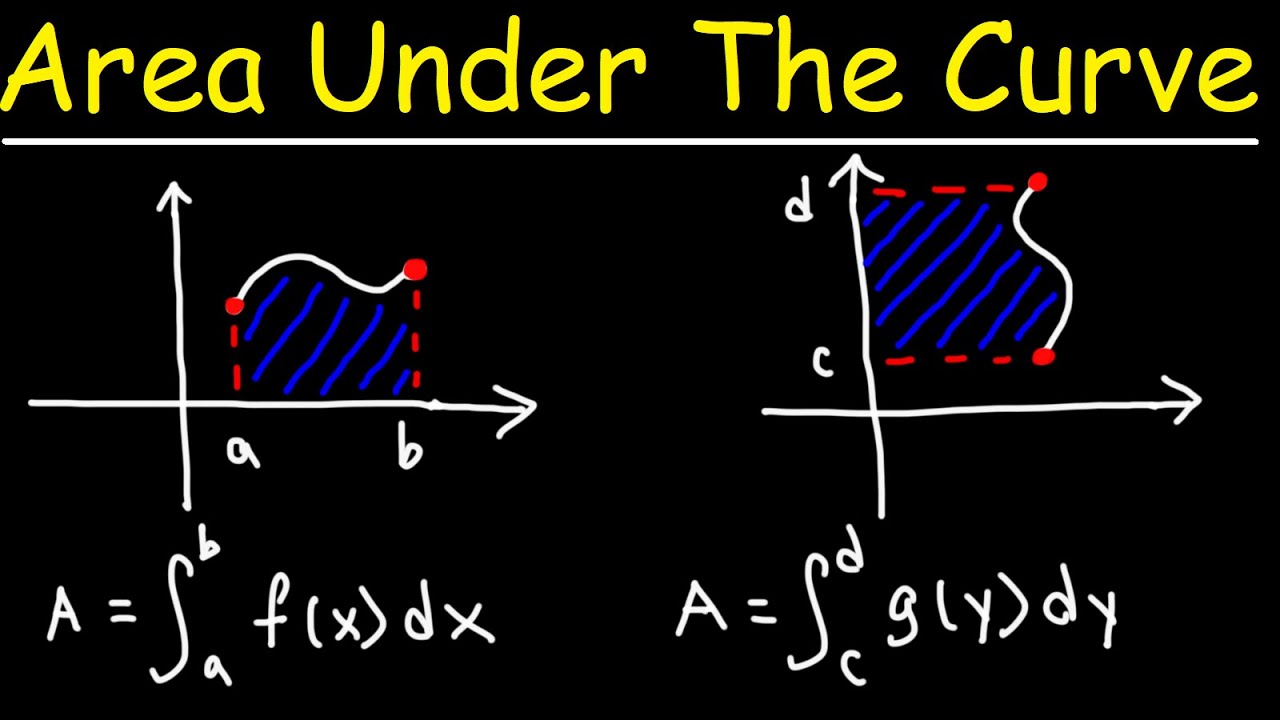
📚 Understanding Definite Integrals
This paragraph explains the concept of definite integrals, emphasizing the evaluation of an antiderivative at the upper and lower bounds. It clarifies that the constant 'C' from indefinite integrals cancels out in definite integrals. The process is exemplified using the function f(x) = x^2 from 0 to 3, illustrating the calculation of the antiderivative and the final integral result.
📈 Calculating Areas Between Curves
The second paragraph delves into finding the total area between a curve, the x-axis, and two vertical lines at x = 0 and x = 3. It discusses the importance of considering the sign of the area (positive or negative) and how to split the integral into two parts to account for areas above and below the x-axis. The process is demonstrated using a function f(x) = 1/4 * x^2 - 1, with the integral split between x = 0 and x = 2, and x = 2 and x = 3.
🧮 Antiderivatives and the Power Rule
The third paragraph focuses on finding the antiderivative of a given function and evaluating it at specific points. It contrasts the derivative power rule, where the exponent is decreased by one, with the antiderivative power rule, where the exponent is increased by one. The process is shown by finding the antiderivative of a function and evaluating it at x = 3, x = 2, and x = 0, emphasizing the importance of the constant 'C' in antiderivatives.
🔢 Evaluating Definite Integrals for Total Area
The fourth paragraph discusses the calculation of definite integrals to find the total area under a curve between two bounds. It highlights the use of Desmos for verification and the importance of splitting the integral into sections to account for areas below the x-axis. The process is shown by calculating the area under the curve y = 1/4 * x^2 - 1 from x = 0 to x = 3, resulting in a total area of 1.91.
🧮 Antiderivative Rules and Substitutions
The fifth paragraph reviews the basic antiderivative rules and substitution methods. It emphasizes the power rule, the antiderivative of e^(kx), and the natural log function. It also discusses the technique of u-substitution, where a suitable substitution is made to simplify the integral, followed by reversing the substitution after finding the antiderivative.
📝 Integration by Parts and Riemann Sums
The sixth paragraph covers integration by parts, a method for finding antiderivatives of products of functions. It explains the process of setting up a table to link terms and how to simplify the expression. It also touches on Riemann sums as a method for approximating the area under a curve and the fundamental theorem of calculus that connects definite integrals with antiderivatives.
🔢 Definite Integrals and Their Evaluation
The seventh paragraph provides a detailed example of evaluating a definite integral. It shows the process of finding the antiderivative of a function and then evaluating it at the upper and lower bounds of the integral. The example demonstrates the calculation for the integral of a function from x = 0 to x = 3, resulting in a value of 12.
🤔 The Challenge of Antiderivatives
The eighth paragraph discusses the challenges associated with finding antiderivatives, noting that some functions do not have an antiderivative that can be expressed using elementary functions. It also mentions the use of Desmos for evaluating definite integrals and the importance of having a strong imagination for visualizing integrals without a calculator.
🧮 U-Substitution and Antiderivative Evaluation
The ninth paragraph illustrates the use of u-substitution to find the antiderivative of a function. It shows the process of selecting a suitable u variable, solving for dy, and substituting back to find the antiderivative. The example provided involves the natural log function and demonstrates the calculation of the definite integral from y = 1 to y = 4.
📈 Finding the Area Between Two Curves
The tenth paragraph outlines the procedure for finding the area between two curves. It involves graphing both curves, determining the curves' intersection points, and setting up a definite integral using the top curve minus the bottom curve as the integrand. The example demonstrates finding the area between y = x^2 and y = 1 - x^2, using the intersection points as the bounds of integration.
💰 Calculating Total Profit Over a Period
The eleventh paragraph applies the concept of definite integrals to calculate total profit over a five-year period for a company like Frito-Lay. It discusses the process of finding the rate of profit and then using the definite integral to sum the total profit, with the bounds of integration corresponding to the years 2010 to 2015.
🧮 Evaluating Profit and Checking Results
The twelfth paragraph continues the application of definite integrals to find total profit, focusing on the antiderivative of the profit rate function. It demonstrates the calculation of the total profit earned by Frito-Lay from 2010 to 2015, emphasizing the need for careful evaluation and checking of the results.
🤔 Reflecting on Calculations and Confirming Results
The thirteenth paragraph briefly reflects on the calculation process and seeks confirmation of the results obtained for the total profit calculation. It acknowledges the possibility of errors and emphasizes the importance of accuracy in mathematical computations.
Mindmap
Keywords
💡Definite Integral
💡Anti-derivative
💡Fundamental Theorem of Calculus
💡Natural Logarithm
💡U-Substitution
💡Integration by Parts
💡Riemann Sums
💡Derivative Power Rule
💡Exponential Function
💡Area Between Curves
💡Profit Function
Highlights
The definite integral is evaluated as the antiderivative of the function at the upper bound minus the antiderivative at the lower bound.
The antiderivative of x squared is one third x cubed, following the power rule for antiderivatives.
When evaluating a definite integral, the constant of integration (+C) cancels out and is not required.
The process of finding the total area between a curve and the x-axis involves breaking the integral into parts to account for areas above and below the x-axis.
The use of definite integrals to calculate areas involves finding the antiderivative and then applying the fundamental theorem of calculus.
Desmos can be used to verify the results of definite integrals and visualize the area under a curve.
Integration by parts and u-substitutions are techniques used to find antiderivatives for complex functions.
The antiderivative power rule involves increasing the exponent by one and dividing by the new exponent.
The definite integral from 0 to 3 of a function can be split into two parts to account for changes in the curve's position relative to the x-axis.
The area between two curves is found by determining where the curves intersect and setting up a definite integral with the top curve minus the bottom curve.
The revenue and cost functions can be used to determine the rate of profit, which is integral to calculating total profit over a period.
Total profit can be calculated using the definite integral of the profit rate function over a given time period.
The process of finding antiderivatives can be complex, and some functions do not have elementary antiderivatives that can be found using standard methods.
Logarithmic functions always pass through the point (1, 0), which is a key property used in evaluating definite integrals involving logarithms.
The natural logarithm is the inverse function of the exponential function, which is a fundamental concept in calculus.
The area between curves is calculated by finding the intersection points and evaluating the definite integral of the difference of the top and bottom functions.
The procedure for finding the area between two curves involves graphing, determining intersections, and integrating the difference of the functions.
In business calculus, the focus is on teaching the most important and simplest cases of calculus that are likely to be used in practical applications.
Transcripts
Browse More Related Video

Definite Integrals!

4.3 - Area and Definite Integrals

Calculus 1 Lecture 4.5: The Fundamental Theorem of Calculus

4.4 - Properties of Definite Integrals

Definite Integral Calculus Examples, Integration - Basic Introduction, Practice Problems
Finding The Area Under The Curve Using Definite Integrals - Calculus
5.0 / 5 (0 votes)
Thanks for rating: