Definite Integral Calculus Examples, Integration - Basic Introduction, Practice Problems

The Organic Chemistry Tutor

19 Dec 201633:51

EducationalLearning

32 Likes 10 Comments

TLDRThe video script is an educational walkthrough of solving various definite integral problems. It covers the use of basic power rule for antiderivatives, integration of polynomial functions, and special techniques like u-substitution and integration by parts for more complex integrals. The examples include integrating functions like x^3, 5x, polynomials, and trigonometric functions, with a focus on finding antiderivatives and evaluating them between given intervals. The script also introduces the concept of using substitution to simplify integrals and emphasizes the importance of knowing the derivatives of common functions for integration.

Takeaways

📚 To find the antiderivative of x to the n, use the formula x to the n+1 divided by n+1.
🔢 For the integral of x cubed from 2 to 4, evaluate (4^4/4) - (2^4/4) to get the result of 60.
🅰️ The antiderivative of a constant is simply the constant times x, as seen with the integral of 5dx from 4 to 10, which equals 5x.
📈 When dealing with polynomial functions, find the antiderivative of each term separately and then combine them.
🤹‍♂️ For the integral of 2x + 3 squared from -1 to 3, first use FOIL to simplify the expression before integrating.
📉 The antiderivative of 1/x squared is x, which can be found by rewriting the expression as x to the negative two and applying the power rule.
🌟 The antiderivative of e to the 3x is (e to the 3x)/3, and when evaluated from 0 to 1, it equals e/3 - 1/3 ≈ 0.5728.
📊 The antiderivative of cosine x is sine x, and for the integral from pi/6 to pi/3, the result is √3 - 1/2 or approximately 0.366.
🧮 For the integral of secant squared dx, the antiderivative is tangent x. The derivative of tangent is secant squared, which helps in solving such integrals.
🔄 U-substitution is a powerful technique for integrating complex functions, as demonstrated by replacing x^3 + 5 with u and solving for dx/(3x^2).
🔧 Integration by parts is another method used when u-substitution isn't applicable, as shown in the integral of x * e^(x squared) from 0 to 1.

Q & A

What is the antiderivative of x cubed evaluated from 2 to 4?
-The antiderivative of x cubed is (x^4)/4. Evaluating from 2 to 4, we plug in the upper limit (4) and subtract the value at the lower limit (2): ((4^4)/4 - (2^4)/4) = (64/4 - 16/4) = 60.
How do you find the antiderivative of a constant?
-The antiderivative of a constant is simply the constant times x. For example, the antiderivative of 5 is 5x.
What is the antiderivative of 3x^2 - 5x + 2 evaluated from 1 to 2?
-The antiderivative is 3x^3/3 - 5x^2/2 + 2x. Evaluating from 1 to 2 gives us (3(2^3)/3 - 5(2^2)/2 + 2(2)) - (3(1^3)/3 - 5(1^2)/2 + 2(1)) = 8 - 4 + 4 - 5/2 + 1 = 3/2.
How do you find the antiderivative of 2x + 3^2 using the FOIL method?
-The FOIL method is used for multiplying binomials. For 2x + 3^2, we treat it as (2x * 2x) + (2x * 3) + (3 * 2x) + (3 * 3), which simplifies to 4x^2 + 6x + 6x + 9, combining like terms gives 4x^2 + 12x + 9, and the antiderivative is (4x^3/3) + (12x^2/2) + 9x.
What is the antiderivative of 1/x^2 evaluated from 1/2 to 1?
-The antiderivative of 1/x^2 is x^(-1) or ln|x|. Evaluating from 1/2 to 1 gives us ln(1) - ln(1/2), which simplifies to ln(1) + ln(2), and since ln(1) = 0, the result is ln(2), which is approximately 0.6931.
What is the antiderivative of the square root of x?
-The antiderivative of the square root of x (x^(1/2)) is (x^(3/2))/(3/2) or (2/3)x^(3/2). Evaluating from 4 to 9 gives us (9^(3/2))/(3/2) - (4^(3/2))/(3/2) = 38/3.
How do you find the antiderivative of x^3 - 5x^2/x using partial fraction decomposition?
-We first separate the terms: x^3/x^2 and -5x^2/x. This simplifies to x and -5x. The antiderivative of x is (x^2)/2, and the antiderivative of -5x is (-5x^2)/2. Evaluating from 2 to 3 gives us (3^2/2 - 2^2/2) - (-5(3^2)/2 + 5(2^2)/2) = -37/6.
What is the antiderivative of e^(3x)?
-The antiderivative of e^(ax) where a is a constant, is (1/a)e^(ax). So for e^(3x), it's (1/3)e^(3x).
What is the antiderivative of cosine x?
-The derivative of sine x is cosine x, so the antiderivative of cosine x is sine x. Evaluating from pi/3 to pi/6 gives us (sin(pi/3) - sin(pi/6)) which is (sqrt(3)/2 - 1/2).
What is the antiderivative of sine 2x?
-The derivative of negative cosine is positive sine, so the antiderivative of sine 2x is negative cosine 2x divided by 2. Evaluating from 0 to pi/2 gives us (-cos(2*pi/2)/2 + cos(0)/2) which simplifies to 1/2.
What is the antiderivative of secant squared dx?
-The derivative of tangent is secant squared, so the antiderivative of secant squared is tangent. Evaluating from pi/4 to 3*pi/4 gives us (tan(3*pi/4) - tan(pi/4)), which is -2.
How do you use u-substitution to find the antiderivative of x^3 + 5?
-We set u = x^3 + 5 and du = (3x^2)dx. Solving for dx gives dx = du/(3x^2). The antiderivative becomes (1/3)e^u evaluated from u=5 at x=0 to u=6 at x=1, which gives (1/3)e^6 - (1/3)e^5.
How do you use integration by parts to find the antiderivative of x * e^(x^2)?
-We set u = x and dv = e^(x^2)dx. Then du = dx and v = (1/2)e^(x^2). Using the integration by parts formula, the antiderivative is uv - ∫vdu, which simplifies to x * e^(x^2) - (1/2)e^(x^2) evaluated from 0 to 1, giving 1 - e.

Outlines

00:00

📚 Solving Definite Integral Problems

This paragraph introduces the topic of definite integrals and presents a method to solve a specific problem involving the antiderivative of x to the third power. The explanation includes a review of basic integration rules, such as the power rule, and demonstrates how to evaluate the integral from 2 to 4. The process involves finding the antiderivative (x to the fourth divided by 4) and then applying the limits of integration to obtain the final answer of 60.

05:02

📈 Antiderivatives of Polynomial Functions

This section delves into finding the antiderivatives of polynomial functions, illustrating the process with an example where the function is 3x squared minus 5x plus 2, integrated from 1 to 2. The explanation breaks down the process term by term, applying the power rule to each term, and then evaluates the resulting expression using the given limits. The final answer is derived by combining the results of each term's integration, leading to 3/2 as the answer.

10:04

🌟 Advanced Integration Techniques

This paragraph discusses advanced integration techniques, such as FOILing and u-substitution. It presents a problem involving the integral of (2x plus 3 squared) from -1 to 3 and explains the steps to solve it using the FOIL method. The explanation then moves on to u-substitution, introducing the technique and applying it to a different problem involving the integral of x cubed plus 5 with respect to x. The paragraph concludes with the solution, which is 364/3.

15:04

📊 Integration of Trigonometric Functions

This section focuses on the integration of trigonometric functions, starting with the antiderivative of one over x squared and then moving on to the antiderivative of the square root of x. The explanation provides the power rule for these cases and evaluates the integrals from specific limits, resulting in final answers of 1 and 38/3, respectively. The paragraph also covers the integration of e to the 3x using u-substitution, concluding with the answer of e/3 - 1/3.

20:04

🔄 Integration by Parts

This paragraph introduces the concept of integration by parts, a technique used when direct integration is not feasible. The explanation walks through the process of applying this method to the integral of x times e to the x squared. By identifying u as x and dv as e to the x dx, the paragraph demonstrates how to apply the integration by parts formula to arrive at the final answer, which is e - x times e to the x.

Mindmap

Keywords

💡Definite Integral

A definite integral represents the signed area under a curve between two points on the x-axis. In the video, the concept is used to solve various problems by finding the antiderivative of functions and evaluating them at given intervals, such as from 2 to 4 for x^3.

💡Antiderivative

An antiderivative is a function whose derivative is the given function. In the context of the video, finding the antiderivative of functions like x^n is a fundamental step in calculating definite integrals.

💡Power Rule

The power rule states that the derivative of x^n (where n is a constant) is nx^(n-1). In the video, the power rule is used to find the antiderivative of functions by increasing the exponent by 1 and dividing by the new exponent.

💡Constant

In the context of integral calculus, a constant can be integrated directly as 'cx', where 'c' is the constant and 'x' is the variable. The video explains that the antiderivative of a constant is simply the constant times 'x'.

💡Polynomial Function

A polynomial function is an expression consisting of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. In the video, polynomial functions are integrated term by term.

💡FOIL

FOIL is a method for multiplying two binomials (two terms in each expression). It stands for First, Outer, Inner, Last, which refers to the order in which the terms are multiplied. In the video, FOIL is used to expand expressions before integrating.

💡U-Substitution

U-substitution is a technique used in integration to simplify the integrand by letting u be an expression involving the variable of integration. It helps in integrating complicated functions by transforming them into more manageable forms.

💡Integration by Parts

Integration by parts is a method for integrating a product of two functions. It is based on the formula ∫u dv = uv - ∫v du, where u and v are functions of x. This technique is used when other integration methods are not applicable.

💡Trigonometric Functions

Trigonometric functions are functions of angle and are commonly used in mathematics and physics. In the video, the antiderivative of trigonometric functions like cosine and sine are found using their respective derivatives.

💡Chain Rule

The chain rule is a technique used in calculus to find the derivative of a composite function. It involves differentiating the outer function and then multiplying by the derivative of the inner function. In the context of the video, the chain rule is not explicitly mentioned but is implied when dealing with more complex functions.

Highlights

The antiderivative of x^n is found using the formula x^(n+1)/(n+1).

The antiderivative of x^3 is calculated by evaluating x^4/4 from 2 to 4, resulting in a final answer of 60.

For a constant's antiderivative, it simplifies to just the constant times x, as seen with the antiderivative of 5 being 5x.

The antiderivative of a polynomial function involves finding the antiderivative of each term separately, as demonstrated with 3x^2 - 5x + 2.

The process of FOILing (First, Outer, Inner, Last) is used to simplify the expression 2x + 3 squared before integrating.

The antiderivative of 1/x^2 is found by rewriting the expression as x^(-2) and applying the power rule, resulting in ln|x|.

The antiderivative of the square root of x, or x^(1/2), is found by using the power rule to transform it to (2/3)x^(3/2).

For the antiderivative of x^3 - 5x^2/x, the fraction is simplified by separating it into two terms: x^2 and -5x.

The antiderivative of e^(3x) is found using the technique of dividing the exponent by the corresponding power, resulting in (e^(3x))/3.

The antiderivative of cosine x is sine x, and the example provided evaluates to (sqrt(3) - 1/2) as the final answer.

The antiderivative of sine 2x is found by dividing by the coefficient of x and using the relationship between sine and cosine, resulting in (-cos(2x)/2).

The antiderivative of secant squared is tangent, as the derivative of tangent is secant squared.

U-substitution is introduced as a technique for solving more complex integrals, such as the one involving x^3 + 5.

Integration by parts is explained as a method for solving integrals where u and dv are chosen to simplify the calculation, demonstrated with x * e^(x^2).

The process of FOILing is used again to simplify the expression x^3 + 5 before integrating, leading to the final answer of 91/9.

The example of 4x * e^(x^2) demonstrates the necessity of using u-substitution when direct integration methods are not feasible.

Integration by parts is applied to the example of x * e^(x), resulting in the final answer of (x * e^(x)) - e^(x).

Transcripts

Browse More Related Video

Calculus 1 - Integration & Antiderivatives

Integration of Rational Functions By Completing The Square - Calculus

BusCalc 13.2 Integration by Substitution

Indefinite Integral - Basic Integration Rules, Problems, Formulas, Trig Functions, Calculus

Integral of a Product

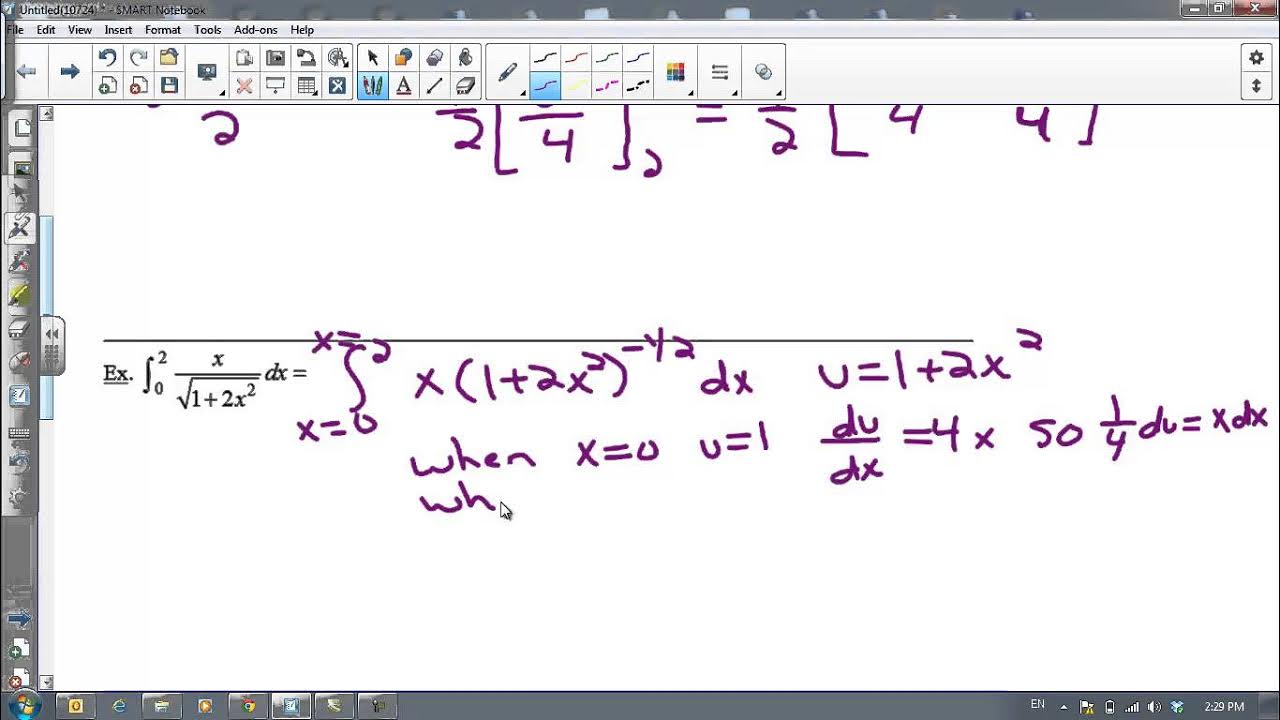
U-Substitution with Definite Integrals

Related Tags

IntegralCalculus Mathematics PolynomialIntegration TrigonometricIntegrals uSubstitution DefiniteIntegrals MathTutorials EducationalContent AdvancedMath CalculusWorkshop