Section 2.7 - Applications of Derivatives to Business and Economics
TLDRThe video script delves into the application of optimization problems in the context of business, focusing on cost, revenue, and profit functions. It introduces the cost function C(x) as the cost of producing x units of a product, revenue as the money brought in from selling x units, and profit as the difference between revenue and cost. The script explains that revenue can also be expressed as x × P, where P can be a fixed price or a function of x, known as the demand equation. Using an office supply company example, the script illustrates how to calculate the price per marker to maximize revenue and the company's maximum profit, highlighting the difference between producing 5,000 markers for revenue maximization versus 4,000 markers for profit maximization. The use of derivatives to find maximums and the importance of understanding the business implications of these calculations are emphasized throughout the discussion.
Takeaways
- 📈 **Cost Function**: C(x) represents the cost of producing x units of a good, which is a crucial factor in business optimization.
- 💰 **Revenue**: Revenue is the money brought in from selling x units of a product, which does not account for costs.
- 📊 **Profit Function**: Profit is calculated by subtracting the cost of production from the revenue, and it can be positive (profit) or negative (loss).
- 🔢 **Revenue as a Function of Price and Quantity**: Revenue can also be described as x times P (where P is the price per unit), which can be fixed or vary based on the quantity sold.
- 📉 **Demand Equation**: P as a function of X (the demand equation) helps determine the price that can be charged to sell a certain quantity of units.
- 🔧 **Maximizing Revenue**: To find the price that maximizes revenue, one must find the quantity where the derivative of the revenue function equals zero and verify it's a maximum.
- 💡 **Maximizing Profit**: Profit maximization may occur at a different quantity than revenue maximization due to the cost function's impact.
- 📌 **Derivative Test**: A derivative test is used to confirm whether a critical point is a maximum or minimum by evaluating the sign of the derivative.
- 📏 **Linear and Parabola Functions**: Understanding the shapes of revenue and cost functions (often linear and parabolic, respectively) helps in determining profit maximization.
- 🧮 **Calculus Application**: Simple derivatives can be used to solve business problems related to optimization of revenue and profit.
- ✅ **Business Decisions with Math**: The script illustrates how mathematical concepts can be applied to make informed business decisions regarding pricing and production quantities.
Q & A
What is the cost function (C of X) in the context of the transcript?
-The cost function (C of X) represents the cost of producing X units of a particular good. It is a mathematical expression that, when given the number of units (X), calculates the total cost incurred in producing those units.
How is revenue defined in the transcript?
-Revenue is defined as the amount of money that a business brings in from selling its goods or services. It is distinct from profit, as it does not account for the costs associated with production.
What is the profit function in the context of the transcript?
-The profit function is the amount of money remaining after subtracting the cost of production from the revenue. It is calculated as Revenue minus Cost (Profit = Revenue - Cost) and can represent either a profit or a loss, depending on whether costs outweigh revenue.
How is revenue also described in the transcript?
-Revenue is also described as the product of the number of units (X) sold and the price (P) at which they are sold, which can be represented as X times P, where P is the price per unit.
What does the demand equation represent?
-The demand equation represents the relationship between the number of units (X) that consumers are willing to buy and the price (P) at which they are willing to buy them. It helps determine the price that needs to be set to sell a certain number of units.
What is the significance of finding the maximum revenue in the context of the example given?
-Finding the maximum revenue is significant because it helps a company determine the optimal number of units to sell to achieve the highest possible income from sales before considering the costs of production.
How does the company determine the price per marker to maximize revenue in the example?
-The company determines the price per marker to maximize revenue by setting the derivative of the revenue function equal to zero and solving for X, which represents the number of markers. The price is then found using the demand equation with the value of X that maximizes revenue.
What is the difference between maximizing revenue and maximizing profit?
-Maximizing revenue focuses on the highest possible income from sales, while maximizing profit takes into account both the revenue and the costs of production. The company may sell more units to increase revenue, but this does not necessarily mean that profit is maximized, as costs also increase with production volume.
How does the cost function impact the company's maximum profit in the example?
-The cost function, which includes a setup cost and a variable cost per marker, is subtracted from the revenue to calculate profit. The company's maximum profit occurs at a different number of markers than the maximum revenue because the cost function changes the point at which profit is highest.
What is the maximum profit for the company in the example, and how many markers should be produced to achieve it?
-The maximum profit for the company is achieved when producing 4,000 markers. This number is determined by setting the derivative of the profit function equal to zero and solving for X, which represents the number of markers to produce.
What price should the company charge per marker to maximize profit, and how is this price determined?
-The company should charge $6 per marker to maximize profit. This price is determined by using the demand equation with the number of markers (4,000) that maximizes profit.
How does the use of derivatives in this context help a business?
-The use of derivatives helps a business by allowing it to calculate the optimal production levels and pricing strategies that will lead to maximum revenue or profit. This mathematical approach provides a scientific basis for business decisions, rather than relying solely on intuition or guesswork.
Outlines
🔍 Introduction to Optimization in Business
The video begins with an introduction to the concept of optimization in the context of business. It discusses the optimization problem related to cost, revenue, and profit. The presenter emphasizes the importance of understanding the cost function (C of X), which represents the cost of producing X units of a good. The video also touches on revenue, which is the money brought in from selling goods, and how it differs from profit, which is revenue minus the cost of production. The presenter also explains that revenue can be expressed as X times P, where P is the price per unit, which can be a fixed value or a function of X, known as the demand equation.
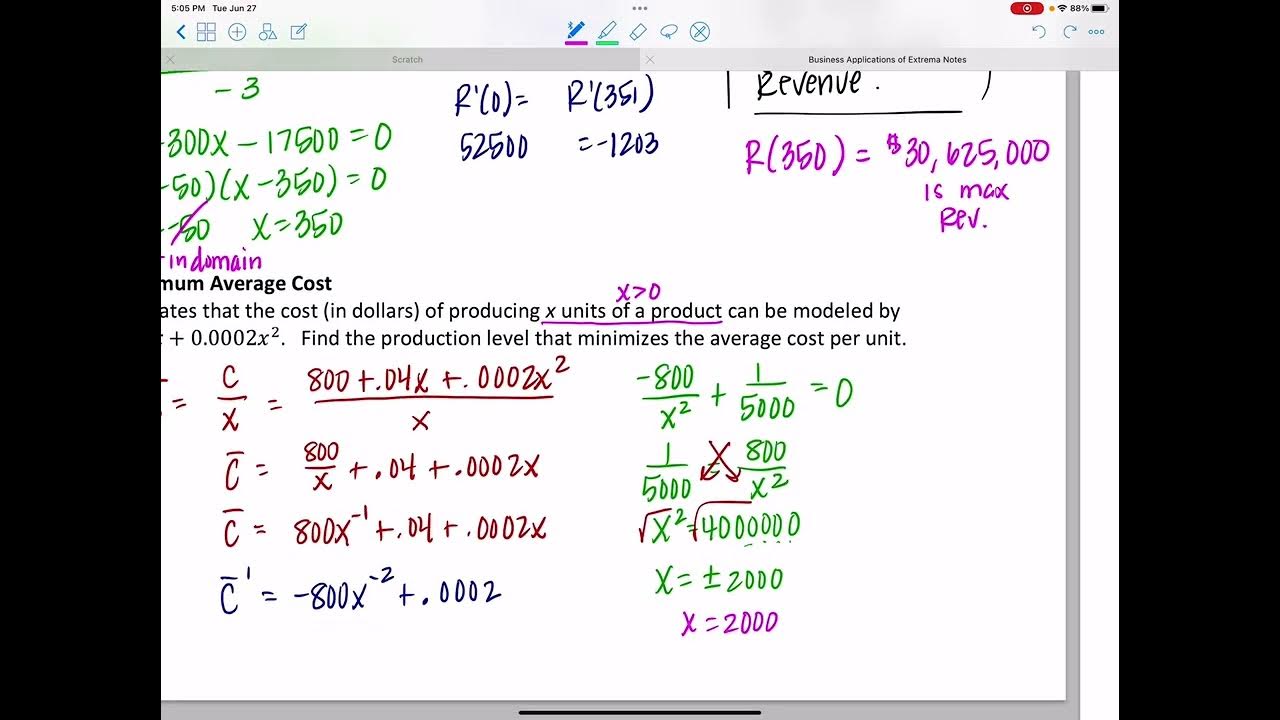
📈 Maximizing Revenue and Identifying the Demand Equation
This paragraph delves into the application of optimization concepts to maximize revenue. An example of an office supply company selling markers is given, with a demand equation P = 10 - 0.01X, illustrating how price per marker decreases as the quantity sold increases. The presenter guides through calculating the maximum revenue by finding the derivative of the revenue function, setting it to zero, and solving for X. It is determined that selling 5,000 markers at $5 each maximizes revenue, resulting in a maximum revenue of $225,000.
📊 Profit Function and Maximizing Profit with Given Costs
The focus shifts to profit maximization with a given cost function C of X = $5,000 setup cost + $2 per marker. The presenter explains that profit is the difference between revenue and cost. By setting up a profit function and taking its derivative, the presenter finds that the company should produce and sell 4,000 markers to achieve maximum profit. This is a different quantity than what maximizes revenue, highlighting the distinction between the two objectives. The presenter also emphasizes the importance of performing a derivative test to confirm that the found solution corresponds to a maximum.
💡 Conclusion and Application of Derivatives in Business
The video concludes with a summary of the key points discussed, including the difference between revenue and profit maximization and how to determine the optimal price and quantity for a product using derivatives. The presenter reiterates the practical application of calculus in business scenarios and encourages viewers to reach out if they have questions. The use of derivatives to find maximum profits and revenues is emphasized as a valuable tool in business decision-making.
Mindmap
Keywords
💡Optimization Problem
💡Cost Function
💡Revenue
💡Profit Function
💡Derivative
💡Demand Equation
💡Maximum Revenue
💡Maximum Profit
💡Setup Cost
💡Variable Cost
💡Price Elasticity of Demand
Highlights
The transcript discusses the optimization problem in the context of business, focusing on cost, revenue, and profit functions.
The cost function C(x) represents the cost of producing x units of a good.
Revenue is the amount of money brought in from selling x units, without considering costs.
Profit is calculated as revenue minus cost. It can be positive (profit) or negative (loss).
Revenue can also be described as x times the price P, where P can be fixed or vary as a function of x.
The demand equation, which shows how price varies with quantity, is crucial for finding total revenue.
An example of an office supply company selling markers is used to illustrate the concepts.
The demand equation for markers is given by P = 10 - 0.01x, showing price decreases as quantity increases.
To maximize revenue, the company should charge a price per marker that results in selling 5,000 markers.
The maximum revenue is $225,000, achieved when selling 5,000 markers at $5 each.
The cost function for the company is C(x) = $5,000 setup cost + $2 per marker.
To maximize profit, the company should produce and sell 4,000 markers, with a price of $6 per marker.
The maximum profit is achieved when the difference between the revenue and cost functions is maximized.
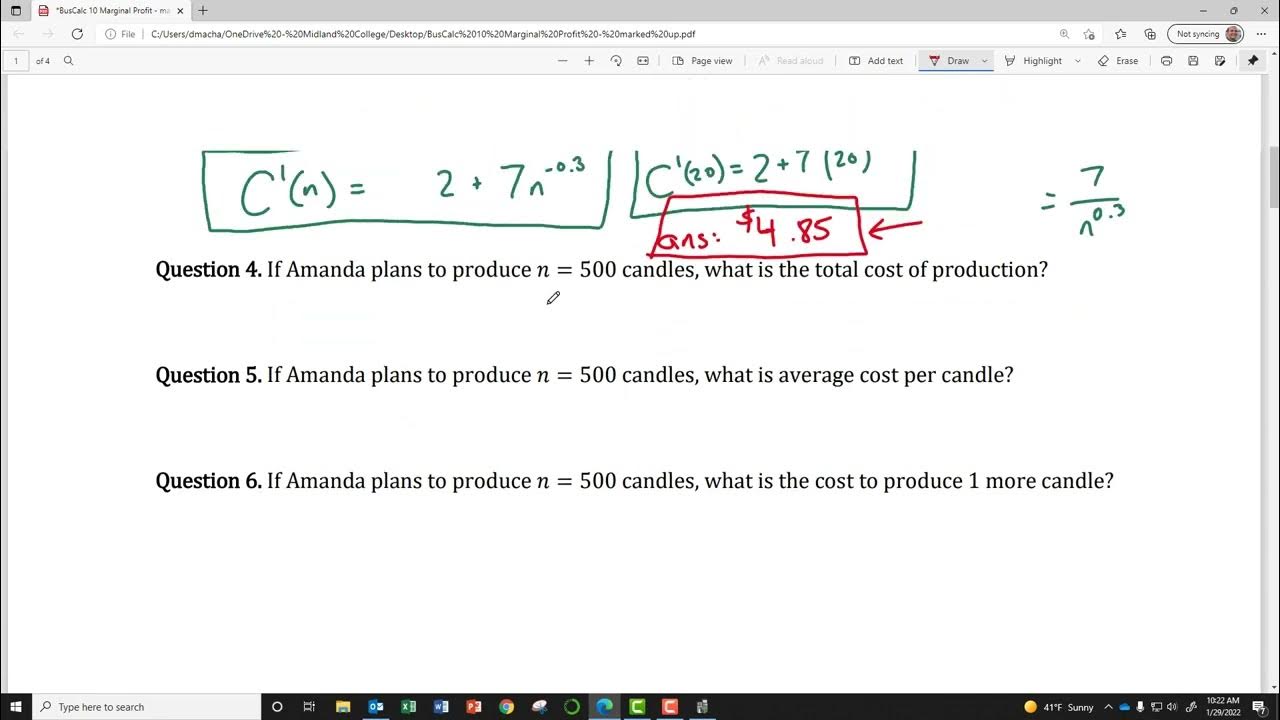
Derivatives are used to find the quantity that maximizes revenue and profit by setting the derivative of the objective function to zero.
The process involves finding the revenue and profit functions, taking derivatives, setting them to zero, solving for quantity, and evaluating the functions at the optimal quantity.
The calculus involved is straightforward - mainly taking derivatives and solving equations, yet it provides valuable insights for business optimization.
The transcript demonstrates the practical application of derivatives in business and economics for optimizing revenue and profit.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: