Business Calculus - Math 1329 - Section 2.5 - Marginal Analysis and Differentials
TLDRThis video script delves into the concept of marginal analysis, a business and economics tool for understanding the rate of change in various economic variables. It explains key terms like marginal revenue, cost, and profit, and how they are derived from their respective functions. The script uses examples to illustrate how to calculate the cost of producing a single unit, such as a skateboard, by finding the difference in total cost between producing 40 and 41 units. It also discusses the use of marginal cost functions for estimation and introduces differentials as an alternative method for estimating changes in function values. The script provides step-by-step solutions to problems involving cost, revenue, and profit functions, and uses differentials to estimate changes in volume, such as the amount of snow needed to increase the radius of a snowball. It concludes with an example of using marginal analysis to estimate the change in total cost when production increases from 3,000 to 3,200 units, demonstrating the practical application of these mathematical concepts in real-world scenarios.
Takeaways
- 📈 **Marginal Analysis**: Marginal analysis involves studying the rate of change, such as marginal revenue (R'(X)), marginal cost (C'(X)), and marginal profit (P'(X)), which are essentially derivatives representing the instantaneous rate of change in total revenue, cost, or profit with respect to the number of items sold.
- 📊 **Marginal Profit Function**: The marginal profit function is derived from the profit function, which is the difference between the revenue and cost functions. It can be found by taking the derivative of the profit function or by subtracting the marginal cost function from the marginal revenue function.
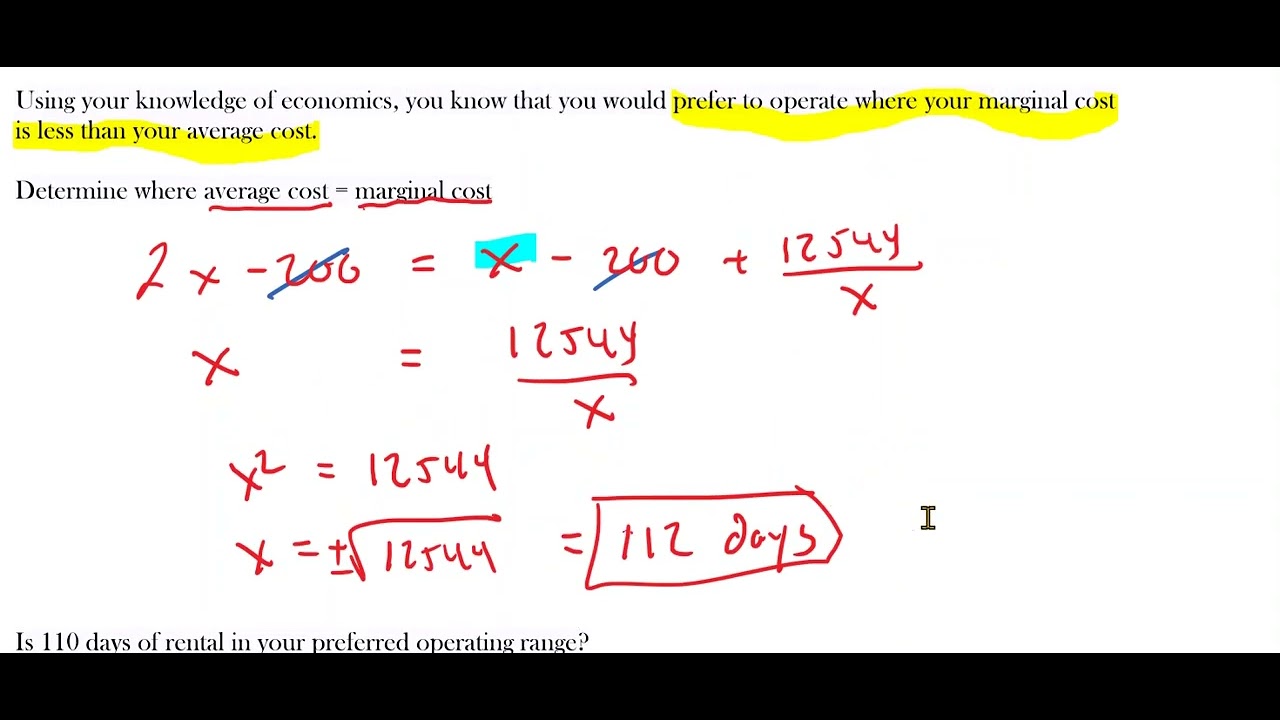
- 🔢 **Exact vs. Approximate Costs**: The cost for each item may not be the same, especially when the cost function is not linear. Marginal cost can be used to estimate the cost of producing an additional item, providing an approximation rather than an exact figure.
- 🛠️ **Application of Marginal Cost**: In business, the marginal cost function can be used to estimate the cost of producing an additional unit, which is helpful for decision-making regarding production levels.
- 📉 **Production Decisions Based on Marginal Profit**: A company should consider whether to increase or decrease production based on the sign of the marginal profit at a given production level. A positive marginal profit indicates potential for increased profit with higher production, while a negative value suggests the need to reduce production.
- 📱 **Revenue, Cost, and Profit Functions in Business**: In the context of selling cellphones, the revenue function is derived from the price demand function, the cost function includes variable and fixed costs, and the profit function is the difference between revenue and cost.
- 🔄 **Differentials for Estimation**: Differentials provide a method to estimate changes in a function's value when the change in the independent variable is small. They are particularly useful when finding the exact change is difficult.
- 📚 **Derivatives and Differentials**: The derivative of a function at a point is the limit of the ratio of the change in the function's value to the change in the variable as the change in the variable approaches zero, which is also the slope of the tangent line at that point.
- 🔢 **Using Differentials for Approximation**: Differentials can be used to approximate the change in the Y values of a function when the change in the X values (ΔX) is small, with dy ≈ ΔY under these conditions.
- ❄️ **Geometric Applications of Differentials**: Differentials can be applied to geometric problems, such as estimating the volume of snow needed to increase the radius of a snowball, by using the derivative of the volume function for a sphere.
- 📏 **Estimating Changes in Total Cost**: For estimating the change in total cost when production is increased from one level to another, differentials can be used to approximate the change in cost, which is the product of the marginal cost function and the change in the number of units produced.
Q & A
What does the term 'marginal' refer to in the context of business and economics?
-In business and economics, 'marginal' refers to the rate of change, often used to describe incremental changes such as marginal revenue, marginal cost, or marginal profit, which are essentially the derivatives of their respective total functions.
How is marginal revenue calculated?
-Marginal revenue is calculated as the derivative of the total revenue function with respect to the number of items sold, represented as R'(X), which gives the instantaneous rate of change in total revenue.
What is the relationship between marginal profit and the marginal revenue and marginal cost functions?
-The marginal profit function is equal to the marginal revenue function minus the marginal cost function, since profit is the difference between revenue and cost. This relationship can be represented as P'(X) = R'(X) - C'(X).
How can you find the cost of producing a specific item, such as the forty-first skateboard, when given the total cost function?
-To find the cost of a specific item, you calculate the total cost for producing one quantity (e.g., 40 items) and the total cost for the next quantity (e.g., 41 items), then find the difference between these two total costs. This difference approximates the cost of the additional item.
Why might the cost for each skateboard be different if the cost function is quadratic?
-A quadratic cost function suggests that there are non-linear factors affecting the cost of production. This could be due to various reasons such as economies of scale, increased operational costs, or the need for additional resources as production increases.
What is the marginal cost function and how is it used to estimate the cost of producing an additional item?
-The marginal cost function is the derivative of the total cost function with respect to the quantity produced. It is used to estimate the cost of producing an additional item by approximating the change in total cost when producing one more unit, often using the limit definition of the derivative and assuming a small change in quantity.
How does the demand function for cellphones influence the revenue function?
-The revenue function is calculated by multiplying the quantity of cellphones sold (X) by the price at which each cellphone is sold (P(X)). If the demand function gives the price as a function of quantity (P = 800 - 1.25X), the revenue function will be the product of X and this price function, resulting in R(X) = 800X - 1.25X^2.
What is the significance of finding the marginal profit function in production decisions?
-The marginal profit function indicates how the profit changes with each additional unit produced. If the marginal profit is positive, it suggests that increasing production will lead to higher profit. Conversely, if it's negative, it indicates that production should be decreased to avoid further reduction in profit.
How are differentials used to estimate changes in a function's value?
-Differentials provide an approximation of the change in a function's value (ΔY) when there is a small change in the independent variable (ΔX). The differential dy is defined as the derivative of the function (f'(x)) times the differential in the independent variable (dx), which gives an estimate of ΔY when ΔX is small.
In the context of the snowball problem, how is the volume differential used to estimate the amount of snow needed to increase the radius?
-The volume differential (dV) is calculated using the derivative of the volume of a sphere function (V = 4/3πR^3) with respect to the radius (R), multiplied by the differential in radius (dR). This gives an estimate of the volume of snow needed to increase the radius from its original size to the new size.
What is the role of marginal analysis and differentials in estimating the change in total cost when production is increased from 3,000 to 3,200 units?
-Marginal analysis and differentials are used to estimate the change in total cost (dC) by taking the derivative of the cost function (C'(x)) with respect to the number of units (x) and multiplying it by the differential in the number of units produced (dx). This provides an approximation of the cost change due to the increase in production.
Outlines
📈 Introduction to Marginal Analysis and Differentials
This paragraph introduces the concept of marginal analysis, which is the rate of change, often used in business to understand how variables like revenue and cost change with respect to production levels. It discusses marginal revenue, marginal cost, and marginal profit, highlighting their relationships and how they are derived. An example is provided where the cost of producing the forty-first skateboard is calculated using the concept of marginal cost.
🛹 Cost Function and Marginal Cost Estimation
The second paragraph delves into the cost function of a skateboard factory, explaining that costs are not uniformly distributed due to the quadratic nature of the cost function. It then moves on to the marginal cost function, showing how to use it to estimate the cost of producing the forty-first skateboard. The concept of the limit definition of the derivative is introduced to approximate the cost using the marginal cost function.
📱 Profit Function and Production Decisions
This section discusses the revenue, cost, and profit functions in the context of cellphone production. It explains how to calculate these functions and use the marginal profit function to make production decisions. The primary goal of the company is to maximize profit, and the paragraph illustrates how to determine whether to increase or decrease production based on the marginal profit at a given production level.
🔄 Differentials and Their Application
The fourth paragraph introduces differentials as another method to estimate changes in function values. It explains the concept using the secant line and how it transforms into the tangent line as the distance between two points approaches zero. The formula for differentials is derived, and it is shown how differentials can be used to approximate changes in function values when exact calculations are difficult.
🔢 Differentials for Function Estimation
The fifth paragraph provides an example of using differentials to estimate the change in the value of a function when the change in the input is small. It demonstrates the process with a function f(x) and shows how to use the differential to estimate the change in the function's value from x=2 to x=2.1, highlighting the practicality of differentials in approximations.
❄️ Estimating Volume Changes with Differentials
This paragraph presents a geometric problem involving the estimation of the amount of snow needed to increase the radius of a snowball. It uses the volume function of a sphere and the concept of differentials to estimate the volume of snow required for the radius increase. The calculation is performed step by step, showing how to apply differentials to a real-world problem.
📊 Marginal Analysis for Cost Estimation
The final paragraph discusses the use of marginal analysis or differentials to estimate the change in total cost when production increases from 3,000 to 3,200 units. It outlines the process of using the derivative of the cost function and the concept of differentials to approximate the change in cost, demonstrating the close relationship between marginal analysis and differentials.
Mindmap
Keywords
💡Marginal Analysis
💡Derivatives
💡Marginal Cost
💡Profit Function
💡Differentials
💡Secant Line
💡Tangent Line
💡Limit Definition
💡Quotient Rule
💡Volume of a Sphere
💡Production Level
Highlights
Marginal analysis is the study of the rate of change, commonly used in business to evaluate marginal revenue, cost, and profit.
Marginal revenue (R'(X)) is the derivative of total revenue with respect to the number of items sold, indicating how revenue changes at a certain production level.
Marginal cost (C'(X)) is the derivative of total cost, showing the instantaneous rate of change in cost with respect to the number of items produced.
Marginal profit is calculated as the difference between the marginal revenue function and the marginal cost function.
The marginal average function can be found by taking the derivative of the average function.
In a factory example, the cost of producing the forty-first skateboard is calculated by finding the total cost for forty and forty-one skateboards and taking the difference.
The cost function for producing skateboards is quadratic, indicating non-linear factors are involved in the cost for each skateboard.
Marginal cost function is used to estimate the cost of producing an additional item, such as the forty-first skateboard, by approximating the derivative.
The limit definition of the derivative is utilized to approximate the actual cost of producing an additional item when the change in quantity is small.
In a cellphone production scenario, the revenue, cost, and profit functions are derived from the price demand function and manufacturing costs.
The marginal profit function is used to determine whether production should be increased or decreased based on the sign of the marginal profit at a given production level.
Differentials are introduced as another method to estimate the change in a function's value, using the concept of the secant line and its transformation into the tangent line.
The formula for differentials, dy = f'(x) * dx, is derived from the limit of the secant line slopes as the distance between points approaches zero.
Differentials are used to approximate the change in Y values (ΔY) when ΔX is small, providing a close estimate of the actual change in the function's value.
An example demonstrates using differentials to estimate the change in the function f(X) = X^2 - 5X + 7 / (X + 8) when X increases from 2 to 2.1.
In a geometry problem, differentials are applied to estimate the amount of snow needed to increase the radius of a snowball from 7 cm to 7.5 cm by using the volume function of a sphere.
The total cost function for producing X thousand units is given by C(X) = 0.2X^3 - 0.6X^2 + 8X + 200, and differentials are used to estimate the change in total cost when production increases from 3,000 to 3,200 units.
The approximation using differentials is shown to be very close to the actual cost difference, highlighting the effectiveness of the method for estimating changes in cost functions.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: