AP Calculus AB Crash Course Day 7 - Differentiation, Related Rates, and the Mean Value Theorem

Dr. Steve Warner

26 Feb 202216:44

EducationalLearning

32 Likes 10 Comments

TLDRThe transcript outlines a comprehensive mathematical exploration covering various calculus topics. It begins with the differentiation of a complex function involving e to the cotangent of 3x over the square root of x, using the quotient rule and chain rule to simplify the expression into a simple fraction. The discussion then shifts to finding the minimum value of another function, applying power rules and analyzing the second derivative to determine critical points. The concept of total distance traveled by a point moving in a straight line, with changing direction, is addressed through the calculation of displacement and distance using derivatives and absolute values. A related rates problem involving the rate of decrease of a spherical balloon's surface area is solved using volume and surface area formulas, with the application of the given rate of radius decrease. The transcript also tackles differential equations, including finding a particular solution and approximating function values using tangent lines. Finally, it explores the existence of a value for a composite function that satisfies a given derivative condition, leveraging the mean value theorem and the properties of differentiable functions.

Takeaways

📚 **Quotient Rule Application**: The script demonstrates the step-by-step application of the quotient rule for differentiating complex functions, emphasizing the importance of breaking down the process for clarity.
🧮 **Chain Rule Utilization**: It highlights the use of the chain rule in differentiating expressions involving exponential functions with trigonometric components.
🔄 **Derivative of Cotangent**: The derivative of cotangent is shown to be the negative of the square of the cosecant, which is a crucial step in the differentiation process.
✅ **Power Rule Simplification**: The script simplifies the power rule by rewriting square roots as fractional exponents, which aids in differentiating the denominator.
🔢 **Second Derivative Test**: The process of finding critical points using the second derivative test is explained, which helps in determining local minima and maxima of functions.
📈 **Sign Chart for Interval Analysis**: A sign chart is used to analyze the behavior of a function's derivative across different intervals, which is a common technique in calculus.
🚀 **Distance Traveled vs. Displacement**: The difference between total distance traveled and displacement is clarified through an example involving a moving point, emphasizing the need to consider direction changes.
🎈 **Related Rates for Spherical Balloons**: A related rates problem is solved to find the rate of change of the surface area of a spherical balloon, given a constant rate of change of its radius.
🔁 **Volume and Surface Area of a Sphere**: The formulas for the volume and surface area of a sphere are used to relate the rate of change of these properties to the radius of the sphere.
📉 **Rate of Decrease in Surface Area**: The script calculates the rate at which the surface area of a balloon decreases, demonstrating the application of derivatives in real-world problems.
🤔 **Existence of a Value for Mean Value Theorem**: The mean value theorem is used to justify the existence of a value 'c' for which the derivative of a composed function equals a given value, under certain conditions.

Q & A

What is the derivative of the function f(x) = e^(cotan(3x))/√x using the quotient rule?
-The derivative of f(x) = e^(cotan(3x))/√x is found by applying the quotient rule, which results in a complex fraction. After simplifying, the derivative is given by multiplying the numerator and denominator by 2√x to eliminate the complex fraction, leading to a simplified form of the derivative.
How do you find the minimum value of the function g(x) = x^7/(7 - x^6/5)?
-To find the minimum value, first take the derivative of g(x) and then the second derivative. Set the second derivative equal to zero to find critical points. Analyze the sign of the second derivative in intervals defined by the critical points to determine where the function is increasing or decreasing, which helps identify the minimum value.
What is the total distance traveled by a point moving in a straight line with a distance function s(t) from t=0 to t=4?
-The total distance traveled is found by computing the position function s(t) at the endpoints and any critical points within the interval. Then, sum the absolute value of the differences between these computed values to account for changes in direction.
How do you find the rate of decrease of the surface area of a spherical balloon when the volume is 288π cubic centimeters?
-Use the given rate of decrease of the radius and the formulas for the volume and surface area of a sphere. Apply related rates by differentiating the surface area formula with respect to time and substituting the given values to find the rate of decrease.
What is the equation of the tangent line to the graph of f at the point (1,1) for the differential equation dy/dx = e^(y - 1) * (2x^3 - 5)?
-The slope of the tangent line at a point is given by the derivative of the function at that point. For the given differential equation, the slope at (1,1) is found by substituting x and y with 1. The equation of the tangent line is then written in point-slope form using this slope and the point (1,1).
How do you approximate f(1.1) using the tangent line to the graph of f at (1,1)?
-Plug the x-value of 1.1 into the equation of the tangent line to find the approximate y-value, which represents f(1.1). This is a linear approximation method assuming that the tangent line closely represents the function near the point (1,1).
Why are there no points on the x-axis in the slope field for the differential equation dy/dx = -x^2/y?
-The slope field is undefined on the x-axis because the denominator y would be zero, resulting in a division by zero in the differential equation. Hence, there are no points on the x-axis in the slope field.
What is the slope of the tangent line at the point (1,1) in the slope field for the differential equation dy/dx = -x^2/y?
-At the point (1,1), the slope is found by substituting x = 1 and y = 1 into the differential equation, which yields a slope of -1 since 1^2/1 equals 1.
Given the functions h(x) and k(x), how can you determine if there exists a value c such that f'(c) = 2, where f(x) = h(k(x))?
-Since h and k are twice differentiable, their composition f(x) = h(k(x)) is differentiable. By the Mean Value Theorem, if f is continuous on a closed interval and differentiable on an open interval, there exists a value c in that interval such that f'(c) equals the average rate of change of f over that interval. Compute f(2), f(-3), and use these values to find the average rate of change, which should equal f'(c) if such a c exists.
What is the value of c that satisfies f'(c) = 2 for the function f(x) = h(k(x)) given the values of h and k at specific points?
-Using the Mean Value Theorem, the value of c that satisfies f'(c) = 2 is found by calculating the average rate of change of f from x = -3 to x = 2. This is done by finding f(2) and f(-3) using the given values of h and k, and then dividing the difference in their values by the difference in x-values (5).
How do you determine the values of h(k(2)) and h(k(-3)) using the given information about h and k?
-The value of h(k(2)) is found by substituting the given value of k(2) which is 8 into the function h, so h(8) = 6. Similarly, h(k(-3)) is found by substituting k(-3) which is 1 into h, so h(1) = -4.

Outlines

00:00

🧮 Derivative of a Complex Function

This paragraph discusses the differentiation of a complex function using the quotient rule. The function in question is f(x) = e^(cotangent(3x))/√x. The process involves applying a triple chain rule to find the derivative of the numerator and a power rule for the denominator. The explanation also includes rewriting √x as x^(1/2) to simplify the derivative. The final step involves multiplying the fraction by 2√x to eliminate the complex fraction and simplify the derivative further.

05:00

📉 Minimization of a Function's Derivative

The second paragraph deals with finding the minimum value of a function g(x) = x^7 / (7 - x^6 / 5). The process involves taking the derivative of g(x) using power rules and then finding the second derivative. By setting the second derivative to zero, critical numbers are found, and a sign chart is used to determine the intervals where the function is increasing or decreasing. The minimum value is then identified at x = 1, as indicated by the sign of the second derivative.

10:02

🚀 Calculating Total Distance Traveled

This paragraph addresses a problem involving a point moving in a straight line with a given distance function. The task is to calculate the total distance traveled from t = 0 to t = 4. The velocity function is derived from the given distance function, and critical points where the velocity is zero are identified. The problem is solved by breaking the time interval into smaller parts and calculating the position function at the endpoints of these intervals. The total distance is found by summing the absolute differences between these positions.

15:04

🎈 Rate of Change of a Spherical Balloon's Surface Area

The fourth paragraph presents a related rates problem concerning the surface area of a spherical balloon whose radius is decreasing at a constant rate. The volume and surface area formulas for a sphere are provided, and the problem asks for the rate of decrease of the surface area when the volume is 288π cubic centimeters. By using the given rate of change of the radius and the volume formula, the radius at the given volume is determined. The derivative of the surface area with respect to time is then calculated, and the rate of decrease of the surface area is found to be -24π square centimeters per second when the radius is 6 centimeters.

🔍 Approximating a Function Using a Tangent Line

The fifth paragraph focuses on using a differential equation to find a particular solution that passes through a specific point and then using the tangent line to approximate a value of the function. The differential equation is given, and the derivative at a point (1,1) is calculated. Using the point-slope form, an equation for the tangent line is derived. This tangent line is then used to approximate the value of the function at x = 1.1, resulting in an approximate value of 0.7 for f(1.1).

📈 Sketching a Slope Field for a Differential Equation

The sixth paragraph involves sketching a slope field for a given differential equation at 12 specified points. The differential equation is provided, and it is noted that there are no points on the x-axis due to the undefined derivative when y = 0. The slopes at various points are calculated by plugging in the x and y values into the differential equation. The resulting slope field illustrates the direction and steepness of the tangent lines at each point, with horizontal tangents where x = 0.

🔢 Existence of a Value for a Composite Function's Derivative

The final paragraph presents a problem involving the existence of a value c such that the derivative of a composite function f(x) = h(k(x)) equals 2. Given values for the functions h and k at specific points, the mean value theorem is applied to find a value c in the interval (-3, 2) where f'(c) meets the condition. The function values at the endpoints of the interval and the derivative of f at c are calculated, leading to the determination of such a value c.

Mindmap

Keywords

💡Differentiation

Differentiation in the context of the video refers to the mathematical process of finding the derivative of a function. It is a fundamental concept in calculus and is used to determine the rate at which a function changes. In the video, the process of differentiating complex functions is demonstrated, such as the function f(x) = e^(cotangent(3x))/√x, which is a central theme.

💡Quotient Rule

The Quotient Rule is a mathematical rule used to differentiate quotients of two functions. It states that the derivative of a quotient is the denominator times the derivative of the numerator minus the numerator times the derivative of the denominator, all divided by the square of the denominator. The video uses this rule to differentiate a complex function, illustrating its application in calculus.

💡Chain Rule

The Chain Rule is a method in calculus for finding the derivatives of compositions of functions. It's used when you need to differentiate a function that is composed of two or more functions. In the video, the Chain Rule is applied to differentiate e^(cotangent(3x)), showing its importance in handling composite functions.

💡Cosecant

Cosecant is a trigonometric function, which is the reciprocal of the sine function. It is represented as csc(x) = 1/sin(x). In the video, the derivative of cotangent(3x) is found to involve cosecant squared, demonstrating the interplay between calculus and trigonometry.

💡Power Rule

The Power Rule is a basic rule in calculus that allows for the differentiation of power functions. It states that the derivative of x^n, where n is a constant, is n*x^(n-1). The video uses the Power Rule to find derivatives of functions like x^(1/2) and x^6, highlighting its utility in various differentiation scenarios.

💡Second Derivative

The second derivative refers to the derivative of the first derivative of a function. It provides information about the concavity and points of inflection of a function. In the video, the second derivative is used to find critical points and to analyze the behavior of a function, such as whether it is increasing or decreasing.

💡Mean Value Theorem

The Mean Value Theorem is a fundamental theorem in calculus that formalizes the idea that if a function is continuous on a closed interval and differentiable on an open interval within that closed interval, then there exists a point at which the derivative of the function equals the average rate of change of the function over that interval. The video uses this theorem to justify the existence of a value c for which f'(c) is a specific value.

💡Related Rates

Related Rates is a topic in calculus where one studies how different quantities are related by rates of change. It often involves solving applied problems where one quantity is given and another is sought. In the video, a related rates problem is solved for the surface area of a spherical balloon, demonstrating how to find an unknown rate of change using given information.

💡Differential Equation

A differential equation is an equation that involves a function and its derivatives. It is used to model various phenomena in physics, engineering, and other disciplines. The video presents a differential equation and discusses finding a particular solution that satisfies certain conditions, which is a common task in differential calculus.

💡Tangent Line

A tangent line to a curve at a given point is a line that 'just touches' the curve at that point. The slope of the tangent line at a point on a curve is equal to the derivative of the function at that point. In the video, the tangent line is used to approximate the value of a function near a specific point, illustrating the geometric interpretation of derivatives.

💡Slope Field

A slope field is a graphical representation of the slopes of the tangent lines to the solutions of a differential equation at various points on the plane. It is used to visualize the behavior of solutions without explicitly solving the differential equation. The video demonstrates how to sketch a slope field for a given differential equation, providing insight into the solution's behavior.

Highlights

Differentiating a complex function using the quotient rule step by step

Applying the chain rule to find the derivative of e to the cotangent of 3x

Rewriting the derivative as a simple fraction by multiplying top and bottom by 2 root x

Finding the minimum value of a function using the first and second derivatives

Factoring the second derivative to find critical numbers and determine behavior

Using a sign chart to test intervals and find where the function has a minimum

Calculating total distance traveled by breaking the motion into intervals

Considering direction changes when computing distance vs. displacement

Solving a related rates problem for the surface area of a spherical balloon

Using the volume and surface area formulas for a sphere to find the radius

Applying the chain rule to find the rate of decrease of surface area

Using the mean value theorem to find a value where the derivative equals a given number

Computing the value of a composite function using given values of the component functions

Sketching a slope field for a differential equation at specified points

Identifying horizontal tangent lines where the derivative is zero

Plugging in values to find accurate slopes at different points in the slope field

Writing an equation for the tangent line to a function at a given point

Approximating a function value using the tangent line equation

Transcripts

Browse More Related Video

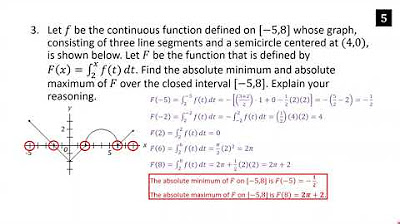
AP Calculus AB Crash Course Day 10 - Integration, IVT, and MVT

AP Calculus AB Crash Course Day 8 - Integration and Riemann Sums

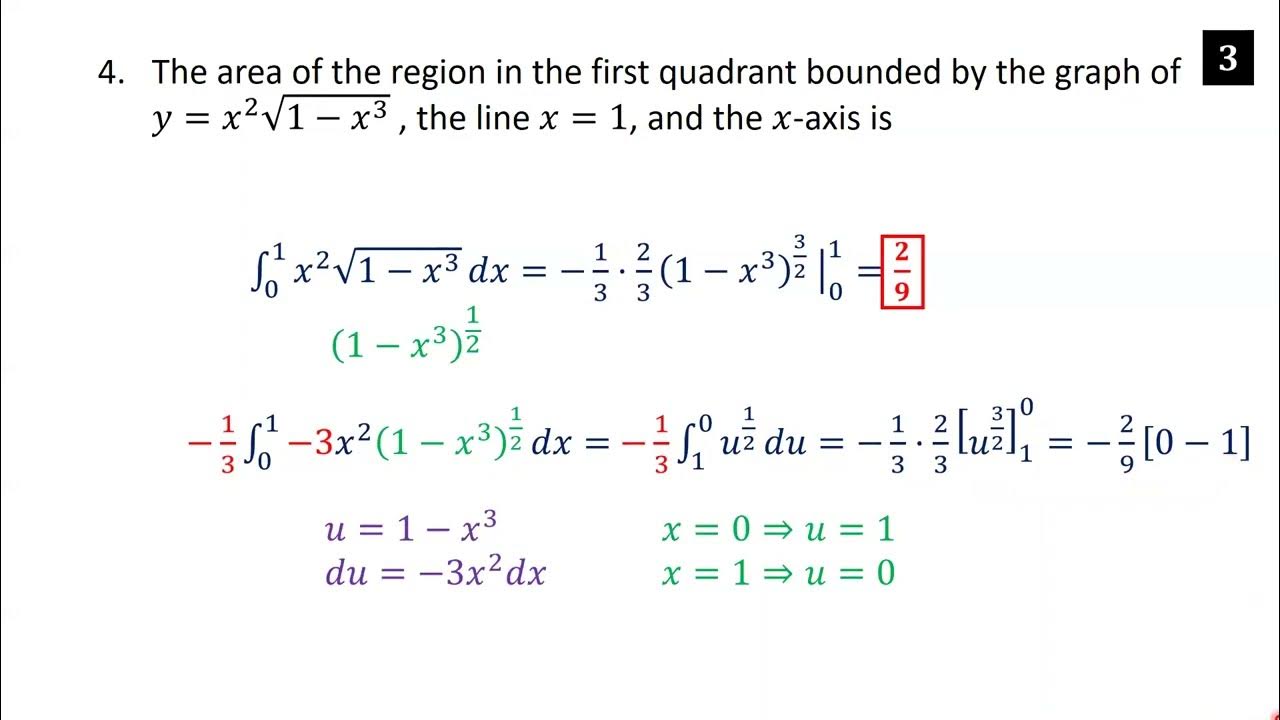
AP Calculus AB Crash Course Day 2 - Integration

2013 AP Calculus AB Free Response #4

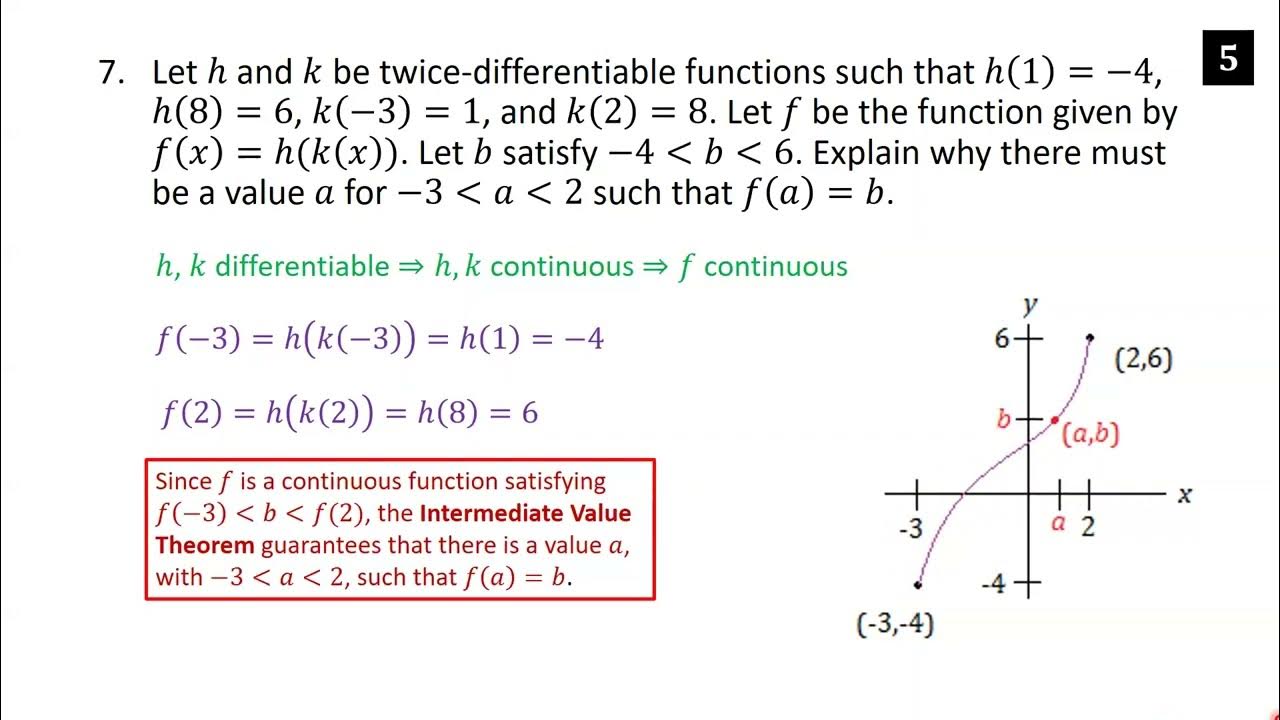
AP Calculus AB Crash Course Day 6 - Limits, Continuity, and the Intermediate Value Theorem

2023 AP Calculus AB & BC FRQ #1