AP Calculus AB Crash Course Day 8 - Integration and Riemann Sums
TLDRThe transcript details a series of mathematical problems and solutions, focusing on integral calculus. It begins with an integral involving the cotangent and cosecant functions, applying substitution methods to simplify and solve the problem. The discussion then shifts to finding the area under a curve defined by y=e^(3x) between x=1 and x=4, using a substitution of u=3x to calculate the integral. Further, the transcript explores the application of substitution in evaluating definite integrals, as demonstrated by transforming the integral of f(3x+k)dx from 2 to 5 into an equivalent form involving the variable u. A left Riemann sum approximation for a function f on the interval [1, 11] is also calculated, using given function values at selected points. The mean value theorem is invoked to find a point where the second derivative of a composite function f(h(k(x))) is zero, assuming certain conditions about the derivatives of h and k. The transcript concludes with finding points of inflection for a function f composed of h and k, which are proven to be linear functions, thus having no points of inflection. Lastly, it involves calculating definite integrals for a piecewise function defined on the interval [-5, 8], which includes line segments and a semicircle, to find the values of the function F at x=8 and x=-1.
Takeaways
- 📖 The integral of a function involving 5 to the power of cotangent x times cosecant squared x can be solved by substitution, leading to the result -5 to the cotangent x over ln(5) plus a constant C.
- 📘 The area under a curve from x=1 to x=4 for the function y = e to the 3x is computed by making a simple substitution in the integral, resulting in the evaluation of one-third times e to the power of three times x, evaluated from 1 to 4.
- 🔨 A substitution method is used to solve the integral of a function from x=2 to x=5, which is scaled and shifted by constants, illustrating how to manipulate and adjust limits and coefficients in integrals.
- 📚 Using the left Riemann sum approximation method, the integral of a function over a specified interval can be estimated by multiplying the function values at specified points by the lengths of subintervals.
- 📷 Sketching the function and the regions under consideration can significantly aid in visualizing and solving calculus problems involving areas under curves.
- 🔧 When functions are compositions of other functions, the derivatives are found using the chain rule, and the mean value theorem can be applied to find specific properties like points where the second derivative equals zero.
- 🎲 For functions that are twice differentiable and their second derivatives are zero everywhere, the functions are linear, and thus the composed function has no points of inflection.
- 🎓 Utilizing geometric shapes like semicircles and triangles to compute definite integrals can simplify the process by applying formulas for areas of basic shapes.
- 📝 The chain rule and product rule are pivotal in finding derivatives of composite functions, particularly when differentiating nested functions involving other basic functions.
- 📱 Converting complicated integrals into simpler forms through substitutions can often clarify the procedure and make the solutions more straightforward.
Q & A
What is the integral of 5 to the cotangent x, times cosecant squared x dx?
-The integral is found by substitution, where u is cotangent x, and du is -cosecant squared x dx. The integral simplifies to -5 to the u over ln(5) plus a constant, and after substituting back, it becomes -5 cotangent x over ln(5) plus a constant.
How do you find the area under the curve y equals e to the 3x between x equals 1 and x equals 4?
-The area is found by computing the integral from 1 to 4 of e to the 3x dx. By substitution, let u = 3x, then du = 3dx, and the integral becomes (1/3)e to the 3x evaluated from 3 to 12.
If the integral from two to five f of three x plus k dx is equal to b, what is the integral from six plus k to fifteen plus k f of x dx in terms of b?
-Using substitution, let u = 3x + k, then du = 3dx. The integral from 6 + k to 15 + k f of x dx is equal to (1/3) of the integral from 2 to 5 f of (3x + k) dx, which simplifies to (1/3)b.
How do you approximate the integral from 1 to 11 f of x dx using a left Riemann sum with the given data points?
-You sum the products of the function values at the left endpoints of the sub-intervals and the lengths of the sub-intervals. The sum is 3(4) + 5(1) + 1(2) - 3(3), which equals 10.
If h and k are twice differentiable functions with h''(x) = k''(x) = 0 for all x, what can be said about the points of inflection on the graph of f(x) = h(k(x))?
-Since h and k are linear functions (as their second derivatives are zero), their composition f(x) is also linear. Linear functions do not have points of inflection, so there are no points of inflection on the graph of f.
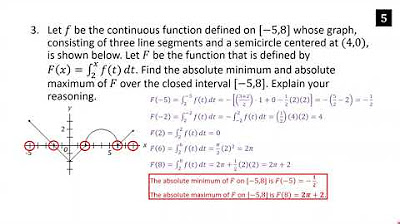
What is the value of F(x) for a function defined as the integral from 2 to x of f(t) dt, given that x is within the closed interval negative 5 to 8?
-The value of F(x) is the sum of the areas under the line segments and semicircle of the graph of f from 2 to x. For example, F(8) would involve the area under the semicircle from 2 to 6 and the triangle from 6 to 8, which evaluates to 2π + 2.
How do you find the value of F(-1) for the function F defined in the previous question?
-Since -1 is less than 2, you need to flip the integral and change the order of integration. The value of F(-1) involves the negative of the integral from -1 to 2 of f(t) dt, which is the negative of the sum of the areas of a trapezoid and a triangle under the graph of f from -1 to 2, resulting in 7.5.
What is the significance of the mean value theorem in the context of the function f(x) = h(k(x)) where h and k are twice differentiable?
-The mean value theorem guarantees the existence of at least one point d in the interval (a, b) where the second derivative of f, f''(d), is equal to the average rate of change of the first derivative of f over the interval, i.e., f'(2) - f'(-3) / (2 - (-3)).
Why does the function f(x) = h(k(x)) have no points of inflection if h''(x) and k''(x) are both zero for all x?
-If the second derivatives of h and k are zero, it implies that h and k are linear functions. The composition of two linear functions is also linear, and linear functions do not exhibit changes in concavity, hence f(x) has no points of inflection.
How is the integral from a to b of a function f(x) related to the area under the curve of f(x) between x = a and x = b?
-The integral from a to b of a function f(x) represents the signed area under the curve of f(x) between x = a and x = b. Positive values of the function contribute positively to the area, while negative values contribute negatively.
What is the geometric interpretation of the integral in the context of the area under a curve?
-The integral of a non-negative function over an interval can be interpreted as the area enclosed by the curve, the x-axis, and the lines x = a and x = b. For functions that change sign within the interval, the integral represents the difference between the area above the x-axis and the area below it.
Outlines
🧮 Integration by Substitution
The paragraph discusses the integration of the function '5 to the cotangent x times cosecant squared x'. It explains the substitution method where 'u' is set as the cotangent of x, leading to the integral of '5 to the u over the natural logarithm of 5'. The process involves a substitution with a negative sign correction, followed by the integration and addition of an arbitrary constant 'c'. The paragraph also covers finding the area under the curve y=e^(3x) between x=1 and x=4, using a substitution of u=3x and then evaluating the integral from the modified limits.
📐 Area Calculation and Function Properties
This section deals with calculating the area under the curve y=e^(3x) from x=1 to x=4, using integration and substitution methods. It also explores the properties of an integral involving the function f(3x+k) and how it relates to another integral with limits shifted by k. The paragraph concludes with a discussion on a function f(x) that is the composition of two functions h and k, and the conditions under which f would have a point of inflection.
📈 Function Composition and Points of Inflection
The paragraph presents a problem involving twice differentiable functions h and k, where the function f is defined as the composition of h and k. Given certain conditions about the derivatives of h and k, the paragraph explains why there must be a value 'd' such that the second derivative of f at 'd' is zero. It also discusses another scenario where both h and k are linear functions due to their second derivatives being zero, leading to f being a linear function with no points of inflection.
🏞️ Geometric Interpretation of Definite Integrals
The final paragraph focuses on the geometric interpretation of definite integrals. It involves calculating the value of a function F, which is defined as the integral of another function f from 2 to x. Two specific cases are considered: F(8) and F(-1). For F(8), the integral is split into the area under a semicircle and a triangle, while for F(-1), the limits are flipped and the integral is split into the area of a trapezoid and a triangle. The geometric shapes are used to find the exact values of these integrals, which are then simplified to provide the final answers.
Mindmap
Keywords
💡Integral
💡Cotangent
💡Cosecant
💡Substitution
💡Derivative
💡Mean Value Theorem
💡Riemann Sum
💡Linear Function
💡Points of Inflection
💡Chain Rule
💡Antiderivatives
Highlights
Deriving the integral of 5 * cot(x) * csc(x)^2 using substitution method by letting u = cot(x)
Solving the integral of e^(3x) from x=1 to x=4 using substitution u = 3x
Using substitution to find the integral from x=2 to x=5 of f(3x+k) dx in terms of b
Approximating the integral from x=1 to x=11 of f(x) dx using a left Riemann sum
Applying the mean value theorem to show f''(d) = 0 for some d between -3 and 2
Proving that f(x) = h(k(x)) is a linear function if h''(x) = k''(x) = 0 for all x
Finding the points of inflection on the graph of f(x) when h(x) and k(x) are linear
Calculating F(8) and F(-1) where F(x) = integral from 2 to x of f(t) dt using geometry
Splitting the integral into two parts to find F(8) using the area of a semicircle and a triangle
Flipping the limits of integration and using the area of a trapezoid and triangle to find F(-1)
Deriving the integral of a function using substitution and the chain rule
Solving the integral using substitution and simplifying the limits of integration
Using a formal substitution to transform the integral and simplify the limits
Approximating the integral using a left Riemann sum and summing the areas of rectangles
Applying the mean value theorem to the derivative of f to find information about the second derivative
Using the chain rule to find the derivative of the composition of two functions
Showing that the composition of two linear functions is also linear
Finding the points of inflection on the graph of a function using the second derivative
Calculating the definite integral using geometry to find the areas of shapes under the curve
Flipping the limits of integration and using the area of a trapezoid and triangle to find the integral
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: