AP Calculus AB Crash Course Day 10 - Integration, IVT, and MVT
TLDRThe transcript explores various mathematical concepts related to calculus, focusing on functions and their derivatives. It introduces a continuous function 'little f' defined on a closed interval with a graph consisting of line segments and a semicircle. The function 'capital f' is defined as the integral of 'little f' from 2 to x. The script delves into finding points of horizontal tangent lines for 'capital f', determining relative extrema, and points of inflection. It also discusses finding the absolute minimum and maximum of 'capital f' over a closed interval and calculates derivatives using the chain rule. The exploration extends to a function 'g' involving the natural logarithm and another function 'h' involving an exponential term. The script concludes with an application of the intermediate value theorem and mean value theorem to establish the existence of certain real numbers that satisfy given conditions for a twice differentiable function 'f'. The summary encapsulates the essence of the mathematical analysis presented in the script.
Takeaways
- 📌 The function f is continuous on the closed interval [-5, 8] and consists of three line segments and a semicircle centered at (4, 0).
- 📚 The function F, defined by the integral from 2 to x of f(t) dt, has its derivative F'(x) equal to f(x) based on the Fundamental Theorem of Calculus.
- 🔍 F has a relative maximum at x = -2 and a relative minimum at x = 2, with neither a minimum nor a maximum at x = 6, determined by the sign changes of f(x).
- 🔑 There are points of inflection at x = 0, x = 4, and x = 6 for the function F, where the concavity of the graph changes.
- 🏁 The absolute minimum of F on the interval [-5, 8] is F(-5) = -1/2, and the absolute maximum is F(8) which is slightly more than 8 due to the addition of 2π to the integral result.
- ⛓ The function g, defined by g(x) = ln(F(x)/2x), has its derivative g'(x) computed using the chain rule and quotient rule, resulting in g'(-4) = 1.25.
- 🧮 The function h, defined by h(x) = f(3x^2 - 2x)/e^(x-1), has a tangent line at x = 1 with a slope of 3, derived from the chain rule and the given function values.
- 🔢 For the twice differentiable function f with given values at x = 1 and x = 3, there exist real numbers a and c between 1 and 3 such that f(a) * f'(c) = b * d, where b is between f(1) and f(3), and d is between f'(1) and f'(3), by the Intermediate Value Theorem.
- 📉 There must be a value c between 1 and 3 such that f''(c) = -2, as guaranteed by the Mean Value Theorem for the continuous and differentiable function f on the interval [1, 3].
- 🤔 The existence of a tangent line's equation, points of inflection, and extreme values are all grounded in the properties of derivatives and the behavior of the function f across the given interval.
- 📐 The problem-solving approach involves the application of calculus theorems such as the Fundamental Theorem of Calculus, the Mean Value Theorem, and the Intermediate Value Theorem to analyze the function f and its derived functions F, g, and h.
Q & A
What is the function capital f defined as in the script?
-The function capital f is defined as the integral from 2 to x of little f of t dt, where little f is a continuous function on the closed interval negative 5 to 8.
How does one determine the points where the graph of capital f has a horizontal tangent line?
-The points where the graph of capital f has a horizontal tangent line are determined by finding where the derivative of capital f, which is equal to little f of x, is equal to zero. These points are x equals negative two, two, and six.
What does it mean for capital f to have a relative maximum or minimum at a certain point?
-Capital f has a relative maximum or minimum at a point if the derivative of capital f changes sign around that point. A relative maximum occurs when the derivative changes from positive to negative, and a relative minimum occurs when it changes from negative to positive.
How can one find the points of inflection on the graph of capital f?
-The points of inflection on the graph of capital f can be found by determining where the second derivative of capital f, which is equal to the derivative of little f, changes sign.
What is the absolute minimum and maximum of capital f over the closed interval negative five to eight?
-The absolute minimum of capital f over the closed interval negative five to eight is negative a half, which occurs at x equals negative five. The absolute maximum is a little more than eight, which is the value at x equals eight.
How is the function g related to capital f and what is its derivative at x equals negative four?
-The function g is defined as the natural logarithm of (capital f of x over 2x). The derivative of g at x equals negative four is found using the chain rule and is equal to 1.25.
What is the equation of the tangent line to the graph of the function h at the point where x equals one?
-The equation of the tangent line to the graph of h at the point where x equals one is y + 1 = 3(x - 1), which simplifies to y = 3x - 2.
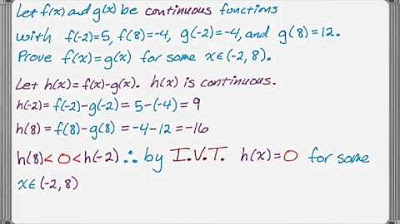
Under what conditions does the existence of real numbers a and c between 1 and 3, such that f of a times f prime of c equals b times d, is guaranteed?
-This condition is guaranteed if f is a twice differentiable function with f(1)=3, f'(1)=5, f''(1)=-2, f(3)=2, f'(3)=1, and f''(3)=0, and if b is between f(3) and f(1), and d is between f'(3) and f'(1).
Is there a value c between 1 and 3 such that the second derivative of f at c is equal to negative two?
-Yes, by the Mean Value Theorem, there exists a value c between 1 and 3 such that f''(c) is equal to (f'(3) - f'(1)) / (3 - 1), which simplifies to -2.
What is the significance of the intermediate value theorem in the context of the function f?
-The intermediate value theorem guarantees the existence of at least one value a between 1 and 3 where f(a) equals a given value b between f(1) and f(3), and similarly for the derivative f', ensuring the existence of a value c where f'(c) equals a given value d between f'(1) and f'(3).
How does the continuity of f and its derivative affect the application of the Mean Value Theorem?
-Since f is twice differentiable, both f and its derivative f' are continuous. This continuity allows for the application of the Mean Value Theorem, which requires continuity of the function and its derivative on the given interval.
What is the role of the fundamental theorem of calculus in finding the derivative of capital f?
-The fundamental theorem of calculus is used to find the derivative of capital f, as it states that the integral of a derivative is the original function, and thus the derivative of the integral (capital f) from 2 to x of little f of t dt is simply little f of x.
Outlines
📈 Calculus Fundamentals: Relative Extrema and Points of Inflection
This paragraph discusses the calculus of a continuous function 'little f' defined on the interval [-5, 8], represented by a graph consisting of line segments and a semicircle. The function 'capital f' is defined as the integral of 'little f' from 2 to x. The focus is on finding points where 'capital f' has a horizontal tangent line, determining whether these points represent relative minima, maxima, or neither. By applying the fundamental theorem of calculus, it is established that 'capital f prime of x' equals 'little f of x', which is zero at x = -2, 2, and 6. Consequently, 'capital f' has a relative maximum at x = -2, a relative minimum at x = 2, and neither at x = 6. Additionally, points of inflection are identified at x = 0, 4, and 6, where 'little f prime' changes sign, indicating a change in the concavity of 'capital f'. Finally, the absolute extrema of 'capital f' over the interval [-5, 8] are calculated by evaluating 'capital f' at critical points and endpoints, resulting in an absolute minimum at x = -5 and an absolute maximum at x = 8.
🧮 Derivatives and Integrals: Exploring Function g and h
The second paragraph introduces two additional functions, 'g' and 'h', derived from 'capital f'. Function 'g' is defined as the natural logarithm of 'capital f' over '2x', and the task is to find 'g prime of negative four'. This involves applying the chain rule and quotient rule to derive 'g prime of x', which is then evaluated at x = -4. The calculation requires determining 'capital f' and its derivative at x = -4, which involves integrating 'little f' over specific intervals. The paragraph concludes with the computation of 'g prime of negative four', resulting in a value of 1.25. Function 'h' is defined as 'little f' of 'three x squared minus two x' over 'e to the x minus one', and the problem is to find the equation of the tangent line to 'h' at x = 1. This involves calculating 'h of one' and 'h prime of x', applying the chain rule and quotient rule, and finding the slope of the tangent line at the given point. The tangent line equation is then formulated using the point-slope form.
🔍 Intermediate Value Theorem and Existence of Real Numbers
The third paragraph presents a twice differentiable function 'f' with specific values and derivatives at points 1 and 3. Given 'b' is between 2 and 3 and 'd' is between 1 and 5, the paragraph aims to show the existence of real numbers 'a' and 'c' within the interval [1, 3] such that 'f of a times f prime of c' equals 'b times d'. By leveraging the continuity of 'f' and its derivative 'f prime', the intermediate value theorem is applied to find 'a' and 'c' that satisfy the given conditions. Additionally, the paragraph inquires whether there exists a value 'c' between 1 and 3 for which 'f double prime of c' equals negative two. Using the mean value theorem, it is deduced that such a 'c' exists, with 'f double prime of c' being the average rate of change of 'f prime' over the interval [1, 3], which is calculated and shown to be negative two.
🧩 Mean Value Theorem Application and Function Analysis
The final paragraph focuses on the application of the mean value theorem to the twice differentiable function 'f'. Given specific values for 'f', 'f prime', and 'f double prime' at points 1 and 3, the paragraph seeks to justify the existence of a value 'c' between 1 and 3 where 'f double prime of c' equals negative two. It is established that 'f prime' is continuous and differentiable on the respective intervals, allowing the application of the mean value theorem. The value 'c' is found by calculating the average rate of change of 'f prime' from 1 to 3, which aligns with the given condition of 'f double prime of c' being negative two, confirming the existence of such a value 'c'.
Mindmap
Keywords
💡Continuous function
💡Integral
💡Derivative
💡Relative maximum/minimum
💡Point of inflection
💡Fundamental theorem of calculus
💡Chain rule
💡Quotient rule
💡Mean value theorem
💡Intermediate value theorem
💡Tangent line
Highlights
The function f is continuous on the closed interval [-5, 8] and consists of three line segments and a semicircle centered at (4, 0).
The function F(x) is defined as the integral from 2 to x of f(t) dt.
F has a relative maximum at x = -2 and a relative minimum at x = 2, with neither at x = 6.
Points of inflection for F occur at x = 0, 4, and 6, where the sign of the second derivative changes.
The absolute minimum of F on the interval [-5, 8] is at F(-5) = -1/2, and the absolute maximum is at F(8) which is slightly more than 8.
To find the derivative of g(x) = ln(F(x) / (2x)), the chain rule and quotient rule are applied.
g'(-4) is calculated using the integral of f from 2 to -4 and the value of f at -4.
The function h(x) is defined as f(3x^2 - 2x) / e^(x - 1), and its derivative h'(x) involves a quotient rule and chain rule.
The equation of the tangent line to the graph of h at x = 1 is derived using point-slope form.
Intermediate Value Theorem is used to show that there exist real numbers a and c such that f(a) * f'(c) = b * d for b between f(1) and f(3), and d between f'(1) and f'(3).
Mean Value Theorem is applied to show that there exists a value c between 1 and 3 such that f''(c) = (f'(3) - f'(1)) / (3 - 1).
It is concluded that there is a value c such that f''(c) = -2, based on the given values of f and its derivatives at 1 and 3.
Differentiability of f implies continuity of both f and its derivative f'.
The function f is twice differentiable, which ensures the continuity and differentiability of f' on the open interval (1, 3).
The mean value theorem is central to finding c such that f''(c) equals a specific value derived from f' at 1 and 3.
The process of finding the absolute extrema of F involves evaluating the function at critical points and endpoints of the interval.
The calculation of g'(-4) involves the fundamental theorem of calculus and the properties of logarithmic and rational functions.
The tangent line equation to h at x = 1 is found using the values of h at 1 and its derivative, showcasing the application of derivatives in geometry.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: