2023 AP Calculus AB & BC FRQ #1
TLDRThis video script presents a comprehensive walkthrough of a calculus problem from the 2023 Calculus AB and BC exams, focusing on a rate of change scenario involving gasoline flow. The problem involves interpreting the integral of a function representing the flow rate of gasoline in gallons per second over a specific time interval and using a right Riemann sum to approximate the total amount of gas pumped. The video also explores the Mean Value Theorem to determine if there exists a critical point where the flow rate's derivative is zero. Additionally, it models the rate of gasoline flow with a cosine function and calculates the average rate of flow over a given interval. Finally, the script concludes with finding and interpreting the derivative of the flow rate function at a specific time, indicating a decrease in flow rate. The video is an excellent resource for those studying calculus and seeking to understand the application of these concepts in real-world scenarios.
Takeaways
- 📈 The integral from 60 to 135 of f(T) dT represents the total amount of gasoline in gallons pumped between 60 and 135 seconds since pumping began.
- 📊 A right Riemann sum is used to approximate the integral, using given subintervals and corresponding function values from a table.
- 🔍 The Mean Value Theorem is applicable here because the function f is differentiable, implying it is also continuous, and there is a need to find a value of C where the derivative equals zero.
- 🤔 The existence of a value C between 60 and 120 such that f'(C) equals zero is guaranteed due to the function's properties and the Mean Value Theorem.
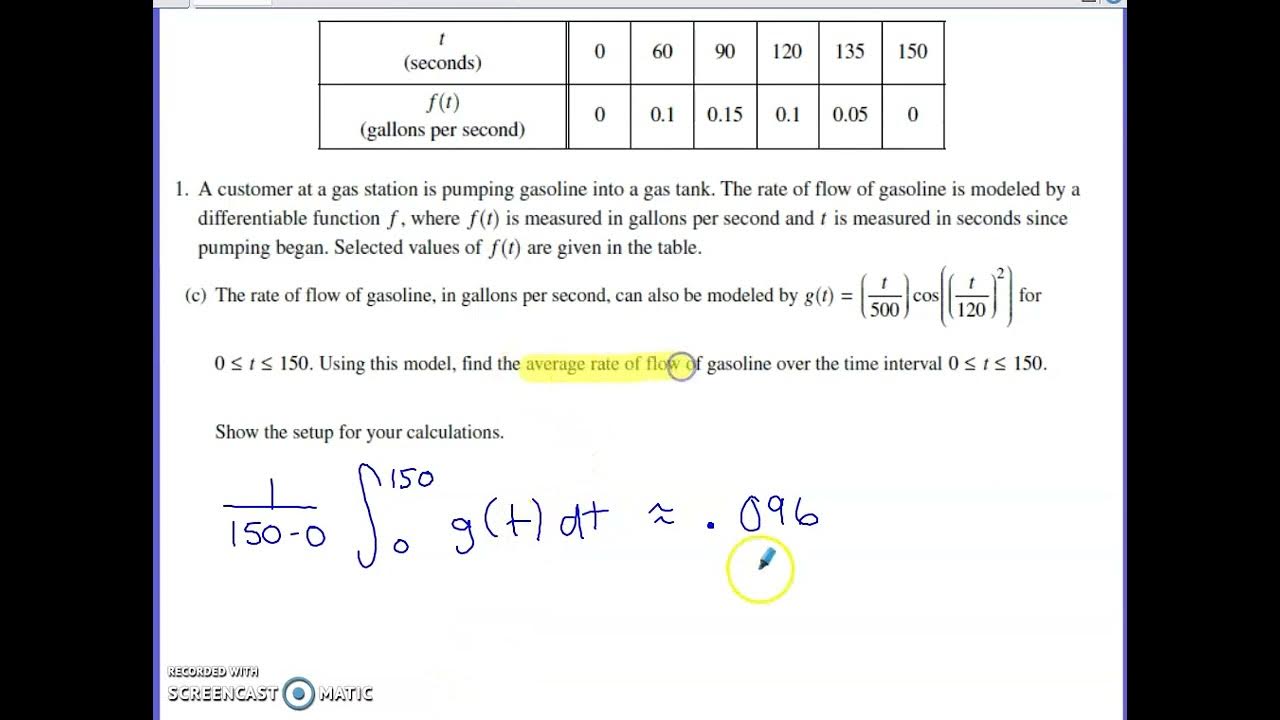
- 🌟 The rate of flow of gasoline can also be modeled by G(T) = T/500 * cos((T/120)^2), which is used to find the average rate of flow over a different interval.
- 🧮 The average rate of flow of gasoline is calculated by dividing the integral of G(T) from 0 to 150 by the interval length, resulting in approximately 0.096 gallons per second.
- ✅ The derivative G'(140) is found using the given model, which represents the rate of change of the flow of gasoline at 140 seconds.
- 📉 A negative value for G'(140) indicates that the rate of flow of gasoline is decreasing at the 140-second mark.
- 💧 The rate of decrease is 0.005 gallons per second per second, which is derived from the units of G and the time measurement.
- 📚 Understanding the context of the problem is crucial for interpreting the meaning of the mathematical results, especially when it comes to rates of change.
- 📝 The video script provides a step-by-step guide on how to approach calculus problems involving rates of change, integrals, and the Mean Value Theorem.
Q & A
What is the integral from 60 to 135 of f(T) dT representing in the context of the problem?
-The integral from 60 to 135 of f(T) dT represents the total amount of gasoline, measured in gallons, that has been pumped into the gas tank between 60 and 135 seconds since the pumping began.
How is the right Riemann sum used to approximate the integral in the problem?
-The right Riemann sum is used to approximate the integral by summing the product of the width of each subinterval and the function value at the right endpoint of each subinterval. In this case, it involves adding the products of the intervals from 60 to 90, 90 to 120, and 120 to 135 with their respective right endpoint values of 0.15, 0.1, and 0.05 respectively.
What does the Mean Value Theorem state in the context of the given problem?
-The Mean Value Theorem states that if a function is continuous on a closed interval and differentiable on an open interval within that closed interval, then there exists a point 'C' in that interval where the derivative of the function (f'(C)) equals the average rate of change over the interval. In this problem, it is used to find a value of 'C' between 60 and 120 where f'(C) equals zero.
How is the average rate of flow of gasoline calculated using the model G(T)?
-The average rate of flow of gasoline is calculated by finding the average value of the function G(T) over the interval from 0 to 150. This is done by dividing the integral of G(T) from 0 to 150 by the length of the interval, which is 150 seconds.
What does the derivative G'(140) represent in the context of the problem?
-The derivative G'(140) represents the rate of change of the flow of gasoline at the time T equals 140 seconds. Since the value is negative, it indicates that the flow rate is decreasing at that specific time.
What is the interpretation of a negative value for G'(140) in the context of the problem?
-A negative value for G'(140) indicates that the rate of flow of gasoline is decreasing at the time T equals 140 seconds. The magnitude of the negative value (approximately -0.005) represents the rate at which the flow is decreasing, measured in gallons per second per second.
Why is it important to consider the units when interpreting the integral and the average rate of flow?
-Considering the units is important because it ensures that the physical quantities are consistent and meaningful in the context of the problem. For example, the integral of the rate of flow should be in gallons since the flow rate is given in gallons per second.
What is the significance of the given table of values for f(T) in part A of the problem?
-The table of values for f(T) is significant as it provides specific data points that can be used to approximate the integral using the right Riemann sum. It allows for a numerical approximation of the total amount of gasoline pumped within a given time frame.
What does the function G(T) represent in the problem?
-The function G(T) represents the rate of flow of gasoline in gallons per second as a function of time T in seconds. It is used to model the flow rate at different times during the pumping process.
How does the Mean Value Theorem help in finding a value of C such that f'(C) equals zero?
-The Mean Value Theorem guarantees the existence of at least one value 'C' in the interval where the derivative of the function equals the average rate of change over that interval. Since the values of f are the same at 60 and 120 seconds, the average rate of change (and thus f'(C)) over this interval is zero.
What is the implication of the function being differentiable in the given problem?
-If the function is differentiable, it implies that it is also continuous. This is a prerequisite for applying the Mean Value Theorem, which is used to find a value 'C' where the derivative equals zero.
What does the term 'average value of a function' mean and how is it calculated?
-The 'average value of a function' over an interval is the integral of the function over that interval divided by the length of the interval. It gives a single value that represents the mean of the function over the entire interval.
Outlines
📈 Calculus Exam Problem: Rate of Change and Riemann Sum
The video begins with an introduction to a calculus problem from the 2023 Calculus AB and BC exams. It involves a rate of change scenario where gasoline is being pumped into a tank at a rate modeled by a differentiable function f(T), with T representing time in seconds and f(T) representing the flow rate in gallons per second. The video explains how to interpret the integral from 60 to 135 seconds as the total amount of gasoline pumped over that interval. It then demonstrates the use of a right Riemann sum to approximate the integral's value using given data points from a table. The problem also touches on the Mean Value Theorem to determine if there's a value C where the derivative of the flow rate equals zero within a specific time frame.
📚 Applying Mean Value Theorem and Calculating Average Rate of Flow
The second paragraph continues the discussion of the calculus problem by addressing part B, which involves the Mean Value Theorem. It confirms the conditions for applying the theorem, noting that differentiability implies continuity, and uses the given data points to show that there is indeed a value C where the derivative of the flow rate is zero. The video then moves on to part C, introducing a new function G(T) to model the rate of gasoline flow and calculating the average rate of flow over a 0 to 150 seconds interval. It emphasizes the process of finding the average value of a function, which involves dividing the integral of the function over the interval by the length of the interval. The solution is obtained using a calculator, resulting in an average rate of flow. Finally, part D focuses on finding the derivative of G at T equals 140 seconds and interpreting its meaning in the context of the problem, indicating that the flow rate is decreasing at that specific time.
Mindmap
Keywords
💡Rate of Change
💡Integral
💡Right Riemann Sum
💡Mean Value Theorem
💡Derivative
💡Average Rate of Flow
💡Gallons per Second
💡Secant Line
💡Differentiable Function
💡Table of Values
💡Contextual Interpretation
Highlights
The video discusses a rate of change problem from the 2023 Calculus AB and BC exams.
The rate of flow of gasoline is modeled by a differentiable function f(T), where T is time in seconds since pumping began.
The integral from 60 to 135 of f(T)dt represents the total amount of gas pumped over that time interval.
A right Riemann sum is used to approximate the integral, utilizing given subintervals and their corresponding function values.
Differentiability of the function f(T) implies continuity, allowing for the application of the Mean Value Theorem.
The Mean Value Theorem is used to find a value of C where the derivative f'(C) equals zero between 60 and 120 seconds.
An alternative model G(T) = T/500 * cos(T/120)^2 is introduced for the rate of flow of gasoline.
The average rate of flow of gasoline over the interval from 0 to 150 is calculated using the new model G(T).
The average value of a function represents the integral of the function divided by the interval.
The average rate of flow is found to be approximately 0.096 gallons per second using the model G(T).
The derivative G'(140) is calculated to interpret the rate of change of the flow of gasoline at 140 seconds.
A negative value for G'(140) indicates that the rate of flow of gasoline is decreasing at 140 seconds.
The rate of decrease is by approximately 0.005 gallons per second per second at 140 seconds.
The interpretation of G'(140) provides insight into the dynamics of gasoline flow at a specific time point.
The video provides a comprehensive walkthrough of solving a calculus problem involving rates of change and integrals.
The use of a table to approximate integrals and the application of the Mean Value Theorem are key components of the problem-solving strategy.
The video concludes with a summary of the entire question, reinforcing the understanding of the problem and its solution.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: