AP Calc AB & BC Practice MC Review Problems #1
TLDRThe video script is an educational walkthrough of calculus problems, presumably for AP Calculus preparation. The speaker begins by introducing the session as a review of 80 calculus multiple-choice questions, emphasizing that while the problems are not from actual AP exams, they are closely modeled after them. The video covers a range of topics including derivatives, integrals, limits, and applications of calculus to real-world scenarios, such as calculating the distance traveled by a truck or the number of people entering a concert hall. Each problem is solved step by step, with the speaker providing clear explanations and alternative methods for solving them. The script also touches on the importance of showing work and checking answers, especially for exams. The session concludes with a reminder that all problems are based on past AP exam questions, encouraging students to practice these types of problems for thorough exam preparation.
Takeaways
- 📚 The video is a calculus multiple-choice review, focusing on problems based on AP exams.
- ✍️ The presenter rewrites the function f(x) = 4/x^2 + 2^x - 4 to make it easier to find the derivative.
- 🔍 The derivative of f(x) is found using the power rule and chain rule, resulting in f'(x) = -8x^(-3) + 2ln2.
- 📉 For limits, the presenter demonstrates how to find them from the left and right, emphasizing the importance of continuity.
- 🧮 The presenter uses the fundamental theorem of calculus to find antiderivatives and solve for constants, like when f(3) = 2.
- 🎫 The video covers how to use Riemann sums to approximate the number of people entering a concert hall over time.
- 🚚 An application of derivatives is shown through calculating the distance a truck traveled using a trapezoidal sum.
- 📈 The product rule and chain rule are applied to find derivatives of functions, such as dy/dx for y = e^(3x)(x^2 - 3).
- 🤔 The presenter emphasizes the importance of understanding all calculus rules for the AP exam, including derivatives and antiderivatives.
- ⏲ The average rate of change is calculated as an example of a basic concept that can appear on the AP calculus exam.
- 📈 The video concludes with a reminder that all problems are based on past AP exam questions, encouraging students to practice them.
Q & A
What is the first problem discussed in the video about?
-The first problem is about finding the derivative of a given function and then evaluating it at a specific point, specifically f'(-2) for the function f(x) = 4/(x^2) + 2^x - 4.
What is the method used to find the integral of 8e^(2x) dx in the second problem?
-The method used is integration by substitution, where e^(2x) is treated as 'u', and the integral is solved by applying the chain rule and then substituting back to get the final answer as 4e^(2x) + C.
How is the limit of f(x) as x approaches 2 determined in the third problem?
-The limit is determined by evaluating the function from both the left and the right as x approaches 2. Since the values from the left and right are different, it is concluded that the limit does not exist.
What is the approach to find f(x) given F'(x) = 9x^2 - (1/2)x + 1 and f(3) = 2 in the fourth problem?
-The approach is to first find the antiderivative of F'(x) to get f(x), then use the given value of f(3) to solve for the constant C, and finally evaluate f(-1).
What is the Riemann sum approximation method used in the fifth problem?
-The left Riemann sum is used with four subintervals and the given data from the table to approximate the number of people who enter the concert hall during the first 20 minutes.
How is the derivative dy/dx of the function y = e^(3x)(x^2 - 3) found in the sixth problem?
-The derivative is found using the product rule, where the derivative of the first part (e^(3x)) is multiplied by the second part (x^2 - 3), and the derivative of the second part is multiplied by the first part, then the two results are added.
What is the process to find the derivative f'(x) of the function f(x) = (3x^4 - 2)^(5/2) in the seventh problem?
-The process involves applying the chain rule to the function, where the derivative of the outer function (5/2) is multiplied by the derivative of the inner function (3x^4 - 2), which is 12x^3.
How is the antiderivative of 2/(5x - 20) found in the eighth problem?
-The antiderivative is found by first factoring out constants and then using a substitution method, which leads to the natural logarithm function, resulting in (2/5) * ln|x - 4| + C.
What is the quotient rule applied to find the derivative f'(x) of the function f(x) = (4 - 3x)/(x^4 + 3) in the ninth problem?
-The quotient rule is used where the derivative of the numerator is multiplied by the denominator and subtracted from the numerator multiplied by the derivative of the denominator, all divided by the square of the denominator.
How is the approximate distance the truck traveled from time T = 0 to T = 7 found in the tenth problem?
-The approximate distance is found using a trapezoidal sum with three subintervals as indicated by the table, which involves calculating the average of the velocities at the endpoints of each subinterval and summing these values.
What is the process to find f'(√(3π)) for the function f(x) = x * sin(x^2) in the eleventh problem?
-The process involves first applying the product rule to find the derivative of the function, then simplifying the derivative, and finally substituting √(3π) into the derivative to evaluate it.
How is the average rate of change of f on the closed interval from -2 to 3 calculated in the twelfth problem?
-The average rate of change is calculated by finding the difference in the function values at the endpoints divided by the difference in the x-values, which is f(3) - f(-2) / (3 - (-2)).
Outlines
📚 AP Calculus Multiple-Choice Review
This video provides a comprehensive review of calculus multiple-choice problems based on AP exams. The presenter walks through each problem, starting with a function f(x) and finding its derivative at a specific point. The video also covers integral calculus, including the integral of 8e^(2x), and the limit of a function as x approaches a certain value. The presenter emphasizes the importance of showing work and checking answers, especially for the AP exam.
🧮 Solving for Antiderivatives and Using the Fundamental Theorem
The second paragraph delves into finding antiderivatives and applying the fundamental theorem of calculus. The presenter solves for F(x) given F'(x) and a point F(3), then calculates F(-1). The process involves integrating the derivative function, plugging in values to solve for the constant C, and finally evaluating the function at the desired point. The explanation highlights the need for precision and methodical work when tackling calculus problems.
🎟️ Approximating the Number of People Entering a Concert Hall
This section focuses on using a Riemann sum with four subintervals to approximate the number of people entering a concert hall during the first 20 minutes. The presenter outlines the steps for a left Riemann sum, emphasizing the importance of using the left endpoint for each interval. By multiplying the rate of entry by the time interval and summing these products, the presenter provides an approximation of the total number of people who entered.
🚚 Calculating the Distance Traveled by a Truck
The fourth paragraph discusses the use of a trapezoidal sum to estimate the distance a truck traveled between two time points. Given the truck's velocity at various times, the presenter demonstrates how to calculate the area under the velocity curve using trapezoidal approximation. The process involves summing the products of the average heights and bases of each trapezoid, providing a practical example of integral calculus in motion problems.
📈 Finding Derivatives and Evaluating Functions
The final paragraph covers several calculus problems involving finding derivatives and evaluating functions. The presenter tackles a variety of problems, including using the product rule, chain rule, and quotient rule for derivatives. Each problem is solved step by step, with the presenter providing clear explanations and emphasizing the importance of understanding and applying calculus rules. The video concludes with a reminder that these problems are based on AP exam questions, encouraging viewers to practice and prepare thoroughly.
Mindmap
Keywords
💡Calculus
💡Derivative
💡Integral
💡Multiple-choice questions
💡Product Rule
💡Chain Rule
💡Quotient Rule
💡Riemann Sum
💡Trapezoidal Rule
💡Average Rate of Change
💡Unit Circle
Highlights
The video provides a review of 80 calculus multiple-choice problems based on AP exams.
The presenter rewrites the function f(x) = 4/(x^2) + 2^x - 4 before finding its derivative.
The derivative of f(x) is found using the power rule and chain rule.
The value of f'(-2) is calculated by substituting into the derivative.
The integral of 8e^(2x) is solved by taking 8 out and applying the chain rule.
The limit of f(x) as x approaches 2 is found by considering the left and right limits.
The limit does not exist since the left and right limits are different.
The antiderivative of 9x^2 - 1/2x + 1 is found by integrating each term.
The constant C is solved for using f(3) = 2.
f(-1) is found by plugging in -1 into the rewritten antiderivative.
A Riemann sum with 4 subintervals is used to approximate the number of people entering a concert hall.
The left Riemann sum is calculated using the given values in the table.
The product rule is applied to find the derivative of y = e^(3x)(x^2 - 3).
The chain rule is used to find the derivative of f(x) = (3x^4 - 2x^5)^(1/2).
An antiderivative problem is solved using natural log and reverse power rule.
The quotient rule is applied to find the derivative of f(x) = (4 - 3x)/(x^4 + 3).
A trapezoidal sum with 3 subintervals is used to approximate the distance a truck travels.
The distance is calculated by summing the areas of the trapezoids.
The derivative of f(x) = x*sin(x^2) is found using the product rule and evaluated at x = sqrt(3*pi).
The average rate of change of f(x) = 2x^3 - 5x^2 on the interval [-2,3] is found using Algebra 1 slope formula.
Transcripts
Browse More Related Video
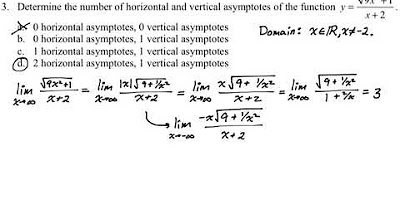
AP Calc AB & BC Practice MC Review Problems #6
AP Calculus AB/BC Multiple Choice Practice Test (2012 AP CED Problems)
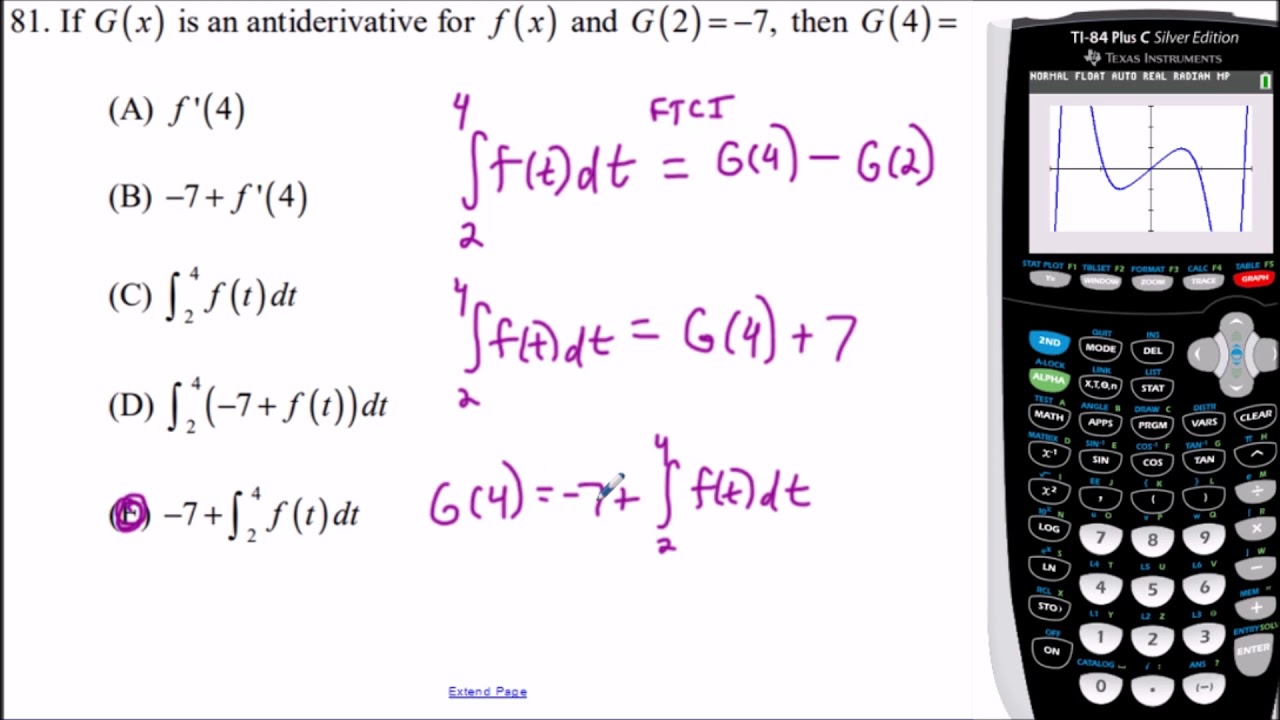
AP Calculus AB 2008 Multiple Choice (Calculator) - Questions 76-92

AP Calculus Multiple Choice Practice Test (2020 AP CED Problems)

AP Calculus: EVERYTHING YOU NEED TO KNOW

AP Calc AB & BC Practice MC Review Problems #2
5.0 / 5 (0 votes)
Thanks for rating: