The Chain Rule - Part 2
TLDRThe video script is an educational guide on the application of the chain rule in calculus, focusing on the differentiation of composite functions. It begins with a straightforward example involving cubing the quantity (3x - 7), illustrating the power rule and the chain rule by breaking down the process into understandable steps. The script then progresses to more complex scenarios, including the differentiation of expressions within radicals and exponential functions, emphasizing the importance of not forgetting to multiply by the derivative of the inner function. It also touches on the derivative of logarithmic functions. The video further explores the use of the chain rule in conjunction with the product and quotient rules, providing detailed examples and emphasizing the need for factoring out common terms for simplification. The script concludes with a quotient rule example, demonstrating how to handle composite functions in the denominator, and encourages viewers to practice simplifying the derived expressions.
Takeaways
- 📚 The concept of a composite function is introduced as a combination of an inner function 'u' and an outer function.
- 🔢 The power rule is applied by bringing down the exponent and reducing the power by one when differentiating.
- 📈 The chain rule involves multiplying the derivative of the outer function by the derivative of the inner function.
- 🎓 For exponential functions, the derivative is the function itself multiplied by the derivative of the exponent.
- 📉 The derivative of a logarithmic function is found by using 1 over the inner function.
- 🤝 The product rule is used when differentiating a product of two functions, combining the derivative of the first times the second, plus the first times the derivative of the second.
- 🔄 When simplifying expressions, look for common factors and factor them out to simplify the derivative.
- 💯 The quotient rule is applied by finding the derivative of the numerator and the denominator, then dividing them and simplifying.
- 📌 The importance of including the derivative of the inner function in the chain rule cannot be overstated, as it is often a point of confusion.
- 🧮 When dealing with more complex expressions, it's crucial to apply the chain rule correctly, ensuring that the derivative of the inner function is included in the final expression.
- 📐 The script provides several examples of applying the chain rule in conjunction with other differentiation rules, such as the product and quotient rules, to solve calculus problems.
Q & A
What is a composite function in the context of calculus?
-A composite function is a function composed of two or more functions, where the output of one function is used as the input for the next function. It is often denoted as f(g(x)) or y = f(u), where u = g(x).
What is the power rule in calculus?
-The power rule is a basic rule used to find the derivatives of functions. It states that if y = x^n, then the derivative dy/dx = n * x^(n-1).
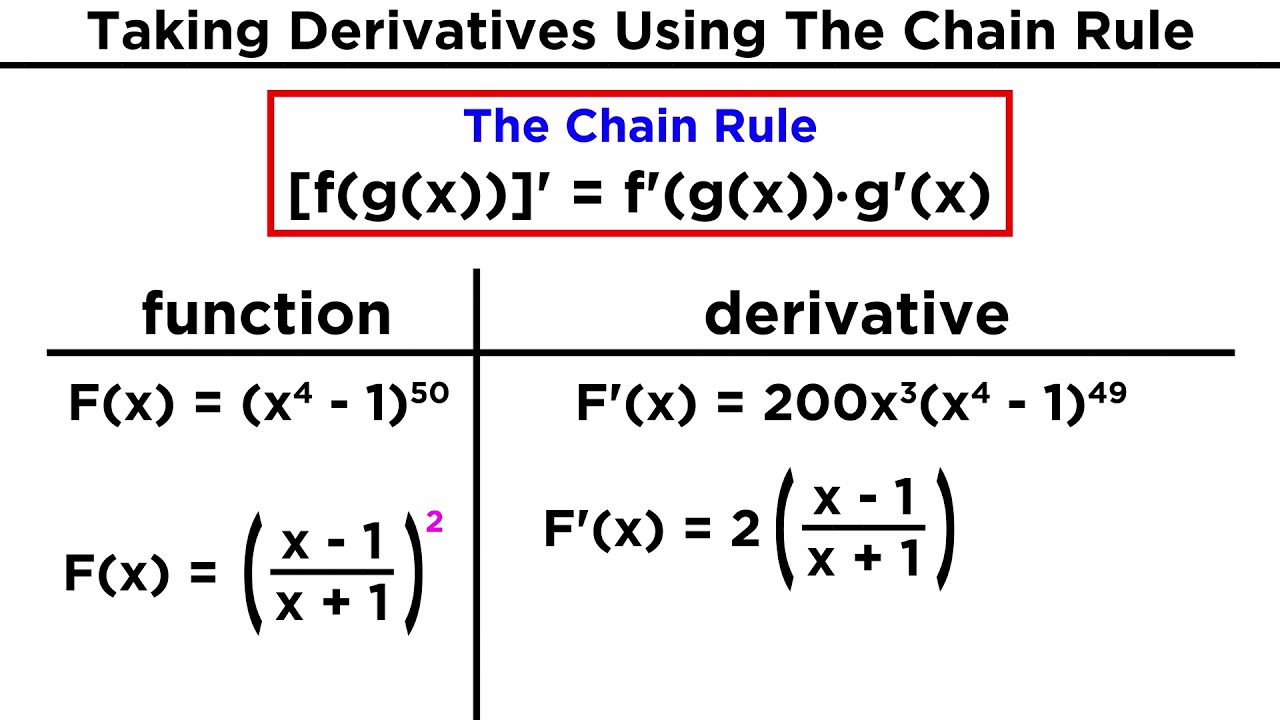
How do you apply the chain rule to the function y = (3x - 7)^3?
-You first apply the power rule by bringing down the exponent (3) and reducing it by 1, resulting in 3 * (3x - 7)^2. Then, you multiply this by the derivative of the inside function, which is 3, to get the final derivative 9 * (3x + 7)^2.
What is the role of the chain rule in differentiating composite functions?
-The chain rule is used to find the derivative of a composite function by multiplying the derivative of the outer function by the derivative of the inner function.
How do you differentiate a function with a square root, like y = √(2x - 5)?
-You apply the power rule by bringing down the exponent (1/2) and reducing it by 1, which gives 1/2 * (2x - 5)^(-1/2). Then, you multiply by the derivative of the inside function, which is 2, to get the final derivative 1 * (2x - 5)^(-1/2) or 1/√(2x - 5).
What is the derivative of an exponential function y = e^x?
-The derivative of y = e^x is simply e^x, as the exponential function e^x is its own derivative.
How do you use the product rule when differentiating a function that is a product of two functions?
-The product rule states that the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function, expressed as (f'g + fg').
What is the quotient rule in calculus?
-The quotient rule states that the derivative of a quotient of two functions is the denominator times the derivative of the numerator minus the numerator times the derivative of the denominator, all divided by the square of the denominator, expressed as (f'g - fg') / g^2.
How do you simplify the derivative of a function using common factors?
-You identify common factors in the terms of the derivative and factor them out. This often involves finding the lowest power of terms that are repeated and factoring out this power, leaving the remaining terms inside parentheses.
What is the derivative of y = ln(x) with respect to x?
-The derivative of the natural logarithm function y = ln(x) with respect to x is 1/x.
Can you combine the chain rule with other differentiation rules such as the product and quotient rule?
-Yes, the chain rule can be combined with the product rule and the quotient rule to find the derivatives of more complex functions that involve products or quotients of composite functions.
Outlines
📚 Introduction to Chain Rule and Composite Functions
This paragraph introduces the concept of the chain rule in calculus, focusing on composite functions. The video demonstrates the process of differentiating a composite function like y = (3x - 7)^3, where (3x - 7) is the inner function 'u', and x^3 is the outer function. The power rule is applied by bringing down the exponent and reducing it by one, followed by multiplying by the derivative of the inner function, which in this case is 3. The process is neatly explained with steps to rewrite the expression in a simplified form, emphasizing the importance of not overcomplicating the final answer.
🔢 Applying Power, Exponential, and Logarithmic Differentiation
The second paragraph delves into various applications of the chain rule, including power functions, exponential functions, and logarithms. It covers the differentiation of y = (5x^2 - 2)^(1/2) using the power rule and chain rule, resulting in y' = (1/2)(2x - 5)^(-1/2) * 2. The paragraph also touches on the derivative of exponential functions, such as y = e^(4x^3 - 2x + 1), and the importance of appending the derivative of the inner function when applying the chain rule. Logarithmic differentiation is also discussed, with the derivative of log(x) being 1/x, and this is applied to an example involving a logarithmic function with an inner function of (3x^2 + 2).
🤝 Combining Chain Rule with Product and Quotient Rules
The final paragraph discusses the application of the chain rule in conjunction with the product and quotient rules. It begins with a product rule example involving f(x) = x^2 and g(x) = (3x - 5)^5, showing how to find the derivative of their product. The process includes finding the derivatives of both functions, applying the product rule, and then simplifying the expression by factoring out common terms. The paragraph concludes with a quotient rule example, where a function is divided by a composite function in the denominator. The quotient rule is applied, and the derivative is simplified by combining like terms and using the chain rule for the denominator. The summary emphasizes the need to find the derivative of both the numerator and the denominator and to simplify the final expression for clarity.
Mindmap
Keywords
💡Chain Rule
💡Composite Function
💡Power Rule
💡Derivative
💡Exponential Function
💡Logarithm
💡Product Rule
💡Quotient Rule
💡Factoring
💡Common Factors
💡Foil Method
Highlights
Introduction to using the chain rule with composite functions
Example 1: y = (3x - 7)^3, applying power rule and chain rule
Example 2: y = (5x^2 - 2)^(1/2), power rule for square root and chain rule
Example 3: y = e^(4x^3 - 2x + 1), derivative of exponential function and chain rule
Example 4: y = ln(3x^2 + 2), derivative of natural log and chain rule
Product rule applied to f(x) = x^2 and g(x) = (3x - 5)^5
Simplifying product rule derivative by factoring out common terms
Quotient rule applied to a composite function in the denominator
Simplifying quotient rule derivative using foil method
Combining chain rule with product and quotient rules for more complex examples
Emphasis on not forgetting to tack on the derivative of the inside function in the chain rule
Using the power rule to bring down the exponent and reduce the power by 1
Leaving the expression in simplified form without expanding everything out
Derivative of an exponential function is the function itself
Derivative of the log of x is simply 1/x
Product rule formula: f'g + fg'
Quotient rule formula: (f'g - fg') / g^2
Simplifying expressions by looking for common factors
Factoring out the lowest power when common factoring
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: