Email Answer about When To Use U-Sub
TLDRThe video script discusses strategies for approaching the integration of generic functions. It emphasizes the importance of starting with basic integration rules, such as the power rule, before attempting more complex methods. If the power rule doesn't apply, the script suggests trying algebraic manipulation to simplify the integral. An example given is transforming (x^2 + 1) over (x) into (x + 1) over (x), which can then be integrated more easily. If neither basic rules nor algebraic simplification is possible, the script recommends using a substitution method, such as setting u = x^2 and du = 2x, to proceed with the integration. The speaker encourages viewers to think critically about each step and to apply these strategies systematically, much like they would when differentiating functions. The summary aims to engage users by highlighting the logical progression of solving integrals and the importance of methodical problem-solving in calculus.
Takeaways
- 📚 Always start by attempting to integrate using basic power rules.
- 🔍 If the power rule doesn't work, consider if algebraic manipulation can simplify the integral.
- 🔢 Apply algebra to break down complex expressions into simpler forms that can be integrated more easily.
- ➗ Utilize the quotient rule when the integral is in the form of a fraction, particularly when it can be simplified.
- 📉 Recognize when to switch to more advanced techniques like u-substitution when basic rules and algebra are insufficient.
- 👉 In u-substitution, choose an appropriate u that simplifies the integral, often involving a substitution that makes the integral resemble a basic form.
- 📐 Use the chain rule in conjunction with u-substitution to find the differential dx in terms of du.
- 🔧 Understand that the process of integration mirrors the process of differentiation, with similar steps of applying rules, algebra, and substitution.
- ⚖️ Balance the need for algebraic simplification with the application of integration rules to effectively solve integrals.
- 🚫 Avoid complex methods initially; only resort to u-substitution when simpler methods like power rules and algebra fail.
- 🤔 Encourage a thoughtful approach where each step is considered before moving on to more advanced techniques.
- 📝 Keep in mind the constant of integration (+C) when performing integration to account for any constant term that may be present in the antiderivative.
Q & A
What is the general strategy for approaching generic integrals?
-The general strategy involves trying basic integration power rules first, then considering algebraic manipulations, and finally using techniques like the quotient rule or u-substitution if necessary.
What is the first step when integrating a function like f(x) = x^3?
-The first step is to check if you can integrate it using a basic power rule, which in this case would be ∫x^3 dx = (1/4)x^4 + C.
When might algebraic manipulation be useful before using integration rules?
-Algebraic manipulation can be useful when the integral has a form that can be simplified or broken down into simpler parts that can be integrated more easily, such as x^2 + 1/x.
How can you simplify the integral of x^2 + 1/x using algebra?
-You can factor out x from the first term and rewrite the integral as ∫(x * (1 + 1/x^2)) dx, which simplifies to ∫(x + 1/x) dx.
What is the quotient rule in the context of integration?
-The quotient rule is used when integrating a function that is the quotient of two other functions. It allows you to integrate functions of the form ∫(a(x) / b(x)) dx.
Can you provide an example of when u-substitution would be necessary?
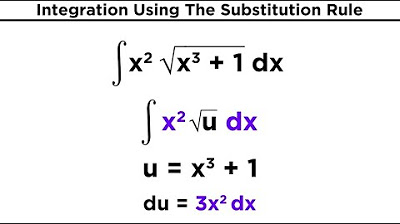
-U-substitution is necessary when the integral does not conform to basic power rules or cannot be simplified through algebra. An example is when you have an integral involving a function of the form ∫(x^n)^m dx.
How do you apply u-substitution to the integral of (x^2)^(-7) dx?
-You would let u = x^2, so du/dx = 2x, and dx = du/(2x). The integral then becomes ∫u^(-7) * (du/(2x)) which simplifies to ∫(u^(-7) / 2) du.
What is the purpose of the constant C in an indefinite integral?
-The constant C is used to represent the constant of integration, which accounts for the fact that indefinite integrals (antiderivatives) can differ by a constant.
Why is it important to try basic integration rules before moving on to more complex methods?
-Trying basic integration rules first is important because it can save time and effort if the integral can be solved easily. If the basic rules don't work, then it's clear that more complex methods are needed.
How does the process of integrating a function relate to the process of differentiating a function?
-Both processes involve trying to simplify the function to apply known rules. In differentiation, you start with basic power rules and move to product, quotient, and chain rules as needed. In integration, you start with basic power rules and move to algebraic manipulation and u-substitution.
What does the phrase 'my dog is acting a fool' mean in this context?
-This is a colloquial expression used to indicate that the speaker's dog is behaving in a playful or disruptive manner. It is not directly related to the mathematical discussion and is likely an aside or interruption.
What is the final step in the strategy for approaching generic integrals after trying basic rules and algebra?
-The final step is to use more advanced integration techniques such as the quotient rule or u-substitution if the integral cannot be solved using basic rules or algebraic manipulations.
Outlines
📚 Introduction to Strategies for Solving Generic Integrals
The paragraph introduces a strategy for tackling generic integrals. It begins by posing a question about the general approach one should take when dealing with integrals, such as when to use regular integration, algebraic manipulation, or integration by parts. The speaker uses the example of the function f(x) = x^3 to illustrate the process of first attempting a straightforward integration using basic rules. The paragraph then moves on to discuss the importance of considering algebraic manipulation before resorting to more complex rules like the product rule or quotient rule. It emphasizes the need to think critically about the problem, similar to the approach one would take when deriving functions.
Mindmap
Keywords
💡Generic Integrals
💡Basic Power Rule
💡Algebraic Manipulation
💡Quotient Rule
💡Integration by Parts
💡U-Substitution
💡Natural Logarithm
💡Absolute Value
💡Constant of Integration (C)
💡Derivative
💡Indefinite Integral
Highlights
Approaching generic integrals involves first trying to integrate using basic power rules.
If the basic power rule doesn't work, consider if algebraic manipulation can simplify the integral.
For complex expressions like x squared plus 1 over X, algebraic manipulation can simplify the integral to X plus 1 over X.
When regular integration and algebra are not possible, use the quotient rule for integration.
The integral of x over x squared plus 1 can be solved by first flipping the fraction and then applying the quotient rule.
Always start with basic integration rules before moving on to more complex methods.
If basic rules are inapplicable, try algebraic distribution to simplify the integral.
For certain integrals, such as 5x to the power of -4, algebra allows for simplification to a basic rule.
When neither basic rules nor algebra work, use u-substitution as a last resort.
In the case of u-substitution, choose an appropriate u variable that simplifies the integral.
For the integral involving x squared to the power of -7, u equals x squared to the power of -7 is a suitable substitution.
After u-substitution, differentiate u with respect to x to find du/dx, which aids in solving the integral.
The process of integration involves a systematic approach: basic rules, algebra, quotient rule, and finally u-substitution.
The strategy for integration mirrors the process of differentiation, emphasizing a logical and structured problem-solving approach.
The importance of understanding when to apply each method in integration is crucial for solving complex integrals.
The transcript provides a clear guideline for students to think through the process of integration in a methodical way.
The speaker encourages students to ask questions to ensure understanding of the integration process.
The transcript concludes with a reminder to always attempt basic rules and algebra before resorting to u-substitution.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: