Marginal cost & differential calculus | Applications of derivatives | AP Calculus AB | Khan Academy
TLDRThe video script discusses the concept of cost functions and their visualization in relation to quantity produced over a week. It explains that even with no production, there are fixed costs such as rent and salaries. As production increases, costs rise at an accelerating rate due to factors like the scarcity of raw materials. The derivative of the cost function with respect to quantity, or marginal cost, represents the rate at which costs increase for each additional unit produced. This concept is crucial for determining the optimal production level, as it helps manufacturers decide when the costs of producing an extra unit exceed the revenue it generates. The script also touches on related economic concepts such as marginal profit and marginal revenue, emphasizing the importance of understanding the rate of change in costs and revenue as production levels vary.
Takeaways
- 📈 **Cost Function Visualization**: The cost of production can be visualized as a function of quantity, with fixed costs and variable costs increasing at different rates.
- 💲 **Fixed Costs**: There are fixed costs that must be paid regardless of production level, such as rent and salaries.
- 📌 **Cost at Specific Quantity**: At a given quantity, like 100 units, the cost includes both fixed and variable costs, which can be represented on the cost function graph.
- 🔄 **Derivative as Marginal Cost**: The derivative of the cost function with respect to quantity (c'(q)) represents the marginal cost, which is the rate at which cost increases with each additional unit produced.
- ↗️ **Slope as Marginal Cost**: The slope of the tangent line to the cost function at any point represents the marginal cost at that quantity level.
- 📉 **Variable Cost Increase**: As quantity increases, variable costs rise, and they may do so at an increasing rate, reflecting factors like scarcity of raw materials.
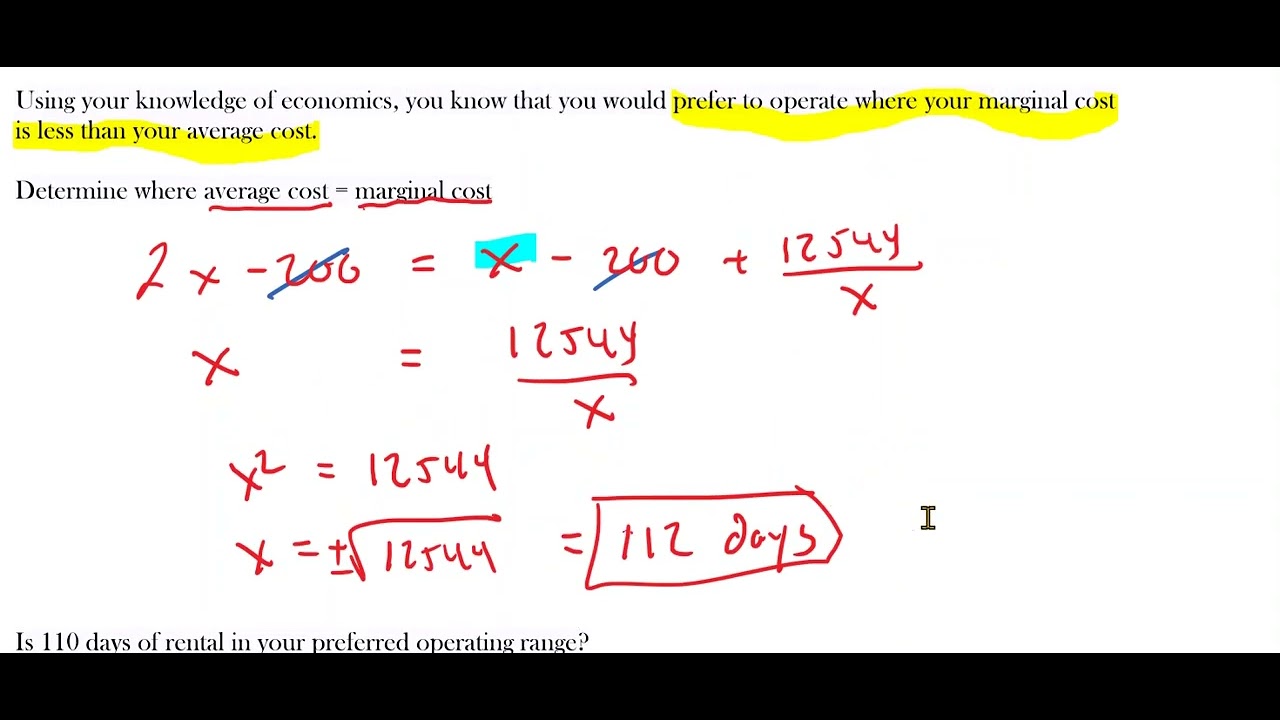
- 🤔 **Economic Decision-Making**: Marginal cost informs production decisions, helping to determine the optimal level of production where additional costs do not exceed revenue.
- 🍊 **Scarcity and Cost**: The cost of raw materials may increase as they become scarcer, leading to higher marginal costs as production levels rise.
- 🔮 **Profit Maximization**: By understanding marginal cost, a producer can decide when to stop production to maximize profit, avoiding producing units that cost more to make than they can be sold for.
- 📚 **Economics and Calculus Connection**: Calculus provides a mathematical framework for economic concepts like marginal cost, which is the derivative of the cost function.
- 📈 **Revenue and Profit Functions**: Similar to cost, revenue and profit can also be modeled as functions of quantity, with their derivatives representing marginal revenue and marginal profit, respectively.
Q & A
What does the cost function represent in the context of the script?
-The cost function represents the total cost of production as a function of the quantity produced over a week. It includes both fixed costs, which are incurred regardless of production levels, and variable costs, which increase with the quantity produced.
What are fixed costs in the context of the factory operation?
-Fixed costs are the expenses that a factory must pay regardless of the level of production, such as rent for the factory and salaries for employees.
How does the cost function change with an increase in production quantity?
-As the production quantity increases, the total cost also increases, but at an accelerating rate. This is due to the variable costs associated with higher production levels.
What does the derivative of the cost function with respect to quantity (c'(q)) represent?
-The derivative c'(q) represents the marginal cost, which is the rate at which the total cost changes with respect to the quantity produced. It is the slope of the tangent line to the cost function at a given quantity.
Why is the concept of marginal cost important for a factory owner?
-Marginal cost is important because it helps the factory owner determine the optimal level of production. It indicates the additional cost of producing one more unit, which can guide decisions on whether to increase or decrease production.
What does the slope of the tangent line to the cost function at a certain quantity signify?
-The slope of the tangent line signifies the instantaneous rate of change of the cost with respect to quantity at that specific point. It tells us how much the cost increases for producing an additional unit of the product.
How does the concept of marginal cost relate to the decision of when to stop producing?
-Marginal cost is used to determine the point of production where the cost of producing one more unit equals the revenue from selling it. If the marginal cost exceeds the selling price, it's no longer profitable to produce more units.
What could cause the marginal cost to increase as production increases?
-The marginal cost could increase due to factors such as scarcity of raw materials, increasing market prices for inputs, or inefficiencies in production at higher volumes.
What is the economic term for the rate at which costs are increasing for an incremental unit?
-The economic term for the rate at which costs are increasing for an incremental unit is 'marginal cost'.
How does the concept of marginal revenue relate to the decision-making process for a factory?
-Marginal revenue is the additional revenue generated from selling one more unit of a product. It is used in decision-making to determine if the revenue from an additional unit exceeds the marginal cost, which would make producing that unit profitable.
What is the role of calculus in understanding economic concepts like cost functions and marginal costs?
-Calculus provides the mathematical tools to analyze economic concepts by allowing for the calculation of derivatives, which represent rates of change. In the context of cost functions, the derivative gives the marginal cost, which is crucial for production and pricing decisions.
How can a factory owner use the marginal cost to optimize their production levels?
-A factory owner can use the marginal cost to find the production level where the marginal cost equals the marginal revenue. This point is typically where the profit is maximized, as producing beyond this point would lead to higher costs than revenue.
Outlines
📈 Understanding Cost Functions and Marginal Cost
The paragraph discusses the concept of cost functions in the context of running a factory. It explains how costs can vary with quantity produced over a week, using a visual representation to illustrate the relationship. The fixed costs, such as rent and salaries, are highlighted, along with the increasing variable costs as production quantity grows. The derivative of the cost function with respect to quantity (marginal cost) is introduced as a measure of the rate at which costs increase for each additional unit produced. The importance of understanding marginal cost is emphasized for decision-making regarding production levels, particularly when considering whether the cost of producing an additional unit is less than the revenue it generates.
Mindmap
Keywords
💡Cost Function
💡Fixed Costs
💡Variable Costs
💡Derivative
💡Marginal Cost
💡Tangent Line
💡Instantaneous Rate of Change
💡Scarcity
💡
💡Profit Maximization
💡Marginal Revenue
💡Quantity on the Margin
Highlights
The cost function of a factory visualized as a graph, illustrating how cost varies with quantity.
Fixed costs, such as rent and salaries, are present even if no production occurs, set at $1,000 per week.
As production quantity increases, costs rise at an accelerating rate due to factors like raw material scarcity.
The derivative of the cost function with respect to quantity represents marginal cost.
Marginal cost is the rate at which costs increase for each additional unit produced.
The concept of the derivative as the slope of the tangent line to the cost function is introduced.
The instantaneous rate of change in cost with respect to quantity is explained through calculus.
The economic significance of marginal cost in determining the optimal level of production is discussed.
The impact of increasing raw material scarcity on the cost of production is examined.
The decision-making process for production based on marginal cost and selling price is explained.
The concept of marginal revenue and its role in economic decision-making is introduced.
The importance of understanding the rate at which costs increase on the margin for business operations is emphasized.
The economic concept of marginal profit and its calculation through the derivative of profit with respect to quantity.
The relationship between the derivative of revenue with respect to quantity and marginal revenue is discussed.
The practical application of calculus in economics to determine the incremental cost of production.
The strategic use of marginal cost to decide when to cease production due to unprofitability.
An example scenario involving orange juice production to illustrate the concept of marginal cost.
The economic rationale for considering transportation costs and market availability in production decisions.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: