The Binomial Distribution: Crash Course Statistics #15

CrashCourse
9 May 201814:15
EducationalLearning
32 Likes 10 Comments

TLDRThis video introduces mathematical shortcuts to calculate probabilities, including the multiplication rule, binomial distribution formula, and Bernoulli distribution. It walks through examples like predicting getting shocked by a toaster, having a certain number of boys and girls in a family, encountering zombies during an apocalypse, and surviving a blood transfusion. These formulas help make probability calculations more efficient so we can spend less time calculating and more time applying probabilities to real-life situations.

Takeaways
  • 😀 Shortcut formulas like the binomial distribution can help calculate probabilities instead of doing lengthy manual calculations.
  • 😃 The binomial distribution calculates the probability of a certain number of successes out of a series of trials.
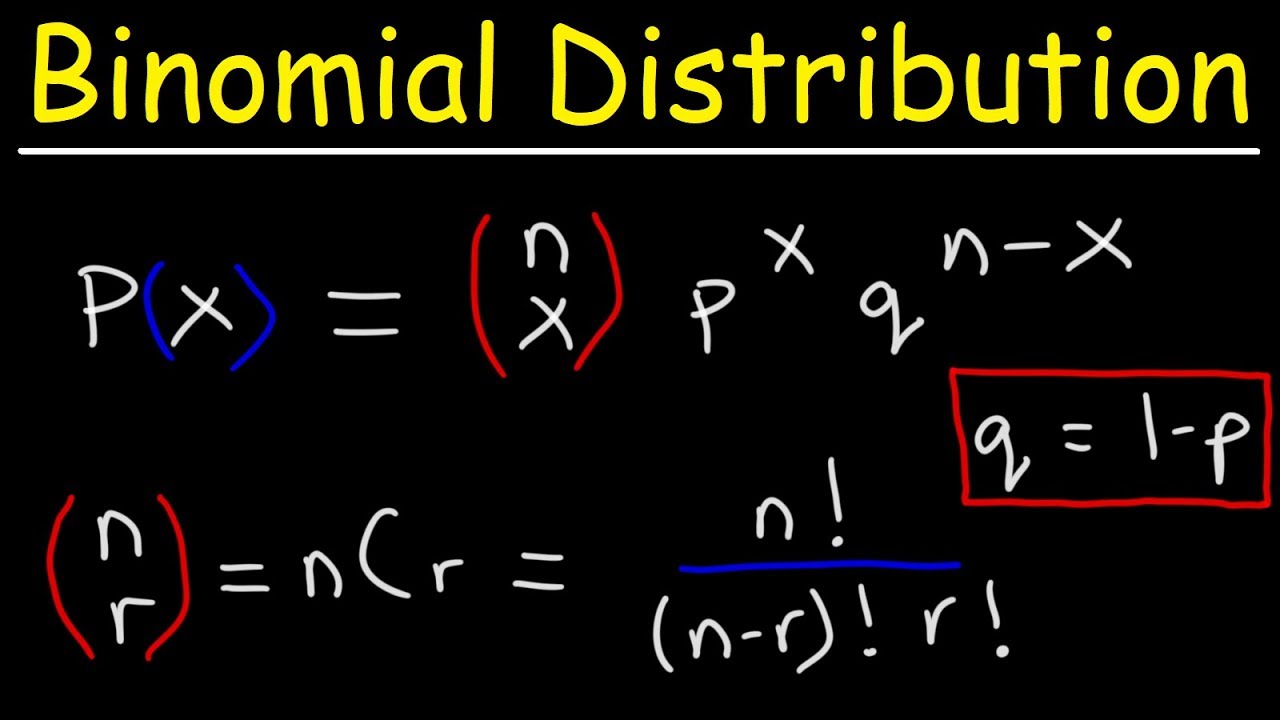
  • 📈 The binomial coefficient tells you the number of ways a certain ratio of successes to failures can occur.
  • 🧮 The binomial distribution formula combines the binomial coefficient and the individual probability of success/failure.
  • 📊 Understanding binomial probabilities helps model things like disease spread during a pandemic.
  • 😮 A special case of the binomial distribution for one trial is the Bernoulli distribution.
  • 🔢 Multiple Bernoulli trials together form a binomial distribution.
  • 🤔 Small individual probabilities can multiply to a high chance over many trials.
  • 🤯 Thinking probabilistically helps make sense of many connected events.
  • 🧠 Applying probability helps make practical predictions and decisions.
Q & A

  • What is the purpose of using mathematical shortcuts in probability?

    -Mathematical shortcuts in probability help to simplify and quickly solve complex problems without spending too much time on calculations.

  • What is the probability of getting shocked by the toaster on any given day?

    -The probability of getting shocked by the toaster on any given day is 20%.

  • How many different ways can you receive exactly one shock in a week?

    -There are five different ways to receive exactly one shock in a week, corresponding to each weekday.

  • What formula is introduced to simplify the calculation of probabilities?

    -The Binomial Distribution formula is introduced to simplify the calculation of probabilities.

  • What does the Binomial Coefficient Formula help to determine?

    -The Binomial Coefficient Formula helps to determine the number of ways a certain ratio of successes to failures can occur.

  • What is the probability of getting shocked only once by the toaster in a week?

    -The probability of getting shocked only once by the toaster in a week is approximately 41%.

  • How can the Binomial Distribution formula be applied to predict the gender distribution in a family with 5 children?

    -By plugging in the appropriate values into the Binomial Distribution formula, one can find out the probability of having 3 girls out of 5 children, which is about 31%.

  • What is the chance that none of the 20 people encountered during a zombie apocalypse are infected?

    -There is about a 36% chance that none of the 20 people encountered will be infected with the zombie virus.

  • What does the Bernoulli Distribution represent?

    -The Bernoulli Distribution represents the probability of getting either a success or a failure in a single trial.

  • What is the expected number of zombie infections in a group of 20 people, given a 5% infection rate?

    -The expected number of zombie infections in a group of 20 people, given a 5% infection rate, is 1.

Outlines
00:00
😊 Introducing the Binomial Distribution Formula

Paragraph 1 introduces the binomial distribution formula as a mathematical shortcut to calculate probabilities for repeated events, like the probability of getting shocked once by a faulty toaster in 5 days. It walks through an example toast shocking scenario to demonstrate using the multiplication rule to calculate probabilities.

05:01
😀 Deriving the Full Binomial Distribution Formula

Paragraph 2 derives the full binomial distribution formula by combining the binomial coefficient formula and probability exponents. It shows how the formula can calculate the probability of different numbers of events, like the chance of having 3 girls out of 5 children.

10:04
🧟 Applying the Formula to Zombie Apocalypse Scenarios

Paragraph 3 applies the binomial distribution formula to zombie apocalypse scenarios to determine the probability of encountering different numbers of zombies. It also discusses the Bernoulli distribution as a special case and uses it to calculate the probability of surviving a zombie blood transfusion.

Mindmap
Keywords
💡probability
Probability refers to the likelihood of an event occurring. It is a core concept in statistics that is used to predict future events. In the video, probability is mentioned in the context of predicting everyday events, like the probability of getting shocked by a toaster, or the probability of encountering zombies during a zombie apocalypse.
💡multiplication rule
The multiplication rule of probability states that the probability of multiple independent events all occurring is equal to the product of their individual probabilities. This rule is used in the video to calculate the probability of only getting shocked by the toaster once in a week.
💡binomial distribution
The binomial distribution gives the probability of a certain number of successes or failures in repeated trials. It is used in the video to calculate probabilities like the chance of having a certain number of boys or girls in multiple births, or the chance of encountering different numbers of zombies.
💡mean
The mean, or average, gives a central value summary of a distribution. The video uses the formula for the mean of a binomial distribution to estimate the average number of zombies expected in an encounter.
💡bernoulli distribution
The Bernoulli distribution gives the probability of a single trial having two possible outcomes, like success or failure. It is used in the video in the context of blood transfusions and latent zombie infections.
💡odds
Odds refer to the ratio of probabilities of an event occurring vs. not occurring. The video talks about odds in the context of the probability of getting a flat tire over a period of years.
💡discrete distribution
A discrete distribution shows the probabilities for distinct, countable values. The video mentions discrete distributions in the context of modeling the number of zombie encounters out of a fixed number of people.
💡factorials
Factorials are used in the binomial coefficient formula to count combinations. They are mentioned when deriving the full binomial distribution formula.
💡combinatorics
Combinatorics is the mathematics of counting combinations and permutations. The binomial coefficient formula comes from combinatorics and is used to count combinations in the binomial distribution.
💡pandemic
A pandemic refers to a disease outbreak affecting large populations across wide geographic areas. The video mentions using binomial probability calculations to model the spread of pandemic viruses.
Highlights

The speaker discusses using neural networks for natural language processing tasks.

Word embeddings are introduced as a way to capture semantic meaning and relationships between words.

Different embedding techniques like Word2Vec, GloVe, and BERT are explained.

Transformers are presented as a novel neural network architecture for NLP.

Attention mechanisms in transformers are highlighted as the key innovation.

BERT is discussed as a bidirectional transformer model that achieves state-of-the-art results.

Fine-tuning BERT for specific NLP tasks is explained as an effective transfer learning approach.

Data augmentation techniques like backtranslation are suggested to increase training data.

The speaker proposes ideas for multilingual and multimodal NLP using BERT.

Challenges like computational cost and model interpretability are acknowledged.

Future directions include generative transformers and reinforcement learning.

NLP benchmarks like GLUE and SQuAD are discussed for model evaluation.

The speaker concludes by emphasizing responsible and ethical AI development.

Questions from the audience highlight key points and lead to engaging discussion.

The speaker provides insightful responses and elaborates on earlier topics.

Transcripts

Browse More Related Video

Finding The Probability of a Binomial Distribution Plus Mean & Standard Deviation

Geometric Distributions and The Birthday Paradox: Crash Course Statistics #16

Finding Probabilities For A Binomial Distribution In StatCru

Binomial Distribution EXPLAINED in UNDER 15 MINUTES!

Math 119 Chapter 5 part 2

Binomial Probability Using the TI-84

Rate This

5.0 / 5 (0 votes)

Thanks for rating: