The Power Rule For Derivatives
TLDRThis video script offers a comprehensive guide to understanding the power rule for derivatives, a fundamental concept in calculus. It explains how to find the first derivative of a function by raising a variable to a constant power, using the formula n times x to the power of n minus one. The script provides clear examples, from differentiating monomials to handling rational and radical functions, emphasizing the importance of practice to master the topic. It also demonstrates how the power rule can be applied to derive the constant rule and simplification of expressions with negative exponents.
Takeaways
- 📈 The power rule for derivatives states that the derivative of a function f(x) = x^n, where x is the variable and n is a constant, is given by f'(x) = n * x^(n-1).
- 🌟 For example, if f(x) = x^2, the first derivative is 2x, demonstrating the power rule by taking the constant (2) and placing it in front, and adjusting the exponent (2-1).
- 🔢 If f(x) = x^3, applying the power rule gives f'(x) = 3x^2, with n being 3 in this instance.
- 🌌 When differentiating monomials using the power rule, the derivative of x^4 is 4x^3, and for x^5, it's 5x^4, following the same pattern of adjusting the exponent.
- 👉 The derivative of x (first power) is 1, as any number raised to the zero power is 1, and thus 1 times x to the power (1-1) which is 0, results in 1.
- 🚫 The derivative of a constant is zero, which can be shown using the power rule by rewriting the constant as the variable to the zero power and applying the rule.
- 📝 When differentiating rational functions like 1/x, the power rule is applied after rewriting the expression by moving the variable to the numerator with a negative exponent.
- 🌠 The derivative of 1/x^2 using the power rule is -2/x^3, after adjusting the exponent and rewriting the expression to have a positive exponent for the variable in the denominator.
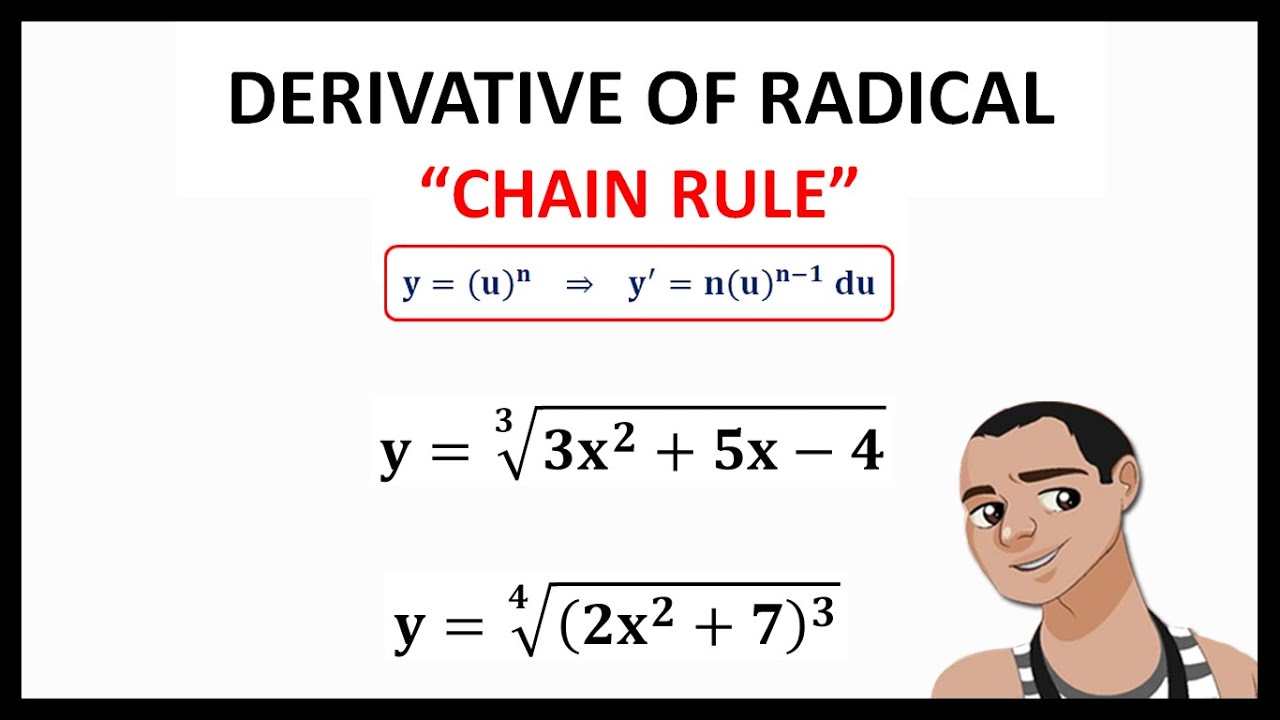
- 🌈 For radical functions, such as the square root of x (x^(1/2)), the power rule is applied by converting the radical to an exponent form (numerator one, denominator two) and then differentiating.
- 🔁 The process of differentiating more complex functions involves applying the power rule to each term, considering the properties of exponents, and simplifying the result as necessary.
Q & A
What is the power rule for derivatives and how is it applied?
-The power rule for derivatives states that if you have a function f(x) = x^n, where x is the variable and n is the constant, the first derivative of that function is given by f'(x) = n * x^(n-1). It is applied by moving the constant n to the front and multiplying it by the variable x raised to the power of (n-1).
What is the first derivative of f(x) = x^2 using the power rule?
-The first derivative of f(x) = x^2 using the power rule is f'(x) = 2x, since n is 2 and you replace it in the power rule formula to get 2 * x^(2-1).
How does the power rule apply to the function f(x) = x^3?
-For the function f(x) = x^3, the power rule gives us the first derivative as f'(x) = 3x^2. Here, n is 3, so we use the power rule formula resulting in 3 * x^(3-1).
What is the derivative of x to the fourth power according to the power rule?
-The derivative of x to the fourth power, or x^4, is 4x^3. Using the power rule with n being 4, we get 4 * x^(4-1), which simplifies to 4x^3.
What is the derivative of a constant using the power rule?
-The derivative of any constant using the power rule is zero. This is because if you have a constant, like 4, it can be written as 4x^0, and when you apply the power rule with n being 0, the result is the constant multiplied by 0x^(-1), which is just 0.
How does the power rule help differentiate rational functions?
-The power rule helps differentiate rational functions by first rewriting the expression so that the variable is in the numerator. For example, to differentiate 1/x, you rewrite it as x^(-1) and then apply the power rule, which results in -1/x^(-1 - 1) or -1/x^2 after simplifying.
What is the first derivative of f(x) = 1/x^2 using the power rule?
-The first derivative of f(x) = 1/x^2 using the power rule is f'(x) = -2/x^3. Here, after rewriting the function as x^(-2), we apply the power rule with n being -2, which gives us -2 * x^(-2 - 1), simplifying to -2/x^3.
How do you differentiate a radical function like the square root of x using the power rule?
-To differentiate a radical function like the square root of x, you first rewrite it in exponential form as x^(1/2). Then you apply the power rule with n being 1/2, which results in (1/2) * x^((1/2) - 1) or 1/(2*sqrt(x)) after finding a common denominator and simplifying.
What happens to the exponent when you move a variable from the denominator to the numerator in a rational function?
-When you move a variable from the denominator to the numerator in a rational function, the sign of the exponent changes. For example, if you have 1/x^n, when you move x^n to the numerator, it becomes x^(-n).
What is the derivative of x to the fifth power using the power rule?
-The derivative of x to the fifth power, or x^5, is 5x^4. By applying the power rule with n being 5, we calculate 5 * x^(5-1), which simplifies to 5x^4.
How do you find the derivative of a function with an exponent that is a fraction?
-To find the derivative of a function with an exponent that is a fraction, like x^(3/4), you first express the exponent as a fraction. Then you apply the power rule, which results in the fraction multiplied by x^((3/4) - 1). After finding a common denominator and simplifying, you get the derivative in a form that is easier to work with.
Outlines
📚 Introduction to the Power Rule for Derivatives
This paragraph introduces the power rule for derivatives, a fundamental concept in calculus. It explains that if we have a function f(x) = x^n, where x is the variable and n is a constant, the first derivative of this function is given by the power rule as n * x^(n-1). The explanation is illustrated with examples, such as f(x) = x^2, where the derivative is 2x, and f(x) = x^3, resulting in a derivative of 3x^2. The paragraph emphasizes the importance of understanding the power rule to master differentiation of monomials and provides several examples for practice, including derivatives of higher powers and the derivative of x itself, which is 1. It also touches on the derivative of constants, showing that the derivative of any constant is zero using the power rule.
📝 Differentiating Rational Functions Using the Power Rule
This paragraph delves into the application of the power rule for differentiating rational functions, which are fractions involving the variable x. The first step is to rewrite the expression by moving the x term to the numerator, which changes the sign of the exponent. The power rule is then applied to the new expression. For example, differentiating 1/x becomes -1 * x^(-2), which simplifies to -1/x^2. Further examples are provided, such as differentiating 1/x^2 to yield -1/x^3, demonstrating the process of simplifying the result by moving the x term back to the denominator. The paragraph also explains how to differentiate radical functions by converting them into exponent form and applying the power rule accordingly.
🧮 Derivatives of Radical Functions and Higher Roots
The final paragraph focuses on the differentiation of radical functions, such as the square root of x. It explains that the square root symbol is equivalent to 1/2 exponent, and higher roots can be expressed as fractional exponents (e.g., cube root as 1/3, fourth root as 1/4). The power rule is applied to these fractional exponents to find derivatives. For instance, the derivative of the square root of x (x^(1/2)) is calculated as (1/2) * x^(-1/2), which simplifies to 1/(2*sqrt(x)). The process of finding a common denominator and rewriting the expression as a single fraction is also discussed. The paragraph emphasizes the importance of understanding how to convert radical expressions into exponent form to apply the power rule effectively.
Mindmap
Keywords
💡Power Rule
💡Derivative
💡Constant
💡Rational Function
💡Exponent
💡Variable
💡Rate of Change
💡Monomial
💡Simplify
💡Radical Function
💡Differentiate
Highlights
The power rule for derivatives is introduced, which is a fundamental concept in calculus.
The power rule states that the derivative of a function f(x) = x^n, where n is a constant, is f'(x) = n * x^(n-1).
A practical example is given to demonstrate the power rule: the derivative of f(x) = x^2 is 2x.
Another example shows that the derivative of f(x) = x^3 is 3x^2 using the power rule.
The video emphasizes the importance of mastering the power rule through numerous examples.
The derivative of x^4 is calculated as 4x^3, showcasing the power rule's application for higher powers.
The derivative of x^5 is given as 5x^4, further illustrating the power rule for higher exponents.
The derivative of x^6 is explained to be 6x^5, continuing the pattern for higher powers.
The derivative of x is determined to be 1, applying the power rule with n as 1.
The power rule is also used to explain that the derivative of any constant is zero.
Differentiating rational functions is discussed, specifically how to handle 1/x using the power rule.
The derivative of 1/x is shown to be -1/x^2 after applying the power rule and simplifying.
The process for differentiating 1/x^2 using the power rule is demonstrated, resulting in -2/x^3.
The differentiation of 1/x^4 is explained, leading to the result of -4/x^5.
The concept of radical functions and their derivatives are introduced, starting with the square root of x.
The derivative of the square root of x is calculated as 1/(2*sqrt(x)), using the power rule for radicals.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: