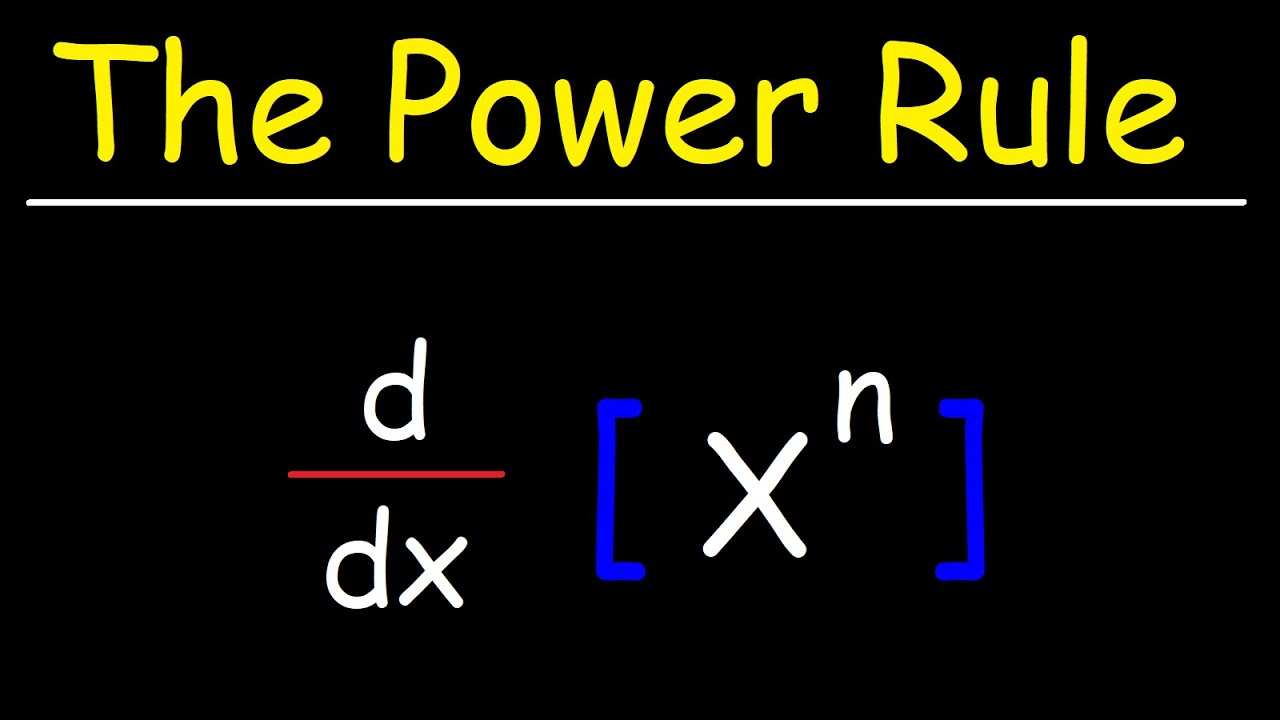Derivatives of Rational Functions

The Organic Chemistry Tutor
25 Feb 201811:52
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a comprehensive guide on differentiating rational functions, focusing on the application of the power rule, chain rule, and quotient rule. The lesson begins with the derivation of simpler functions like 1/x and progresses to more complex expressions involving sums and differences within the numerator and denominator. Through a series of worked examples, the script methodically demonstrates how to manipulate and simplify expressions to find their derivatives, ultimately aiming to solidify the viewer's understanding and provide ample practice to master the topic.

Takeaways
  • 📚 The process of differentiating a rational function involves using the power rule for expressions like 1/x, which is rewritten as x^(-1) and then applying the power rule to find the derivative.
  • 🔄 When differentiating rational functions, it's important to rewrite the function in a way that allows for the application of the power rule and chain rule, if necessary.
  • 🌟 The derivative of 1/x is found by applying the power rule, resulting in -1/x^2.
  • 🔢 For rational functions with a sum in the denominator, like 1/(x+5), the power and chain rules are used, leading to the derivative of -1/(x+5)^2.
  • 📈 When dealing with more complex rational functions, such as 1/(4x-7), the chain rule and power rule are combined, resulting in a derivative of -4/(4x-7)^2.
  • 🌐 For rational functions with a polynomial in the denominator, like 9/(x^2-6), the derivative involves multiplying the numerator by the derivative of the denominator and vice versa, simplifying to -18x/(x^2-6).
  • 🛠️ The quotient rule is essential for differentiating complex rational functions where the numerator and denominator both contain variables, expressed as (g*f' - f*g') / g^2.
  • 🔧 When applying the quotient rule, it's necessary to find the derivatives of both the numerator (f') and the denominator (g'), and then apply the rule to find the derivative of the entire function.
  • 📊 The process of simplifying derivatives of rational functions often involves combining like terms and reducing expressions to their simplest form for clarity and understanding.
  • 📚 Practice is key to mastering the differentiation of rational functions, as demonstrated by the numerous examples provided in the script.
Q & A

  • What is the main topic of the lesson?

    -The main topic of the lesson is how to find the derivative of a rational function.

  • What is the derivative of 1/x?

    -The derivative of 1/x is -1/(x^2).

  • How does the power rule apply in this context?

    -The power rule applies by taking the exponent of the function, moving it to the front, and then subtracting one from the exponent to find the derivative of the function.

  • What is the role of the chain rule in differentiating rational functions?

    -The chain rule is used when differentiating a function that is a composite of other functions, allowing us to take the derivative of the outer function and multiply it by the derivative of the inner function.

  • How do you differentiate 1/(x+5) using the power and chain rules?

    -You first rewrite the expression as (x+5)^(-1), then apply the power rule to get -1 * (x+5)^(-2), and finally find the derivative of the inside function, which is 1, to get the final answer of -1/(x+5)^2.

  • What is the derivative of 1/(4x - 7)?

    -The derivative of 1/(4x - 7) is -4/((4x - 7)^2).

  • How do you find the derivative of a complex rational function like 9/(x^2 - 6)?

    -You apply the power rule to the numerator and the chain rule to the denominator, then combine the results to get the derivative as -18x/((x^2 - 6)^2).

  • What is the quotient rule and when is it used?

    -The quotient rule is used when differentiating a quotient of two functions. It states that the derivative of f/g is (g*f' - f*g')/g^2.

  • How do you differentiate the expression (5 - 9x)/(x^2 + 4)?

    -You apply the quotient rule, finding the derivatives of the numerator and the denominator separately, and then use the formula (g*f' - f*g')/g^2 to get the derivative as (9x^2 - 10x - 36)/(x^2 + 4)^2.

  • What is the final expression's derivative when (5 - 2x) is over (x + 4)^5?

    -The derivative is -65*(5 - 2x)^4/(x + 4)^6.

  • What is the significance of simplifying the final answer in differentiation problems?

    -Simplifying the final answer makes it easier to understand and interpret, and it can also help in further calculations or analysis involving the derivative.

Outlines
00:00
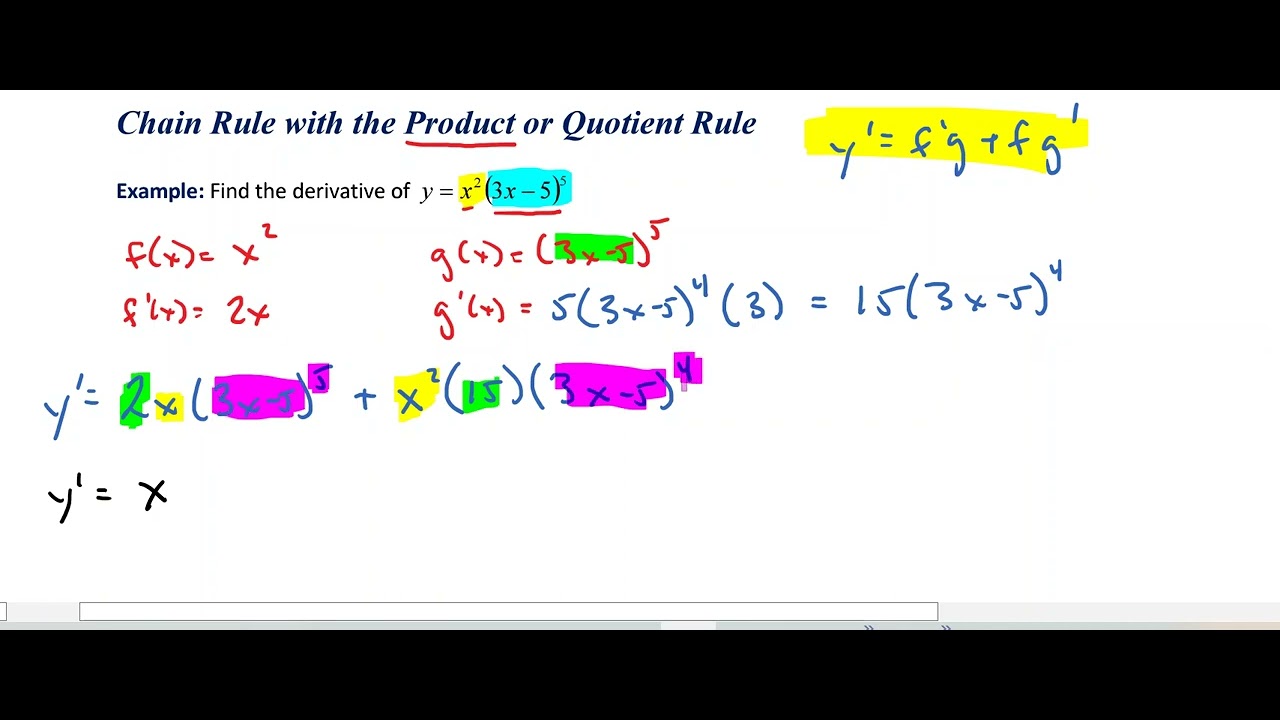
📚 Introduction to Differentiating Rational Functions

This paragraph introduces the concept of finding the derivative of a rational function. It begins with the differentiation of the function 1/x, explaining the process of rewriting the expression as x to the power of negative one and applying the power rule. The explanation continues with the application of the power rule and chain rule for more complex examples, such as differentiating 1/(x+5) and 1/(4x-7). The paragraph emphasizes the importance of understanding the power rule and chain rule in solving these types of derivatives, and it encourages practice to master the topic.

05:00
🧮 Applying the Quotient Rule for Complex Rational Functions

This paragraph delves into the application of the quotient rule for differentiating more complex rational functions. It starts by rewriting the expression 9/(x^2 - 6) and applying the quotient rule, which involves multiplying the derivative of the numerator by the denominator and subtracting the product of the derivative of the denominator and the numerator, all divided by the square of the denominator. The paragraph then moves on to another example, 12/(4-8x)^4, and demonstrates the process of applying the quotient rule, including the use of the chain rule to find the derivative of the inner function. The explanation is detailed, walking through each step of the differentiation process.

10:03
🔢 Solving Compound Rational Functions using Quotient and Chain Rules

The focus of this paragraph is on solving compound rational functions using a combination of the quotient rule and chain rule. It begins with the differentiation of the function (5-9x)/(x^2+4), explaining the application of the quotient rule when the numerator and denominator both contain the variable. The paragraph then tackles the function (5-2x)/(x+4)^5, showcasing how to apply the chain rule to the inner function before using the quotient rule. The explanation is thorough, highlighting the steps of distributing and combining like terms, and simplifying the final expression. The paragraph concludes with a comprehensive summary of the process, reinforcing the methods used to find the derivatives of compound rational functions.

Mindmap
Keywords
💡derivative
The derivative is a fundamental concept in calculus that represents the rate of change of a function at a particular point. It is used to analyze the behavior of functions, such as determining the slope of a curve at a given point. In the video, the derivative is calculated for various rational functions, illustrating the process of differentiation using different rules like the power rule and the quotient rule.
💡rational function
A rational function is a function that is expressed as the quotient of two polynomial functions. These functions are fundamental in calculus and have various applications in mathematics, physics, and engineering. The video focuses on finding the derivatives of rational functions, which involves using specific rules like the power rule and the chain rule.
💡power rule
The power rule is a basic differentiation rule that states if y = x^n, where n is a constant, then the derivative dy/dx = n * x^(n-1). This rule is used to find the derivative of functions that involve variables raised to constant powers. In the video, the power rule is applied to expressions like x^(-1) and (x+5)^(-1) to find their derivatives.
💡chain rule
The chain rule is a fundamental rule in calculus used to find the derivative of a composite function, which is a function made up of other functions. The chain rule states that the derivative of a composite function is the derivative of the outer function times the derivative of the inner function. In the video, the chain rule is applied to expressions like (4x - 7)^(-1) and (x + 4)^5 to calculate their derivatives.
💡quotient rule
The quotient rule is a calculus rule used to find the derivative of a quotient of two functions. It states that if you have a function f(x)/g(x), then the derivative is [g(f') - f(g')] / g^2, where f' and g' are the derivatives of f and g, respectively. The video demonstrates the quotient rule by differentiating complex rational functions like (5 - 9x) / (x^2 + 4).
💡differentiation
Differentiation is the process of finding the derivative of a function, which helps in understanding the function's behavior, such as its rate of change or slope at any point. It is a core operation in calculus with wide applications in various fields. The video is centered around the concept of differentiation, teaching viewers how to differentiate rational functions using different rules.
💡function
In mathematics, a function is a relation that pairs each element from a set (called the domain) with exactly one element from another set (called the range). Functions are essential in calculus as they represent relationships between variables and can be analyzed using derivatives. The video discusses functions in the context of differentiation, focusing on rational functions.
💡slope
Slope is a measure of the steepness of a straight line, and in the context of functions, it represents the rate of change of the function's value with respect to its independent variable. The derivative of a function at a specific point gives the slope of the tangent line to the graph of the function at that point. The video discusses the concept of slope while explaining the derivative of functions.
💡composite function
A composite function is a function that is formed by combining two or more functions in such a way that the output of one function becomes the input of another. The chain rule is used to differentiate composite functions. In the video, composite functions are discussed in the context of the chain rule, where the derivative of the outer function is applied to the result of the inner function.
💡exponent
An exponent is a mathematical notation that indicates the number of times a base is multiplied by itself. Exponents are used in expressions to represent powers or multiples of the base. In the context of the video, the power rule is used to differentiate functions with variables raised to exponents, such as x^n.
💡constant
A constant is a value that does not change. In the context of calculus and functions, constants do not affect the derivative of a function as their rate of change is zero. The derivative of a constant is always zero, which is a basic concept used when differentiating functions that include constant terms, such as in the expression 9 / (x^2 - 6).
Highlights

Introduction to finding the derivative of rational functions

Differentiating 1/x using the power rule

Derivative of 1/x is -1/x^2

Differentiating 1/(x+5) with power and chain rule

Derivative of 1/(x+5) is -1/(x+5)^2

Differentiating 1/(4x-7) using chain and power rules

Derivative of 1/(4x-7) is -4/(4x-7)^2

Differentiating 9/(x^2-6) using power rule

Derivative of 9/(x^2-6) is -18x/(x^2-6)^2

Differentiating 12/(4-8x^4) using power rule

Derivative of 12/(4-8x^4) is 384/(4-8x^4)^5

Differentiating (5-9x)/(x^2+4) using quotient rule

Derivative of (5-9x)/(x^2+4) is (9x^2-10x-36)/(x^2+4)^2

Differentiating (5-2x)/(x^5+4^5) with quotient and chain rules

Derivative of (5-2x)/(x^5+4^5) is (5-2x)(-13)/(x+4)^6

Innovative method of applying both quotient and chain rules for complex rational functions

Practical applications of derivatives in understanding the behavior of functions

Theoretical contribution to the understanding of rational functions and their derivatives

Engagement of learners through practice problems to master the concept of derivatives

Transcripts

Browse More Related Video

The Chain Rule - Part 2

The Power Rule For Derivatives

Math 11 - Section 2.3

The Constant Multiple Rule For Derivatives

Calculus AB Lesson 3.4 The Chain Rule

The Chain Rule

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusDerivativesRational FunctionsMath TutorialPower RuleChain RuleEducational ContentMathematics EducationOnline LearningMath Practice