AP Physics Workbook 4.L The Sign of Work
TLDRThis physics tutorial delves into the concepts of work and energy in relation to motion. It presents a scenario with two identical blocks on a track, one ascending and the other descending, and predicts which block will travel further based on energy transformation. The analysis involves the work equation and Newton's second law, considering factors like friction and gravitational force. The experiment's data supports the prediction, and the equations derived for the distances traveled by the blocks on the track are explained. The conclusion highlights the inverse relationship between net force and the distance traveled.
Takeaways
- 📚 The tutorial covers Unit 4 on Work and Energy, focusing on the concept of work and its sign in the context of physics.
- 🔧 The scenario involves identical blocks on a track with familiar frictions, where one block goes up and the other goes down, illustrating the effects of work on their motion.
- 🏎️ Block one comes to a stop after traveling a length l1, while block two stops after traveling l2, prompting a prediction on which block would travel further based on energy transformation.
- ⚙️ The prediction is that block two (going down) would travel further due to having more kinetic energy, as gravity aids its motion down the ramp.
- 🎯 The experiment's data supports the prediction, with block two traveling a greater distance, especially after removing an outlier from the third trial.
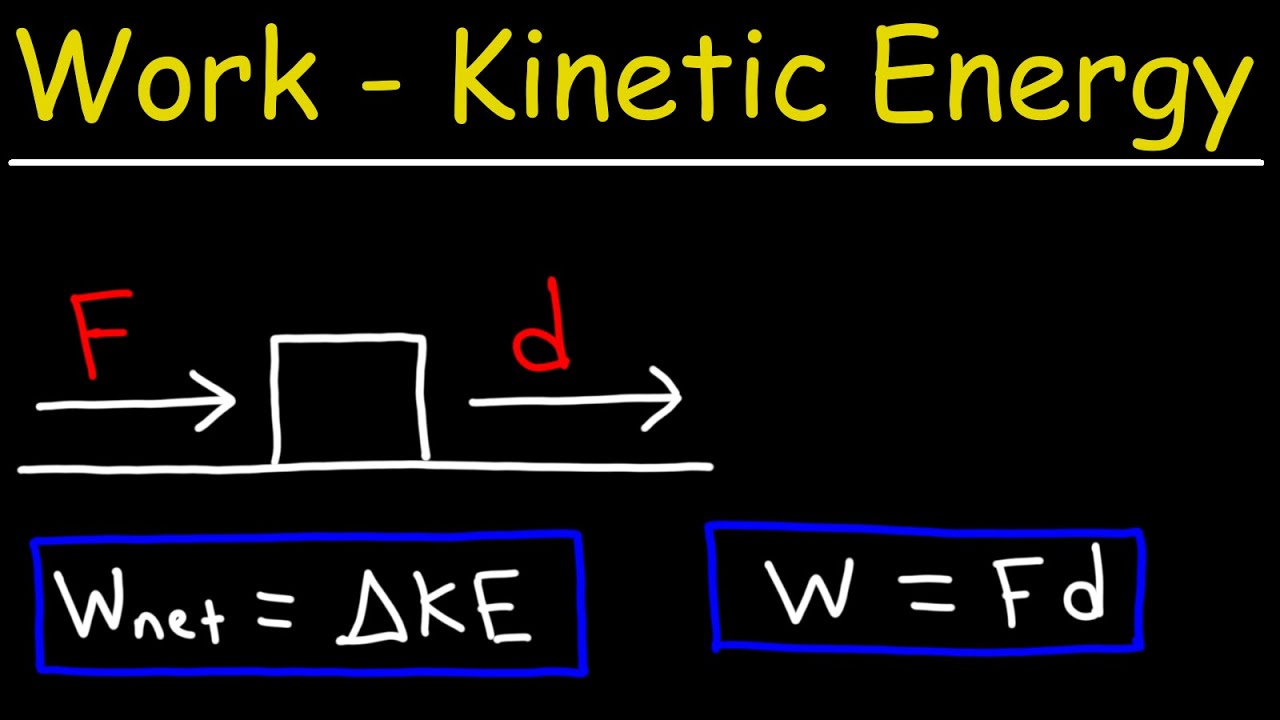
- 📝 The work equation is used to derive equations for l1 and l2, with the understanding that work is equal to the change in kinetic energy.
- 👎 The initial reasoning provided in the script contains a mistake, as the relationship between net force and distance traveled is inverse, not direct.
- 📉 The net force on the blocks is a combination of gravitational force components and friction, with friction always acting in the opposite direction of motion.
- 🔄 The force of gravity assists block two (going down) by adding energy, while it opposes block one (going up), leading to different distances traveled.
- 🧠 The understanding of the problem improves as the tutorial progresses, with a realization that the initial reasoning was incorrect and a re-evaluation of the net force's effect on the blocks' motion.
- 📈 The final takeaway is that the block going down the ramp (l2) will indeed travel further due to the net force being smaller, as it is affected by the gravitational force's component in the direction of motion.
Q & A
What is the main topic of the physics workbook tutorial?
-The main topic of the tutorial is Unit 4 - Work and Energy, specifically focusing on the concept of work and its sign in the context of friction and motion on an inclined track.
What is the scenario presented in the tutorial?
-The scenario involves two identical blocks with familiar frictions, one moving up an inclined track and the other moving down, both starting with an initial speed and coming to rest after traveling a certain distance.
What is the prediction made by the student in the tutorial?
-The student predicts that Block 2, which is moving down the track, would travel further before coming to rest because it would have more kinetic energy due to the assistance of gravity.
How does the student apply the work-energy principle to explain the motion of the blocks?
-The student uses the work-energy principle, stating that work is equal to the force times distance (W = Fd) and also equal to the change in kinetic energy. They set up an equation comparing the initial and final kinetic energies to predict the motion of the blocks.
What does the student assume about the initial kinetic and potential energy of the blocks?
-The student assumes that the initial kinetic and potential energies are zero for both blocks, allowing them to cancel out these terms when comparing the motion of Block 1 and Block 2.
What does the experimental data show in relation to the student's prediction?
-The experimental data, after removing an outlier, supports the student's prediction that Block 2 travels a greater distance (l2 > l1) due to having a larger net force acting on it, which is facilitated by gravity.
How does the student derive equations for l1 and l2?
-The student starts with the work equation, equating work to the change in kinetic energy, and applies Newton's second law to determine the net force acting on the blocks. They then solve for the distance traveled (l1 and l2) by considering the forces of gravity and friction and their components along the inclined track.
What is the significance of the angle in the work equation?
-The angle in the work equation accounts for the direction of the force and displacement on the inclined track. The cosine of the angle is used to find the component of the force and displacement that is parallel to the track.
How does the student's analysis of the force of friction differ for Block 1 and Block 2?
-For Block 1, moving up the track, the force of friction acts downward, opposing the motion. For Block 2, moving down, the force of gravity assists the motion, and the force of friction acts in the opposite direction to the sine of the angle, reducing the net force acting on the block.
What is the relationship between the net force and the distance traveled by the blocks?
-The distance traveled by the blocks has an inverse relationship with the net force. A larger net force results in a smaller distance traveled, and a smaller net force results in a larger distance traveled, as the net force determines the acceleration and deceleration of the blocks.
What correction does the student make to their initial reasoning?
-The student corrects their initial reasoning by acknowledging that the net force is not simply the force of gravity but is a result of the force of gravity's components (cosine and sine of the angle) minus the force of friction. This correction aligns their analysis with the experimental data.
Outlines
📘 Introduction to Work and Energy Concepts
This paragraph introduces the topic of work and energy in the context of a physics workbook tutorial. It sets up a scenario involving two identical blocks on a track with friction, where block one moves up while block two moves down. The challenge is to predict which block will travel further before coming to rest, using the principles of energy transformation. The explanation relies on the concept that work is equal to force times distance, and the change in kinetic energy is considered in the analysis. The initial assumption is that both blocks start with zero initial kinetic and potential energy, allowing for a comparison based on the force of gravity and the track's orientation.
🧠 Analysis of Experimental Data and Prediction Confirmation
In this paragraph, the presenter examines experimental data that supports the initial prediction made in the first part of the tutorial. The data shows that block 2 (l2) travels a greater distance (l2) than block 1 (l1), which aligns with the prediction. An outlier in the third trial is identified and removed to confirm the consistency of the results. The presenter then proceeds to derive equations for l1 and l2, starting with the work equation and applying Newton's second law to determine the net force acting on the blocks. The analysis involves breaking down the force of gravity into its horizontal and vertical components and considering the angle of the ramp.
📚 Derivation of Equations for l1 and l2
The presenter continues the derivation of equations for l1 and l2, focusing on the net force acting on the blocks as they move along the ramp. The force of friction is calculated, and the equation for l1 is simplified by canceling out common terms. The process for l2 is similar but with a crucial difference in the sign of the sine theta term, reflecting the direction of movement (up or down the ramp). The presenter then connects the derived equations back to the experimental data, noting that the average distance traveled by l2 is greater than l1, which is consistent with the equations. However, the presenter realizes and corrects a mistake in the reasoning, emphasizing that the net force's effect on the distance traveled is inversely proportional.
🎓 Conclusion and Clarification of Net Force
The final paragraph concludes the tutorial by clarifying the relationship between the net force and the distance traveled by the blocks. The presenter corrects the previous mistake, emphasizing that the net force is not simply the force of gravity but rather a combination of mu cosine theta minus sine theta. The clarification highlights that l2 travels a greater distance when the net force is smaller, confirming the experimental results. The tutorial ends with a reaffirmation of the principles of work and energy and their application in understanding the motion of objects on inclined planes.
Mindmap
Keywords
💡Work and Energy
💡Friction
💡Kinetic Energy
💡Potential Energy
💡Net Force
💡Newton's Laws
💡Experiment
💡Data Analysis
💡Derive Equation
💡Outlier
💡Energy Transformation
Highlights
The tutorial covers Unit 4 on Work and Energy in physics, focusing on the concept of the sign of work.
A scenario is presented involving identical blocks on a track with familiar frictions, where one block goes up and the other goes down.
The initial speed (v naught) of the blocks is set, and they come to rest after traveling different distances (l1 and l2) on the track.
The prediction made is that Block 2 (l2) would travel further due to having more kinetic energy, based on the work equation (work equals force times distance).
The experiment's data supports the prediction, showing that l2 has a larger average travel distance than l1, especially after removing an outlier.
The derivation of an equation for l1 involves starting with the work equation and applying Newton's second law.
The force of friction is considered, which is always in the opposite direction of the velocity.
The force of gravity is broken down into its vertical and horizontal components to analyze the net force.
The equation for l1 is derived, showing that the distance traveled (l1) is inversely related to the net force.
The equation for l2 is also discussed, noting that the only difference is the sign in front of the sine theta term, reflecting the direction of travel.
The results from the experiment confirm the theoretical analysis, with l2 having a larger average than l1, which is explained by the derived equations.
The tutorial provides a clear demonstration of how work and energy principles apply to real-world scenarios, such as the motion of blocks on a track.
The process of deriving equations for physical phenomena is explained, showcasing the application of fundamental physics concepts.
The importance of considering the direction of forces and the impact of net force on the distance traveled is highlighted.
The tutorial emphasizes the value of experimental data in validating theoretical predictions and refining understanding.
The concept of the sign of work is explored, showing how it affects the calculation of work done and the resulting motion.
The tutorial concludes with a re-evaluation of the initial reasoning, demonstrating the iterative nature of scientific inquiry.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: