U-substitution With Definite Integrals

The Organic Chemistry Tutor
11 Mar 201811:02
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial demonstrates the technique of u-substitution for evaluating definite integrals. It walks through three examples, showing how to transform the integral expression by substituting variables and adjusting the limits of integration accordingly. The examples cover a range of functions, from polynomials to rational exponents, and illustrate the process of finding antiderivatives and computing the resulting values. The video is an informative guide for those looking to master u-substitution in calculus.

Takeaways
  • 📚 The video focuses on evaluating definite integrals using the method of u-substitution, which is a technique for integrating functions by substitution.
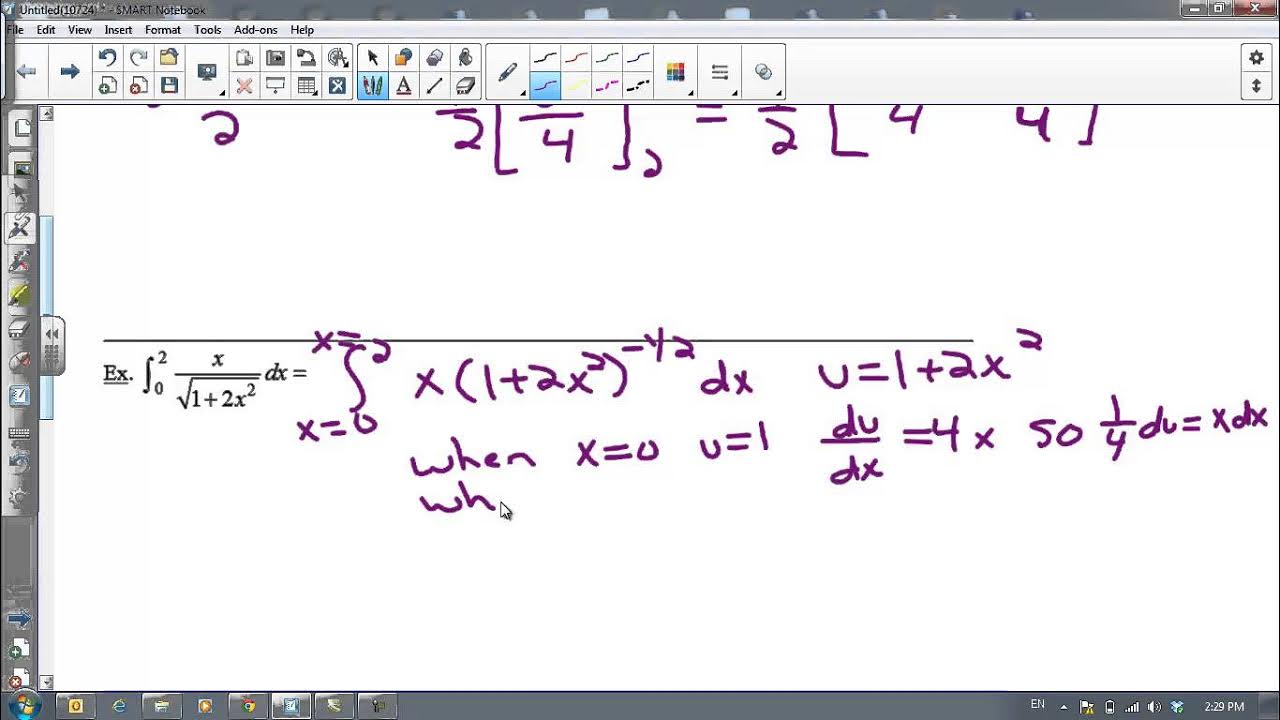
  • 🔄 The first example demonstrates how to evaluate the integral of 2x * (x^2 + 4)^2 from 0 to 2, using u as x^2 + 4, and du as 2x dx.
  • 🌟 The process involves isolating dx and replacing it with du/(2x), simplifying the integral to u^2 * du/(2x), and adjusting the limits of integration to u values.
  • 🎯 The lower limit u when x is 0 is 4, and the upper limit u when x is 2 is 8, leading to the evaluation of the integral as 448/3.
  • 📝 The second example involves the integral of √(16 - x^2) from 0 to 4, with u set as 16 - x^2 and du as -2x dx, after solving for dx in terms of du.
  • 🔢 The integral is then rewritten as 4x * √u du/(-2x), and the limits of integration are adjusted to u(16) = 16 and u(4) = 0, resulting in the final answer of 256/3.
  • 🧠 A third example is presented, evaluating the integral of (2x) / (1 + x^2)^3 from 1 to 2, with u as 1 + x^2 and du as 2x dx, leading to the integral in terms of 1/u^3.
  • 🔄 The limits of integration are u(1) = 2 and u(2) = 5, and the integral is evaluated as -1/(2u^2) from 2 to 5, with the final result being 21/200.
  • 📊 The video emphasizes the importance of changing the limits of integration to their corresponding u-values when using u-substitution.
  • 👓 It also highlights the need to simplify the resulting expressions and evaluate the antiderivative at the new limits to find the value of the integral.
  • 🔍 The process of u-substitution is shown to be a powerful tool for evaluating integrals, especially when the integrand contains a function that can be isolated and simplified.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is evaluating definite integrals using u-substitution.

  • How does u-substitution help in evaluating definite integrals?

    -U-substitution simplifies the process of evaluating definite integrals by transforming the integral into a form that is easier to work with, particularly when the integrand involves terms that can be expressed as derivatives of a single function.

  • What was the first example integral evaluated in the video?

    -The first example integral evaluated in the video was the definite integral of 2x * (x^2 + 4)^2 from 0 to 2.

  • What was the u-substitution used for the first example?

    -For the first example, u was set to x^2 + 4, which allowed the elimination of the 2x term in the integral.

  • How were the limits of integration adjusted for the first example after applying u-substitution?

    -The lower limit was changed from 0 to u when x is 0, which is 4, and the upper limit was changed from 2 to u when x is 2, which is 8 (since 2^2 + 4 = 8).

  • What was the final result of the first example integral?

    -The final result of the first example integral was 448/3.

  • What was the second integral evaluated in the video?

    -The second integral evaluated in the video was the definite integral of 4x * sqrt(16 - x^2) dx from 0 to 4.

  • What was the u-substitution used for the second example?

    -For the second example, u was set to 16 - x^2, and dx was expressed as du / (-2x).

  • What was the final result of the second example integral?

    -The final result of the second example integral was 256/3.

  • What was the third integral evaluated in the video?

    -The third integral evaluated in the video was the definite integral of 2x / (1 + x^2)^3 from 1 to 2.

  • How were the limits of integration adjusted for the third example after applying u-substitution?

    -The lower limit was changed from 1 to u when x is 1, which is 1 + 1^2 = 2, and the upper limit was changed from 2 to u when x is 2, which is 1 + 2^2 = 5.

  • What was the final result of the third example integral?

    -The final result of the third example integral was 21/200.

Outlines
00:00
📚 Evaluating Definite Integrals with u-Substitution

This paragraph introduces the concept of evaluating definite integrals using u-substitution. It begins with an example of finding the value of the integral of 2x(x^2 + 4)^2 from 0 to 2. The process involves setting u equal to x^2 + 4, which allows the removal of the 2x term. The integral is then transformed to u^2 du/(2x), and the limits of integration are adjusted accordingly. The antiderivative of u^2 is u^3/3, and by substituting the new limits, the final answer is calculated as 448/3. The paragraph emphasizes the importance of changing variables and adjusting limits when using u-substitution.

05:01
🔢 Solving a Second Integral Using u-Substitution

The second paragraph continues with u-substitution, focusing on a different integral. Here, u is set to 16 - x^2, and the derivative is calculated to find dx in terms of du. The integral is then rewritten in terms of u and evaluated from 16 to 0. The antiderivative of u^(1/2) is determined to be (3/2)u^(3/2), and the final calculation results in 256/3. The explanation includes the process of raising rational exponents and the method of simplifying the final fraction.

10:02
🧮 Evaluating a Third Integral with u-Substitution

The third paragraph presents another example of evaluating a definite integral using u-substitution. The integral involves the function 2x(1 + x^2)^3 from 1 to 2. By setting u to 1 + x^2 and solving for dx, the integral is transformed and the limits of integration are updated. The antiderivative of 1/u^3 is used, and the final calculation involves simplifying the expression by finding a common denominator and reducing the fractions to arrive at the answer, 21/200.

Mindmap
Keywords
💡Definite Integral
A definite integral represents the area under a curve of a function over a specified interval. In the video, the process of evaluating definite integrals is the main focus, with examples given to illustrate how to calculate these areas using different techniques, such as u-substitution.
💡U-Substitution
U-substitution is a technique used in calculus to simplify the process of integrating a function by substituting a new variable (u) for the original variable (x). This method is particularly useful when the integrand involves the original variable raised to a power or in a complex expression.
💡Antiderivative
An antiderivative is a function whose derivative is the integrand of a given integral. Finding the antiderivative is a crucial step in evaluating definite integrals, as it allows us to apply the Fundamental Theorem of Calculus to find the area under the curve.
💡Limits of Integration
The limits of integration are the boundaries of the interval over which the definite integral is calculated. These limits are essential in determining the extent of the area under the curve that we want to find.
💡Derivative
The derivative of a function is a measure of how the function changes with respect to its input variable. It is a fundamental concept in calculus and is used to find the expression for du/dx when performing u-substitution.
💡Integration
Integration is the process of finding the antiderivative of a function, which can be used to calculate areas, volumes, and other quantities that involve accumulation. It is the inverse operation of differentiation.
💡Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus connects differentiation and integration by stating that if a function is continuous over an interval, then the definite integral of the function over that interval can be found by evaluating its antiderivative at the interval's endpoints and taking the difference.
💡Substitution
Substitution is a general mathematical technique used to replace one expression with another in order to simplify a problem or to make it more manageable. In the context of integration, u-substitution is a specific type of substitution that replaces the variable of integration with a new variable.
💡Algebraic Manipulation
Algebraic manipulation involves the use of algebraic rules and operations to transform and simplify mathematical expressions. It is a key skill in solving mathematical problems, including those related to integration.
💡Area Under a Curve
The area under a curve of a function represents the quantity that a definite integral calculates. It is a visual and geometric interpretation of the integral, where the area is enclosed by the curve, the x-axis, and the interval's limits.
💡Calculus
Calculus is a branch of mathematics that deals with rates of change and accumulation. It consists of two main subfields: differential calculus, which deals with derivatives, and integral calculus, which deals with integrals.
Highlights

The video focuses on evaluating definite integrals using u-substitution, a fundamental technique in calculus.

The first example involves integrating 2x * (x^2 + 4)^2 from 0 to 2, showcasing the setup and application of u-substitution.

In u-substitution, the variable u is chosen to simplify the integral, here u is x^2 + 4 to eliminate the 2x term.

The derivative of u with respect to x, du/dx, is used to transform the differential dx, which becomes du/(2x).

The lower and upper limits of integration are adjusted according to the value of u at the bounds of the original integral.

The antiderivative of u^2 is u^3/3, and the limits are evaluated from 4 to 8 to find the integral's value.

The final answer for the first example is 448/3, demonstrating the power of substitution in simplifying complex integrals.

The second example involves the integral of 4x * sqrt(u) with du/(-2x), highlighting the process of changing the differential and the limits.

The problem of evaluating the integral of 2x(1 + x^2)^3 from 1 to 2 is presented, showcasing another application of u-substitution.

For the third example, u is 1 + x^2, and the derivative 2x is used to cancel out the 2x term in the integral.

The integral simplifies to 1/u^3, and the limits of u are 2 and 5, which are the u-values corresponding to x-values 1 and 2.

The antiderivative of u^(-3) is -1/(2u^2), and the limits are evaluated from 2 to 5 to find the integral's value.

The final answer for the second example is 256/3, illustrating the method's effectiveness in solving more complex integrals.

The third example's final answer is 21/200, demonstrating the method's utility in evaluating definite integrals with higher-degree polynomials.

Throughout the transcript, the importance of changing the limits of integration based on the u-variable is emphasized, which is crucial for accurate results.

The methodical approach to u-substitution, as described in the transcript, is a valuable tool for students and professionals in calculus and mathematical analysis.

Transcripts

Browse More Related Video

How To Integrate Using U-Substitution

Calculus AB/BC – 6.9 Integrating Using Substitution

Calculus 1 - Integration & Antiderivatives

How to Integrate Using U-Substitution (NancyPi)

U-Substitution with Definite Integrals

Indefinite Integral - Basic Integration Rules, Problems, Formulas, Trig Functions, Calculus

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsCalculusIntegrationu-SubstitutionDefinite IntegralsEducationalTutorialMath TechniquesProblem SolvingMathematics Education