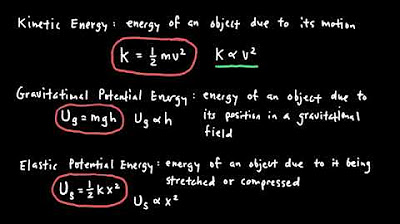Conservation of Energy Physics Problems
TLDRThe video script presents a series of physics problems involving conservation of energy and motion. It covers scenarios such as a block sliding down an incline, a block compressing a spring, and a roller coaster moving through different points. The script explains how to calculate the speed of objects at various stages, taking into account factors like gravitational potential energy, kinetic energy, and the work done by friction. The problems are solved using equations and principles from classical mechanics, illustrating the transformation of energy in different physical systems.
Takeaways
- 📚 The problem-solving approach involves the use of conservation of energy principles, specifically mechanical energy conservation.
- 🎢 In the inclined plane scenario, the block's potential energy at the top is converted into kinetic energy as it slides down.
- 🚀 The final speed of the block at the bottom of the incline can be calculated using the formula: √(2gh), where 'g' is the acceleration due to gravity and 'h' is the height of the incline.
- 🌐 For the spring-block system, the elastic potential energy stored in the compressed spring is converted into the block's kinetic energy upon release.
- 🔄 The speed of the block released from the spring can be found using the equation derived from energy conservation: (1/2)kx^2 = (1/2)mv^2, where 'k' is the spring constant and 'x' is the compression distance.
- 🏞️ In the case of a block sliding down a hill with initial speed, the final speed at the bottom is determined by adding the initial kinetic energy to the potential energy converted into kinetic energy.
- 🛤️ When friction is present, the work done by friction (negative work) reduces the final kinetic energy and thus the final speed of the object.
- 🚗 In a collision scenario where a block or a car comes to a complete stop, the initial kinetic energy is transformed into thermal energy.
- 🎢 For the roller coaster problem, the speed at different points can be found using conservation of energy, with the potential energy at the starting point converted into kinetic energy at subsequent points.
- 📈 The height reached by the roller coaster at a point with a given speed can be calculated by setting the initial potential energy equal to the sum of the potential energy at that point and the kinetic energy.
- 🔢 The problems solved in the script demonstrate the application of energy conservation in various scenarios, including inclined planes, springs, hills, and roller coasters, highlighting the transformation between potential and kinetic energy.
Q & A
What principle is used to solve the first problem of the block sliding down the inclined plane?
-The principle of conservation of energy is used to solve the problem, where the initial potential energy is converted into kinetic energy as the block slides down.
What is the formula for the speed of the block at the bottom of the incline?
-The speed of the block at the bottom of the incline is given by the formula v = sqrt(2gh), where g is the gravitational acceleration and h is the height of the incline.
How is the speed of the 8 kg block released from the compressed spring calculated?
-The speed is calculated using the conservation of energy principle, where the elastic potential energy stored in the spring is converted into the kinetic energy of the block. The formula used is v = sqrt((1/2)kx^2 / m), where k is the spring constant, x is the compression, and m is the mass of the block.
What is the final speed of the 10 kg block sliding down a 200-meter tall hill with an initial speed of 12 m/s?
-The final speed of the block is calculated using the formula v^2 = v_initial^2 + 2gh. Plugging in the values, the final speed is v = sqrt((12 m/s)^2 + 2*9.8 m/s^2 * 200 m).
How does the presence of friction affect the final speed of the block in part b of the third problem?
-Friction reduces the final speed of the block because it does work against the motion of the block, converting some of the mechanical energy into thermal energy. The work done by friction is subtracted from the potential energy to find the final kinetic energy and speed.
What is the amount of thermal energy produced when the 12 kg block crashes into a wall at 15 m/s and comes to a stop?
-The thermal energy produced is equal to the initial kinetic energy of the block, which is calculated as (1/2)mv^2. For the 12 kg block at 15 m/s, this amounts to (1/2)*12 kg * (15 m/s)^2 = 1350 Joules.
In the car collision problem, what happens to the kinetic energies of the two cars before and after the collision?
-Before the collision, the kinetic energies of both cars are positive values based on their masses and velocities. After the collision, when both cars come to a complete stop, their kinetic energies become zero, and all the initial kinetic energy is converted into thermal energy due to the collision.
How is the speed of the roller coaster at point b calculated in the final problem?
-The speed of the roller coaster at point b is calculated using the conservation of energy principle, where the potential energy at the top (point a) is converted into kinetic energy at the bottom (point b). The formula used is v = sqrt(2gh), where g is the gravitational acceleration and h is the height from point a to point b.
What is the height of point c relative to point b on the roller coaster?
-The height of point c relative to point b is found by setting the potential energy at point a equal to the sum of the potential energy and kinetic energy at point c. Using the formula gh_initial = gh_final + (1/2)mv_final^2 and solving for h_final gives the height difference.
How fast is the roller coaster moving at point d after it has traveled from point a to point d?
-The speed at point d is determined by equating the potential energy at point a to the sum of the potential and kinetic energy at point d. Using the modified energy conservation equation and the given final height and initial potential energy, the final speed is calculated.
What is the significance of the conservation of energy principle in solving these types of problems?
-The conservation of energy principle is crucial in these problems as it allows us to relate different forms of energy at various points in a system. It simplifies the analysis by allowing us to equate the total mechanical energy (potential and kinetic) at different stages of the motion, making it easier to calculate unknown quantities such as final speeds or heights.
Outlines
📚 Conservation of Energy: Block on an Inclined Plane
This paragraph discusses the application of the conservation of energy principle to calculate the speed of a block sliding down a 150-meter inclined plane from rest. The initial potential energy due to gravity is converted into kinetic energy at the bottom of the incline. By setting up an equation based on energy conservation, the final speed of the block is determined to be √(2gh), where g is the acceleration due to gravity and h is the height of the incline. The calculation yields a speed of 54.22 meters per second, assuming no friction.
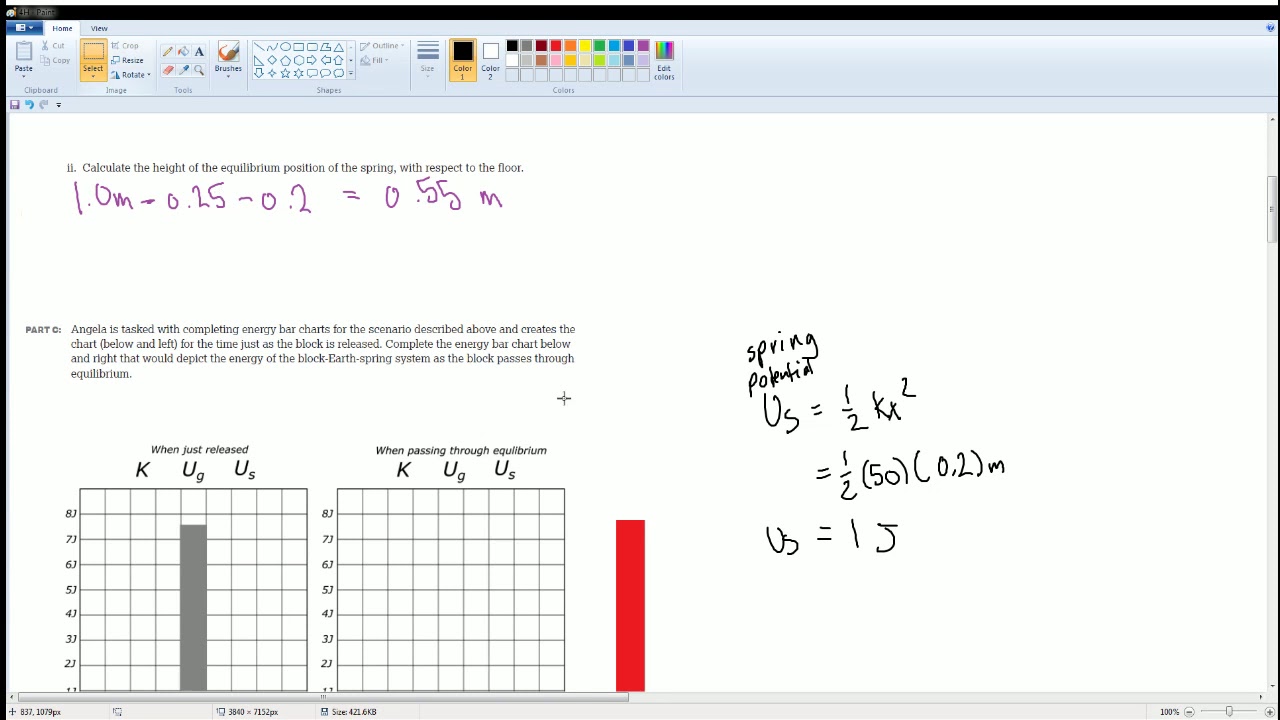
🏗️ Elastic Potential Energy and Block-Spring System
The focus of this paragraph is on a block-spring system where an 8-kilogram block compresses a horizontal spring by 2.5 meters. The goal is to find the block's speed upon release. Initially, the system possesses elastic potential energy stored in the compressed spring. Upon release, this energy is converted into the block's kinetic energy. Using the relationship between elastic potential energy (1/2 kx^2) and kinetic energy (1/2 mv^2), the block's final speed is calculated to be 15.31 meters per second. The paragraph further explores the block's subsequent motion, calculating how high it will ascend a hill before coming to rest, resulting in a height of approximately 12 meters.
🚀 Block Sliding Down a Hill with Initial Speed
This paragraph examines a 10-kilogram block sliding down a 200-meter tall hill with an initial speed of 12 meters per second. The task is to calculate the block's speed at the bottom of the hill, assuming no friction. By applying the conservation of energy principle, the potential energy at the top (mgh) and the initial kinetic energy (1/2 mv^2) are equated to the final kinetic energy at the bottom (1/2 mv^2). The final speed is found using the equation v^2 = v_initial^2 + 2gh, yielding a speed of 63.75 meters per second. The paragraph then introduces friction into the scenario and calculates the work done by friction, leading to a reduced final speed of 44.8 meters per second after traveling a total distance of 500 meters on a frictionless portion of the incline.
💥 Collision Energy Transfer
This paragraph explores the concept of energy transfer during collisions. It begins with a 12-kilogram block moving at 15 meters per second that crashes into a wall and comes to a complete stop. The kinetic energy of the block before the collision is converted into thermal energy upon impact. The thermal energy produced is calculated using the formula for kinetic energy (1/2 mv^2), resulting in 1350 joules of thermal energy. The paragraph then discusses a more complex scenario involving a 1500-kilogram car and an 1800-kilogram car colliding head-on, both coming to a complete stop. The total thermal energy produced by the collision is determined by summing the initial kinetic energies of both cars, amounting to 1,728,075 joules.
🎢 Roller Coaster Energy Conservation
The final paragraph of the script focuses on a roller coaster scenario where the roller coaster is released from rest at point A and its speed at various points (B, C, and D) is calculated using the conservation of energy principle. At point B, the potential energy at the top (mgh) is converted entirely into kinetic energy, resulting in a speed of 31.3 meters per second. At point C, the roller coaster has both potential and kinetic energy, and the height is determined by setting the initial potential energy equal to the sum of the final potential and kinetic energies. The height at point C is calculated to be 29.59 meters. Lastly, the speed at point D is found using a similar energy conservation approach, with the roller coaster moving at 26.19 meters per second.
Mindmap
Keywords
💡Conservation of Energy
💡Potential Energy
💡Kinetic Energy
💡Mechanical Energy
💡Gravitational Acceleration
💡Elastic Potential Energy
💡Friction
💡Work Done
💡Thermal Energy
💡Collision
💡Inclined Plane
Highlights
Conservation of energy principle used to solve physics problems involving inclined planes and blocks.
Block slides down a 150-meter incline from rest, reaching a speed of 54.22 meters per second at the bottom.
An 8-kilogram block compresses a horizontal spring by 2.5 meters, gaining a release speed of 15.31 meters per second.
Block ascends a hill after releasing from the spring, reaching a height of approximately 12 meters before stopping.
A 10-kilogram block slides down a 200-meter tall hill with an initial speed of 12 meters per second, reaching a speed of 63.75 meters per second at the bottom without friction.
When friction is introduced, the final speed of the block下滑 the hill is reduced to 44.8 meters per second after traveling 500 meters.
A 12-kilogram block moving at 15 meters per second crashes into a wall, producing 1350 joules of thermal energy.
In a car collision, a 1500-kilogram car moving at 35 meters per second and a 1800-kilogram car moving at 30 meters per second both come to a stop, producing 1,728,075 joules of thermal energy.
Roller coaster released from rest at point A, reaches a speed of 31.3 meters per second at point B using conservation of energy.
At point C, the roller coaster moves at 20 meters per second and is 29.59 meters higher than the ground level at point B.
The roller coaster at point D, after descending from point C, moves at a speed of 26.19 meters per second.
The potential and kinetic energy转换 when a block slides down an incline and comes to rest is explained using conservation of energy.
The concept of elastic potential energy stored in a compressed spring and its conversion to kinetic energy upon release is discussed.
The impact of friction on the mechanical energy of a sliding block and the resulting change in speed is analyzed.
The transformation of kinetic energy into thermal energy during a collision that brings an object to a stop is illustrated.
A step-by-step approach to solving physics problems using conservation of energy is provided, with clear explanations and calculations.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: