Basic Integration Using Power Formula

enginerdmath

9 Jan 202320:41

EducationalLearning

32 Likes 10 Comments

TLDRIn this informative video, the presenter delves into the fundamentals of basic integration, guiding viewers through the process of integrating algebraic expressions. The video covers various integral formulas, including the power rule, and demonstrates how to apply them to different scenarios, such as integrating monomials and rational expressions. The presenter also explains the concept of the constant of integration and provides step-by-step solutions to several integral problems, aiming to enhance the viewer's understanding of integration techniques.

Takeaways

👋 Hi there! This video is your go-to guide for mastering basic integration, especially with algebraic expressions.
✍ The fundamental concept of integration taught here begins with the integral of DX, which equals X plus C, highlighting the importance of the constant of integration in indefinite integrals.
💻 Through practical examples, the video demonstrates how to integrate expressions with constants, using the property that allows constants to be placed in front of the integral for simplification.
⬇ The power rule for integration is introduced, which is a crucial tool for integrating expressions like x squared, x cubed, and beyond, emphasizing that the exponent (n) should not equal -1.
📖 A deeper dive into the power formula showcases how even the simplest integrals, such as the integral of DX, can be derived using this approach, reinforcing the foundational principles of integration.
🤖 For more complex expressions, such as the square root of x or fractions involving x to a power, the video guides on transforming these into more manageable forms using fractional exponents before applying the power rule.
⚡ When encountering integrals involving multiple terms, the strategy of distributing the integral sign to each term and integrating term by term is thoroughly explained, offering clarity on tackling more complex problems.
🎯 The video also tackles integrals involving polynomial expressions multiplied by other polynomials, demonstrating how to expand, distribute, and then integrate term by term effectively.
✂ Techniques for simplifying expressions before integration, such as expanding squares of binomials and dealing with radicals in denominators, are clearly presented, making challenging problems more approachable.
✔ Finally, the importance of applying the constant of integration (+C) in indefinite integrals is emphasized throughout the video, ensuring a comprehensive understanding of basic integration principles.

Q & A

What is the integral of DX?
-The integral of DX is X + C, where C represents the constant of integration.
How do you integrate a constant multiplied by a function, as shown with the integral of 2dx?
-When integrating a constant multiplied by a function, place the constant in front of the integral sign. So, the integral of 2dx becomes 2 times the integral of dx, which simplifies to 2X + C.
What is the power rule for integration?
-The power rule for integration states that the integral of x^n dx is x^(n+1)/(n+1) + C, where n ≠ -1. This formula is used to evaluate the integrals of algebraic functions.
How is the integral of x squared calculated using the power rule?
-Using the power rule, the integral of x^2 dx is x^(2+1)/(2+1) + C, which simplifies to x^3/3 + C.
How can you derive the formula for the integral of DX using the power formula?
-You can express 1 as x^0 since x raised to the power of 0 is 1. Applying the power formula, the integral of x^0 dx becomes x^(0+1)/(0+1) + C, which simplifies to X + C.
How do you integrate expressions involving radicals, like the square root of x?
-To integrate expressions involving radicals, convert them into fractional exponents first. For example, the square root of x can be expressed as x^(1/2), and then you can apply the power rule.
What technique is used to integrate expressions like DX over X cubed?
-For expressions like DX over X cubed, apply the negative exponent law first to transform the denominator into x raised to a negative power, then apply the power rule for integration.
How do you integrate a polynomial function, as demonstrated with the integral of 3x^3 + 2x^2 - x + 5?
-To integrate a polynomial function, distribute the integral sign to each term and integrate term by term, applying the power rule and simplifying the expression.
What method is used to integrate a function multiplied by a constant, such as the integral of 5dx over the cube root of x squared?
-First, place the constant outside the integral sign. Then, express the cube root of x squared as x raised to a negative fractional exponent, and apply the power rule to integrate.
How do you simplify and integrate a complex function like x^3(x^2 - 2)^2?
-First, expand the binomial and distribute x^3 across each term. Then, apply the power rule to each term after distributing the integral sign, and simplify the expression.

Outlines

00:00

📚 Introduction to Basic Integration

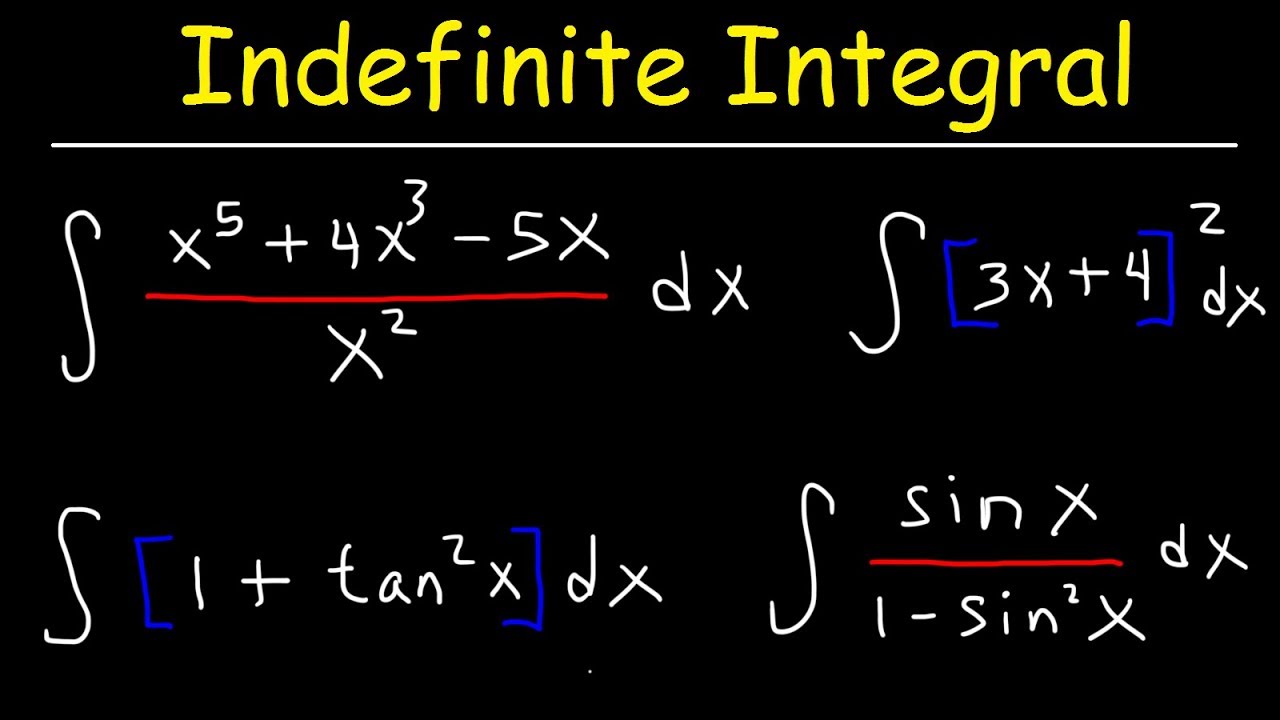
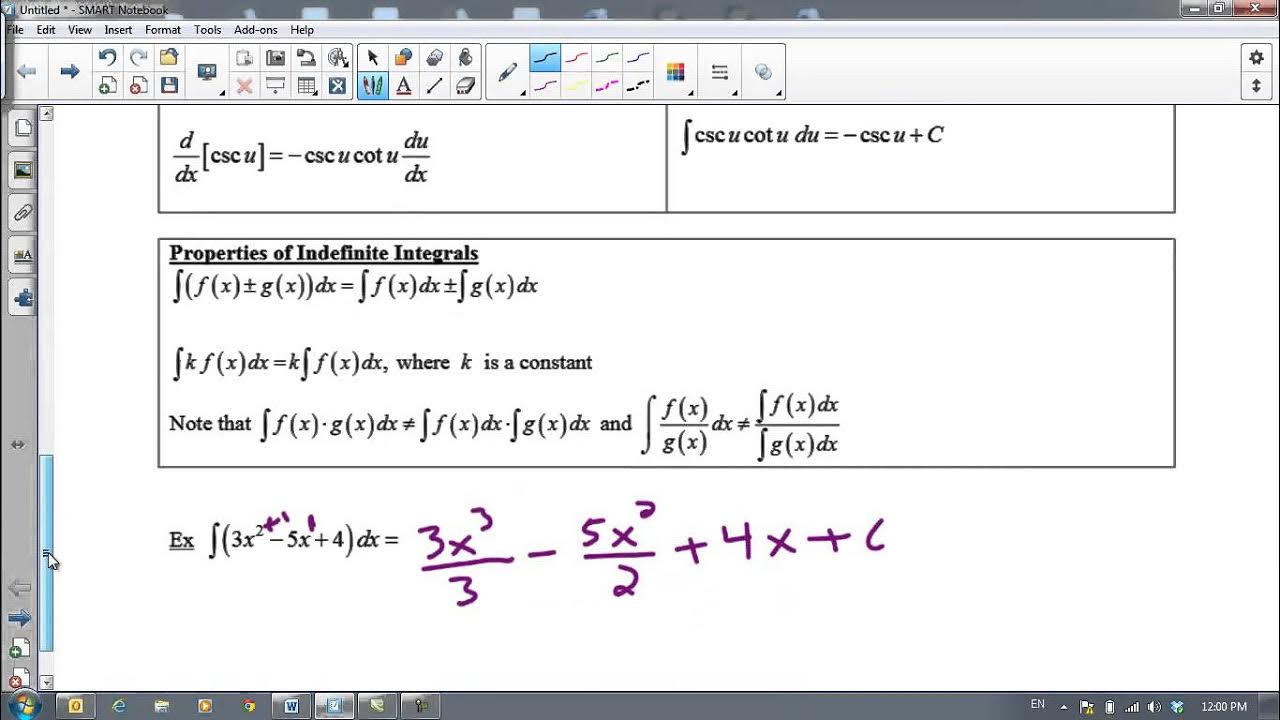
This paragraph introduces the concept of basic integration, focusing on integrating algebraic expressions. The video begins by explaining the integral of DX, which is the simplest form of integration, and emphasizes the importance of the constant of integration (C) in indefinite integrals. It then proceeds to illustrate how to handle constants in integrals and introduces the power rule for integration, which is a fundamental formula for integrating functions of the form x^n. The explanation includes the derivation of the integral of DX using the power rule and provides a step-by-step approach to solving basic integration problems.

05:01

🔢 Integration of Algebraic Functions

This section delves into the integration of more complex algebraic functions, including polynomials and radical expressions. It demonstrates how to apply the power rule to integrate x^n and how to handle integrals with radicals, such as the square root of x, by converting them into fractional exponents. The paragraph also covers the integration of functions with negative exponents, like 1/x^3, and explains the transformation of these expressions into positive exponents using the negative law of exponents. The integration process for each type of function is thoroughly explained, with a focus on the application of integration properties and rules.

10:03

📈 Integrating Polynomials and Trigonometric Functions

This paragraph discusses the integration of polynomials with multiple terms, such as 3x^3 + 2x^2 - x + 5, and emphasizes the distribution of the integral symbol across each term. It also introduces the integration of trigonometric functions, specifically focusing on the square root of x, and explains how to handle radical expressions in the denominator. The process of integrating each term individually and then combining the results is detailed, along with the application of the power rule to each component. The paragraph concludes with the final integrated form of the given polynomial and trigonometric function.

15:05

🧮 Complex Integration Techniques

This section presents advanced integration techniques for handling more complex expressions, such as products and quotients of functions. It explains how to simplify expressions before integration, using the example of x^3(x^2 - 2)^2. The paragraph outlines the process of expanding binomials, distributing the integrand, and integrating term by term. It also covers the integration of expressions with negative exponents and radicals in the numerator, providing a step-by-step guide on how to transform and simplify these expressions using the power rule and properties of exponents. The final integrated form of the complex expression is presented, highlighting the application of various integration rules and techniques.

20:06

🎓 Summary and Conclusion

In conclusion, the video provides a comprehensive overview of basic integration, covering a range of techniques from simple to complex integration problems. It emphasizes the importance of understanding the power rule, handling constants, and managing negative exponents and radicals. The video also encourages viewers to practice these integration techniques to solidify their understanding. The presenter expresses hope that viewers have gained valuable knowledge from the video and thanks them for their attention and participation.

Mindmap

Keywords

💡Integration

Integration, in the context of calculus, is a mathematical process of finding the integral of a function. It essentially measures the area under the curve of a graph of the function. The video focuses on teaching basic integration, specifically integrating algebraic expressions, highlighting its fundamental role in understanding calculus. Examples include integrating simple functions like dx and more complex expressions involving powers of x, showcasing integration as a versatile tool for solving a wide range of mathematical problems.

💡Indefinite Integral

An indefinite integral represents a family of functions that differ by a constant and is written without specific upper and lower limits. In the video, the concept is introduced to describe integrals that are evaluated without specifying bounds, emphasizing the importance of adding a '+C' (constant of integration) to the result. This concept is fundamental for understanding how to integrate expressions like dx, x squared, and more, as it highlights the essence of indefinite integrals in capturing a broad set of possible antiderivatives.

💡Constant of Integration

The constant of integration, denoted as 'C' in integration, represents an arbitrary constant that is added to the function when computing an indefinite integral. The video emphasizes this concept by explaining that every indefinite integral must include '+C' in the final answer to account for all possible antiderivatives. It is crucial in ensuring the completeness of the solution to an indefinite integral, such as when integrating dx to get x + C.

💡Power Rule

The power rule for integration is a fundamental formula used to integrate expressions involving powers of x. It states that the integral of x raised to the power of n dx is equal to x raised to the power of (n+1) divided by (n+1) plus C, given that n is not equal to -1. This rule is extensively used in the video to solve various integration problems, like integrating x squared or the square root of x, showcasing its importance in simplifying the integration process for polynomial functions.

💡Algebraic Expression

Algebraic expressions consist of constants, variables, and arithmetic operations. In the context of the video, integrating algebraic expressions involves finding the integral of expressions composed of such elements. Examples include the integration of 2dx, x squared dx, and more complex functions. This concept is central to the video's theme as it demonstrates the application of integration to a wide range of algebraic expressions, showcasing the diversity of functions that can be integrated using basic techniques.

💡Fractional Exponents

Fractional exponents represent powers of variables that are fractions, such as x raised to the 1/2. The video explains how to integrate expressions with fractional exponents, like the square root of x, by first converting the radical into a fractional exponent and then applying the power rule. This concept is crucial for understanding how to handle radical expressions within the integration process, broadening the scope of functions that can be integrated using basic techniques.

💡Negative Exponents

Negative exponents are used to represent expressions involving the reciprocal of a variable raised to a positive exponent, such as x to the power of -n equaling 1 over x to the n. The video discusses integrating expressions with negative exponents, like dx over x cubed, by first converting the expression into a form with a negative exponent and then applying the power rule. This approach is vital for integrating functions involving variables in the denominator, illustrating the adaptability of integration techniques to a range of mathematical expressions.

💡Binomial

A binomial is a polynomial with two terms. In the video, integrating expressions involving binomials, such as the square of (x-1), is demonstrated by first expanding the binomial and then applying integration techniques to each term separately. This method is part of teaching how to integrate more complex functions, emphasizing the importance of algebraic manipulation in preparing expressions for integration.

💡Complex Fraction

A complex fraction contains a fraction in either the numerator or the denominator. The video addresses integrating expressions that lead to complex fractions, illustrating the process of simplification to make the integration more manageable. For example, when integrating the square root of x, the result involves simplifying a complex fraction. This concept highlights the mathematical skill of simplification in solving integration problems efficiently.

💡Term-by-term Integration

Term-by-term integration refers to the technique of integrating each term of a polynomial or a series individually. This approach is showcased in the video when dealing with integrals of functions with multiple terms, such as 3x cubed plus 2x squared minus x plus 5. It demonstrates the procedural aspect of integration, where each term's integral is calculated independently before combining them for the final solution, illustrating a methodical approach to integrating complex expressions.

Highlights

Introduction to basic integration and its application in evaluating integrals of algebraic functions.

Explanation of the integral of DX, which is equal to X plus C, where C represents the constant of integration.

Discussion on the property of integrals where a constant can be factored out and multiplied by the integral of the function.

Introduction to the power rule for integration, which states that the integral of x^n dx equals (x^(n+1))/(n+1) + C, with the caveat that n should not be equal to -1.

Derivation of the integral of DX as X plus C using the power rule and algebraic manipulation.

Explanation of how to handle radicals in integrals by converting them into fractional exponents.

Integration of functions with negative exponents by applying the negative law of exponents and the power rule.

Method for integrating a constant over a radical expression by applying the power rule and simplifying complex fractions.

Process of distributing the integral symbol to each term in a polynomial and integrating term by term.

Expansion of binomials and distribution of terms in the integral for more complex expressions.

Integration of terms with the same base by applying the division law of exponents.

Explanation of how to handle integrals with a radical in the denominator by transforming it into a fractional exponent.

Demonstration of integrating a binomial squared by expanding it and applying the power rule to each term.

Integration of a complex expression involving a combination of terms with different exponents and roots.

Final summary of the video, emphasizing the learning outcomes and thanking the viewers for their attention.

Transcripts

Browse More Related Video

Indefinite Integral

INTEGRATION PART 1: SIMPLE CALCULATOR TECHNIQUES FOR SOLVING INDEFINITE INTEGRAL- WASSCE MATHS

Antiderivatives and Indefinite Integrals

Integration Using u-Substitution

Definite integral of rational function | AP Calculus AB | Khan Academy

Basic Integration... How? (NancyPi)

Related Tags

Mathematics Integration Algebra Education Online Tutorial Power Rule Integral Formulas Math Education Video Lesson Mathematics Teaching