Indefinite Integral

The Organic Chemistry Tutor
7 Mar 201810:47
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial delves into the process of integrating polynomial and basic functions, focusing on the concept of anti-derivatives. It demonstrates the use of the power rule for integration and provides step-by-step solutions to various problems, including integrating expressions like 3x - x^4, 6x^2 + √x, and the product (x + 5)(2x - 3). The video also covers integrating fractions by dividing terms in the numerator by the denominator and introduces the integration of trigonometric functions, using key identities to simplify the process. The content is designed to help viewers understand and apply integration techniques effectively.

Takeaways
  • 📚 The anti-derivative of a function is found using integration techniques, such as the power rule.
  • 🔢 For x to the power of n, the anti-derivative is (x^(n+1))/(n+1) + C, where C is the constant of integration.
  • 🧩 To integrate a sum or difference, integrate each term separately.
  • 🌟 The power rule can be applied to individual terms when integrating expressions like 6x^2 + √x.
  • 📈 When integrating a product, FOIL (First, Outer, Inner, Last) can be a helpful method before applying integration rules.
  • 🤹‍♂️ The anti-derivative of a polynomial is a sum of the anti-derivatives of its terms.
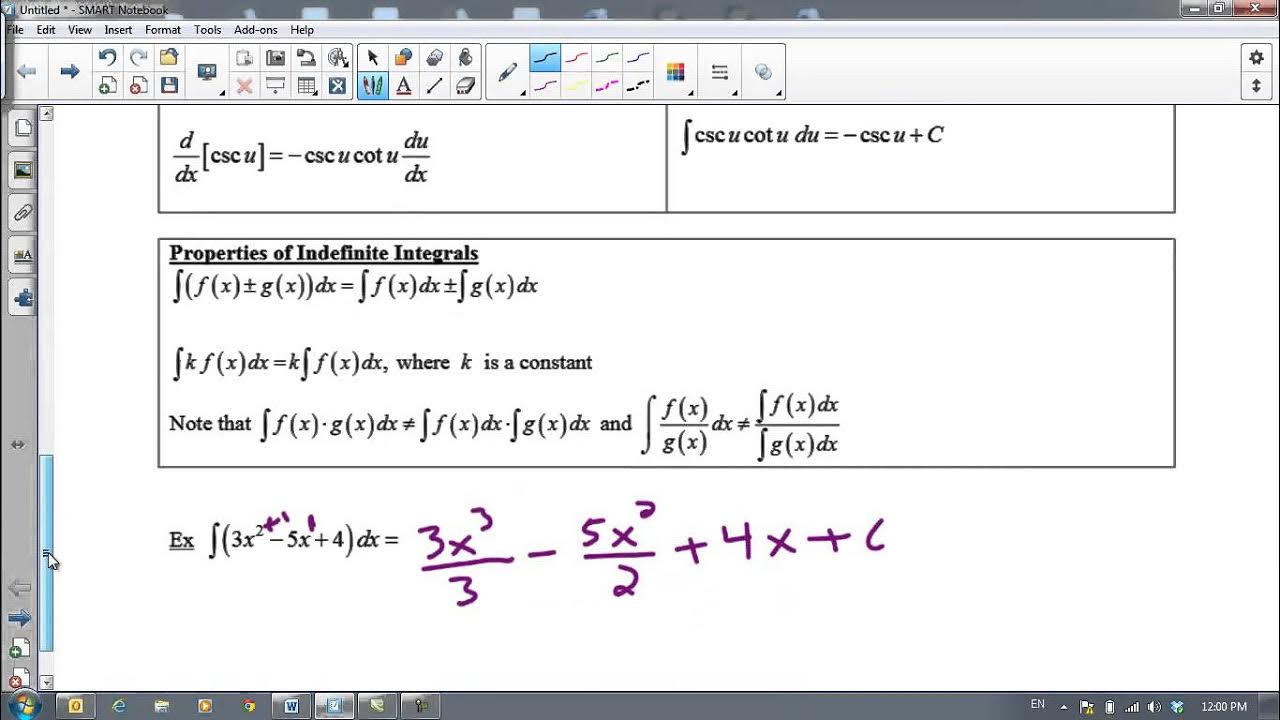
  • 📐 The anti-derivative of a squared binomial like (3x + 4)^2 can be found by expanding and integrating each term.
  • 📊 Rational functions are integrated by separating the expression into simpler fractions and integrating each term individually.
  • 🌐 For trigonometric integrals, knowledge of trigonometric identities such as Pythagorean and reciprocal identities is crucial.
  • 📚 The anti-derivative of 1 + tan^2(x) is tan(x), using the Pythagorean identity.
  • 🎓 Understanding the derivatives of trigonometric functions is key to finding their anti-derivatives, as seen with secant and tangent functions.
Q & A

  • What is the anti-derivative of 3x minus x to the fourth?

    -The anti-derivative of 3x is 3x^2/2, and the anti-derivative of -x to the fourth is -x^5/5. So, combining these, the anti-derivative of 3x - x to the fourth is 3x^2/2 - x^5/5 + C, where C is the constant of integration.

  • How do you find the anti-derivative of a function using the power rule?

    -The power rule states that the anti-derivative of x to the n is x to the power (n+1) divided by (n+1) plus C, where C is the constant of integration.

  • What is the purpose of rewriting the square root of x as x to the power of 1/2 when integrating?

    -Rewriting the square root of x as x to the power of 1/2 allows you to apply the power rule for integration more easily, since it transforms the square root into a standard power function.

  • How do you simplify the expression 6x squared plus the square root of x?

    -First, rewrite the square root of x as x to the power of 1/2. Then, apply the power rule to both terms. The anti-derivative of 6x squared is (6/3)x^(2+1) = 2x^3, and the anti-derivative of x to the 1/2 power is (2/3)x^(1.5). So, the simplified expression is 2x^3 + (2/3)x^(3/2) + C.

  • What is the process for finding the anti-derivative of a polynomial function like x plus 5 times (2x minus 3)?

    -First, distribute the constant (5) across the binomial (2x - 3) using the FOIL method (First, Outer, Inner, Last). This results in 2x^2 - 3x + 10x - 15. Then, combine like terms to get 2x^2 + 7x - 15. Finally, apply the power rule for integration: the anti-derivative of 2x^2 is (2/3)x^3, the anti-derivative of 7x is (7/2)x^2, and the anti-derivative of a constant (-15) is -15x. The result is (2/3)x^3 + (7/2)x^2 - 15x + C.

  • What is the anti-derivative of (x plus 4) squared?

    -Using the binomial expansion (FOIL method), (x + 4)^2 becomes x^2 + 8x + 16. The anti-derivative of x^2 is (1/3)x^3, the anti-derivative of 8x is (8/2)x^2, and the anti-derivative of 16 is 16x. So, the anti-derivative of (x + 4)^2 is (1/3)x^3 + 4x^2 + 16x + C.

  • How do you handle a fraction like x^5 plus 4x^3 minus 5x divided by x^2 when integrating?

    -Divide each term in the numerator by x^2. This results in 3x^3 plus 4x minus 5/x. Now, integrate each term separately: the anti-derivative of 3x^3 is (3/4)x^4, the anti-derivative of 4x is 2x^2, and the anti-derivative of -5/x is -5ln|x|. Combine these with the constant of integration C to get the final answer: (3/4)x^4 + 2x^2 - 5ln|x| + C.

  • What is the antiderivative of trigonometric functions, specifically in the case of 1 plus tangent squared x?

    -The antiderivative of 1 plus tangent squared x is tangent x plus C, as 1 plus tangent squared x is equivalent to secant squared x, and the derivative of secant squared is tangent.

  • How do you find the antiderivative of sine x divided by one minus sine squared x?

    -Use the Pythagorean identity sine squared x plus cosine squared x equals one to rewrite the expression as sine x divided by cosine squared x. Then, use the reciprocal identity where sine divided by cosine is tangent, and the derivative of secant is tangent, to find that the antiderivative is secant plus C.

  • What trigonometric identities are particularly useful for integration?

    -The Pythagorean identities (sine squared plus cosine squared equals one) and the reciprocal identities (such as sine divided by cosine is tangent, and cosine divided by sine is cotangent) are particularly useful for integrating trigonometric functions.

  • Why is it important to know trigonometric identities when integrating?

    -Trigonometric identities are crucial for simplifying complex trigonometric expressions before integration, allowing for the application of standard integration techniques and making the process more manageable.

  • What is the significance of the constant of integration, C, in anti-derivatives?

    -The constant of integration, C, is included because the derivative of a constant is zero. When finding an anti-derivative, the constant is added to account for the infinite number of functions that could have the same derivative, reflecting the arbitrary constant that can arise from the process of integration.

Outlines
00:00
📚 Introduction to Anti-Derivatives and Polynomial Integration

This paragraph introduces the concept of anti-derivatives and focuses on integrating polynomial functions. It begins with a problem involving the anti-derivative of 3x minus x to the fourth, using the power rule to find the solution. The explanation includes breaking down the problem into simpler integrals and combining them to reach the final answer. The paragraph then proceeds to solve additional problems, demonstrating the use of the power rule and the process of simplifying expressions before integration.

05:01
📈 Solving Fractional and Trigonometric Integrals

The second paragraph delves into integrating fractional and trigonometric expressions. It starts by explaining how to handle a fraction with a polynomial in the denominator by dividing each term in the numerator by the denominator. The paragraph then moves on to trigonometric integrals, emphasizing the importance of knowing Pythagorean identities and how to apply them to find anti-derivatives of trigonometric functions. The explanation includes working through examples that involve tangent and secant functions, showcasing the process of simplifying and integrating these expressions.

10:01
🎓 Review of Trigonometric Identities and Conclusion

The final paragraph reinforces the importance of understanding trigonometric identities and functions for integration. It provides a brief overview of the process of integrating trigonometric functions and encourages viewers to review these concepts further through a playlist on trigonometry available on the channel. The video concludes with a thank you note to the viewers for watching.

Mindmap
Keywords
💡Anti-derivative
The anti-derivative, also known as the integral, is a fundamental concept in calculus that represents the reverse process of differentiation. It is used to find the original function from which a given derivative could have been obtained. In the video, the anti-derivative of various functions like 3x - x^4 is calculated using the power rule, which states that the integral of x^n is x^(n+1)/(n+1) + C, where C is the constant of integration.
💡Power Rule
The power rule is a basic rule in calculus that allows the calculation of the derivative of a function that is a power of the variable. It states that the derivative of x^n, where n is any real number, is n*x^(n-1). In the context of integration, the power rule is used to find the anti-derivative of a function, which is the reverse process of differentiation.
💡Integration by FOIL
Integration by FOIL is a method used to integrate a polynomial function that is a product of two binomials. FOIL stands for First, Outer, Inner, Last, which refers to the terms that are multiplied in pairs when expanding the product. The method involves expanding the expression using FOIL, then integrating each term separately.
💡Trigonometric Functions
Trigonometric functions are mathematical functions that relate angles to the ratios of the sides of a right triangle. The most common trigonometric functions are sine (sin), cosine (cos), and tangent (tan). In the video, the antiderivative of trigonometric functions is discussed, using identities like 1 + tan^2(x) = sec^2(x) to simplify the integration process.
💡Pythagorean Identities
Pythagorean identities are fundamental relationships between trigonometric functions that are derived from the Pythagorean theorem. The most common identity is sin^2(x) + cos^2(x) = 1. These identities are crucial for simplifying trigonometric expressions and solving problems in calculus.
💡Integration by Substitution
Integration by substitution is a technique used to integrate functions that are not easily integrable using basic rules. It involves replacing a part of the integrand with a new variable, often to transform the function into a simpler form that can be integrated more easily.
💡Rational Functions
Rational functions are mathematical functions that have a ratio of two polynomials as their definition. They can be expressed in the form f(x)/g(x), where f(x) and g(x) are polynomials. Integrating rational functions often requires special techniques, such as partial fraction decomposition or substitution.
💡Natural Logarithm
The natural logarithm, denoted as ln(x), is the logarithm to the base e (where e is the mathematical constant approximately equal to 2.71828). It is a fundamental concept in calculus, particularly in integration, where the integral of 1/x is the natural logarithm of the absolute value of x plus a constant.
💡Secant
Secant is a trigonometric function that is the reciprocal of the cosine function. It is denoted as sec(x) and is defined as 1/cos(x). In the context of integration, the antiderivative of sec^2(x) is the tangent of x.
💡Tangent
Tangent is a trigonometric function that represents the ratio of the opposite side to the adjacent side in a right triangle. It is denoted as tan(x) and is equal to sin(x)/cos(x). The antiderivative of tan(x) is ln|sec(x)| or -ln|cos(x)|, depending on the context.
💡Integration
Integration is a fundamental process in calculus that involves finding the anti-derivative or the area under the curve of a given function. It is the reverse operation of differentiation and is used to solve a variety of problems, from determining the accumulated quantity to solving differential equations.
Highlights

Integrating polynomial functions and basic functions is the focus of the video.

The anti-derivative of 3x - x to the fourth is calculated using the power rule.

For x to the fourth, the anti-derivative is x to the fifth over five plus a constant.

The anti-derivative of 6x squared plus the square root of x is found by rewriting the square root as x to the one half and applying the power rule.

The final answer for the integral of 6x squared plus the square root of x is two x cubed times two thirds x raised to the three halves plus c.

When integrating an expression like x plus 5 times 2x minus 3, it's best to use the FOIL method before integrating.

The anti-derivative of a constant is simply the constant times x.

For the integral of three x plus four squared, the expression should be simplified by using the FOIL method and then integrated term by term.

The anti-derivative of a fraction involving a polynomial divided by x squared is found by dividing each term in the numerator by x squared and integrating each fraction separately.

The anti-derivative of x to the fifth power plus 4x cubed minus 5x divided by x squared simplifies to x cubed plus 4x minus five times the natural log of x plus a constant.

The antiderivative of 1 plus tangent squared x is secant squared, which can be found using a Pythagorean identity.

The antiderivative of sine x divided by one minus sine squared is secant, found by using trigonometric identities and rewriting the expression.

Trigonometric functions and identities, including reciprocal and Pythagorean identities, are essential for integrating certain functions.

The video provides a comprehensive guide on integrating various types of functions, including polynomials and trigonometric functions.

The power rule is a fundamental method for finding the anti-derivatives of monomials.

The FOIL method is recommended for integrating expressions that are products of binomials.

Understanding and applying trigonometric identities is crucial for integrating certain trigonometric functions.

The video demonstrates the process of simplifying complex integrals by using algebraic methods like FOIL and by applying trigonometric identities.

Transcripts

Browse More Related Video

Calculus AB Homework 6.7 U-Substitution

Definite Integral Calculus Examples, Integration - Basic Introduction, Practice Problems

Integration Using u-Substitution

Basic Integration Using Power Formula

Integration by Parts

Antiderivatives and Indefinite Integrals

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusIntegrationPolynomialsPower RuleTrigonometryAnti-DerivativeFoil MethodTrig IdentitiesMath EducationOnline Learning