Antiderivatives and Indefinite Integrals
TLDRThis educational video script delves into the concepts of anti-derivatives and indefinite integrals, presenting them as creative and challenging puzzles. It explains how anti-derivatives 'undo' derivatives, using the power rule in reverse to find integrals. The script covers basic integration rules, properties of indefinite integrals, and the lack of product and quotient rules for integration. It provides step-by-step examples of integrating polynomials, rational functions, and trigonometric functions, emphasizing the importance of including the constant of integration. Additionally, it demonstrates solving differential equations and finding position functions from velocity, showcasing the practical application of integration in various mathematical contexts.
Takeaways
- 🔍 Anti-derivatives and indefinite integrals are essentially the reverse of derivatives, allowing us to 'undo' a derivative to find the original function.
- 🧩 The process of finding an anti-derivative is likened to solving a puzzle, emphasizing the creative and challenging nature of the task.
- 📈 When finding an anti-derivative, the resulting function may not be unique due to the constant of integration, which can be any real number.
- 📚 The integral symbol, represented by a squiggly 's', is used to denote the process of integration, similar to how 'dx' is used in differentiation.
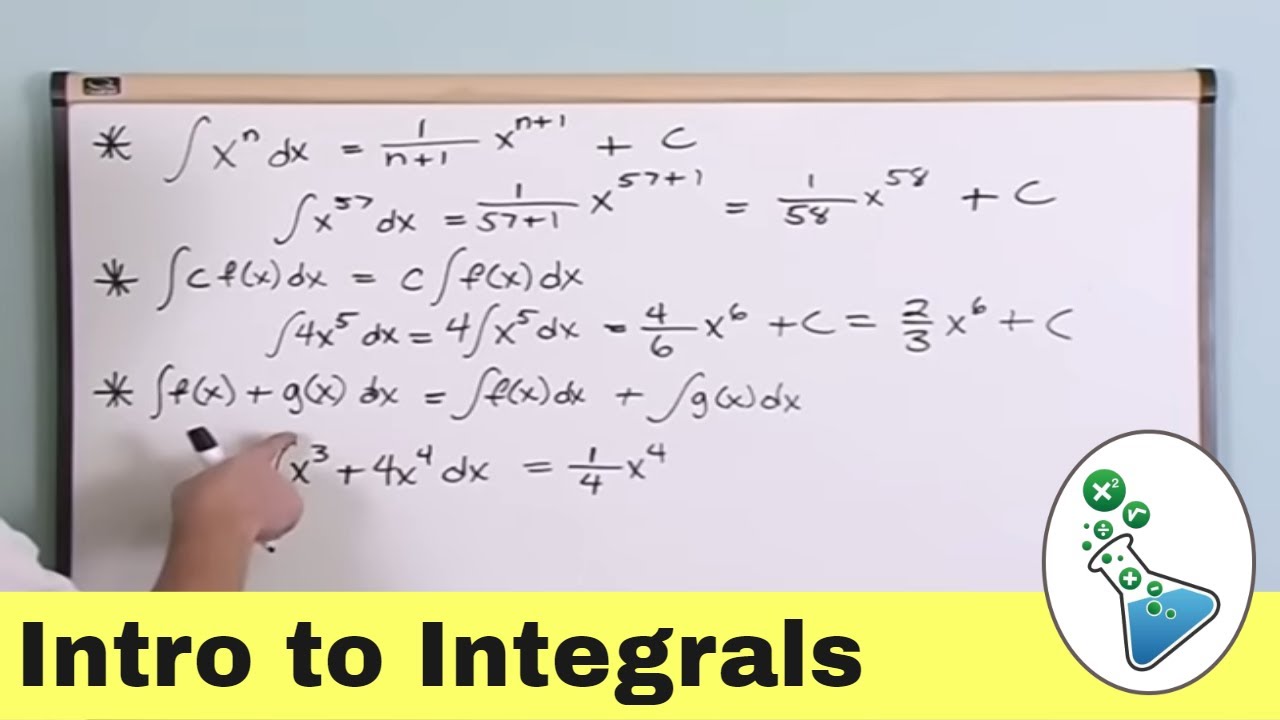
- 🔢 Basic integration rules involve reversing the power rule for derivatives, which involves adding one to the exponent and dividing by that new exponent.
- 🔄 Properties of indefinite integrals include the ability to distribute constants outside the integral and to handle integrals of sums by integrating each term separately.
- 🚫 Unlike derivatives, there is no product or quotient rule for integrals, meaning integration of a product or quotient of functions does not simplify in the same way.
- 📉 To check the correctness of an integral, one can differentiate the result and verify if it matches the original function to be integrated.
- 📚 The process of integrating functions can involve combining algebraic manipulation with the application of integration rules, especially for polynomials.
- 🔄 When dealing with differential equations, one can find the original function by integrating the given derivative, and using additional information to solve for constants.
- 🚀 The script also touches on practical applications, such as finding position functions from velocity functions in physics, by integrating and solving for constants using given points.
Q & A
What is an anti-derivative and why is it also called an indefinite integral?
-An anti-derivative is a function that reverses the process of differentiation. It is also called an indefinite integral because it represents the family of functions that could have produced a given derivative, including an arbitrary constant, hence the term 'indefinite'.
Why can't we be certain of the exact function when we find an anti-derivative?
-When finding an anti-derivative, we can't be certain of the exact function because any constant can be added to the anti-derivative without changing its derivative. This is why the anti-derivative is often written as the function plus an arbitrary constant, typically denoted by 'C'.
What is the general rule for integrating a function of the form f(x) = x^n?
-The general rule for integrating a function of the form f(x) = x^n is to add one to the exponent and then divide by the new exponent. This is derived from reversing the power rule for differentiation.
How do you integrate a function with a constant in front of it?
-When integrating a function with a constant in front of it, you can factor the constant out in front of the integral sign. The constant is then multiplied by the result of the integral of the function inside.
What are the properties of indefinite integrals when dealing with plus or minus signs?
-When dealing with plus or minus signs in indefinite integrals, you can integrate each function separately and then add or subtract the results. This is because integration is a linear operation.
Why is there no product rule or quotient rule for integrals like there is for derivatives?
-There is no product rule or quotient rule for integrals because integration is not a commutative operation like differentiation. The integral of a product or quotient of functions does not simply decompose into the product or quotient of their integrals.
How can you check if your integral is correct?
-You can check if your integral is correct by differentiating your result. If the derivative of your integral matches the original function you were integrating, then your integral is correct.
How do you integrate a polynomial function?
-To integrate a polynomial function, you can integrate each term separately using the rule for integrating powers of x, and then combine the results. Remember to add the constant of integration at the end.
What is a differential equation and how does it relate to anti-derivatives?
-A differential equation is an equation that involves a function and its derivatives. To solve a differential equation, you often need to find an anti-derivative of the given derivative to get back to the original function.
How can you find the exact function from a derivative and a point on the curve?
-You can find the exact function from a derivative and a point on the curve by first finding the anti-derivative of the derivative, which gives you the family of functions. Then, you use the point on the curve to determine the specific constant in the family of functions.
What is the relationship between position, velocity, and acceleration in the context of motion?
-In the context of motion, acceleration is the derivative of velocity, velocity is the derivative of position, and position is the integral (or anti-derivative) of velocity. These relationships allow you to move between different aspects of motion when given one of them.
Outlines
📚 Introduction to Anti-derivatives and Indefinite Integrals
The video script begins with an introduction to anti-derivatives and indefinite integrals, explaining that they are processes to reverse derivatives. The instructor likens the process to solving puzzles and challenges the audience with the question of finding a function given its derivative. The concept of anti-derivatives being uncertain but certain in their powers of x is introduced, along with the notation for integrals. The instructor also explains the basic integration rules that are the reverse of differentiation rules, such as the power rule for derivatives, and emphasizes the importance of the constant of integration.
🔍 Detailed Explanation of Integration Rules and Properties
This paragraph delves deeper into the rules of integration, discussing how to integrate functions with plus or minus signs and the impact of constants on integrals. The instructor clarifies that there are no product or quotient rules for integrals, unlike in differentiation, and provides an example of integrating a sum of functions. The process of integrating each term separately and adding a constant at the end is demonstrated, along with a method to check the correctness of integration by differentiating the result.
📝 Practical Integration Techniques and Examples
The script continues with practical examples of integration, including the integration of polynomials and the handling of like terms. The instructor shows step-by-step integration processes, emphasizing the importance of adding the constant of integration. The paragraph also covers the integration of functions involving square roots and how to simplify expressions by separating terms and applying the power rule in reverse. The instructor cautions against incorrectly applying quotient or product rules to integrals and illustrates the correct approach.
🔧 Solving Differential Equations and Applications in Physics
In this section, the script discusses the application of integration in solving differential equations, which are essentially equations derived from a function. The process of finding the original function from its derivative is demonstrated using a given point on the curve. The instructor also applies these concepts to physics problems involving position, velocity, and acceleration, showing how to find the position function from a velocity function and solve for the constant of integration using a known position at a specific time.
Mindmap
Keywords
💡Anti-derivatives
💡Indefinite Integrals
💡Derivative
💡Integration Rules
💡Constant of Integration
💡Differential Equation
💡Position Function
💡Velocity Function
💡Acceleration Function
💡Trigonometric Functions
Highlights
Introduction to anti-derivatives and indefinite integrals, emphasizing the concept of reversing derivatives.
Explanation of anti-derivatives as creative and challenging, likened to solving puzzles.
Discussion on the possibility of different functions having the same derivative, such as x^3 and x^3 - 7.
Graphical representation of functions with the same derivatives to illustrate their differences.
Introduction of the integral symbol and its origin, relating it to the process of integration.
Clarification that indefinite integrals are synonymous with anti-derivatives.
Integration rules for undoing derivatives, such as the power rule in reverse.
Properties of indefinite integrals, including handling plus/minus signs and constants.
Absence of product and quotient rules in integration, contrasting with differentiation.
Demonstration of integrating a sum of functions and the importance of adding a constant.
Process of checking integration work by differentiating the result back to the original function.
Integration of polynomials and the use of algebraic manipulation for easier integration.
Dealing with integrals involving square roots by rewriting them as fractional exponents.
Solving differential equations by integrating given derivatives to find original functions.
Using additional information to determine the constant in an indefinite integral.
Application of integration to physics problems, such as finding position from velocity.
Final summary of the process for integrating to find functions in physics, including solving for constants.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: