Basic Integration... How? (NancyPi)
TLDRIn this informative video, Nancy guides viewers through the fundamentals of integration, explaining it as the reverse process of differentiation. She covers basic integration rules, such as the Power Rule, and demonstrates how to apply them to various functions, including polynomials, roots, and trigonometric functions. The video also touches on the importance of the constant of integration, '+c', and how it represents all possible antiderivatives. Nancy emphasizes the use of algebraic manipulation and trigonometric identities to simplify complex integrals, providing a solid foundation for those new to calculus.
Takeaways
- 📚 Integration is the reverse process of differentiation, used to find the original function given its derivative.
- 📈 The Power Rule states that the integral of x^n (where n ≠ -1) is x^(n+1)/(n+1) + c.
- 🔢 When a constant is multiplied by x^n, the constant remains in the integral and is multiplied by the result of the power rule.
- 🌟 The integral of x to the power of 1 (or implicitly 1) is x^2/2, following the Power Rule.
- 🅰️ The integral of a constant is simply the constant times x, which is x times the constant.
- 🔄 The Sum and Difference rules allow you to integrate each term of a polynomial separately and then combine them.
- 💡 The Power Rule cannot be applied to a power of -1; the integral of 1/x is ln|x| + c.
- 🌐 For negative and fractional powers, as well as roots, the Power Rule can still be applied after rewriting them in an appropriate form.
- 📊 Trigonometric integrals often involve sine and cosine and can be found in a table of integrals, showing relationships that are cyclical in nature.
- 🔧 Some integrals may require algebraic manipulation, such as separating terms or using trigonometric identities, to fit a basic integration rule.
- 🛠️ More complex integrals may need advanced techniques like integration by parts, u-substitution, or other methods to simplify them for integration.
Q & A
What is integration?
-Integration is the reverse process of differentiation. It is used to find the original function from which a given derivative is obtained.
What does the 'dx' in an integral represent?
-The 'dx' in an integral indicates the differential with respect to the variable of integration, which in this case is 'x'.
What is the significance of the '+ c' at the end of an integral?
-The '+ c' represents the constant of integration, which accounts for all possible antiderivatives since the original function could have had any constant term.
How does the Power Rule work in integration?
-The Power Rule states that the integral of x to the power 'n' is x to the power 'n+1' divided by 'n+1', plus 'c', where 'n' is not equal to -1.
What happens when a constant is multiplied in front of x in an integral?
-When a constant is multiplied in front of x, you can keep the constant and then apply the Power Rule to the 'x' part of the expression.
How is the integral of a constant term handled?
-The integral of a constant term is simply the constant multiplied by 'x'.
What is the integral of a negative power of x?
-The integral of a negative power of x can still be found using the Power Rule, but the result will be a negative exponent, which is often rewritten to avoid negative powers.
What is the integral of 1/x?
-The integral of 1/x is the natural logarithm of the absolute value of x, denoted as ln|x| + c.
How can you handle integrals with roots?
-Integrals with roots can be rewritten as powers, where the root index becomes the exponent, and then the Power Rule can be applied.
What is the relationship between the derivatives and integrals of sine and cosine?
-The derivatives and integrals of sine and cosine are cyclical and opposite operations. For example, the derivative of sine is cosine, and the integral of cosine is sine.
What should you do if an integral does not directly fit a basic integration rule?
-If an integral does not directly fit a basic rule, you may need to use more advanced techniques such as integration by parts, u-substitution, partial fractions, improper integrals, or trigonometric substitution.
Outlines
📚 Introduction to Integration
Nancy introduces the concept of integration, explaining it as the reverse process of differentiation. She outlines the basic rules of integration, including the Power Rule and the importance of the constant (+c) at the end of the integral. Nancy demonstrates how to integrate polynomials by integrating each term individually and then combining them. She emphasizes the relationship between integration and differentiation rules, highlighting that while many are similar, some, like the Product Rule or Quotient Rule, do not have an integration counterpart.
🔢 Dealing with Powers and Roots
The video continues with an exploration of integrating various powers, including negative and fractional powers, and roots. Nancy explains how to apply the Power Rule to non-integer powers and how to convert roots into fractional powers for integration. She also addresses the special case of the -1 power, which is not defined by the Power Rule but instead results in the natural logarithm of the absolute value of x. The paragraph emphasizes the importance of rewriting expressions into a form that fits basic integration rules and the use of algebraic simplification in the integration process.
📈 Advanced Integration Techniques
Nancy discusses more complex integrals that do not directly fit basic rules, such as those involving quotients and radicals. She advises on using algebraic techniques to simplify the integral before applying integration rules. The video touches on trigonometric integrals and the use of trig identities to transform expressions into integrable forms. Nancy also mentions more advanced techniques like integration by parts, u-substitution, and partial fractions, suggesting that viewers explore her other videos for a deeper understanding of these methods.
🎓 Wrapping Up and Encouragement
In the final paragraph, Nancy wraps up the video by encouraging viewers to apply the basic integration techniques learned. She acknowledges that calculus might not be everyone's favorite subject but invites viewers to engage with the content by liking or subscribing to her videos. Nancy's approach is to make complex topics like integration more accessible and less intimidating for learners.
Mindmap
Keywords
💡Integration
💡Power Rule
💡Antiderivative
💡Constant Rule
💡Trigonometric Integrals
💡Trig Identities
💡Sum and Difference Rules
💡Differential
💡Negative Power
💡Fractional Power
💡Root
Highlights
Integration is the reverse process of differentiation.
The integral of a function找回 the original function.
The Power Rule states that the integral of x^n is x^(n+1)/(n+1) + c, where n ≠ -1.
Constants can be taken outside the integral and then applied to the result.
The integral of a constant term is the number times x.
The Power Rule can be applied to negative powers, but not -1.
The integral of 1/x is ln|x| + c.
Fractional powers can be treated with the Power Rule by adding 1 and simplifying.
Roots can be rewritten as powers to apply the Power Rule.
Trigonometric integrals often involve sine and cosine and are cyclical.
Trig identities can help rewrite integrals in a form that can be integrated using basic rules.
The integral of e^x is e^x + c.
Complex integrals may require advanced techniques like integration by parts or u-substitution.
The + c at the end of an integral represents all possible antiderivatives.
Integration can be done term by term for polynomials with added and subtracted terms.
The Sum and Difference rules allow for the separation and combination of terms in integration.
Some integrals require rewriting in a form that fits basic integration rules.
The video provides a foundation for understanding basic integration techniques and rules.
Transcripts
Browse More Related Video
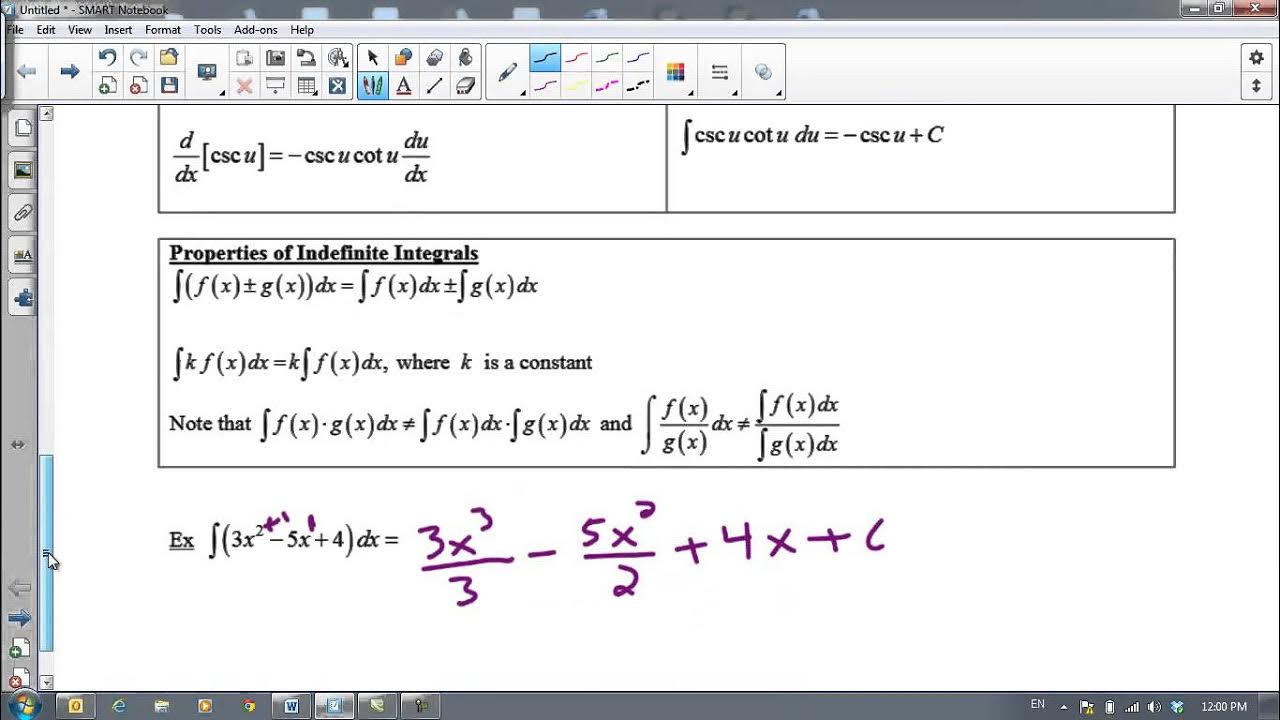
Antiderivatives and Indefinite Integrals

Definite Integral Calculus Examples, Integration - Basic Introduction, Practice Problems

Calculus AB Homework 6.1 Antiderivatives
Indefinite Integral - Basic Integration Rules, Problems, Formulas, Trig Functions, Calculus

How to Integrate Using U-Substitution (NancyPi)
Calculus 1 - Integration & Antiderivatives
5.0 / 5 (0 votes)
Thanks for rating: