Existence and Uniqueness of Solutions (Differential Equations 11)

Professor Leonard

8 Oct 201844:32

EducationalLearning

32 Likes 10 Comments

TLDRThe video delves into the conditions for the existence and uniqueness of solutions to differential equations. It explains that solutions are typically discussed in relation to specific points and introduces three possible scenarios: no solution, infinitely many solutions, or a unique solution. The importance of continuity and differentiability in ensuring a unique solution is emphasized, with examples illustrating how to check for these conditions at given points.

Takeaways

📚 The video discusses the existence and uniqueness of solutions to differential equations, focusing on the conditions under which solutions are guaranteed.
🔍 Existence of a solution to a differential equation at a point is not guaranteed; it depends on the continuity of the function around that point.
🌐 A general solution is represented by a slope field, while a particular solution is a specific curve through a point on the slope field.
📈 The video outlines three cases for solutions at a point: no solution, infinitely many solutions, and a unique solution.
🚫 Case one: A differential equation may not have a particular solution through a specific point, even though it has solutions through other points.
∞ Case two: A differential equation can have infinitely many solutions through a specific point, making the solution non-unique.
💡 Case three: A unique solution exists for a differential equation at a point if the function is continuous around that point and the partial derivative with respect to Y is also continuous.
🔄 Continuity is crucial for both existence and uniqueness of solutions; it must be checked for the function and its partial derivative.
📌 The concept of 'neighborhood' is introduced to explain the importance of considering points before and after a given point to determine continuity.
📐 The video emphasizes that endpoints are not allowed for continuity; an open interval is required to ensure the existence and uniqueness of solutions.
🔢 Examples are provided to demonstrate how to check for continuity and determine the existence and uniqueness of solutions for different types of differential equations.

Q & A

What are the three cases for the existence of solutions to a differential equation at a point?
-The three cases are: 1) No solution exists at the specific point, 2) Infinitely many solutions exist through that point, and 3) A unique solution exists in the neighborhood of that point.
What is the importance of general solutions and specific solutions in differential equations?
-General solutions provide a broad understanding of the possible solutions to a differential equation, while specific solutions give exact solutions through a particular point with a specific initial value.
Why is it important to consider solutions through a point for differential equations?
-Solutions through a point are important because they allow us to zoom in on specific cases and provide concrete solutions that can be used for further analysis or practical applications.
What does it mean for a differential equation to have no solution at a specific point?
-It means that there is no curve or function that satisfies the differential equation at that particular point, although the equation might have solutions at other points.
How does the concept of a neighborhood play a role in the uniqueness of solutions to a differential equation?
-A neighborhood around a point is necessary to ensure that a solution is unique because it allows for the consideration of continuity and differentiability in the vicinity of that point, which are key for guaranteeing a unique solution.
What is the role of continuity in determining the existence of a solution to a differential equation?
-Continuity is crucial because it ensures that the function under consideration is well-behaved around a specific point, which is a prerequisite for the existence of a solution to the differential equation at that point.
What does it mean for a solution to a differential equation to be unique?
-A unique solution means that there is only one curve or function that satisfies the differential equation in the neighborhood of the given point, without any other solutions overlapping or intersecting it.
What is a partial derivative, and how is it used in the context of differential equations?
-A partial derivative is a derivative that treats all other variables as constants except for one, which is varied. In differential equations, taking partial derivatives with respect to the variable of interest (usually 'y') helps in determining the uniqueness of solutions.
Why is it necessary for the point of interest in a differential equation to not be at the endpoint of a continuity interval?
-Endpoints are not allowed because they can disrupt the continuity of the function, which is essential for the existence and uniqueness of solutions. An open interval ensures that the function is well-defined and differentiable at the point of interest.
How does the presence of discontinuities affect the existence and uniqueness of solutions to a differential equation?
-Discontinuities can prevent the existence of a solution at a specific point and can also lead to multiple solutions, thus violating the uniqueness of the solution. Continuity without discontinuities is necessary for guaranteed existence and uniqueness.

Outlines

00:00

📘 Introduction to Differential Equation Solutions

This paragraph introduces the topic of solving differential equations, emphasizing the importance of understanding when solutions exist and when they are unique. It sets the stage for discussing the conditions under which solutions to a differential equation are guaranteed and how to identify unique solutions through a point.

05:01

🔍 Understanding Continuity for Solution Existence

The paragraph delves into the concept of continuity as it relates to the existence of solutions for differential equations. It explains that to determine if a curve is continuous at a point, one must consider a neighborhood around that point, which includes points before and after it. The discussion highlights the open interval nature of continuity and its significance for differentiability and uniqueness of solutions.

10:03

📌 Continuity and Partial Derivatives for Uniqueness

This section focuses on the conditions for uniqueness of solutions to a differential equation. It outlines that if the function is continuous around a point and the partial derivative of the function with respect to Y is also continuous around that point, then the solution to the differential equation is unique in the neighborhood of that point.

15:04

🧐 Case Study: dy/dx = 2x^2y^2

The paragraph presents a specific example of a differential equation, dy/dx = 2x^2y^2, and examines the existence and uniqueness of its solutions at the point (1, -1). It demonstrates how to check for continuity of the function and its partial derivative, leading to the conclusion that there is one unique solution at that point.

20:04

🔎 Analyzing Different Points for Existence and Uniqueness

This section continues the analysis by looking at different points for the differential equation dy/dx = x * ln(y). It shows how the continuity of the function and its partial derivative can vary depending on the point being considered, affecting the existence and uniqueness of solutions.

25:06

🤔 Challenging Cases: Square Roots and Continuity

The paragraph discusses the challenges in determining the continuity of square root functions, emphasizing the importance of ensuring that the radicand is positive for continuity. It also addresses common mistakes in identifying intervals of continuity and the implications for the existence and uniqueness of solutions to differential equations.

30:06

📝 Summary of Continuity Checks for Differential Equations

The final paragraph wraps up the discussion by reiterating the process of checking for continuity of the function and its partial derivative to determine the existence and uniqueness of solutions to differential equations. It encourages viewers to practice these concepts with provided examples to solidify their understanding.

Mindmap

Keywords

💡Differential Equations

Differential equations are mathematical equations that involve an unknown function and its derivatives. In the context of the video, they are the central topic, with the discussion focusing on when solutions to these equations exist and are unique. The video explains that solutions are often considered at specific points, leading to particular solutions that pass through a given point on a slope field.

💡Solutions

In the context of differential equations, a solution is a function that satisfies the equation. The video emphasizes the difference between general solutions, which are solutions to the differential equation anywhere, and particular solutions, which are solutions through a specific point. The existence and uniqueness of these solutions are key points discussed in the video.

💡Slope Fields

A slope field is a graphical representation of the possible slopes of a function at every point in the plane. In the video, slope fields are used to illustrate the general solutions to differential equations. They provide a visual way to understand the behavior of solutions and to find particular solutions by following the slopes through a specific point.

💡Continuity

Continuity in mathematics refers to the property of a function that does not have any gaps, jumps, or breaks. In the context of the video, continuity is crucial for the existence and uniqueness of solutions to differential equations. The function's continuity around a point ensures that there is a smooth transition in values, which is necessary for the existence and uniqueness of solutions.

💡Partial Derivatives

A partial derivative is a derivative of a function with respect to one variable, treating all other variables as constants. In the video, partial derivatives are used to check for the uniqueness of solutions to differential equations. The continuity of the partial derivative around a point is one of the conditions that ensure a unique solution.

💡Neighborhood

In the context of the video, a neighborhood refers to an open interval around a point, which is used to discuss the continuity of a function and its partial derivatives. The existence and uniqueness of solutions to differential equations are discussed in relation to the neighborhood of a point, emphasizing the importance of considering points before and after the given point to ensure continuity.

💡Existence

Existence in the context of the video refers to the condition where a solution to a differential equation exists at a given point or within a certain region. The video explains that the existence of a solution is guaranteed if the function is continuous around the point in question, but not at an endpoint of the interval of continuity.

💡Uniqueness

Uniqueness in the context of the video pertains to the condition where there is only one solution to a differential equation at a given point. The video explains that uniqueness is guaranteed if the function and its partial derivative with respect to Y are both continuous around the point, and the point is not at an endpoint of the interval of continuity.

💡Endpoints

Endpoints in the video refer to the boundaries of an interval. The concept is important when discussing continuity and the existence and uniqueness of solutions to differential equations. The video emphasizes that endpoints are not allowed when considering the continuity of a function or its partial derivatives; the interval must be open, meaning it does not include its endpoints.

💡General Solution

A general solution to a differential equation is a solution that is valid for all values of the independent variable without specifying any particular initial conditions. In the video, the general solution is contrasted with particular solutions, which are specific solutions that satisfy an initial condition. The general solution is represented by a slope field, which provides information about the possible paths of particular solutions.

Highlights

The video discusses the conditions for the existence and uniqueness of solutions to differential equations.

Differential equations are often considered at specific points, leading to particular solutions.

A general solution is represented by a slope field, while a particular solution is through a specific point.

There are three cases for solutions to a differential equation at a point: no solution, infinitely many solutions, or a unique solution.

A differential equation can have many solutions but may not have a particular solution through a certain point.

For a curve to be continuous at a point, it is necessary to consider a neighborhood around that point.

Continuity is an open idea, requiring a little bit before and after the point to ensure an open interval.

Differentiability, which involves derivatives, requires continuity for the function to be differentiable at a point.

The existence of a solution to a differential equation at a point is guaranteed if the function is continuous around an open interval including that point's x-value.

The uniqueness of a solution is ensured if the partial derivative of the function with respect to y is also continuous around the point.

Examples are provided to illustrate how to check for the existence and uniqueness of solutions to differential equations at specific points.

The importance of checking for continuity and differentiability in the neighborhood of a point is emphasized for solving differential equations.

The video explains that the x-value of a point must not be an endpoint for the interval of continuity.

A function's continuity around a point does not guarantee uniqueness; the partial derivative's continuity must also be checked.

The video provides a method for checking the continuity of functions and their partial derivatives to determine the existence and uniqueness of solutions.

The concept of an open interval and its relation to continuity and the existence of solutions is clarified.

The video concludes with a preview of upcoming content on techniques for solving differential equations.

Transcripts

Browse More Related Video

Lec 4: Square systems; equations of planes | MIT 18.02 Multivariable Calculus, Fall 2007

The Theory of 2nd Order ODEs // Existence & Uniqueness, Superposition, & Linear Independence

Lec 1 | MIT 18.03 Differential Equations, Spring 2006

AP Calculus AB: Lesson 7.2 Slope Fields

Ch. 10.1 Systems of Linear Equations in Two Variables

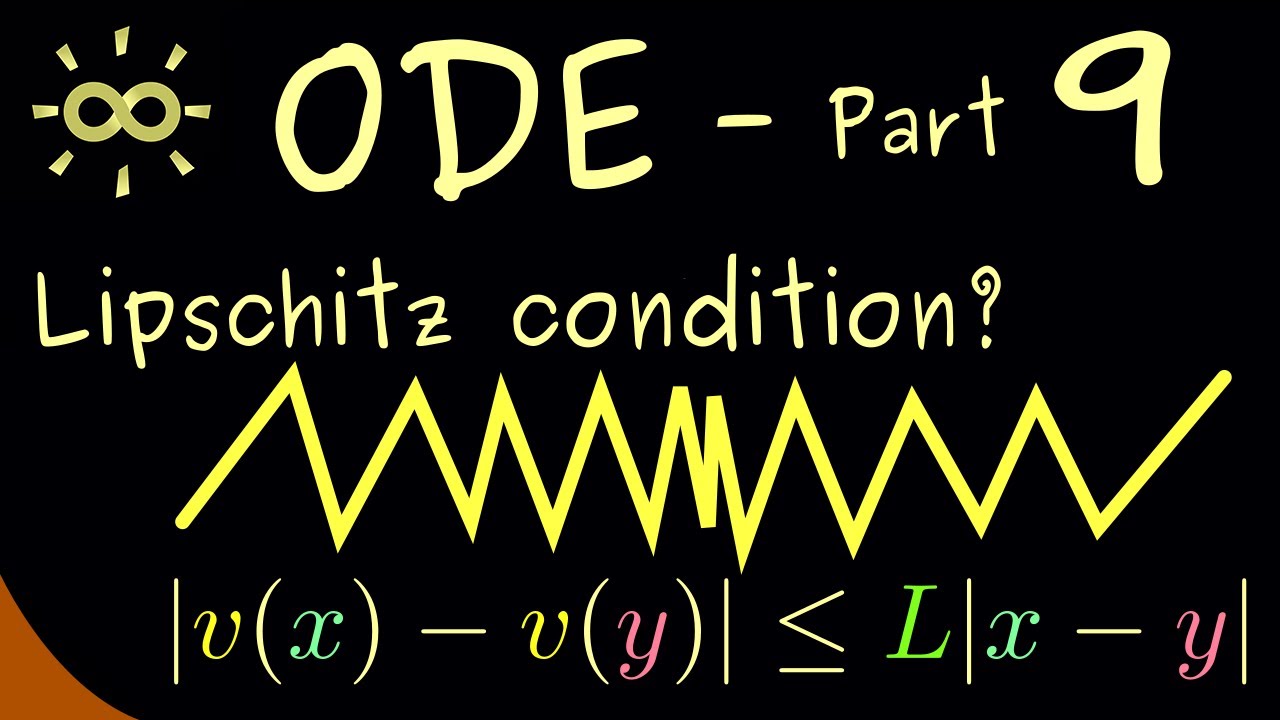
Ordinary Differential Equations 9 | Lipschitz Continuity [dark version]

Related Tags

Differential Equations Mathematics Existence & Uniqueness Slope Fields General Solutions Partial Derivatives Continuous Functions Educational Content Math Tutorial College Level