Statics: Crash Course Physics #13

CrashCourse
23 Jun 201609:08
EducationalLearning
32 Likes 10 Comments

TLDRThis script discusses the physics of statics and equilibrium. It explains that objects not accelerating are in equilibrium, with balanced forces and torques. Using a ladder example, it shows how to calculate unknown forces by setting net force and torque equal to zero. It then discusses stress, strain, Young's modulus, and how materials deform under different types of stress. Key concepts covered include tensile, compressive, shear stress, and pressure. The summary highlights how physics principles are applied in engineering to design stable structures.

Takeaways
  • 😀 Statics is the study of objects that are not accelerating, which helps explain how buildings and bridges stay stable.
  • 😲 Objects in equilibrium have balanced forces and torques acting on them.
  • 📏 You can calculate unknown forces on objects using distances and torques.
  • 😮Objects deform based on the strength and type of force applied.
  • 🤯 Tensile and compressive stress cause stretching and compressing.
  • 🚨 Too much force can permanently deform or break an object.
  • 📐 Shear stress causes sliding deformation parallel to the force.
  • 💦 Pressure causes shrinkage by compacting an object's volume.
  • 📊 The modulus values indicate a material's resistance to deformation.
  • 🏗 Engineering design uses statics to create stable structures.
Q & A

  • What is statics?

    -Statics is the study of how objects behave when they are not accelerating, either because they are completely still or moving at a constant velocity.

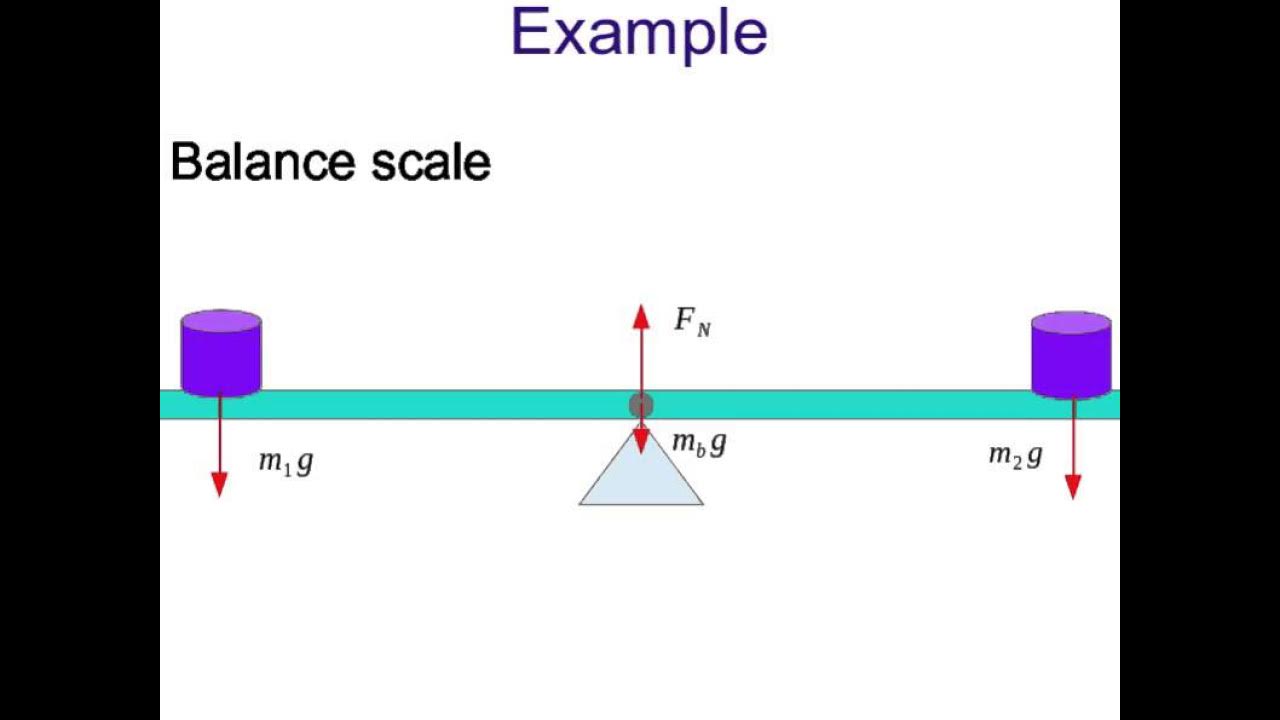
  • What two conditions must be met for an object to be in equilibrium?

    -For an object to be in equilibrium, the net force on it must equal zero and the net torque must also equal zero.

  • How can you use torque to calculate the force on a ladder leaning against a wall?

    -Choose the point where the ladder touches the floor as the axis of rotation. Then set the clockwise torque from gravity equal to the counterclockwise torque from the wall force. Use this to calculate the force from the wall.

  • What are the three main ways that forces can affect an object's shape?

    -The three main ways forces affect an object's shape are: 1) Changing its length through tensile or compressive stress, 2) Deforming it through shear stress, and 3) Changing its volume through pressure.

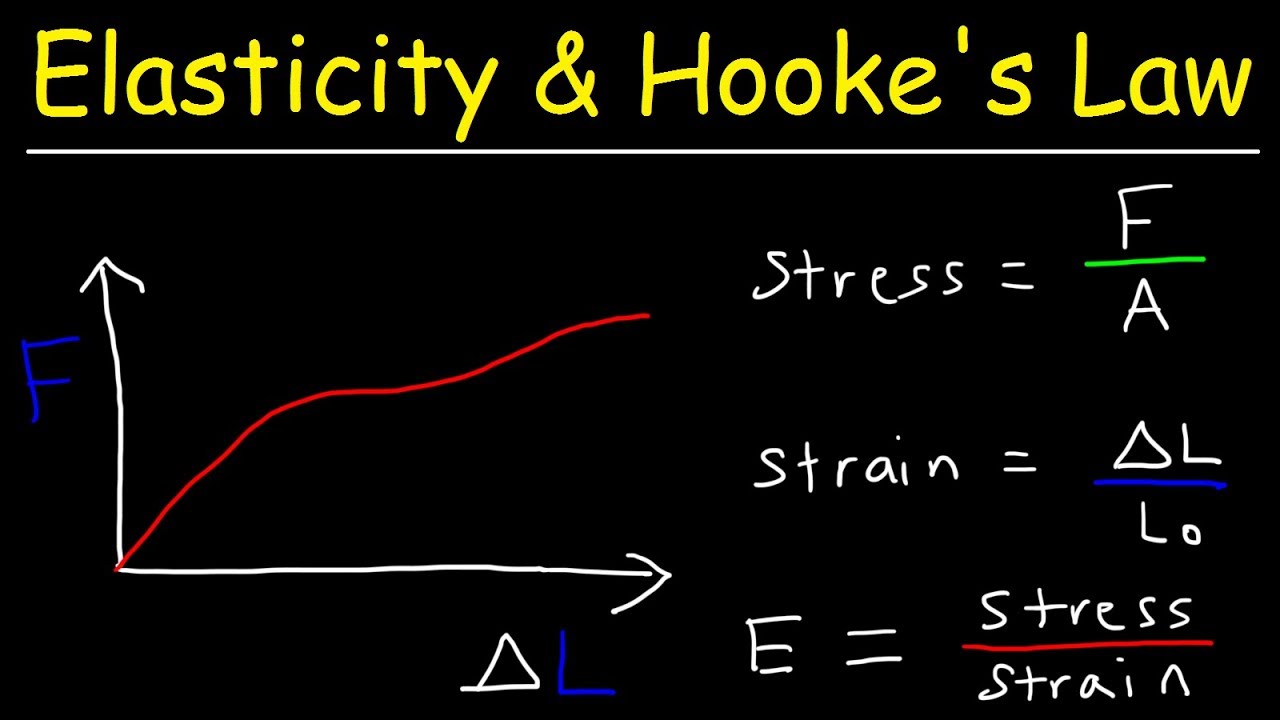
  • What is Young's modulus?

    -Young's modulus is a number that tells you how stiff or elastic a material is. It relates stress to strain in an object and allows you to predict how much an object will stretch or compress.

  • What causes shear stress?

    -Shear stress occurs when parallel forces are applied across an object, causing its layers to slide over one another. For example, when you push a book along a table.

  • How does the original size of an object affect how much it deforms?

    -The larger an object's original length, area, or volume, the more it will deform when a force is applied. Longer objects stretch and compress more. Objects with larger cross-sectional areas deform less.

  • What is stress?

    -Stress refers to a force applied over an area. It is calculated by dividing a force by the cross-sectional area perpendicular to that force.

  • How is strain calculated?

    -Strain is calculated by dividing an object's change in length by its original length. So it represents the deformation as a fraction of the original size.

  • Why is this physics important for structural engineers?

    -Understanding concepts like stress, strain, and deformation allows structural engineers to predict how buildings, bridges, and other objects will respond to forces. This helps them design safe, stable structures.

Outlines
00:00
😲 How statics keeps you safe by calculating forces

Introduces statics, the physics of objects that aren't accelerating, and how engineers use it to design safe buildings and bridges by calculating stresses and strains. Explains key concepts like equilibrium, net force, torque, and shows an example of calculating forces on a ladder against a wall.

05:01
😵‍💫 How materials deform under stress and pressure

Explains how materials stretch, compress, or shear when forces are applied, using concepts like tensile stress, compressive stress, shear stress, Young's modulus, shear modulus, pressure, and bulk modulus. Shows equations for how deformation depends on original size, force, area, and the material's inherent resistance.

Mindmap
Keywords
💡Statics
Statics is the branch of physics that studies objects at rest or moving at constant velocity. It explains how stationary or non-accelerating objects behave when forces are applied to them. The video introduces statics as the science that determines the stability of buildings and bridges. For example, statics allows engineers to calculate where supports should be placed in a building.
💡Equilibrium
Equilibrium refers to the state where the net force and net torque on an object are zero. This means that all the forces and torques acting on the object balance each other out, so there is no net acceleration. The video explains that an object like a ladder leaning against a wall is in equilibrium, allowing us to calculate the forces acting on it.
💡Torque
Torque is the rotational equivalent of force. It causes objects to rotate around an axis. The video states that for an object to be in equilibrium, the net torque on it must be zero, in addition to the net force. Torque helps determine the forces acting on a ladder in equilibrium.
💡Stress
Stress refers to the internal forces in an object that resist an applied external force. It can cause deformation. The video introduces concepts like tensile stress, compressive stress and shear stress that act on objects. Stress depends on factors like force and cross-sectional area.
💡Strain
Strain is a measure of deformation in an object under stress. It refers to the change in an object's length or volume compared to its original dimensions. Strain increases with more applied stress according to the equations introduced in the video.
💡Young's modulus
Young's modulus quantifies the stiffness of a material. It appears in the equations for tensile and compressive strain. A higher Young's modulus means the material is harder to deform elastically. It helps determine how much an object will compress or stretch.
💡Shear modulus
The shear modulus measures a material's resistance to shear stress. It is used in the equation for shear strain. A high shear modulus means the material does not deform as much under shear stress. The video relates this to a book sliding over a table.
💡Bulk modulus
Bulk modulus measures a material's resistance to changes in volume under pressure. Materials with higher bulk modulus will shrink less when compressed from all directions uniformly. It appears in the equation for volumetric strain.
💡Elastic zone
The elastic zone is the stress range where an object will deform but return to its original shape after the stress is removed. The video states that engineers want to keep stresses within this zone to prevent permanent damage.
💡Plastic zone
The plastic zone refers to higher stresses that permanently deform an object so that it does not return to its original shape once the load is removed. The video contrasts this with the elastic zone.
Highlights

The study used a novel combination of MRI and PET imaging to examine brain connectivity.

Researchers found a strong correlation between functional connectivity in the prefrontal cortex and measures of cognitive flexibility.

The experimental group showed increased functional connectivity between prefrontal and parietal regions after cognitive training.

Cognitive flexibility was significantly improved in the training group compared to controls.

Changes in functional connectivity predicted improvements in task switching performance.

The study provides evidence for training-induced neuroplasticity in brain networks involved in cognitive control.

Results suggest functional connectivity could be used as a biomarker to track cognitive training progress.

Cognitive training may aid rehabilitation and improvement of executive function in certain patient groups.

The brain training program was adaptive and adjusted difficulty based on individual performance.

The study had a relatively small sample size and lacked longer-term follow-up.

More research is needed to determine optimal training approaches for improving cognitive flexibility.

The control group engaged in regimented computerized activities without adaptivity or cognitive engagement.

The cognitive training group showed generalized improvements on untrained executive function tasks.

The combination of neuroimaging and behavioral measures provides compelling evidence for training effects.

Overall, the study makes an important contribution to our understanding of neuroplasticity and cognitive training.

Transcripts

Browse More Related Video

How to solve Rotational Equilibrium Problems

Static Equilibrium: concept

Elasticity & Hooke's Law - Intro to Young's Modulus, Stress & Strain, Elastic & Proportional Limit

Physics 15 Torque (23 of 25) More Examples: 5 F(A)=? F(B)=? of Simple Truss

Ladder Example for Static Equilibrium

Understanding How Torque Works

Rate This

5.0 / 5 (0 votes)

Thanks for rating: