AP Physics 1: Rotational Dynamics Review

Flipping Physics
28 Mar 201508:37
EducationalLearning
32 Likes 10 Comments

TLDRIn this engaging lesson, Mr. P delves into the fundamentals of rotational dynamics as covered in the AP Physics 1 exam. Starting with the concept of torque, he explains its relation to angular acceleration and uses the everyday example of opening a door to illustrate the principle. The discussion then moves to the rotational form of Newton's second law, introducing the moment of inertia as a measure of resistance to angular acceleration. The lesson continues with an exploration of rotational kinetic energy, the conservation of mechanical energy in rolling objects, and the equations for the velocity of a rolling object's center of mass. Concluding with angular momentum and impulse, Mr. P emphasizes their vector nature and the significance of these concepts in understanding rotational dynamics. The lesson is a comprehensive review that effectively bridges theoretical concepts with practical applications.

Takeaways
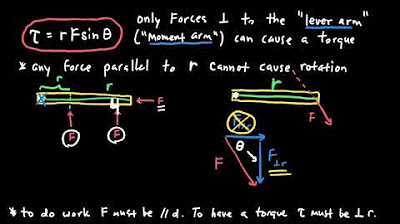
  • 📌 Torque (τ) is the ability to cause an angular acceleration of an object and is calculated as the lever arm (r) multiplied by the force.
  • 🚪 Opening a door efficiently demonstrates the principle of torque; applying force further from the hinge (axis of rotation) results in greater torque.
  • 🔢 The moment arm's effectiveness is maximized when force is applied at a 90-degree angle, as the sine of the angle (θ) equals one, leading to the maximum moment arm (r).
  • 🏷️ Torque is a vector and is measured in Newton meters (Nm), which is equivalent to joules but is kept separate to distinguish it from energy.
  • 🔄 The rotational form of Newton's second law states that the net torque (τ) equals the moment of inertia (I) times the angular acceleration (α).
  • 🥊 Moment of inertia (I) is a measure of an object's resistance to angular acceleration and depends on the mass distribution relative to the axis of rotation.
  • 🏓 The moment of inertia for a solid sphere is calculated as (2/5) times the mass times the radius squared.
  • 💰 Rotational kinetic energy is given by (1/2) times the moment of inertia times the square of the angular velocity (ω) and is measured in joules (J).
  • 🌐 When an object rolls without slipping, it has both translational and rotational kinetic energy, and a larger moment of inertia results in more rotational kinetic energy and less translational kinetic energy.
  • 🚀 The velocity of the center of mass of a rolling object without slipping is the radius of the object times its angular velocity.
  • 🔄 Angular momentum is the rotational equivalent of linear momentum and is calculated as the moment of inertia times the angular velocity (ω), with units of kilograms times meters squared divided by seconds (kg·m²/s).
Q & A

  • What is the symbol for torque?

    -The symbol for torque is a lowercase tau (τ).

  • What does torque represent in physics?

    -Torque represents the ability to cause an angular acceleration of an object.

  • What is the equation for torque?

    -The equation for torque is the lever arm (or moment arm) multiplied by the force.

  • Why is it easier to open a door when you apply force farther from the hinge?

    -Applying force farther from the hinge increases the moment arm (r perpendicular), which results in a larger torque and therefore a larger angular acceleration, making the door easier to open.

  • What is the most efficient angle to apply force on a door?

    -The most efficient angle to apply force on a door is at 90 degrees to the door, as the sine of 90 degrees equals one, making the moment arm equal to r, which is the maximum value.

  • How is the direction of torque described?

    -The direction of torque is described using clockwise and counterclockwise, as the AP Physics 1 curriculum does not include the right-hand rule.

  • What are the dimensions for torque?

    -The dimensions for torque are Newton meters (N·m), which is equivalent to joules (J), but torque is kept in Newton meters to distinguish it from energy.

  • What is the rotational form of Newton's second law?

    -The rotational form of Newton's second law states that the net torque is equal to the moment of inertia (I) times the angular acceleration.

  • How is the moment of inertia defined?

    -The moment of inertia (I) is a measure of an object's resistance to angular acceleration and is defined as the sum of the mass of each particle times the square of the distance each particle is from the axis of rotation.

  • What is the relationship between moment of inertia and rotational kinetic energy?

    -The moment of inertia (I) relates to rotational kinetic energy (KE) through the equation KE = 1/2 * I * angular velocity squared (ω^2). A larger moment of inertia means a larger rotational kinetic energy when an object rolls without slipping.

  • How can the velocity of the center of mass of a rolling object be calculated?

    -The velocity of the center of mass (V_cm) of a rolling object without slipping can be calculated using the equation V_cm = radius of the object * angular velocity (ω).

  • What is angular momentum and how is it calculated?

    -Angular momentum is a measure of the rotational motion of an object and is calculated as the moment of inertia (I) times angular velocity (ω). It has the dimensions of kilograms times meters squared divided by seconds (kg·m^2/s).

  • What is angular impulse and how does it relate to torque and angular momentum?

    -Angular impulse is the change in angular momentum of an object and is equal to the net torque applied to the object multiplied by the change in time during a collision. It has the same dimensions as angular momentum, which are kilograms times meters squared over seconds (kg·m^2/s).

Outlines
00:00
📚 Introduction to Rotational Dynamics

This paragraph introduces the topic of rotational dynamics, which is a key area in the AP Physics 1 exam. The discussion begins with the concept of torque, its symbol (lowercase tau), and its definition as the ability to cause an angular acceleration of an object. The equation for torque is explained as the product of the lever arm (or moment arm) and the force applied. The real-world example of opening a door is used to illustrate how the position of the applied force affects the torque and consequently the ease of opening the door. The concept of moment arm and its relationship with the axis of rotation is detailed, emphasizing the maximum torque at a 90-degree angle. The paragraph also touches on the vector nature of torque and the lack of inclusion of the right-hand rule in the AP Physics 1 curriculum. The dimensions of torque (Newton meters) are related to joules, but the distinction between the two is clarified. The paragraph transitions into a discussion of the rotational form of Newton's second law, defining the moment of inertia as a measure of an object's resistance to angular acceleration. The formula for calculating the moment of inertia for a system of particles is provided, and examples of moments of inertia for specific rigid objects are discussed.

05:01
🚀 Rotational Kinetic Energy and Rolling Dynamics

The second paragraph delves into the concepts of rotational kinetic energy and the dynamics of an object rolling without slipping. It explains how both translational and rotational kinetic energies are gained as an object rolls down an incline, with a focus on the conservation of mechanical energy. The relationship between an object's moment of inertia and its rotational kinetic energy is explored, highlighting that a larger moment of inertia results in more rotational kinetic energy and less translational kinetic energy. The discussion continues with the impact of an object's moment of inertia on the time it takes to reach the bottom of an incline and its resulting linear velocity. The equation for the velocity of the center of mass of a rolling object is introduced and compared to the equation for tangential velocity. The paragraph concludes with an explanation of angular momentum, its vector nature, and its dimensions. The concept of angular impulse is introduced, relating it to the change in angular momentum during a collision and its dimensions. The review of rotational dynamics is summarized, and the viewer is directed to further resources for AP Physics 1 exam preparation.

Mindmap
Keywords
💡Torque
Torque, symbolized by the lowercase tau, is a measure of the force's tendency to cause an object to rotate around an axis. In the context of the video, it is defined as the product of the lever arm (or moment arm) and the applied force. The concept is illustrated through the everyday action of opening a door, where applying force farther from the hinge (axis of rotation) results in greater torque and easier opening. Torque is a vector quantity, having both magnitude and direction, and is measured in Newton meters, which is equivalent to joules, although it's important to note the distinction between the two due to their different physical meanings.
💡Angular Acceleration
Angular acceleration is the rate of change of angular velocity per unit time. It is a measure of how quickly an object's rotational speed is changing. In the video, angular acceleration is directly related to the concept of torque, as it is the result of torque acting on an object. The greater the torque applied to an object, the higher its angular acceleration.
💡Lever Arm
The lever arm, also known as the moment arm, is the perpendicular distance from the axis of rotation to the line of action of the force applied. It is a critical factor in calculating torque, as it multiplies the force applied to determine the amount of torque. The lever arm concept is used in the video to explain why applying force further from the hinge of a door results in a larger torque and easier rotation.
💡Moment of Inertia
Moment of inertia, often denoted by the symbol I, is a measure of an object's resistance to rotational motion or its tendency not to rotate. It depends on both the mass of the object and the distribution of that mass relative to the axis of rotation. In the video, the moment of inertia is described as the 'rotational mass' and is calculated by summing the product of the mass of each particle in a system and the square of its distance from the axis of rotation.
💡Rotational Kinetic Energy
Rotational kinetic energy is the energy an object possesses due to its rotation. It is calculated as half the product of the moment of inertia and the square of the angular velocity. This concept is directly related to the object's resistance to angular acceleration, as described by the moment of inertia. In the context of the video, rotational kinetic energy is a part of the mechanical energy of an object rolling without slipping, which also includes translational kinetic energy.
💡Rolling Without Slipping
Rolling without slipping refers to a scenario where an object rolls down an incline and its point of contact with the incline does not slide, maintaining a pure rolling motion. This concept is important in the conservation of mechanical energy, as the object's gravitational potential energy is converted into both rotational and translational kinetic energy. The video explains how the moment of inertia affects the distribution of these energies.
💡Angular Momentum
Angular momentum is a measure of the rotational motion of an object and is given by the product of the moment of inertia (rotational mass) and the angular velocity. Like linear momentum, angular momentum is a vector quantity, having both magnitude and direction. It is conserved in the absence of external torques and is an important concept in understanding the dynamics of rotating systems.
💡Angular Impulse
Angular impulse is the change in angular momentum of an object, which occurs during a collision or an impulsive force application. It is equal to the net torque applied to the object multiplied by the time interval during which the force is applied. Angular impulse is a measure of the effectiveness of the force in causing a change in the object's rotational motion and is a fundamental concept in collision dynamics.
💡Newton's Second Law - Rotational Form
The rotational form of Newton's second law states that the net torque acting on an object is equal to the product of its moment of inertia (rotational mass) and its angular acceleration. This law extends the linear form of Newton's second law to rotational motion, highlighting the relationship between torque, moment of inertia, and angular acceleration.
💡Conservation of Mechanical Energy
The conservation of mechanical energy states that in a closed system with no external forces, the total mechanical energy (the sum of potential and kinetic energy) remains constant. In the context of the video, this principle is applied to an object rolling down an incline without slipping, where the initial gravitational potential energy is converted into a combination of rotational and translational kinetic energy.
Highlights

Torque is defined as the ability to cause an angular acceleration of an object.

The equation for torque is the lever arm (moment arm) multiplied by the force.

Applying force far from the hinge (axis of rotation) is more effective for opening a door, demonstrating the principle of torque.

The moment arm's efficiency is maximized when force is applied at a 90-degree angle, as the sine of the angle equals one.

Torque is a vector and its direction can be described as clockwise or counterclockwise.

Torque is measured in Newton meters, which is equivalent to joules, but they are kept separate to distinguish between vector and scalar quantities.

The rotational form of Newton's second law states that the net torque is equal to the moment of inertia times the angular acceleration.

The moment of inertia, or rotational mass, is a measure of an object's resistance to angular acceleration.

The moment of inertia for a system of particles is the sum of the mass of each particle times the square of its distance from the axis of rotation.

The moment of inertia for a solid sphere around its center of mass is calculated as 2/5 times the mass times the radius squared.

Rotational kinetic energy is calculated as 1/2 times the moment of inertia times the angular velocity squared, and is measured in joules.

An object rolling without slipping gains both translational and rotational kinetic energy, with a larger moment of inertia leading to more rotational kinetic energy.

The larger the moment of inertia of an object, the more time it takes to reach the bottom of an incline, resulting in a smaller linear velocity.

The velocity of the center of mass of a rigid object rolling without slipping is the radius of the object times its angular velocity.

Angular momentum is calculated as the moment of inertia times angular velocity, and like linear velocity, angular velocity is also a vector.

Angular impulse is equal to the change in angular momentum, which is the net torque applied during a collision multiplied by the change in time.

Angular impulse has the same dimensions as angular momentum, measured in kilograms times meters squared over seconds.

Transcripts

Browse More Related Video

AP Physics 1 - Rotational Dynamics

College Physics 1: Lecture 25 - Moment of Inertia and Rotational Dynamics

AP Physics 1 Torque and Rotational Motion Review

AP Physics 1 - 10 Minute Recap

2022 Live Review 6 | AP Physics C: Mechanics | Rotational Dynamics and Angular Momentum

2022 Live Review 7 | AP Physics 1 | Understanding Torque and Rotational Motion

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsEducationRotationalDynamicsAPExamReviewTorqueExplanationAngularAccelerationMomentInertiaKineticEnergyMechanicalEnergyFlippingPhysicsLearningFun