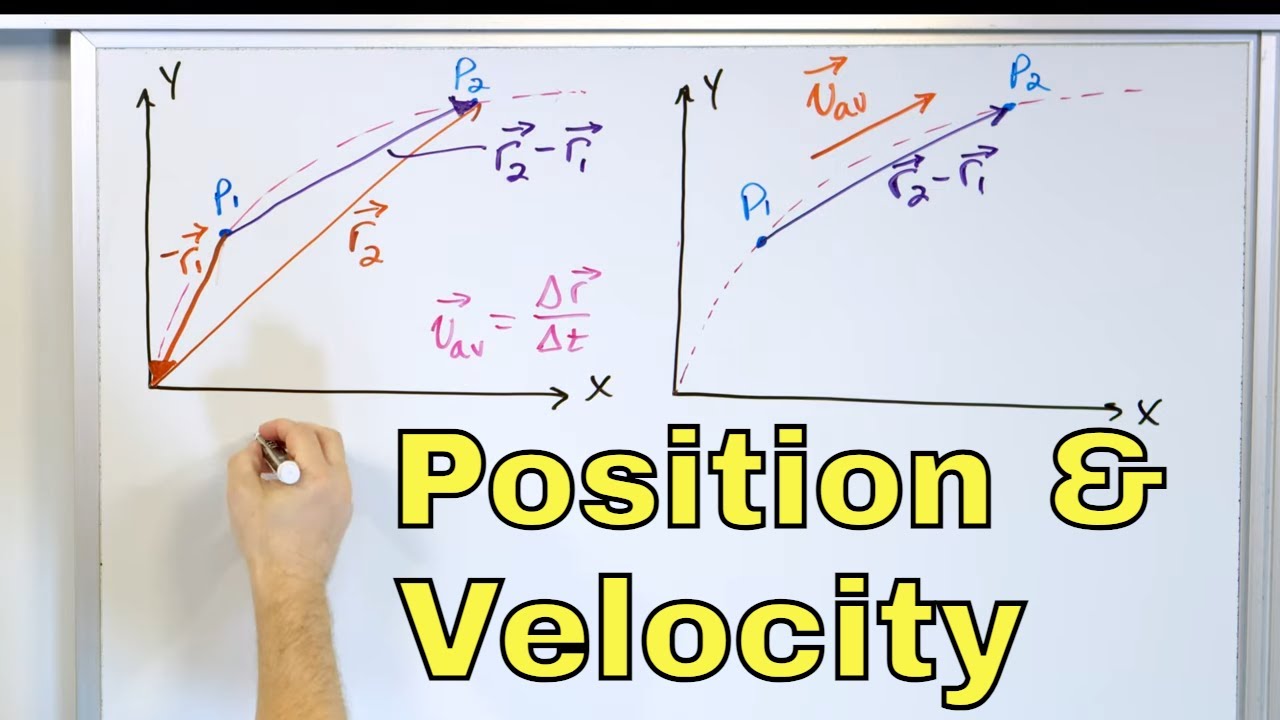
2. Vectors in Multiple Dimensions

Professor Ramamurti Shankar begins the lecture by summarizing the previous session's discussion on the motion of a particle in one dimension. He emphasizes the importance of understanding the basic equations that describe the particle's location and velocity over time. The professor introduces the concept of initial conditions (x_0 and v_0) and explains how they, combined with the constant acceleration (a), can predict the future behavior of the particle. The discussion then transitions to the application of these principles in more complex scenarios, such as vertical motion under gravity, highlighting the necessity of knowing both the initial conditions and the acceleration to fully describe the motion.

The lecture continues with an exploration of motion in higher dimensions, specifically two and three dimensions. The professor explains that while we live in a three-dimensional world, understanding two-dimensional motion is crucial as it introduces the concept of vectors. Vectors are used to describe displacement, incorporating both magnitude and direction. The professor discusses the addition of vectors and introduces the idea of unit vectors (i and j), which are used to express any vector in terms of its components along the x and y axes. The lecture also touches on the concept of a null vector and the multiplication of vectors by scalars, setting the foundation for further discussions on vector operations.

This paragraph delves deeper into the rules of vector addition, emphasizing the commutative nature of vector addition but noting that the composition of vector operations is not always commutative. The professor introduces the zero vector, which has no magnitude and does not affect the vector to which it is added. The concept of scalar multiplication is further explained, with examples illustrating how multiplying a vector by a number stretches or compresses it proportionally. The idea of a negative vector is also introduced, which is essentially the original vector pointing in the opposite direction.

The professor discusses the transformation of vector components when switching between different coordinate systems. By introducing primed unit vectors (i' and j'), the lecture explains how the components of a vector (Ax and Ay) change when a new set of axes is used. The relationship between the old and new components is derived using trigonometry, highlighting the importance of understanding how the orientation of axes affects the representation of vectors. The invariance of the vector's length under coordinate transformations is also emphasized, reinforcing the concept of an invariant quantity.

The lecture introduces the concept of taking derivatives of vectors with respect to time, leading to the definition of the velocity vector. By differentiating the position vector, the velocity vector is obtained, which describes the rate of change of the position. The professor then presents a specific example of a particle moving in a circular path, using trigonometric functions to express the position vector. The velocity vector is derived from this motion, and its components are analyzed. The lecture concludes with the calculation of the tangential speed of the particle in circular motion and introduces the concept of angular velocity (ω), which characterizes the rate of change of the angle over time.

The professor continues the discussion on circular motion by analyzing the acceleration of a particle moving in a circle. By taking the second derivative of the position vector, the acceleration vector is found to be directed towards the center of the circle, with a magnitude of v^(2)/r, where v is the tangential speed and r is the radius of the circle. This result is significant as it shows that even though the speed is constant, there is an acceleration due to the continuous change in direction. The concept is further explained with the help of a car moving on a racetrack, emphasizing that circular motion inherently involves acceleration towards the center of the circle.

The lecture addresses the relationship between a particle's position, velocity, and acceleration in motion. The professor explains that the position vector can be used to derive both the velocity and acceleration vectors through differentiation. The example of a particle moving in a circle is used to illustrate this, with the acceleration vector found to point towards the center of the circle. The lecture also touches on the concept of velocity addition, explaining how the velocity of an object in a moving frame can be found by adding the frame's velocity relative to a stationary observer. This principle is applied to the scenario of a particle moving in a circle, showing that the acceleration is always directed towards the center of the circle, regardless of the particle's speed.

The lecture concludes with a discussion on projectile motion, focusing on the optimal angle for launching a projectile to achieve maximum range. The professor presents the equations of motion for a projectile launched at an angle θ and explains how to find the range and the time of flight. The importance of understanding trigonometry is highlighted in deriving the optimal launch angle, which is found to be 45 degrees. The lecture emphasizes the importance of not memorizing formulas but instead understanding the underlying principles and deriving the necessary equations from first principles.

In the final paragraph, the professor reflects on the presentation of physics problems and their real-world applications. He critiques the tendency of textbooks to embellish problems with unrealistic scenarios, such as a horse inside a railway carriage, to make them more engaging. The professor argues that while such examples can sometimes be helpful, they can also be distracting and increase the cost of textbooks without adding substantial value. He emphasizes the importance of understanding the fundamental concepts and being able to apply them to various situations, whether they involve point particles or more complex systems like a horse.