Translating Motion Graphs: Position, Velocity, and Acceleration - IB Physics
TLDRThis video script offers a comprehensive guide on translating between position, velocity, and acceleration graphs in physics. It explains how each graph represents different aspects of the same motion and outlines the relationships between them: velocity as the slope of the position graph, acceleration as the slope of the velocity graph, and the areas under these curves indicating changes in velocity and position. The script provides examples of translating from one graph type to another, emphasizing the importance of understanding slopes and their positive or negative values in determining the characteristics of the motion depicted in the graphs.
Takeaways
- 📈 Position, velocity, and acceleration graphs can represent the same motion, each focusing on a different aspect of motion.
- 🔄 The relationship between the graphs is defined by the slope: velocity is the slope of the position graph, and acceleration is the slope of the velocity graph.
- 📊 The area under the acceleration graph represents the change in velocity, while the area under the velocity graph represents the change in position.
- 📈📉 A steep upward slope in a position graph indicates a more positive velocity, while a steep downward slope indicates a more negative velocity.
- 🖌️ To translate curved position graphs, use tangent lines to determine the changing velocity at different points in time.
- 🔄 Translating from a velocity graph to a position graph requires considering the starting position, which is not provided by the velocity graph.
- 🔄🔄 A constant positive velocity results in a position graph with a constant positive slope, while a constant negative velocity results in a position graph with a constant negative slope.
- 📈📊 A velocity graph with a constant slope (positive or negative) translates to an acceleration graph with the same constant slope.
- 🔄📈 For translating from an acceleration graph to a velocity graph, the constant or changing slope of the acceleration graph directly translates to the slope of the velocity graph.
- 🔄📊📈 Translating between all three graphs involves understanding how the slope (and area under the curve) changes and how these changes represent the physical quantities in different aspects of motion.
Q & A
How are position, velocity, and acceleration graphs related to each other?
-Position, velocity, and acceleration graphs are related as they represent different aspects of the same motion. The velocity graph's slope corresponds to the position graph's rate of change, and the acceleration graph's slope corresponds to the velocity graph's rate of change.
What does the slope of a position graph represent?
-The slope of a position graph represents the velocity of the object at any given time. A constant slope indicates a constant velocity, while a changing slope indicates varying velocity.
How can you determine the acceleration from a velocity graph?
-Acceleration can be determined from a velocity graph by looking at the slope of the graph. The slope of the velocity graph at any point is equal to the acceleration at that moment.
What does a flat line on a velocity graph indicate?
-A flat line on a velocity graph indicates that the object has a constant velocity, meaning there is no acceleration or deceleration occurring at that time.
How can you translate a curved position-time graph into a velocity-time graph?
-To translate a curved position-time graph into a velocity-time graph, you draw tangent lines at various points along the curve of the position graph. The slope of these tangent lines represents the velocity at those specific points in time.
What is the significance of the area under the curve in an acceleration graph?
-The area under the curve in an acceleration graph represents the change in velocity over the time period covered by the graph. It can be used to determine the total increase or decrease in velocity.
How can you translate a velocity graph back to a position graph?
-To translate a velocity graph back to a position graph, you consider the slope of the velocity graph (which represents acceleration) and integrate it over time to find the position changes. The starting point of the position graph is not determined by the velocity graph, so it can be assumed arbitrarily.
What does a negative slope on an acceleration graph indicate?
-A negative slope on an acceleration graph indicates that the object is decelerating or experiencing a decrease in velocity over the time period represented by that section of the graph.
What is the relationship between the area under the curve of a velocity graph and the change in position?
-The area under the curve of a velocity graph is directly related to the change in position. It represents the total distance traveled by the object over the time period covered by the graph, accounting for both direction and magnitude of the velocity.
How can you determine the initial position or velocity of an object from its graphs?
-The initial position or velocity of an object cannot be determined solely from its velocity or position graph, as these graphs represent rates of change or slopes. Additional information or assumptions are needed to establish the starting point.
What is the significance of the direction of the slope in the context of velocity and acceleration graphs?
-The direction of the slope in velocity and acceleration graphs indicates whether the object is speeding up (positive slope) or slowing down (negative slope) for velocity, and whether it is accelerating (positive slope) or decelerating (negative slope) for acceleration.
Outlines
📈 Introduction to Translating Motion Graphs
This paragraph introduces the concept of translating between different types of motion graphs: position, velocity, and acceleration. It explains that these graphs represent the same motion from different perspectives and emphasizes the relationship between them. The velocity graph is described as the slope of the position graph, and the acceleration graph as the slope of the velocity graph. The paragraph sets the stage for examples that will demonstrate how to translate between these graphs effectively.
🏃♂️ Translating Position to Velocity Graphs
The focus of this paragraph is on translating position time graphs to velocity time graphs and vice versa. It explains how to use tangent lines to determine the slope (velocity) at various points on the position graph. The paragraph provides examples of how different slopes on the position graph correspond to different velocities over time. It also touches on how to translate a constant velocity back to a position graph, emphasizing that the starting position can vary.
🔄 Translating Velocity to Position and Acceleration Graphs
This paragraph delves into the process of translating velocity graphs to both position and acceleration graphs. It describes how a changing velocity indicates a changing slope on the position graph, resulting in a curved line. The paragraph also explains how the slope of the velocity graph at any given moment is the acceleration at that moment. Examples are provided to illustrate the translation process, including how to handle negative velocities and the impact on position and acceleration graphs.
📊 Comprehensive Graph Translation Examples
The final paragraph provides a comprehensive overview of translating between all three types of motion graphs. It reiterates the rules for translating position to velocity and velocity to acceleration graphs, and vice versa. The paragraph concludes with a series of examples that demonstrate the application of these rules in translating complex motion scenarios across different graph types, reinforcing the understanding of the interrelationship between position, velocity, and acceleration graphs.
Mindmap
Keywords
💡Position Graph
💡Velocity Graph
💡Acceleration Graph
💡Slope
💡Tangent Lines
💡Area Under the Curve
💡Constant Velocity
💡Deceleration
💡Projectile Motion
💡Translation of Graphs
Highlights
The video discusses translating between three types of motion graphs: position, velocity, and acceleration.
Multiple different graphs can represent the same motion using position, velocity, and acceleration graphs.
The position-time graph represents how an object's position changes over time.
The velocity-time graph shows the object's velocity over time, starting large and going down to zero.
The acceleration-time graph reflects the object's acceleration over time, with negative acceleration indicating slowing down.
The relationship between the three graphs is defined by velocity being the slope of the position graph and acceleration being the slope of the velocity graph.
The area under the curve of an acceleration graph represents the change in velocity, while the area under the velocity graph represents the change in position.
The video provides examples of translating between position and velocity graphs and back.
A constant velocity is represented by a flat line on the position graph, indicating a constant slope.
Changing slopes on the position graph indicate changing velocities, which are translated into a velocity-time graph with varying slopes.
Curved position-time graphs require understanding the changing slopes at different points, which is done by drawing tangent lines.
The velocity graph can be translated back to a position graph by understanding the constant or changing slopes indicate the object's position changes over time.
Translating from velocity graphs to acceleration graphs involves understanding that the slope of the velocity graph equals the acceleration.
The video concludes by providing examples of translating between all three types of graphs, emphasizing the importance of understanding the relationship between the graphs.
The process of translating graphs is akin to translating a language, expressing the same motion or idea through different graphical representations.
The video includes a chart to remind viewers when slopes are positive, negative, larger, or smaller, aiding in the translation process.
Transcripts
Browse More Related Video

Velocity Time Graphs, Acceleration & Position Time Graphs - Physics
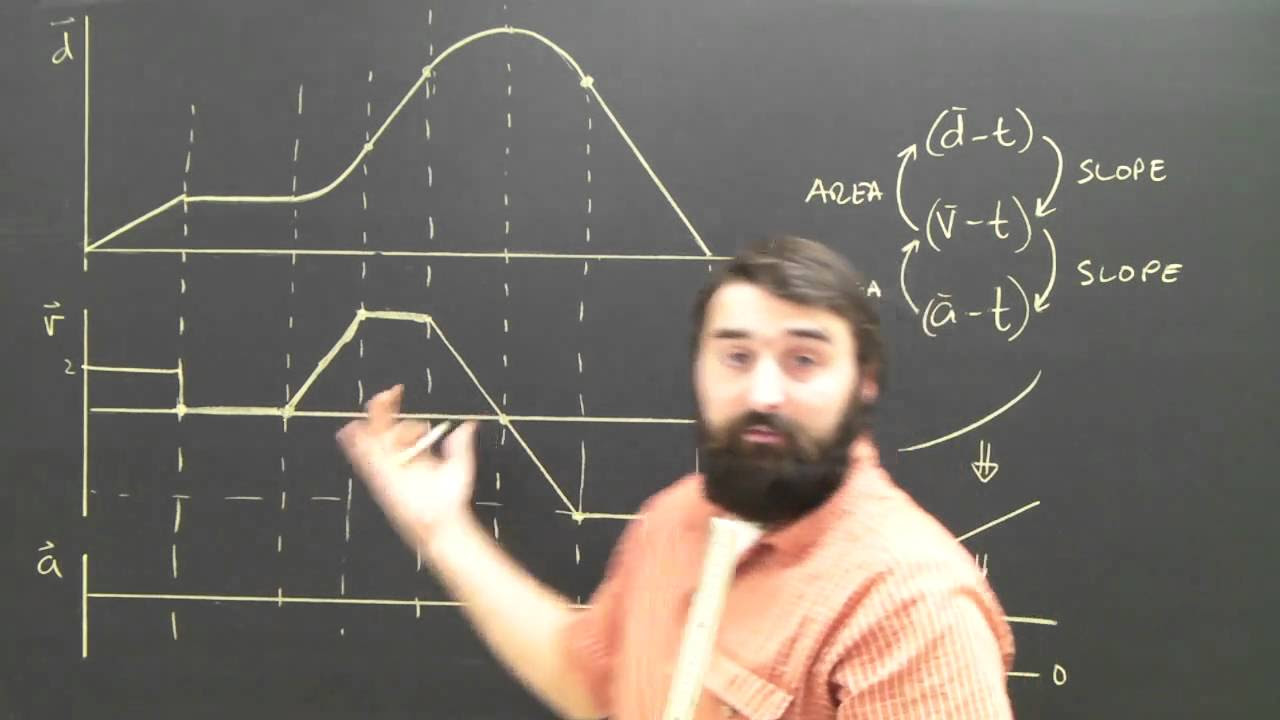
Distance (position) to Velocity Time Graph Physics Help

Visualizing Motion Using Tape Charts and Motion Graphs | SCIENCE 7 QUARTER 3 MODULE 2 WEEK 3
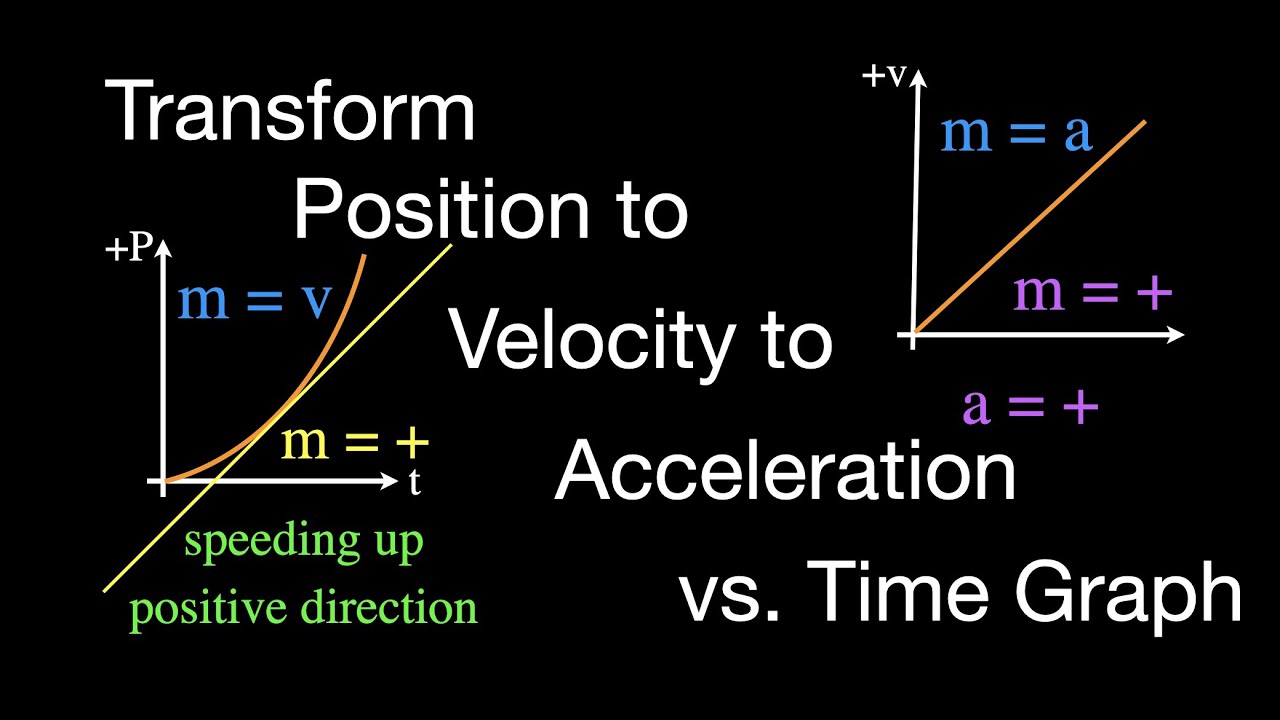
Motion Graphs: Transforming Position to Velocity to Acceleration vs Time
Motion Graphs - AP Physics 1: Kinematics Review Supplement
Position vs Time, Velocity vs Time & Acceleration vs Time Graph (Great Trick to Solve Every Graph!!)
5.0 / 5 (0 votes)
Thanks for rating: