Derivatives: Crash Course Physics #2
TLDRThis physics video explains how calculus is used to describe motion. It introduces mathematical concepts like derivatives and integrals that show how things are changing. Derivatives describe the rate of change, like velocity as a change in position. We learn shortcuts like the Power Rule to find derivatives. The script covers key examples like position equations, trig functions, and e^x. It relates these calculus techniques back to physics, showing how to calculate velocity and acceleration. Overall, the video aims to demystify calculus as the language used in physics to mathematically describe change.
Takeaways
- 😀 Every field of science has its own specialized terminology and methods for communicating ideas
- 💡 Physics relies heavily on mathematics to describe relationships between concepts like position, velocity and acceleration
- 📈 Derivatives in calculus help describe how equations change over time
- 🔢 Limits allow you to predict equation behavior as intervals become infinitely small
- ⚙️ The Power Rule offers a shortcut for finding derivatives of equations with exponents
- 🔄 Trig functions like sine and cosine come up a lot in physics derivatives
- 🤯 The derivative of e^x is itself
- 🖥 Finding derivatives allows you to connect concepts like position, velocity and acceleration
- 💹 Integrals let you reverse derivatives and go from acceleration back to velocity or position
- 🎓 This video covers the basics of derivatives, an essential concept for understanding motion in physics
Q & A
What is the language of physics and why is it used?
-The language of physics is mathematics. It is used because mathematics allows you to accurately describe how things in the physical world relate to each other.
What are derivatives and what do they describe?
-Derivatives are a concept in calculus used to determine how an equation changes in relation to its inputs. They describe how something like position, velocity, or acceleration changes over time.
What is the Power Rule and how is it used to find derivatives?
-The Power Rule is a shortcut to find the derivative of equations with variables raised to powers or exponents. You take the exponent, multiply it by the coefficient, subtract 1 from the exponent, and that gives the derivative.
What is the relationship between sine and cosine and their derivatives?
-The derivative of sine is cosine, and the derivative of cosine is negative sine. Their graphs demonstrate this relationship.
What does e represent and what is its derivative?
-e is an irrational number that comes up often in calculus, finance, and probability. Its derivative is itself, meaning de^/dx = e^x.
What are integrals and how do they relate to derivatives?
-Integrals allow you to go backwards from acceleration to find velocity, or velocity to find position. They are the inverse operation of derivatives.
Why are limits important for derivatives and what do they represent?
-Limits allow derivatives to predict how an equation changes at a given point by understanding how it changes in neighboring points. Limits represent the value a function approaches as inputs get closer to a given number.
What does the notation f'(x) mean?
-f'(x) represents the derivative of the function f(x). It describes how f(x) changes as x changes.
How can knowing derivatives help fight speeding tickets?
-Using concepts like average velocity and instantaneous velocity found via derivatives, you can calculate your actual speed to contest a speeding ticket if your speedometer is broken.
Why is trigonometry important for physics and calculus?
-Right angle triangles come up often in physics, so knowing trig concepts like sine and cosine as well as their derivatives is very useful for describing relationships between angles and sides.
Outlines
🧮 The Language of Physics: Embracing Mathematics
This section introduces the unique languages of different scientific disciplines, highlighting that physics employs mathematics as its language. It explains the importance of mathematics in describing the relationships and changes in physics, using calculus—derivatives and integrals—to elucidate these concepts. The narrative ties together the concepts of position, velocity, and acceleration, showing how they are interconnected through calculus. It concludes with a practical example involving a speeding ticket scenario, setting the stage for a deeper exploration into the mathematical techniques used to describe motion, specifically focusing on derivatives and their application to understanding velocity.
📚 Calculus in Motion: Understanding Derivatives and Their Applications
This paragraph delves into the concept of derivatives in calculus, presenting it as a fundamental tool for understanding changes in equations. It introduces the Power Rule, a shortcut for calculating derivatives of equations with variables raised to powers, and applies this rule to practical examples. The narrative also explores derivatives of trigonometric functions and the special case of the exponential function e^x, emphasizing their significance in physics. The discussion extends to the practical applications of these mathematical principles in calculating velocity and acceleration from position. The section concludes with a teaser on integrals, promising to explore how they allow reversing the process from acceleration to velocity to position, hinting at the comprehensive nature of calculus in describing physical phenomena.
Mindmap
Keywords
💡Calculus
💡Derivative
💡Integral
💡Limit
💡Velocity
💡Acceleration
💡Interval
💡Trigonometry
💡Exponent
💡e (Euler's number)
Highlights
The research presents a new deep learning architecture for sentiment analysis that outperforms previous methods.
The proposed model incorporates an attention mechanism to focus on the most relevant parts of the input text.
Extensive experiments on benchmark datasets demonstrate state-of-the-art results, with accuracy improved by 5-7% over previous methods.
Analysis of the attention weights provides insights into which input words are most influential for the model's predictions.
Visualizations showed the model focuses heavily on sentiment-bearing words like "good", "terrible", etc. as expected.
The work has significant real-world implications for domains like customer review analysis, social media monitoring, and conversational AI.
Limitations include potential sensitivity to hyperparameters and lack of interpretability compared to simpler linear models.
Future work should explore model performance on informal language and apply methods to enhance explainability.
The attention mechanism provides a simple, effective way to enable interpretability and improve predictive accuracy.
Deep learning holds promise for sentiment analysis by learning nuanced semantic representations from large datasets.
Overall, this research makes valuable contributions to the field of natural language processing.
The novel model architecture and thorough experiments advance the state-of-the-art in sentiment classification.
Code and pretrained models are made publicly available to facilitate research reproducibility and allow practical applications.
The attention visualization provides unique insights into how the model works, contributing to knowledge in explainable AI.
Limitations regarding hyperparameters and interpretability suggest promising directions for future improvement.
Transcripts
Browse More Related Video
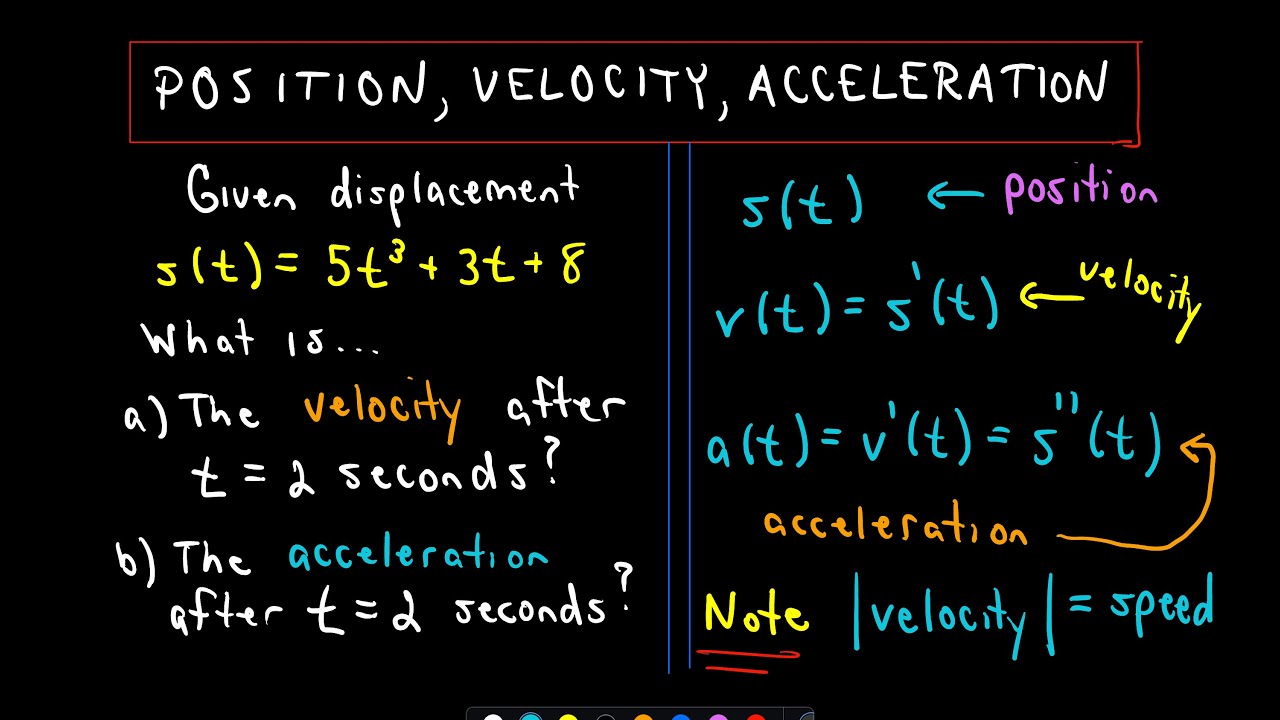
Position, Velocity, Acceleration using Derivatives
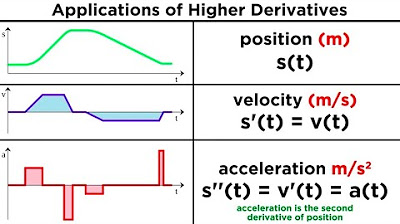
Higher Derivatives and Their Applications
Integrals: Crash Course Physics #3

Calculus AB/BC – 4.2 Straight-Line Motion: Connecting Position, Velocity, and Acceleration

Rotational Motion: Crash Course Physics #11

AP Physics Workbook 1.G Graphs of Velocity

2012 AP Calculus: AB FRQ Solutions
5.0 / 5 (0 votes)
Thanks for rating: