Rotational Motion: Crash Course Physics #11

CrashCourse
9 Jun 201608:55
EducationalLearning
32 Likes 10 Comments

TLDRThis video explains rotational motion, a type of physics distinct from but related to translational motion. It covers key concepts like angular position, velocity, acceleration, and rolling without slipping. Equations describe rotational motion using familiar terms from translational motion. For example, tangential velocity depends on radius and angular velocity. A key difference is that points on a rolling object without slipping have counteracting velocities that sum to zero, unlike translational motion. Overall, the logic of rotational motion parallels translational motion, relying on similar concepts and mathematical relationships.

Takeaways
  • 😀 Rotational motion involves rotation and spin, unlike translational motion which involves straight line movement.
  • 👉 Angular position of a rotating object is described using angles measured in radians rather than linear position using x and y coordinates.
  • ⚙️ Angular velocity measures rotational rate of change of angular position over time.
  • 🌀 Tangential velocity of a point on a rotating object depends on its angular velocity and distance from the center.
  • 🎡 Periodic rotational motion repeats after a set time period T, with frequency measured in revolutions per second.
  • 🚴 No slipping between a rolling object and surface means zero relative velocity at the point of contact.
  • 🔃 Angular acceleration describes rate of change of angular velocity over time.
  • 👆 Radial acceleration points inward on a rotating body, while tangential rotates around.
  • ⚖️ Rotational motion equations resemble those for translational motion when described using parallel terminology.
  • 🤯 A point on the edge of a rolling wheel can have zero velocity relative to the ground while the wheel rolls along.
Q & A

  • What is translational motion?

    -Translational motion refers to the movement of an object through space without it rotating.

  • How does rotational motion differ from translational motion?

    -Rotational motion involves an object rotating around an axis, unlike translational motion where the object moves through space without rotating.

  • Why is rotational motion important in the context of a football's flight?

    -The spin of a football affects how it flies through the air, making the understanding of rotational motion crucial for analyzing its trajectory.

  • What unit is primarily used to describe the angle of rotation in physics?

    -The radian is primarily used to describe the angle of rotation in physics, which is based on the radius of a circle.

  • How do you convert degrees to radians?

    -To convert degrees to radians, multiply the number of degrees by pi and divide by 180.

  • What is angular velocity?

    -Angular velocity is a measure of an object's change in angle over time, representing the rate of rotation.

  • How is tangential velocity related to angular velocity?

    -Tangential velocity is equal to the angular velocity multiplied by the radius of the path, representing the linear velocity at a point on the edge of a rotating object.

  • What does the concept of rolling without slipping refer to?

    -Rolling without slipping refers to the motion where a rotating object, like a tire, moves such that the point at the bottom does not have translational velocity, effectively not slipping on the surface.

  • How can the bottom of a wheel have a total velocity of zero while the wheel is moving?

    -The bottom of a wheel can have a total velocity of zero due to the cancellation of translational velocity and tangential velocity, which are in opposite directions, resulting in no net movement relative to the ground.

  • What are the two types of acceleration a point on a rotating object can experience?

    -A point on a rotating object can experience radial (centripetal) acceleration, directed inward, and tangential acceleration, describing the point's speed up or slow down along the path.

Outlines
00:00
🤔 Introduction to rotational motion

The first paragraph introduces rotational motion as another important type of motion, in contrast to translational motion which we've mainly focused on until now. It provides examples of rotational motion like a spinning football, explains that the physics of rotational motion is similar to translational motion, and notes some key differences like using angles instead of positions.

05:03
👉 Understanding angular position, velocity, and acceleration

The second paragraph dives deeper into the specifics of rotational motion. It explains important concepts like angular position (theta), angular velocity (omega), tangential velocity, frequency, angular acceleration (alpha), and equations relating them. It also covers special cases like rolling without slipping.

Mindmap
Keywords
💡translational motion
Translational motion refers to motion in a straight line, where an object moves from one point to another but does not rotate. This type of motion is contrasted with rotational motion, which is the main focus of the video. Examples from the script include: 'which is when something moves through space, but doesn’t rotate' and 'we’ve mostly been focusing on only one type of motion: translational motion.'
💡rotational motion
Rotational motion refers to the spinning or circular movement of an object around an axis or center point. This is the key concept explored in the video. Examples include: 'But rotational motion is also a thing -- and an important one' and 'the physics of rotational motion isn’t all that different from the physics of translational motion.'
💡angular velocity
Angular velocity measures how fast an object rotates around an axis or center point. It represents the rate of change of the angular position or angle. The video explains: 'rotational velocity is a measure of an object’s change in angle. This is known as its angular velocity.'
💡tangential velocity
Tangential velocity refers to the speed of a point on a rotating object, moving along a circular path. It depends on the object's angular velocity and radius. As explained: 'its tangential velocity will be equal to its angular velocity, times the radius.'
💡radians
Radians are a unit used to measure angles of rotation. The video introduces radians as an alternative to degrees when quantifying rotational motion. It explains: 'there’s another, unit that physicists use a lot, and it’ll be the main one we’ll use in this episode and the next. That unit is called the radian.'
💡rolling without slipping
This refers to rotational motion where the bottom point of a rolling wheel is momentarily at rest relative to the ground, so no slipping occurs. The video calls this case 'super weird' and explains why the velocity at the bottom of the wheel is zero.
💡angular acceleration
Angular acceleration measures how quickly the angular velocity changes over time. As stated: 'angular acceleration is the derivative of angular velocity.'
💡radial acceleration
Also called centripetal acceleration, radial acceleration refers to the acceleration of a point on a rotating object inward toward the center. The video relates it to angular velocity and radius.
💡tangential acceleration
Tangential acceleration measures the speeding up or slowing down of a point on a rotating object. The video explains it depends on 'the distance between the point, and the center of the object.'
💡torque
Though not explicitly mentioned, torque is a critical concept for rotational motion. It measures the twisting force that causes rotation. The physics of torque would further enrich understanding rotational motion principles covered in the video.
Highlights

Researchers developed a new method to detect exoplanets using machine learning algorithms.

The new technique can identify exoplanets missed by traditional detection methods, improving discoveries.

By training neural networks on real and simulated data, the model learns subtle patterns missed by humans.

This machine learning approach is more sensitive and can detect smaller, fainter exoplanets around distant stars.

The model was tested on Kepler space telescope data and verified known exoplanets with 96% accuracy.

Researchers plan to apply this technique to data from TESS to potentially uncover new exoplanets.

Machine learning is opening up new possibilities for analysis in astronomy and astrophysics.

The ability to process vast amounts of data beyond human capabilities allows for new discoveries.

This approach can be adapted to search for exoplanets around even more distant stars.

The use of simulations in training allows machine learning to recognize hard-to-detect planets.

Researchers are excited by the promise of AI to expand the frontiers of space science and exploration.

Machine learning models keep improving as they process more data, increasing scientific returns over time.

The combination of AI and advanced telescopes will lead to an explosion in exoplanet discoveries.

This new technique will help focus follow-up studies to characterize and learn more about exoplanets.

Machine learning is becoming an indispensable tool for 21st century astronomy and physics.

Transcripts

Browse More Related Video

AP Physics 1 - Rotational Kinematics

Rotational Kinetic Energy | Rolling Without Slipping (AP Physics 1)

Pure Rolling Motion | Physics with Professor Matt Anderson | M12-12

College Physics 1: Lecture 23 - Rotational Motion

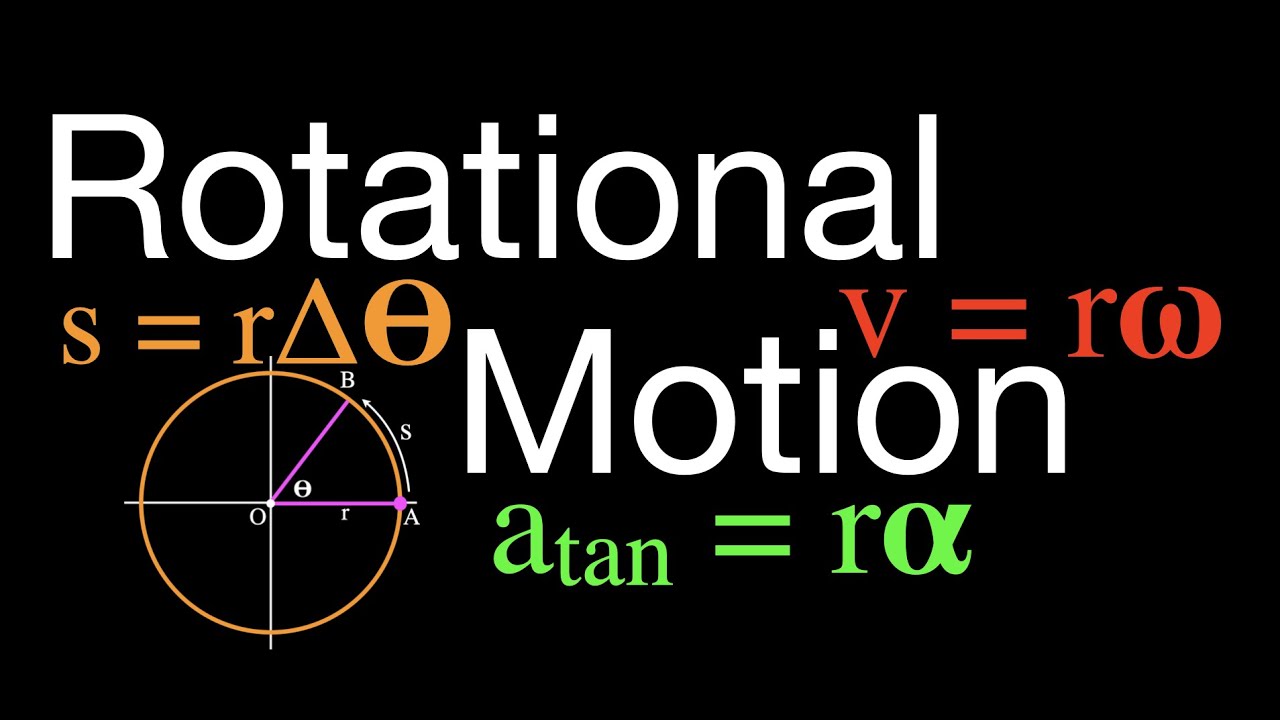
Rotational Motion: An Explanation, Angular Displacement, Velocity and Acceleration

AP Physics C: Rotational Kinematics Review (Mechanics)

Rate This

5.0 / 5 (0 votes)

Thanks for rating: