Integrals: Crash Course Physics #3
TLDRThis video explains integrals, the inverse of derivatives in calculus. Integrals represent the area under a curve on a graph. Though less straightforward than derivatives, shortcuts like the power rule help in solving integrals. Constants pose a challenge since derivatives of constants are 0, so mathematicians added 'C' to represent constants in integrals. Integrals connect concepts like acceleration, velocity and position over time. An example shows how to use integrals to calculate the height of a window by timing a ball's fall. More complex calculus concepts will build on this introduction.
Takeaways
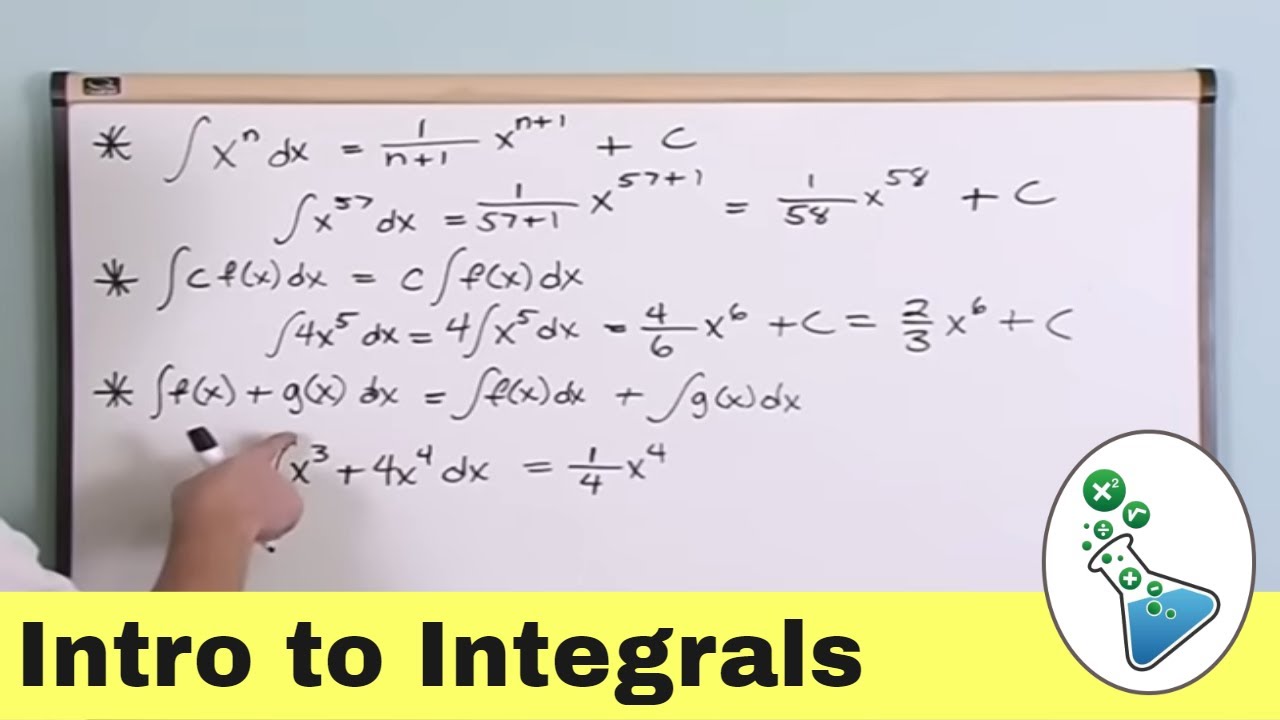
- 😀 Integrals are useful for finding the area under a curve on a graph. They are the inverse of derivatives.
- 📈 You can use integral shortcuts like the power rule to evaluate some integrals, similar to derivatives.
- 🔢 Constants pose a challenge with integrals because the derivative of a constant is 0.
- 🤔 Mathematicians add a '+C' to integrals to represent the infinite number of possible constants.
- 💡 Initial values can help determine where an integral curve intersects the vertical axis.
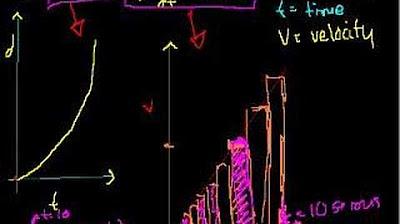
- 📊 Integrating acceleration gives velocity; integrating velocity gives position.
- 🎯 Applying integrals to a falling ball's motion derives the kinematic equations.
- 🌟 Integrals link velocity, acceleration and position - the key motion qualities.
- 🧠 Calculus integrals have many complex real-world applications beyond these basics.
- 🎥 Integrals help measure displacement and analyze changes for physics calculations.
Q & A
What is the main difference between derivatives and integrals?
-Derivatives describe the rate of change of a function, while integrals find the area under the curve of a function.
Why are integrals useful in physics and math?
-Integrals allow you to determine quantities like displacement and velocity from acceleration data by finding the area under a curve.
What is the power rule for finding integrals?
-The power rule lets you find some basic integrals by adding 1 to the exponent of the variable, dividing by that new exponent, and adding +C.
What does the +C represent when finding indefinite integrals?
-The +C represents an unknown constant that appears because an infinite number of functions can have the same derivative.
How can you eliminate the +C when finding definite integrals?
-You can eliminate +C by using the initial values of velocity, position, etc. to locate where the function intersects the vertical axis.
How did the example show the link between calculus and kinematic equations?
-By integrating acceleration twice to find velocity and position, the example derived two of the common kinematic equations.
What information did the falling ball experiment provide?
-It provided acceleration due to gravity (9.81 m/s^2), time to fall (1.7 s), and enough information to calculate final velocity and displacement.
What is the next step after learning the basics of integration?
-After learning basics like the power rule, the next step is applying integration to more complex examples over the course of university calculus.
What topics related to integrals were not covered here?
-Many additional techniques and applications of integration like substitution, by parts, volumes, differential equations etc. were not introduced.
How can I learn more about integration and calculus?
-You can take university calculus courses, read calculus textbooks, or watch more advanced lessons from sources like Khan Academy to continue learning.
Outlines
📝 Paragraph 1: Introducing integrals, the inverse of derivatives
This paragraph introduces integrals, describing them as the inverse of derivatives and explaining how they relate position, velocity and acceleration. It states that integrals represent the area under a curve on a graph, providing information about how an equation changes over time or space. The paragraph then previews shortcuts for calculating integrals and how they can describe motion using displacement curves.
📈 Paragraph 2: Using integrals and initial values to solve a physics problem
This paragraph provides an example physics problem of using integrals to calculate the height of a window by timing a falling ball. It steps through the process of going from acceleration to velocity to position using integrals, introducing the need for a constant C to account for infinite possible integral equations. It then shows how knowing the initial value can replace C. Finally, it solves the full problem by plugging in the initial values, resulting in one of the kinematic equations for displacement.
Mindmap
Keywords
💡derivatives
💡integrals
💡acceleration
💡velocity
💡position
💡area
💡power rule
💡constants
💡initial value
💡kinematic equations
Highlights
Integrals tell you the area between an equation's graph and the horizontal axis
Velocity is the integral of acceleration, and position is the integral of velocity
There are shortcuts to find integrals, like running the power rule backwards
Constants pose a problem for integrals because the derivative of a constant is 0
Mathematicians added a 'C' to integrals to represent infinite possible constants
An initial value can replace 'C' by showing where the integral crosses the vertical axis
Integrating acceleration gets velocity; integrating velocity gets position
Integrals linked acceleration to the kinematic equations for velocity and position
This showed how to calculate the height a ball falls using integrals
Only basics of calculus covered here; people study it their whole lives
Integrals find the area between a graph and the horizontal axis
Shortcuts help calculate integrals
Initial values replace the 'C' by showing where integrals cross the vertical axis
Integrating connects acceleration to velocity to position
Showed using integrals to calculate height a ball falls
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: