Video 84 - Riemann Tensor Symmetries & Identities
TLDRThis video delves into the symmetries and identities of the Riemann tensor using Riemann normal coordinates. It demonstrates the tensor's anti-symmetry in the first two and last two indices and its symmetry when swapping pairs of these indices. These properties significantly reduce the complexity of the tensor's components. The video also introduces two key identities, the first and second Bianchi identities, which further constrain the tensor's degrees of freedom, simplifying its evaluation in various dimensions.
Takeaways
- 📚 The video continues the exploration of the Riemann tensor using Riemann normal coordinates, focusing on its symmetries and identities.
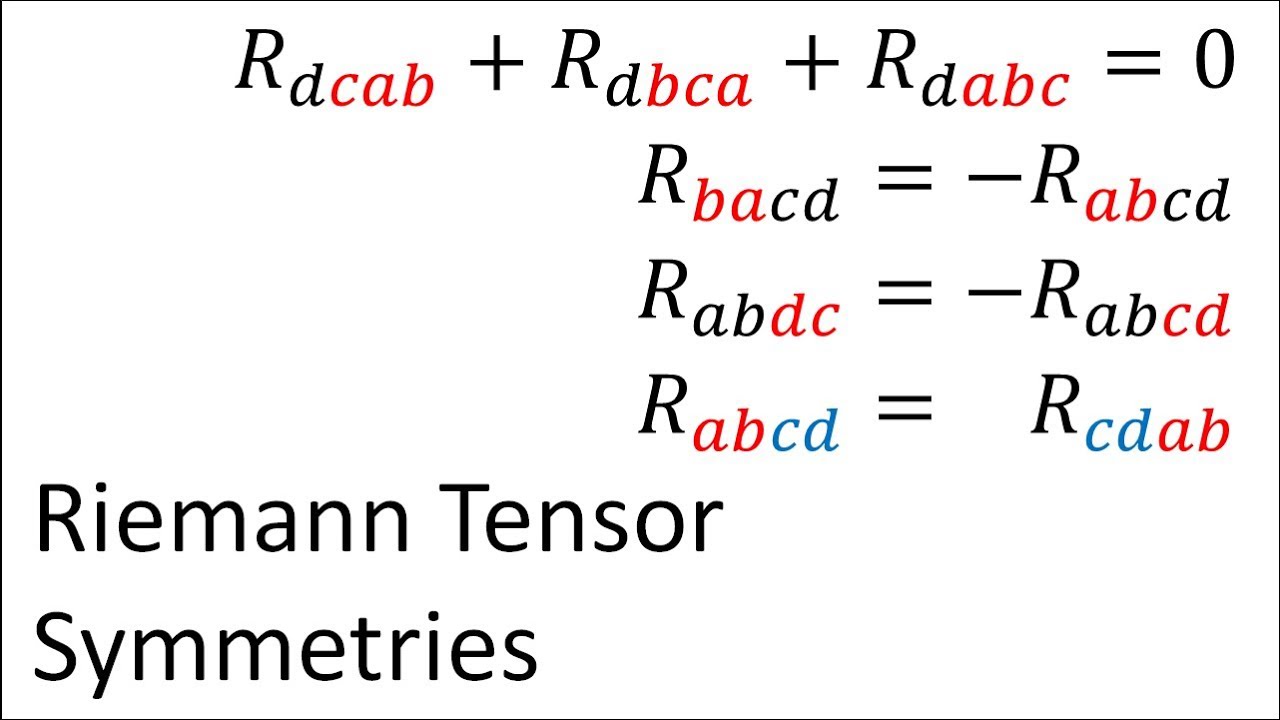
- 🔄 The Riemann tensor is shown to be anti-symmetric with respect to the first two indices and the third and fourth indices when those indices are swapped.
- 🔄 The tensor also exhibits symmetry when the first two indices are swapped with the third and fourth, maintaining the tensor's value.
- 📉 The symmetries of the Riemann tensor greatly reduce its complexity, with many of its components being zero due to these anti-symmetric properties.
- 📏 In two dimensions, the Riemann tensor has 16 components, but due to symmetries, only four have a degree of freedom, simplifying the analysis.
- 📊 In three dimensions, out of 81 components, 45 are zero, and the remaining 36 are limited to six degrees of freedom.
- 📈 In four dimensions, 256 components are present, with 112 being zero and the other 144 limited to 21 degrees of freedom.
- 🔗 The first Bianchi identity is introduced, which states that when the Riemann tensor is permuted cyclically with its last three indices and summed, the result is zero.
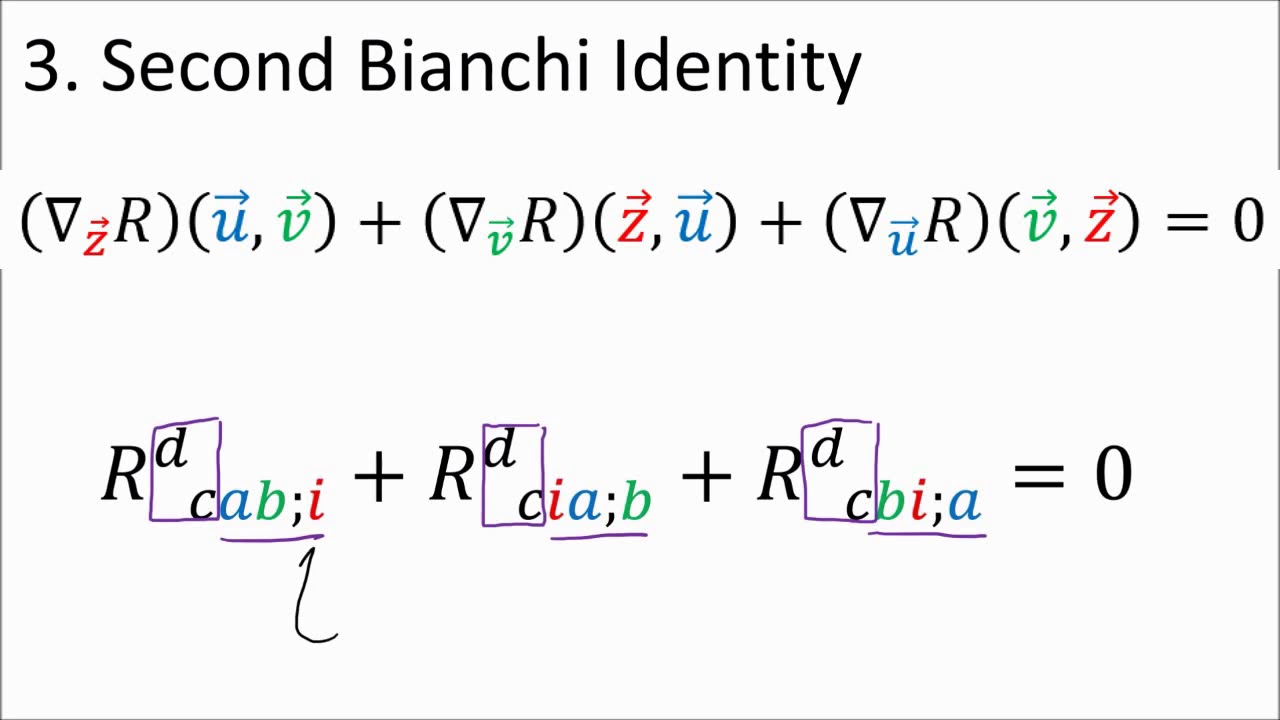
- 🔄 The second Bianchi identity involves the covariant derivative of the Riemann tensor and shows a similar cancellation when indices are cyclically permuted.
- 📘 The use of Riemann normal coordinates simplifies calculations, as the Christoffel symbols are zero, making the covariant derivative equal to the partial derivative.
- 🔑 Both Bianchi identities are crucial for understanding the constraints on the Riemann tensor and are foundational for further analysis in differential geometry and general relativity.
Q & A
What is the main focus of video 84 on tensor calculus?
-The main focus of video 84 is to explore the various symmetries and identities of the Riemann tensor using Riemann normal coordinates.
How does the Riemann tensor's expression in Riemann normal coordinates simplify the analysis?
-In Riemann normal coordinates, the Christoffel symbols evaluate to zero, which simplifies the expression for the Riemann tensor and makes the analysis more straightforward.
What property of the Riemann tensor is demonstrated when the first two indices are swapped?
-Swapping the first two indices of the Riemann tensor introduces a negative sign, demonstrating that the Riemann tensor is anti-symmetric with respect to the first two indices.
How does the symmetry of the covariant metric tensor affect the Riemann tensor's expression?
-The symmetry of the covariant metric tensor allows for the indices to be swapped without changing the value of the tensor, which simplifies the expression by showing that certain terms are negatives of each other.
What is the significance of the tensor relationship in proving the general validity of the Riemann tensor's properties?
-The tensor relationship is significant because if a property is proven to hold in one coordinate system, such as Riemann normal coordinates, it holds for all coordinate systems due to the nature of tensor transformations.
What does the anti-symmetry of the Riemann tensor with respect to the third and fourth indices imply?
-The anti-symmetry with respect to the third and fourth indices implies that swapping these indices also introduces a negative sign, which is another property that reduces the complexity of the tensor.
How does the symmetry of the Riemann tensor when flipping the pairs of indices reduce the number of components?
-This symmetry means that swapping the first and second with the third and fourth indices does not change the value of the tensor, reducing the number of independent components and simplifying the evaluation of the tensor.
In two dimensions, how does the symmetry of the Riemann tensor affect the number of non-zero components?
-In two dimensions, due to the anti-symmetry, 12 out of 16 components are zero, and the other four components are limited to a single degree of freedom.
What is the First Bianchi Identity, and what does it represent?
-The First Bianchi Identity is an important relationship where the Riemann tensor is added in three forms with the last three indices permuted cyclically, resulting in a sum of zero. It represents an additional limitation on the degrees of freedom of the tensor.
What is the Second Bianchi Identity, and how does it relate to the covariant derivative?
-The Second Bianchi Identity is similar to the First, but it involves the covariant derivative of the Riemann tensor. When the indices are permuted cyclically and the expressions are added together, the result is zero. This identity further constrains the tensor's degrees of freedom.
Why are Riemann normal coordinates advantageous when working with the Riemann tensor?
-Riemann normal coordinates are advantageous because they simplify the calculations by making the Christoffel symbols equal to zero, reducing the complexity of the tensor expressions and making the analysis more manageable.
Outlines
📚 Exploring Riemann Tensor Symmetries
In this segment, the video delves into the symmetries and identities of the Riemann tensor using Riemann normal coordinates. It begins by re-expressing the tensor with swapped indices, revealing the anti-symmetry with respect to the first two and third and fourth indices. The video explains that these symmetries hold true for all coordinate systems due to the nature of tensor relationships. The discussion then moves to the simplification of the tensor's complexity, showing that in two dimensions, the tensor is reduced to one degree of freedom with only four non-zero components, significantly simplifying its evaluation.
🔍 Reducing Complexity with Symmetries
This paragraph further explores the implications of the Riemann tensor's symmetries on its complexity. It explains how in two, three, and four dimensions, the number of non-zero components is greatly reduced due to these symmetries. The video provides specific numbers for each dimension, illustrating how the degrees of freedom are limited. It also introduces the concept of Bianchi identities, setting the stage for their exploration in the subsequent paragraphs.
🧩 Deriving Bianchi Identities
The video script describes the process of deriving two important identities related to the Riemann tensor, known as the Bianchi identities. It begins by permuting the last three indices of the tensor and adding the resulting expressions, leading to the first Bianchi identity, which states that the sum of these permutations equals zero. The video emphasizes the significance of this identity in reducing the degrees of freedom in the tensor. The second part of this paragraph discusses the covariant derivative of the Riemann tensor and sets up for the derivation of the second Bianchi identity in the next paragraph.
📉 Second Bianchi Identity and Recap
The final paragraph concludes the video by summarizing the findings. It reiterates the simplification of the Riemann tensor in Riemann normal coordinates and the discovery of its symmetries, which reduce the complexity of the tensor significantly. The paragraph then explains the second Bianchi identity, which involves the covariant derivative of the Riemann tensor and results in a similar cancellation leading to zero. The video ends with a recap of the key points covered, emphasizing the importance of these symmetries and identities in understanding the Riemann tensor and its applications in future videos.
Mindmap
Keywords
💡Riemann Tensor
💡Riemann Normal Coordinates
💡Symmetry
💡Anti-symmetry
💡Tensor Relationship
💡Christoffel Symbols
💡Covariant Derivative
💡Bianchi Identities
💡Degrees of Freedom
💡Cyclic Permutation
Highlights
Introduction to using Riemann normal coordinates to explore the symmetries and identities of the Riemann tensor.
Re-expression of the Riemann tensor by switching the first two indexes to reveal anti-symmetry.
Explanation of the covariant metric tensor's symmetry allowing index order swaps.
Demonstration of the Riemann tensor's anti-symmetry with respect to the first two indexes.
Generalization of the anti-symmetry property to all coordinate systems due to tensor nature.
Swapping indexes alpha and beta to show another anti-symmetry property of the Riemann tensor.
Symmetry of the Riemann tensor when flipping pairs of the first and third indexes.
Reduction in complexity of the Riemann tensor due to its symmetric relationships.
Specific example of how symmetries reduce the number of components in a 2-dimensional Riemann tensor.
Extension of the analysis to 3 and 4 dimensions, showing a decrease in non-zero components.
Introduction to the first Bianchi identity through permuting the last three indices of the Riemann tensor.
Cancellation of terms in the first Bianchi identity leading to an important tensor relationship.
Taking the covariant derivative of the Riemann tensor in Riemann normal coordinates.
Simplification due to zero Christoffel symbols in Riemann normal coordinates.
Derivation of the second Bianchi identity involving the covariant derivative.
Reflection on the ease of calculations in Riemann normal coordinates compared to other systems.
Final summary of the symmetries and identities of the Riemann tensor explored in the video.
Transcripts
Browse More Related Video
Tensor Calculus 23: Riemann Curvature Tensor Components and Symmetries
Tensor Calculus Lecture 8e: The Riemann Christoffel Tensor & Gauss's Remarkable Theorem
Tensor Calculus 26 - Ricci Tensor/Scalar Properties
Video 43 - Tensor Symmetry
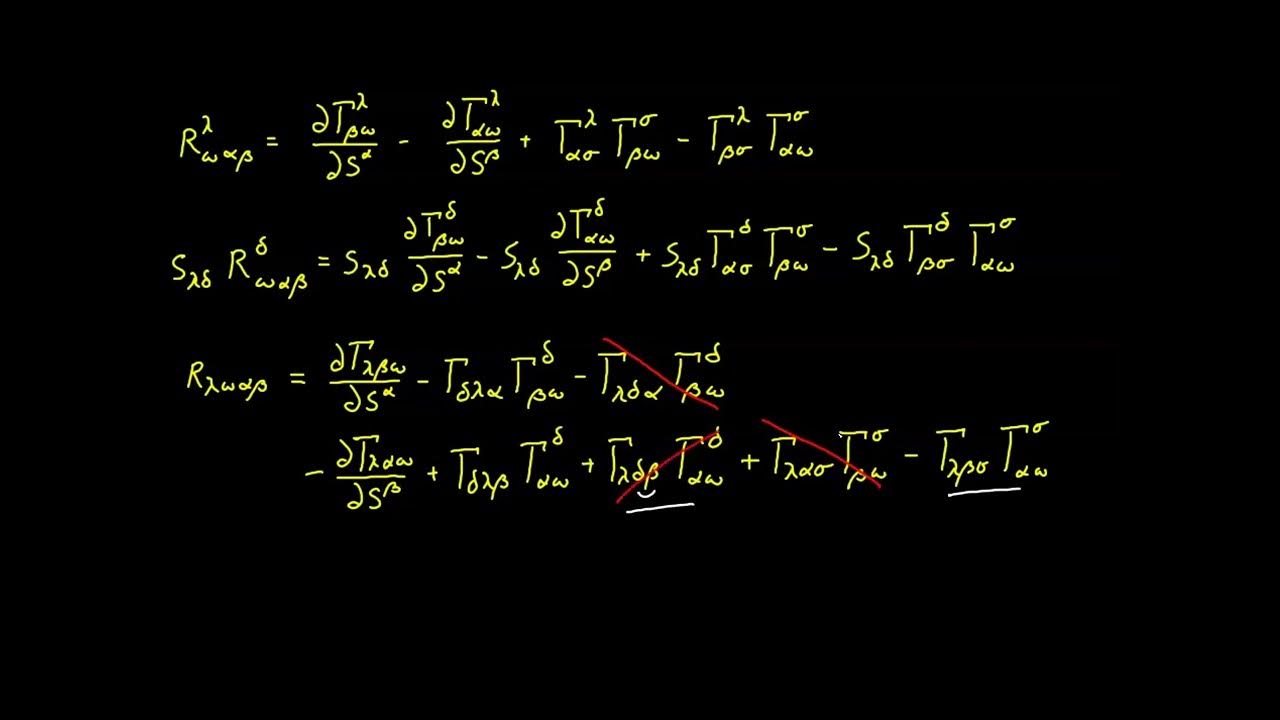
Video 82 - Riemann Christoffel Tensor
Tensor Calculus Lecture 6c: The Covariant Derivative 2
5.0 / 5 (0 votes)
Thanks for rating: