Tensor Calculus 23: Riemann Curvature Tensor Components and Symmetries
TLDRThis video delves into the Riemann curvature tensor, a fundamental concept in understanding space curvature. It explains the tensor's definition, symmetries, and how it can determine if a space is flat or curved. The script guides viewers through the computation of the Riemann tensor for 2D polar coordinates and a 2D sphere, illustrating the tensor's role in identifying space properties. The video concludes with a look ahead to the Ricci curvature tensor and its significance in Einstein's field equations.
Takeaways
- 📚 The video introduces the Riemann curvature tensor components and discusses their symmetries, focusing on their significance in determining the curvature of space.
- 🔍 The Riemann tensor is derived from the parallel transport of a vector around a parallelogram defined by two vector fields, indicating curvature when the transported vector differs from the original.
- 📈 The script explains that the Riemann tensor is a multilinear map, implying linearity in each of its inputs, simplifying computations to the action on a basis set of vectors.
- 🔗 The video emphasizes the importance of symmetries in the Riemann tensor, which include the three-for symmetry, Bianchi identity, 1/2 symmetry, and flip symmetry, greatly reducing the number of components to compute.
- 🧩 The script demonstrates that in two dimensions, the Riemann tensor's components can be reduced to a single independent component due to these symmetries.
- 🌐 The video provides an example of computing the Riemann tensor for 2D polar coordinates, revealing that the space is flat as all components are zero.
- 🌍 It also computes the Riemann tensor for a 2D surface of a sphere, showing that the space is curved due to nonzero tensor components.
- 📉 The script clarifies that a zero Riemann tensor indicates a flat space, while a nonzero tensor suggests curvature, which affects the parallel transport of vectors.
- 🔑 The video mentions that the symmetries of the Riemann tensor reduce the 16 components in two dimensions and 256 in four dimensions to a much smaller set of independent components.
- 🔮 The upcoming video will discuss the Ricci curvature tensor, another key tensor in describing space curvature and its role in Einstein's field equations of general relativity.
- 🤓 The video encourages viewers to check the description for links to related content and to support the creator for more informative content.
Q & A
What is the Riemann curvature tensor and why is it significant in the study of curved spaces?
-The Riemann curvature tensor is a mathematical object that describes the curvature of a space. It is significant because it quantifies the extent to which a space is curved. The tensor gives a measure of how a vector changes as it is parallel transported around a small parallelogram defined by two vector fields. If the resulting difference vector is nonzero, it indicates that the space is curved.
What does it mean for the Riemann tensor to be a multi-linear map?
-For the Riemann tensor to be a multi-linear map means that it is linear in each of its inputs. This property implies that when computing the Riemann tensor acting on any three vector inputs, one can factor out the vector components from the formula, simplifying the computation to understanding how the Riemann tensor acts on a set of basis vectors.
Why is the Lie bracket of basis vectors always zero?
-The Lie bracket of basis vectors is always zero because basis vectors represent partial derivatives, and the order of taking partial derivatives should not affect the outcome. Additionally, in coordinate lines, there are no gaps between flow curves, and since the Lie bracket measures the gap between flow curves, it is zero when there are no gaps.
How does the symmetry of the Riemann tensor help simplify its computation?
-The symmetries of the Riemann tensor allow for many of its components to be determined by a smaller set of independent components. For instance, in two dimensions, the 16 components of the Riemann tensor can be reduced to a single independent component due to its symmetries, significantly simplifying the computation.
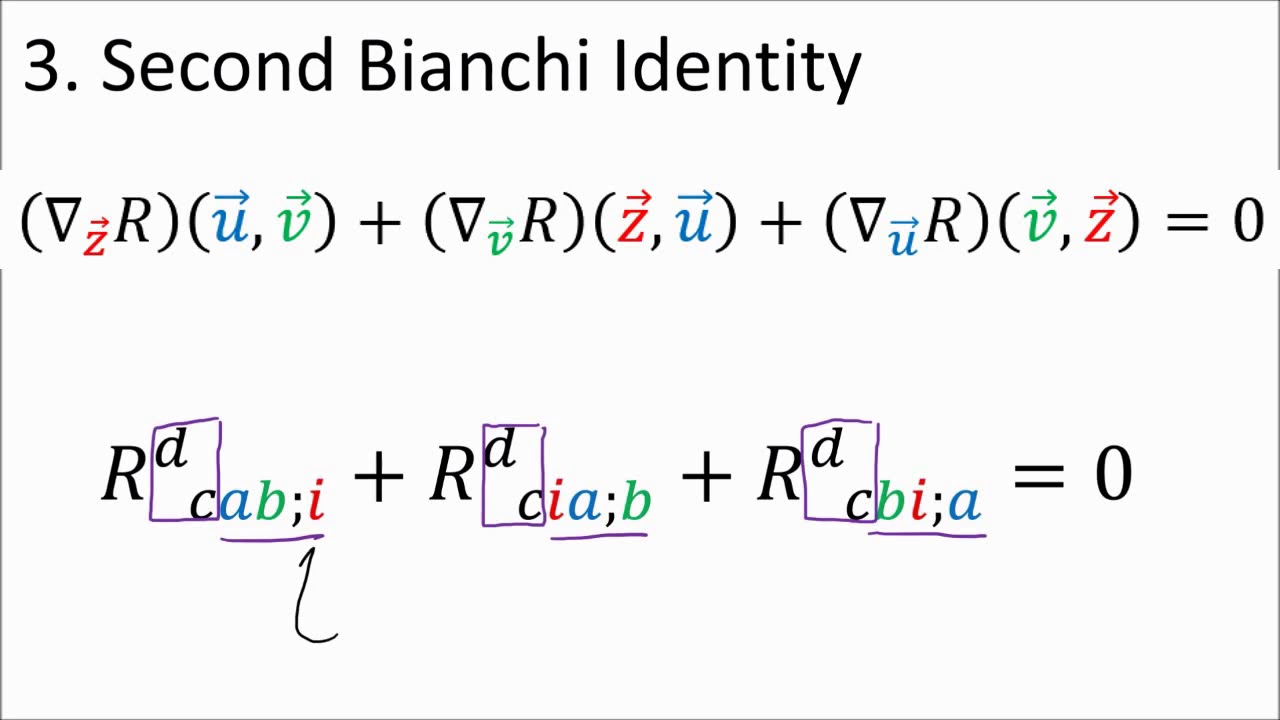
What is the first Bianchi identity and how does it relate to torsion-free connections?
-The first Bianchi identity is a symmetry of the Riemann tensor stating that when the tensor's components are added together in a specific way, the result is zero. This identity applies only to torsion-free connections, which are connections where the covariant derivative of one basis vector in the direction of another is the same as the covariant derivative of the second basis vector in the direction of the first.
What is the significance of the metric compatibility property in the context of the Riemann tensor?
-The metric compatibility property ensures that the covariant derivative obeys a product rule over the dot product. This property is crucial when deriving the symmetries of the Riemann tensor and when proving that certain components of the tensor are equal to zero or related to each other.
How does the script demonstrate the computation of the Riemann tensor components for 2D polar coordinates?
-The script demonstrates the computation by showing that in 2D polar coordinates, most of the connection coefficients are zero, which simplifies the formula for the Riemann tensor components. It then shows that the only non-zero component is the one with indexes 1/2 1/2, which turns out to be zero, indicating that the space described by 2D polar coordinates is flat.
What does it mean for a space to be flat according to the Riemann curvature tensor?
-A space is considered flat if the Riemann curvature tensor has zero components everywhere. This implies that there is no curvature in the space, and parallel transporting a vector around a small loop does not change its direction.
How does the script illustrate the computation of the Riemann tensor for the 2D surface of a sphere?
-The script computes the Riemann tensor for the 2D surface of a sphere by focusing on the 1/2 1/2 component, using the connection coefficients specific to the sphere. The computation results in a non-zero component, indicating that the 2D surface of a sphere is curved.
What is the Ricci curvature tensor and how does it relate to the Riemann curvature tensor?
-The Ricci curvature tensor is another mathematical object that describes the curvature of a space. It is derived from the Riemann curvature tensor by contracting two of its indices. The Ricci tensor is particularly important as it appears in the Einstein field equations of general relativity, which describe the fundamental interaction of gravitation as a result of spacetime being curved.
Outlines
📚 Introduction to Riemann Curvature Tensor
This paragraph introduces the Riemann curvature tensor, a fundamental concept in differential geometry used to describe the curvature of a space. The video promises to explain the tensor's components and their symmetries. The presenter also references a previous video where the tensor was derived, highlighting the tensor's role in measuring the change in a vector when parallel transported around a parallelogram defined by other vector fields. The importance of basis vectors and their properties, such as having zero Lie brackets, is discussed, simplifying the computation of the Riemann tensor.
🔍 Symmetries of the Riemann Curvature Tensor
The second paragraph delves into the symmetries of the Riemann curvature tensor, which are crucial for simplifying calculations in higher dimensions. The presenter outlines four key symmetries: the three-for symmetry, the Bianchi identity, the 1/2 symmetry, and the flip symmetry. These symmetries reduce the number of unique components that need to be calculated, transforming a seemingly complex task into a more manageable one. The explanation includes assumptions about the use of the Levi-Civita connection and its properties, such as metric compatibility and being torsion-free.
📘 Derivation of Riemann Tensor Symmetries
In this paragraph, the presenter provides a detailed derivation of the symmetries of the Riemann tensor, starting with the assumption of the Levi-Civita connection. The explanation involves the manipulation of terms and application of properties like metric compatibility and torsion-free nature of the connection. The Bianchi identity is derived by adding certain components of the Riemann tensor and showing they sum to zero. The paragraph also demonstrates how the other symmetries can be proven using similar algebraic manipulations and properties of the connection coefficients.
🌐 Zero Components of the Riemann Tensor in 2D
The fourth paragraph focuses on the simplification of the Riemann tensor in two dimensions, where many of its components are found to be zero due to the symmetries discussed earlier. The presenter lists the zero components and explains how the symmetries can be used to reduce the 16 potential components of the tensor in two dimensions to just one independent component. This reduction is significant as it simplifies the analysis of 2D spaces and their curvature properties.
📉 Computing Riemann Tensor for 2D Polar and Spherical Coordinates
This paragraph discusses the computation of the Riemann curvature tensor for two specific 2D coordinate systems: polar coordinates and the surface of a sphere. For polar coordinates, the presenter shows that all components of the Riemann tensor are zero, confirming that this space is flat. In contrast, for the 2D surface of a sphere, the computation reveals nonzero components, indicating that the space is curved. The presenter uses the connection coefficients for these coordinate systems and applies the formula for the Riemann tensor components to arrive at these conclusions.
🌌 Summary of Riemann Tensor Concepts and预告 of Ricci Tensor
The final paragraph summarizes the key points covered in the video about the Riemann curvature tensor, including its definition, geometric interpretation, and the linearity property that simplifies its computation. The presenter also hints at the next topic to be covered in the series: the Ricci curvature tensor, which is another important concept in the study of space curvature and plays a role in Einstein's field equations of general relativity. The summary reinforces the understanding of the Riemann tensor and its significance in determining whether a space is curved or flat.
🚀 Conclusion and Call to Action
In the closing paragraph, the presenter concludes the video with a brief recap of the topics covered and a call to action for viewers to support the channel by checking the links in the description. This paragraph serves as a polite reminder and encouragement for viewers to engage further with the content and the creator.
Mindmap
Keywords
💡Riemann Curvature Tensor
💡Symmetries
💡Connection Coefficients
💡Covariant Derivative
💡Basis Vectors
💡Li Bracket
💡Metric Compatibility
💡Torsion Free
💡Polar Coordinates
💡2D Surface of a Sphere
Highlights
Introduction to Riemann curvature tensor components and their symmetries.
Explanation of how the Riemann tensor indicates space curvature through parallelogram vector transport.
Proof that the Riemann tensor is a multi-linear map, emphasizing its linearity for each input.
Demonstration of the simplification process for computing the Riemann tensor using basis vectors.
Clarification on the Li bracket of basis vectors being zero and its implications for the Riemann tensor formula.
Derivation of the Riemann tensor components using connection coefficients and the product rule.
Identification of the Riemann tensor's symmetries, including the three-for symmetry and the Bianchi identity.
Explanation of the 1/2 symmetry and the flip symmetry of the Riemann tensor components.
Proof of the first Bianchi identity for torsion-free connections.
Application of metric compatibility property to derive the one-two index symmetry.
Derivation of the final symmetry from the other three, flipping the first and second with the third and fourth indexes.
Reduction of 16 Riemann tensor components in two dimensions to a single independent component using symmetries.
Computation of the Riemann tensor components for 2D polar coordinates, revealing a flat space.
Calculation of the Riemann tensor for a 2D sphere, demonstrating a curved space with nonzero components.
Summary of the significance of the Riemann tensor in determining space curvature and its relation to flatness.
Introduction to the Ricci curvature tensor and its importance in Einstein's field equations.
Transcripts
Browse More Related Video
Tensor Calculus Lecture 8e: The Riemann Christoffel Tensor & Gauss's Remarkable Theorem
Tensor Calculus 22: Riemann Curvature Tensor Geometric Meaning (Holonomy + Geodesic Deviation)
Tensor Calculus 24: Ricci Tensor Geometric Meaning (Sectional Curvature)
Tensor Calculus Lecture 6c: The Covariant Derivative 2
Tensor Calculus 26 - Ricci Tensor/Scalar Properties
Video 82 - Riemann Christoffel Tensor
5.0 / 5 (0 votes)
Thanks for rating: