Applying the empirical rule to an example that uses an interval that is not symmetric
TLDRThe script explains how to determine the percentage of men with weights between 120 and 198 pounds, given a normal distribution with a mean of 172 pounds and a standard deviation of 26 pounds. It guides through drawing a bell curve, placing the mean, and identifying the interval. Using the empirical rule, the video calculates the area under the curve within one and two standard deviations from the mean, summing these to approximate 81.5% of men falling within the specified weight range.
Takeaways
- 📊 The script discusses the normal distribution of men's weight with a mean of 172 pounds and a standard deviation of 26 pounds.
- 📈 The Empirical Rule (68-95-99.7 Rule) is mentioned as a tool to estimate the percentage of data within certain intervals in a normal distribution.
- 📝 The problem asks for the percentage of men whose weight falls between 120 and 198 pounds.
- 🎨 The speaker suggests drawing a bell curve to visualize the distribution and to mark the mean, standard deviation, and the interval of interest.
- ⚖️ The process involves calculating how many standard deviations away from the mean the interval boundaries (120 and 198 pounds) are.
- 🔢 For 198 pounds, it is one standard deviation above the mean, which corresponds to approximately 34% of the data.
- 🔢 For 120 pounds, it is calculated to be two standard deviations below the mean, which corresponds to approximately 47.5% of the data.
- 🔄 The bell curve's symmetry is emphasized to understand the distribution of data on either side of the mean.
- 🧩 The final step is to add the percentages from both sides of the mean to find the total percentage of men within the interval, which is approximately 81.5%.
- 📐 The explanation provides a clear method to apply the Empirical Rule to find the area under the curve for a given interval.
- 📝 The script serves as a tutorial on how to interpret and calculate with normal distributions in a statistical context.
Q & A
What is the mean weight of men according to the transcript?
-The mean weight of men is 172 pounds.
What is the standard deviation of men's weight in the given distribution?
-The standard deviation of men's weight is 26 pounds.
What is the Empirical Rule mentioned in the transcript?
-The Empirical Rule states that for a normal distribution, approximately 68% of data falls within one standard deviation of the mean, 95% within two standard deviations, and 99.7% within three standard deviations.
How does the bell curve represent the distribution of men's weights?
-The bell curve represents the distribution of men's weights with the mean at the center and symmetrically decreasing as it moves away from the mean, indicating the proportion of men with weights at different distances from the mean.
What is the significance of the numbers 120 and 198 in the context of the transcript?
-The numbers 120 and 198 represent the weight range within which the percentage of men is being calculated.
How does the transcript suggest finding the percentage of men between 120 and 198 pounds?
-The transcript suggests using the Empirical Rule and the properties of the normal distribution to find the percentage of men within one and two standard deviations from the mean, and then summing these percentages to find the total percentage between 120 and 198 pounds.
What is the percentage of men who weigh more than 198 pounds according to the transcript's calculations?
-The transcript calculates this as 34%, which is half of the 68% that falls within one standard deviation from the mean.
What is the percentage of men who weigh less than 120 pounds according to the transcript?
-The transcript calculates this as 47.5%, which is half of the 95% that falls within two standard deviations from the mean.
How does the transcript approximate the total percentage of men between 120 and 198 pounds?
-The transcript approximates the total percentage by adding the percentages of men who weigh more than 198 pounds (34%) and less than 120 pounds (47.5%), resulting in approximately 81.5%.
What is the importance of symmetry in the bell curve when calculating these percentages?
-The symmetry of the bell curve allows for the easy calculation of the percentage of data within certain intervals from the mean, as the percentages on either side of the mean are equal, simplifying the process of finding the total percentage within a range.
What is the final approximate percentage of men whose weights are between 120 and 198 pounds, as concluded in the transcript?
-The final approximate percentage is 81.5%.
Outlines
📊 Understanding Normal Distribution and Empirical Rule
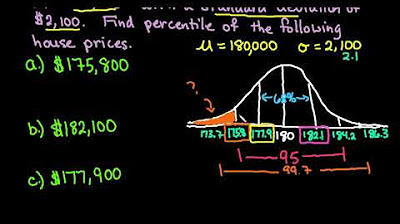
This paragraph discusses the concept of a normal distribution, specifically in relation to men's weight with a mean of 172 pounds and a standard deviation of 26 pounds. It introduces the empirical rule, which states that approximately 68% of data falls within one standard deviation of the mean, 95% within two standard deviations, and 99.7% within three. The speaker uses this rule to estimate the percentage of men whose weight falls between 120 and 198 pounds by visualizing the distribution as a bell curve and marking the mean, standard deviation, and the interval of interest. The process involves calculating the number of standard deviations each boundary (120 and 198 pounds) is from the mean, which helps determine the area under the curve within this range.
🔢 Calculating the Percentage of Men within a Weight Range
The second paragraph continues the discussion on the normal distribution of men's weight, focusing on calculating the exact percentage of men with weights between 120 and 198 pounds. The speaker uses the empirical rule to determine that 34% of men weigh between the mean (172 pounds) and one standard deviation above (198 pounds). By symmetry, the same percentage applies to the range below the mean. To find the percentage between 120 and 198 pounds, the speaker calculates the area two standard deviations below the mean (which is 47.5%) and adds it to the 34% found earlier. The final calculation approximates that 81.5% of men fall within the specified weight range, emphasizing the use of the empirical rule and the symmetry of the bell curve for this estimation.
Mindmap
Keywords
💡Normal Distribution
💡Mean
💡Standard Deviation
💡Bell Curve
💡Empirical Rule
💡Percentage
💡Interval
💡Symmetric
💡Standard Deviations (K)
💡Area Under the Curve
💡Approximation
Highlights
The problem involves a normally distributed data set of men's weights with a mean of 172 pounds and a standard deviation of 26 pounds.
The task is to estimate the percentage of men whose weights fall between 120 and 198 pounds.
The concept of the bell curve is introduced as a visualization tool for the normal distribution.
The mean (172 pounds) and standard deviation (26 pounds) are labeled on the bell curve.
The numbers 120 and 198 are plotted on the bell curve to represent the weight interval of interest.
The Empirical Rule (68-95-99.7 rule) is mentioned as a method to approximate the percentage of data within certain intervals.
The bell curve's symmetry is utilized to simplify calculations by dividing the Empirical Rule percentages by two.
A formula is used to calculate how many standard deviations away from the mean the weights 198 and 120 are.
198 pounds is found to be one standard deviation above the mean, corresponding to 34% of the data.
120 pounds is calculated to be two standard deviations below the mean.
The area representing two standard deviations below the mean is approximately 47.5% of the data.
The total area between 120 and 198 pounds is found by adding the two calculated percentages.
The final approximated percentage of men within the weight interval is 81.5%.
The process emphasizes the importance of understanding the bell curve and the Empirical Rule in statistical analysis.
The solution approach is practical and demonstrates how to apply statistical concepts to real-world data.
The transcript provides a step-by-step guide that is easy to follow for those learning about normal distributions.
The problem-solving method is innovative in its use of visual aids and the Empirical Rule for approximation.
Transcripts
Browse More Related Video

Using the Empirical rule to determine the approximate percentage of data less than a given value

Math 14 HW 6.4.12-T Using the Central Limit Theorem
Empirical Rule 68-95-99.7 Rule to Find Percentile
Empirical Rule of Standard Deviation in Statistics

The Probability an Elevator is Overloaded Central Limit Theorem with StatCrunch

What is a Bell Curve or Normal Curve Explained?
5.0 / 5 (0 votes)
Thanks for rating: