Math 14 HW 6.4.12-T Using the Central Limit Theorem

Fiorentino Siciliano
11 Mar 202311:36
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the redesign of ejection seats for fighter jets to accommodate female pilots, who were previously excluded from this role. The seats, initially designed for men weighing 130-201 pounds, were recalculated for women with a mean weight of 162 pounds and a standard deviation of 43 pounds. The script explains the statistical process of determining the probability of a woman's weight falling within the original design range using a normal distribution. It also addresses the probability for the mean weight of a group of women, concluding that the individual probability is more relevant for ejection seat performance.

Takeaways
  • 🚀 Women were initially excluded from being fighter jet pilots due to ejection seats designed only for men, with a weight range of 130 to 201 pounds.
  • 🔍 The average weight of women is 162 pounds with a standard deviation of 43 pounds, necessitating a redesign of ejection seats to accommodate this distribution.
  • 📊 The script discusses calculating probabilities using a normal distribution, with the mean and standard deviation provided for the weight of women.
  • 📘 Part A of the script focuses on finding the probability that a randomly selected woman weighs between 130 and 201 pounds, using z-scores and a normal distribution.
  • 📐 A z-score calculation is performed for both the lower (130 pounds) and upper (201 pounds) weight limits to determine the probability of a woman's weight falling within this range.
  • 📊 The calculated z-scores for 130 and 201 pounds are -0.74 and 0.91, respectively, which are used to find the area under the normal distribution curve.
  • 🔢 Using StatCrunch, the probability that a woman's weight is between 130 and 201 pounds is found to be approximately 0.5889.
  • 👥 Part B of the script addresses the probability that the mean weight of a sample of 29 women is between 130 and 201 pounds, considering the sample mean and standard deviation.
  • 📏 The standard deviation of the sample means is calculated by dividing the population standard deviation by the square root of the sample size (29).
  • 📉 Z-scores for the sample mean weights of 130 and 201 pounds are calculated as -4.01 and 4.88, respectively, indicating a very high probability for the sample mean to fall within this range.
  • 💯 The probability that the mean weight of a group of 29 women is between 130 and 201 pounds is found to be 1.000, suggesting that the sample mean will always fall within this range given the sample size.
  • 🛠️ The final decision on which probability is more relevant depends on whether the focus is on individual performance or the average performance for a group, with the individual performance being more critical for ejection seat design.
Q & A

  • Why were ejection seats redesigned for female pilots?

    -Ejection seats were originally designed for men, with weight considerations between 130 and 201 pounds. Since the average weight of women pilots is around 162 pounds with a different distribution, the redesign was necessary to accommodate their weight range.

  • What is the mean weight of women pilots mentioned in the script?

    -The mean weight of women pilots is 162 pounds.

  • What is the standard deviation of the weight of women pilots?

    -The standard deviation of the weight of women pilots is 43 pounds.

  • What is the probability that a randomly selected woman's weight is between 130 and 201 pounds?

    -The probability is approximately 0.5889, based on the normal distribution with the given parameters.

  • How was the z-score calculated for a woman weighing 130 pounds?

    -The z-score was calculated as (130 - 162) / 43, which equals approximately -0.74.

  • How was the z-score calculated for a woman weighing 201 pounds?

    -The z-score was calculated as (201 - 162) / 43, which equals approximately 0.91.

  • What statistical tool was used to find the probability between two z-scores?

    -StatCrunch was used to determine the probability between the z-scores of -0.74 and 0.91.

  • What is the difference between the probabilities calculated in Part A and Part B of the script?

    -Part A calculates the probability for an individual woman's weight, while Part B calculates the probability for the mean weight of a group of 29 women.

  • Why is the standard deviation of the sample means different from the standard deviation of individual weights?

    -The standard deviation of the sample means is the standard deviation of individual weights divided by the square root of the sample size (n=29), reflecting the variability expected in the average of a group compared to individuals.

  • What is the relevance of the probabilities calculated in Part A and Part B for the ejection seat redesign?

    -Part A's probability is more relevant for the ejection seat redesign because it focuses on the performance of the seat for a single pilot, which is critical for safety.

  • What is the calculated probability that the mean weight of 29 women is between 130 and 201 pounds?

    -The calculated probability is 1.000, indicating that it is almost certain that the mean weight of such a group would fall within this range.

  • Why is the probability in Part B so high compared to Part A?

    -The high probability in Part B is due to the law of large numbers, where the mean of a larger sample is more likely to be closer to the overall mean, thus making it almost certain to fall within the specified range.

Outlines
00:00
🚀 Ejection Seat Redesign for Female Pilots

This paragraph discusses the necessity for engineers to redesign ejection seats in fighter jets to accommodate female pilots. Originally designed for men weighing 130 to 201 pounds, the new average weight for women is 162 pounds with a standard deviation of 43 pounds. The task involves calculating the probability that a randomly selected woman's weight falls within the original design range using the normal distribution formula. The process includes determining z-scores for the weight limits and using a bell curve to find the area between these scores, which represents the probability. The calculation is demonstrated using a step-by-step approach, including the use of a calculator or software like StatCrunch, to find the probability of 0.5889.

05:00
📊 Probability of Mean Weight for a Group of Women

The second paragraph extends the discussion to the probability of the mean weight of a group of 29 women falling between 130 and 201 pounds. The focus shifts from an individual to a sample mean from a normally distributed population. The process involves using the formula for the standard deviation of the sample means, which is the original standard deviation divided by the square root of the sample size. Z-scores for the group's mean weight at the specified limits are calculated, resulting in significantly different values due to the reduced standard deviation for sample means. Using StatCrunch, the probability that the mean weight of the group falls within the range is found to be 1.000, indicating a certainty that the mean weight will be within the specified range when considering a sample of this size.

10:02
🛠 Relevance of Probability in Ejection Seat Design

The final paragraph addresses the relevance of the calculated probabilities in the context of ejection seat redesign. It contrasts the probability for an individual pilot with that for the average of a group, highlighting the importance of the individual probability in the case of ejection seat performance. The individual probability from Part A is deemed more relevant because the performance of the ejection seat for a single pilot is of paramount importance, as it directly affects the safety and survival of that individual in an emergency situation.

Mindmap
Keywords
💡Ejection Seat
An ejection seat is a safety device used in military aircraft to quickly and safely remove the pilot from the cockpit in case of an emergency. In the script, it is mentioned that ejection seats were originally designed for male pilots, and a redesign was needed to accommodate the weight distribution of women pilots, highlighting the importance of inclusive design in safety equipment.
💡Fighter Jets
Fighter jets are high-performance military aircraft designed for air-to-air combat and other missions. The script discusses the historical exclusion of women from piloting such aircraft and the subsequent need for engineering adjustments to accommodate female pilots, emphasizing the evolution of gender inclusivity in the military.
💡Normal Distribution
Normal distribution, also known as Gaussian distribution, is a probability distribution that is commonly used in statistics to represent real-valued random variables. In the script, normal distribution is used to describe the weight distribution of women, which is essential for calculating the probability of a woman's weight falling within a certain range for ejection seat redesign.
💡Mean
The mean, often referred to as the average, is a measure of central tendency in a set of numbers. In the context of the script, the mean weight of women is given as 162 pounds, which is a key parameter in determining the probability of weight falling within a specific range using the normal distribution.
💡Standard Deviation
Standard deviation is a measure that indicates the amount of variation or dispersion in a set of values. The script mentions a standard deviation of 43 pounds for the weight of women, which is crucial for calculating the spread of weights around the mean and for determining probabilities.
💡Z-Score
A z-score represents the number of standard deviations an element is from the mean within a normal distribution. The script calculates z-scores for the weights 130 and 201 pounds to find the probability of a woman's weight falling between these values, which is a fundamental step in the statistical analysis.
💡Probability
Probability is a measure of the likelihood that a given event will occur. The script involves calculating the probability of a woman's weight being within the range suitable for the ejection seat, which is essential for understanding the redesign requirements and ensuring safety.
💡Sample Mean
The sample mean is the average of a subset of data drawn from a larger population. In the script, the concept of sample mean is used to calculate the probability that the average weight of a group of 29 women falls within a certain range, which is different from calculating the probability for an individual.
💡Statcrunch
Statcrunch is a statistical software tool used for data analysis and graphing. The script mentions using Statcrunch to compute probabilities associated with the normal distribution, demonstrating the practical application of statistical software in solving real-world problems.
💡Inclusivity
Inclusivity refers to the practice of including and accommodating all individuals, particularly those who may have been historically marginalized or excluded. The script discusses the need for redesigning ejection seats to be inclusive of women, illustrating the importance of inclusivity in design and engineering.
💡Safety Equipment
Safety equipment refers to devices or tools designed to protect individuals from harm or injury. The script highlights the redesign of ejection seats as a form of safety equipment that must be adapted to ensure the safety of all pilots, regardless of gender.
Highlights

Engineers had to redesign ejection seats for fighter jets to accommodate women pilots, as the original design was based solely on male weight distribution.

The ejection seats were initially designed for men weighing between 130 and 201 pounds.

The mean weight of women is now normally distributed with a mean of 162 pounds and a standard deviation of 43 pounds.

A normal distribution model is used to calculate the probability of a woman's weight falling between 130 and 201 pounds.

The first step in calculating the probability is to find the z-scores for the given weight range using the mean and standard deviation.

Z-score 1 is calculated for the lower weight limit (130 pounds), resulting in a value of -0.74.

Z-score 2 is calculated for the upper weight limit (201 pounds), resulting in a value of 0.91.

The probability of a single woman's weight being between 130 and 201 pounds is found using a normal distribution calculator.

The calculated probability is approximately 0.5889, indicating a 58.89% chance for a randomly selected woman.

For a sample of 29 women, the probability of their mean weight being within the range is calculated differently, considering the sample mean.

The standard deviation of the sample means is calculated by dividing the original standard deviation by the square root of the sample size (29).

Z-scores for the sample mean at 130 and 201 pounds are calculated, resulting in values of -4.01 and 4.88, respectively.

The probability of the mean weight of 29 women falling between 130 and 201 pounds is found to be 1.000, indicating certainty.

When redesigning ejection seats, the probability relevant to individual performance (Part A) is deemed more important than the average group performance (Part B).

The importance of individual seat performance for a single pilot is highlighted, emphasizing safety and suitability for all pilots, regardless of gender.

The transcript provides a detailed statistical approach to solving real-world engineering problems with implications for gender inclusivity in design.

Transcripts

Browse More Related Video

Math 14 HW 6.4.15-T Using the Central Limit Theorem

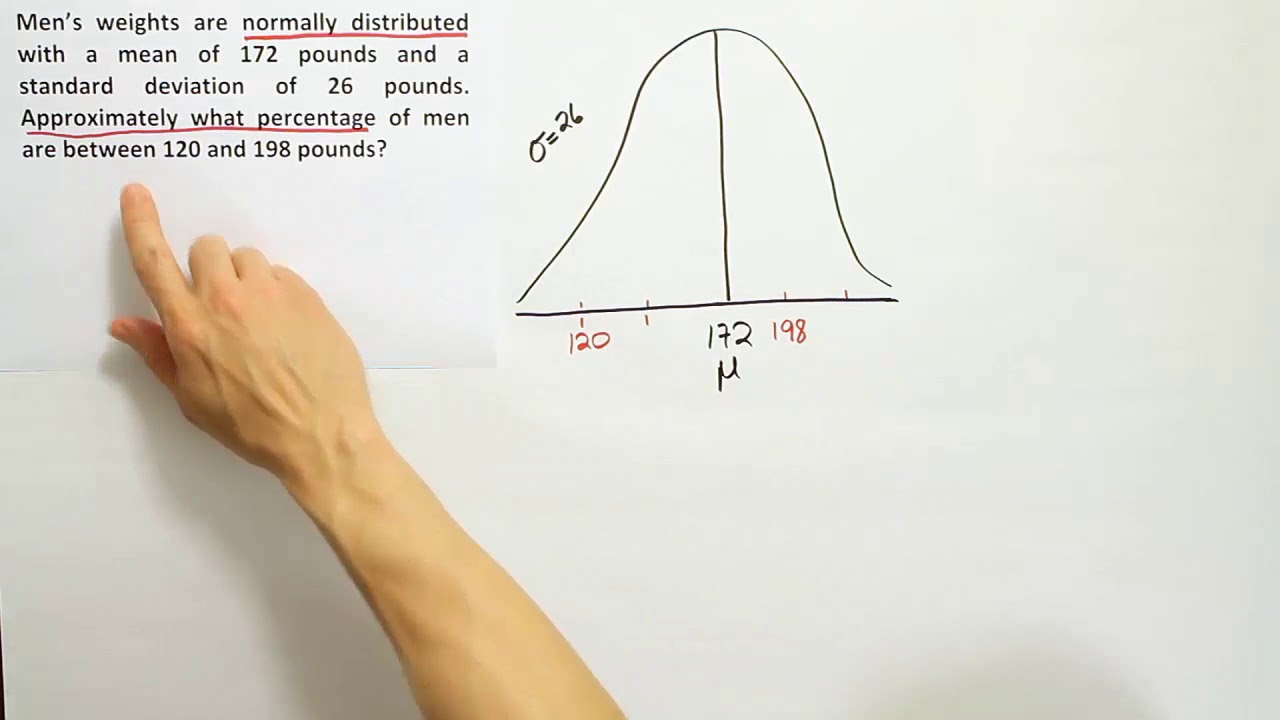
Applying the empirical rule to an example that uses an interval that is not symmetric

The Probability an Elevator is Overloaded Central Limit Theorem with StatCrunch

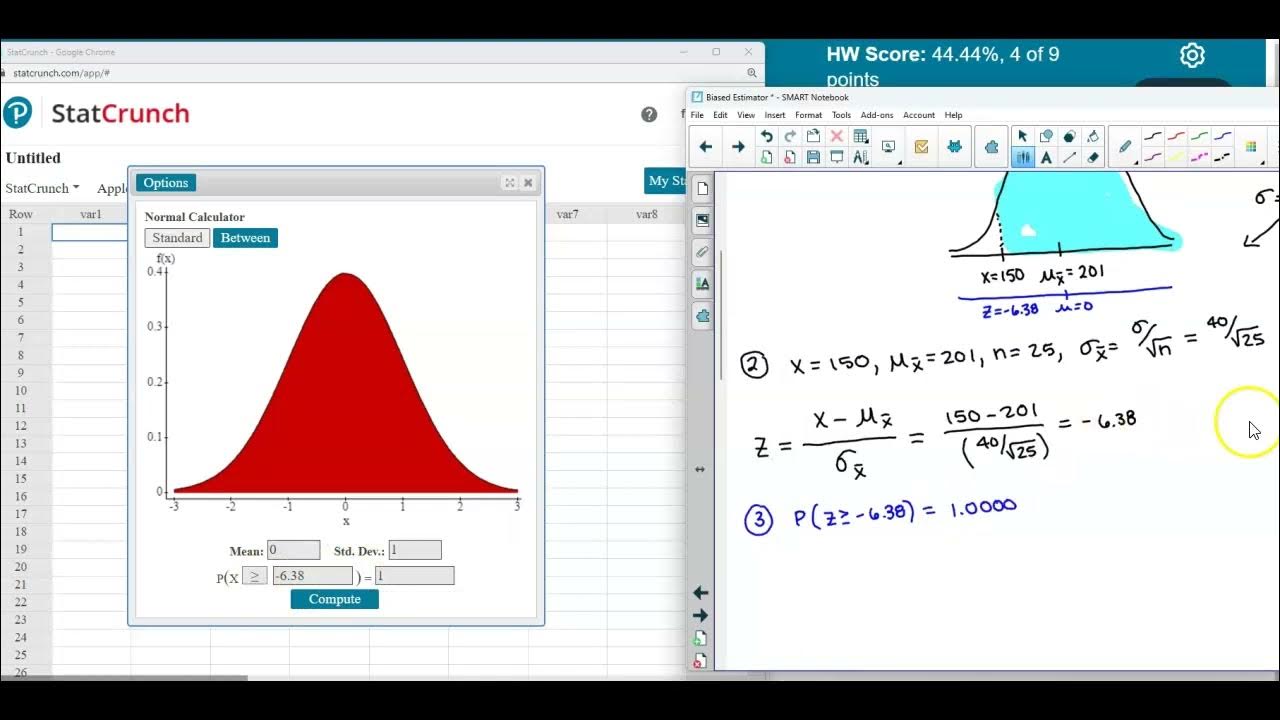
Math 14 HW 6.4.11-T Using the Central Limit Theorem

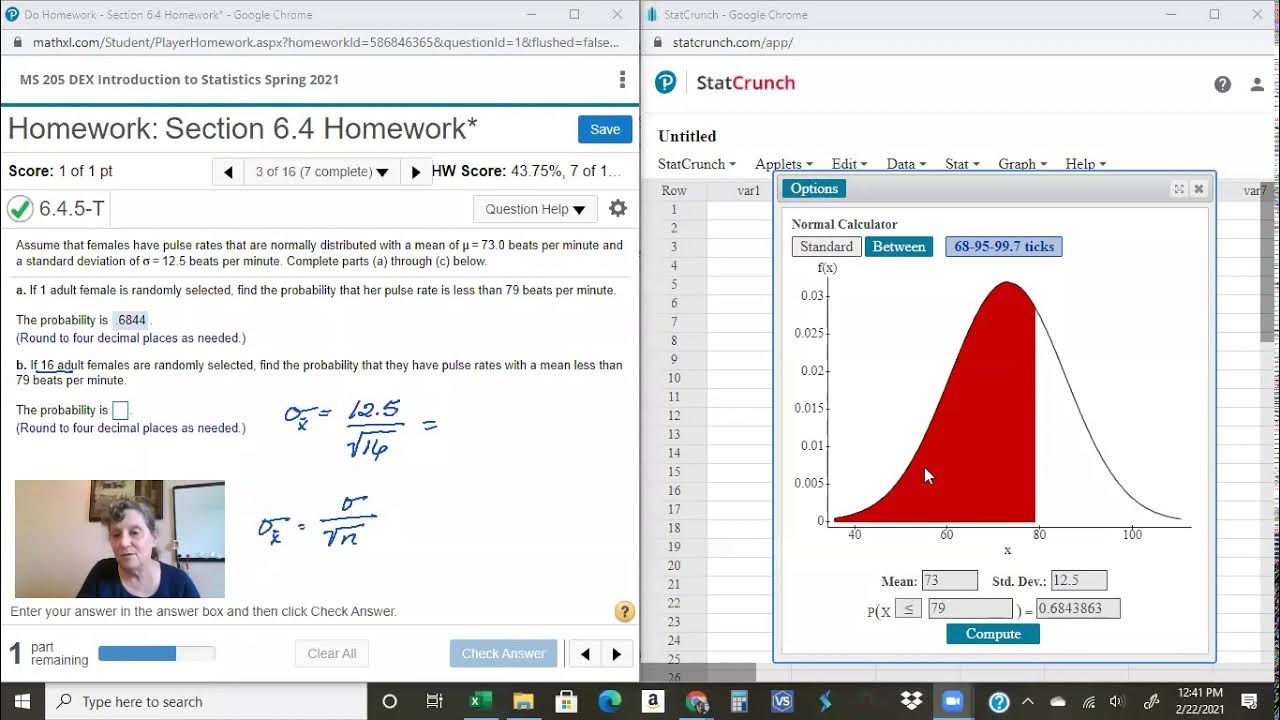
Calculating Probabilities of Sample Distributions with StatCrunch

Central Limit Theorem Probability Question with Pulse Rates in StatCrunch

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Ejection SeatFemale PilotsWeight DistributionProbability AnalysisStatistical CalculationEngineering DesignGender InclusionSafety StandardsNormal DistributionStatistical Tools