What is a Confidence Interval? | Puppet Master of Statistics

MarinStatsLectures-R Programming & Statistics
29 Jan 201705:57
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, Mike Merin explains the concept of a confidence interval for estimating a population mean. He illustrates the importance of not relying solely on a sample mean due to sampling variability, and introduces the idea of a margin of error to create a confidence interval. Using the example of estimating the mean length of fish in a lake, he demonstrates how to calculate a 95% confidence interval and acknowledges the inherent uncertainty in statistical estimation. The video also hints at the application of confidence intervals to other statistical summaries and the testing of hypotheses.

Takeaways
  • 🔍 The concept of a confidence interval is used to estimate a population mean from a sample, acknowledging that there will be some sampling variability.
  • 🧐 A single sample mean is not enough to estimate the population mean accurately due to the possibility of variation in different samples.
  • 📊 A confidence interval provides a range with a margin of error, indicating the level of confidence that the true population mean falls within this range.
  • 🎯 The sampling distribution of the mean is a theoretical set of all possible sample means, which helps in understanding the variability of sample means.
  • 📚 A common rule of thumb is that about 95% of sample means will fall within two standard errors of the true mean, under certain conditions.
  • 📉 The true mean is usually within two standard errors of the sample mean, but there's a 5% chance that it might not be, due to sampling variability.
  • 📝 The margin of error is calculated by extending two standard errors from the sample mean, creating a confidence interval that is likely to contain the population mean.
  • 🐟 An example given in the script is estimating the mean length of fish in a lake, where a sample mean of 41 cm with a standard deviation of 12 cm leads to a 95% confidence interval of 36.2 to 45.8 cm.
  • 📉 The width of the confidence interval is affected by the sample size; larger sample sizes result in smaller standard errors and narrower intervals.
  • 🔬 Confidence intervals can also be constructed for estimates that summarize relationships between variables, such as differences in means or correlations.
  • 👨‍🏫 The script suggests that further discussion on using the sampling distribution to test hypotheses about a population mean will be provided in a separate video.
Q & A

  • What is the main topic discussed by Mike Merin in the video?

    -The main topic discussed is the concept of a confidence interval for estimating a population mean in research.

  • Why might relying solely on the sample mean be problematic?

    -Relying solely on the sample mean is problematic because it does not account for the sampling variability, which means that different samples taken at different times could yield different results.

  • What is the purpose of a confidence interval in statistics?

    -The purpose of a confidence interval is to provide a range of values that, with a certain level of confidence, is likely to contain the true population mean, accounting for sampling variability.

  • What is the theoretical basis for the creation of a confidence interval?

    -The theoretical basis for creating a confidence interval is the sampling distribution of the mean, which describes the distribution of all possible sample means that could be obtained from a population.

  • What is the rule of thumb mentioned in the video for estimating the confidence interval?

    -The rule of thumb mentioned is that about 95% of all sample means will be less than two standard errors away from the true mean, which is used to estimate the margin of error for a 95% confidence interval.

  • Why is it important to understand that not all confidence intervals will capture the true mean?

    -It is important to understand this because it acknowledges the inherent uncertainty in statistical estimation. Even with a 95% confidence interval, there is still a 5% chance that the true mean is not captured, reflecting the limits of statistical inference.

  • How does the sample size affect the width of the confidence interval?

    -The width of the confidence interval is affected by the sample size because the standard error, which determines the margin of error, decreases as the sample size increases, resulting in a narrower and more precise confidence interval.

  • Can confidence intervals be constructed for other statistical estimates besides the mean?

    -Yes, confidence intervals can also be constructed for other statistical estimates such as differences in means or correlations, based on the same concepts and principles.

  • What is the relationship between the sample mean and the true mean in the context of a confidence interval?

    -In the context of a confidence interval, the sample mean is the best estimate of the true mean, but the true mean is usually less than two standard errors away from the sample mean, which is used to define the interval within which the true mean is likely to fall.

  • What is the significance of the margin of error in a confidence interval?

    -The margin of error in a confidence interval signifies the range within which the true population mean is expected to fall, given the sample data and the level of confidence. It reflects the precision of the estimate and the impact of sampling variability.

Outlines
00:00
📊 Understanding Confidence Intervals for Population Mean

In this paragraph, Mike Merin introduces the concept of a confidence interval for estimating a population mean from a sample. He explains the limitations of relying solely on the sample mean due to sampling variability and how a confidence interval, with a margin of error, can account for this variability. The idea is illustrated with an example of estimating the mean length of fish in a lake, where the true population mean is known to be 40 cm. The paragraph also discusses the rule of thumb that about 95% of sample means fall within two standard errors of the true mean, creating a 95% confidence interval. The concept of the sampling distribution is highlighted as crucial for attaching a margin of error to the estimate, leading to the creation of a confidence interval.

05:02
🔍 Further Exploration of Confidence Intervals and Hypothesis Testing

The second paragraph briefly mentions that the principles discussed for constructing confidence intervals can also be applied to other statistical estimates, such as differences in means or correlations. It also hints at a future video that will delve into how the sampling distribution can be utilized to test hypotheses about a population mean. The paragraph concludes with a reminder to check out accompanying statistics visualizations and a casual, personal anecdote that seems unrelated to the main topic.

Mindmap
Keywords
💡Confidence Interval
A confidence interval is a range of values, derived from a statistical model, that is likely to contain the value of an unknown population parameter. In the video, the concept is central to understanding how to estimate the mean of a population, such as the mean length of fish in a lake. The script uses the example of a sample mean of fish lengths to illustrate how a confidence interval can be calculated and interpreted, providing a range within which the true population mean is likely to fall.
💡Population Mean
The population mean refers to the average value of a particular variable for an entire population. In the context of the video, the population mean is the true average length of all fish in the lake. The script discusses how researchers use sample data to estimate this value, acknowledging that due to sampling variability, the sample mean will not exactly equal the population mean.
💡Sample Mean
The sample mean is the average of the values in a sample, which is a subset of the population. In the video, the sample mean is used as an estimate for the population mean. The script provides an example where a sample of 25 fish has a mean length of 41 cm, which is then used to infer information about the entire population of fish in the lake.
💡Sampling Variability
Sampling variability is the natural fluctuation in the results that occur when different samples are taken from a population. The video explains that because of this variability, different samples may have different means, and thus, relying solely on a single sample mean can be problematic. It is a key concept in understanding the need for confidence intervals.
💡Margin of Error
The margin of error is the range plus or minus a certain value from the sample statistic that indicates the precision of an estimate. In the video, the margin of error is calculated as two standard errors from the sample mean, which helps to create a confidence interval. It is used to express the uncertainty associated with the sample mean as an estimate of the population mean.
💡Standard Error
The standard error is a measure of the average distance that sample estimates differ from the true population value. The script explains that the standard error is a critical component in calculating the margin of error and, by extension, the confidence interval. It is used to quantify the sampling variability of the sample mean.
💡Sampling Distribution
A sampling distribution is the probability distribution of a given statistic based on a random sample. The video script uses the concept of the sampling distribution of the mean to explain how all possible sample means are theoretically distributed and how this distribution helps in attaching a margin of error to the estimate.
💡95% Confidence Level
The 95% confidence level is a common threshold used in statistical analysis, indicating that if the procedure is repeated many times, the true population parameter will fall within the calculated interval in 95% of the cases. The video script discusses this level in the context of creating a confidence interval for the mean length of fish in the lake.
💡Standard Deviation
Standard deviation is a measure of the amount of variation or dispersion in a set of values. In the video, the sample standard deviation of fish lengths is given as 12 cm, which is a key statistic used in calculating the standard error and, subsequently, the confidence interval.
💡Hypothesis Testing
Hypothesis testing is a statistical method used to make decisions about a population parameter based on sample data. Although not the main focus of the video, the script mentions that the sampling distribution can be used for hypothesis testing about a population mean, indicating a broader application of the concepts discussed.
💡Statistical Estimation
Statistical estimation involves using sample data to infer characteristics about a population. The video is centered around the process of estimation, specifically estimating the mean length of fish in a lake. It discusses how confidence intervals provide a range within which the true population mean is likely to fall, based on the sample data.
Highlights

The concept of a confidence interval for a population mean is discussed.

A sample is used to estimate the population mean, but relying solely on the sample mean can be problematic due to sampling variability.

A margin of error is attached to the estimate to account for differences between samples.

The sampling distribution describes the theoretical set of all possible estimates.

A rule of thumb states that about 95% of sample means will be within two standard errors of the true mean.

The true mean is usually within two standard errors of the sample mean, allowing for a confidence interval to be created.

5% of sample means will be more than two standard errors away from the true mean, not capturing it within the confidence interval.

The standard error and confidence interval width depend on the sample size, becoming smaller and more precise with larger sample sizes.

An example is given estimating the mean length of fish in a lake, with a 95% confidence interval calculated.

Confidence intervals can also be constructed for estimates summarizing relationships between variables.

The sampling distribution is used to test hypotheses about a population mean in a separate video.

The video includes statistics visualizations to accompany the concepts discussed.

The importance of accepting the limitations of statistical estimates is emphasized.

The video explains the process of creating a confidence interval using a sample mean and standard error.

The impact of sampling variability on the accuracy of population mean estimates is highlighted.

The video provides a practical application of confidence intervals in estimating the mean length of fish.

The role of the sample size in determining the precision of the confidence interval is explained.

Transcripts

Browse More Related Video

What is a Hypothesis Test and a P-Value? | Puppet Master of Statistics

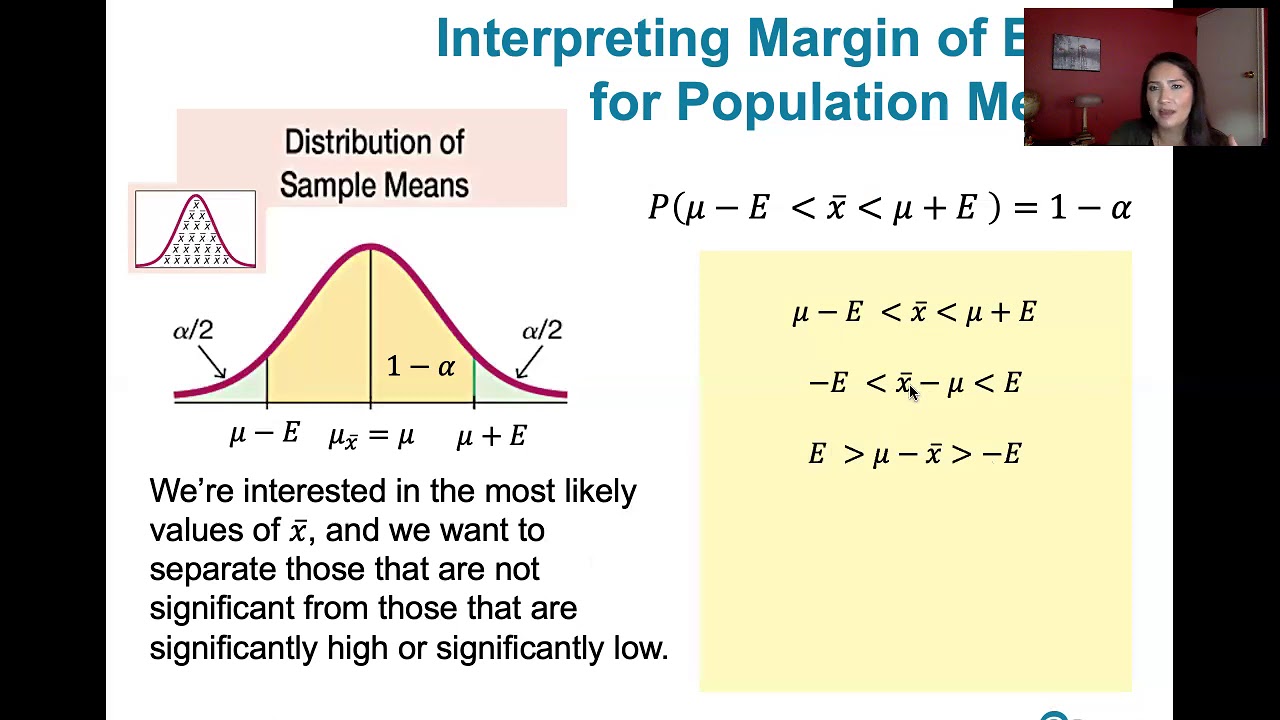
7.2.2 Estimating a Population Mean - Sample Mean, Margin of Error, and Finding Confidence Intervals

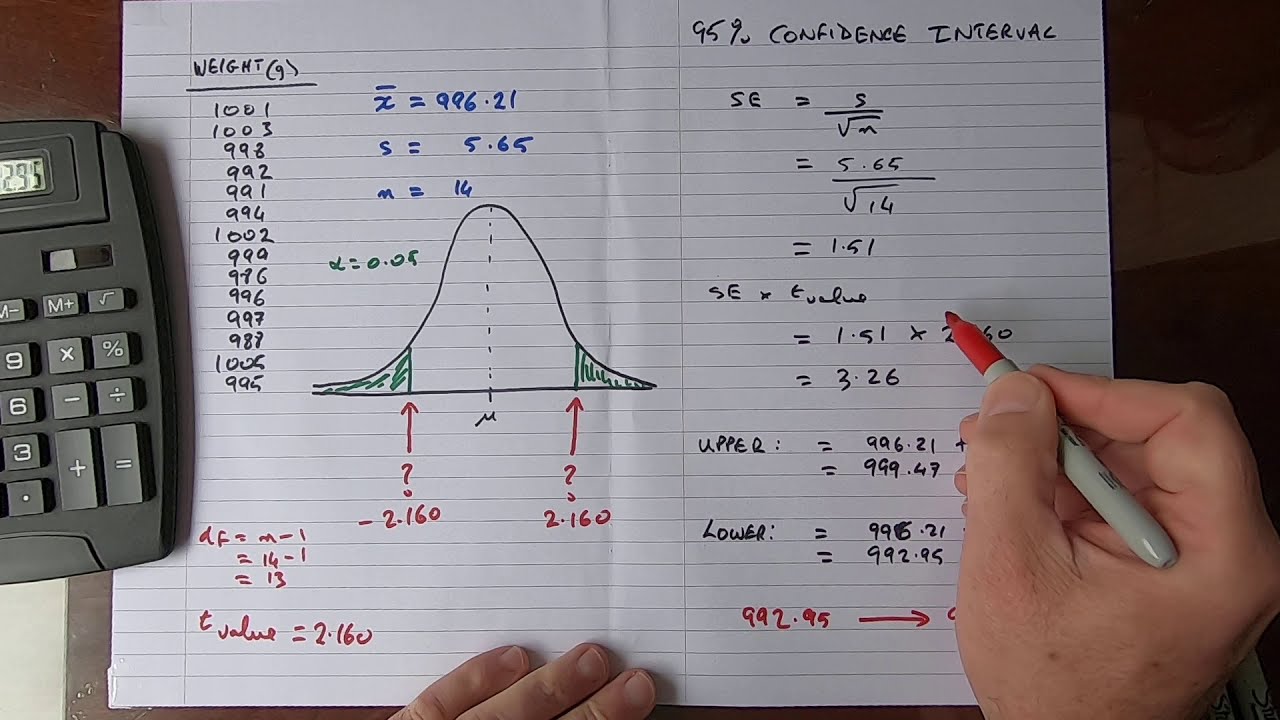
How To...Calculate the Confidence Interval for a Sample

Confidence Interval for a population mean - t distribution

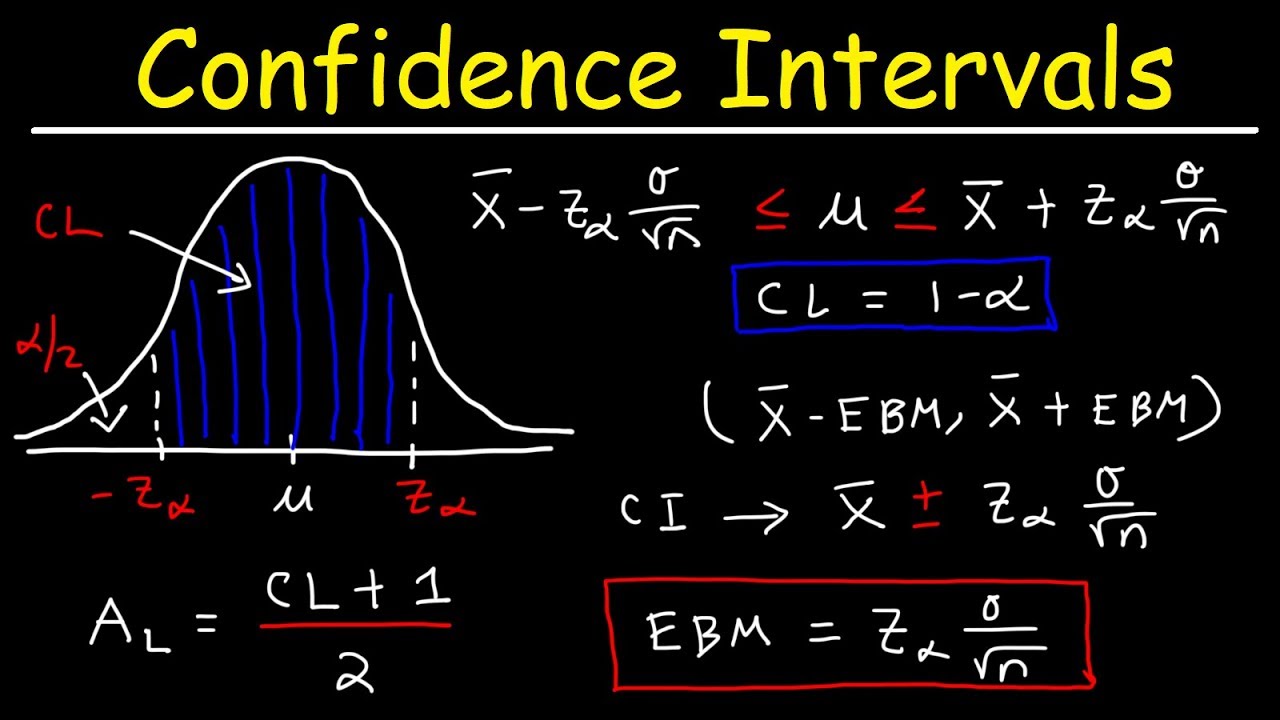
How To Find The Z Score, Confidence Interval, and Margin of Error for a Population Mean

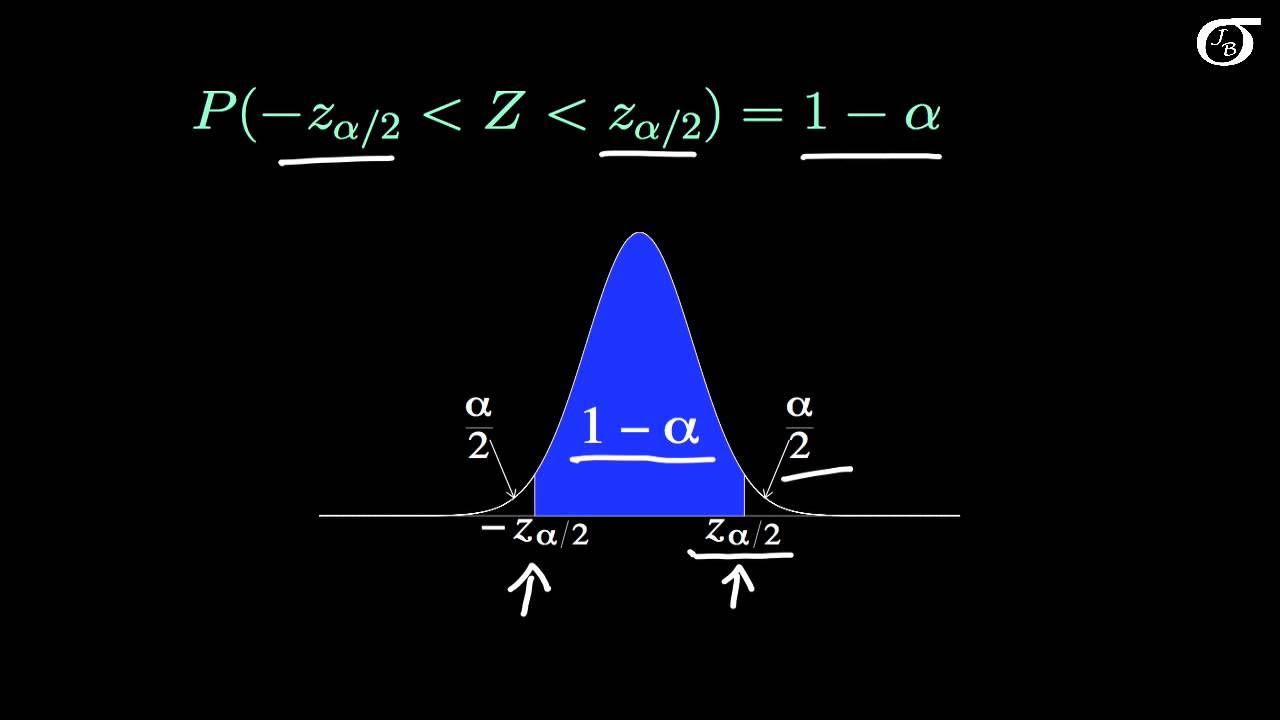
Deriving a Confidence Interval for the Mean (The Rationale Behind the Confidence Interval Formula)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Confidence IntervalPopulation MeanSampling VariabilityStatistical ResearchEstimation MethodsSample MeanMargin of ErrorStandard ErrorStatistical AnalysisData Interpretation