What is Flux? + an Introduction to Gauss Law (Electromagnetism – Physics)
TLDRThis physics tutorial explores the concept of flux in electromagnetism, using the water flow analogy to illustrate how field lines represent the strength and direction of a field. It introduces Gauss's Law, a fundamental principle in electrostatics, demonstrating how electric field flux through a closed surface relates to enclosed charges. The video also derives Coulomb's Law from Gauss's Law, showing the independence of flux from the shape or size of the surface and its dependence solely on enclosed charges, providing a clearer understanding of electromagnetism's core concepts.
Takeaways
- 🌐 The concept of flux is central to understanding electromagnetism, illustrating the flow of fields such as electric and magnetic through an area.
- 💧 Flux can be compared to the flow of water in a tube, where the density of flow lines represents the velocity and the number of lines through an area represents the flow rate.
- 📚 Gauss's Law is a fundamental principle in electromagnetism, stating that the electric field flux through a closed surface is proportional to the enclosed charge.
- 📏 The strength of a field is represented by the density of field lines, and the flux is the amount of field flowing through a given area, analogous to the number of field lines passing through.
- 📉 Flux is independent of the shape or size of the closed surface and is not affected by charges located outside the surface.
- 📚 The calculation of flux involves taking the dot product of the field strength vector and the area vector, considering the angle between them.
- 🔍 For non-uniform fields or curved areas, flux is calculated by integrating the dot product over a differential area.
- 🌀 The video uses an analogy of an object in water flow to help visualize the concept of a field flowing, although it's noted as an approximation.
- 🔗 The relationship between flux and field strength is such that field strength is essentially the flux density, a term sometimes used interchangeably in texts.
- 🌟 Gauss's Law is applied to derive Coulomb's Law, showing the force between two charges is proportional to the product of the charges and inversely proportional to the square of the distance between them.
- 🎯 The video concludes by emphasizing the importance of understanding flux for grasping more complex concepts like inductance and magnetic fields.
Q & A
What is the concept of flux in the context of electromagnetism?
-Flux in electromagnetism refers to the amount of a field, such as an electric or magnetic field, that passes through a given area. It is analogous to the flow rate of water through a pipe, where the density of field lines represents the strength of the field, and the number of field lines passing through an area represents the flux.
How is the concept of flux illustrated in the video?
-The video illustrates the concept of flux by comparing it to the flow of water in a tube with varying diameters. The density of the flow lines, which represent the velocity of the water, is used as an analogy for the strength of an electric or magnetic field, and the number of flow lines passing through a cross-section represents the flux.
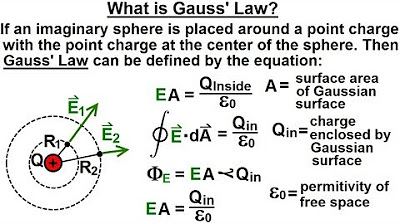
What is Gauss's Law and how is it related to the concept of flux?
-Gauss's Law states that the electric field flux through a closed surface is proportional to the total charge enclosed by that surface. It is the first of Maxwell's equations and is fundamental to understanding electrostatics. The law directly relates the concept of flux to the distribution of electric charges in a field.
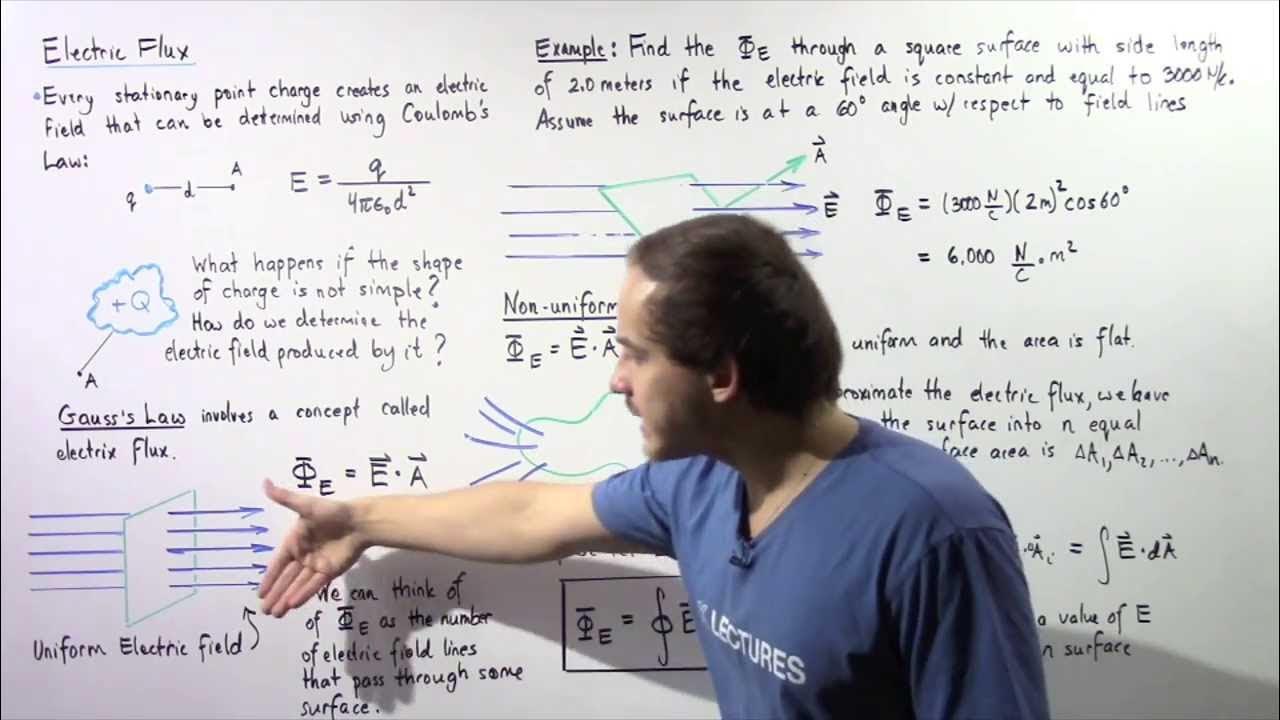
How is the flux calculated for a uniform field passing through a flat surface?
-For a uniform field passing through a flat surface, the flux is calculated by multiplying the magnitude of the field strength vector by the magnitude of the area vector, which is perpendicular to the surface.
What is the significance of the area vector in calculating flux?
-The area vector is significant in calculating flux because its direction is perpendicular to the plane containing the area, and its magnitude is proportional to the size of the area. It helps in determining the component of the field that is perpendicular to the area, which is essential for calculating the flux through that area.
How does the flux change when the field is not uniform or the area is curved?
-When the field is not uniform or the area is curved, the flux is calculated by considering a differential area in which the field can be considered constant. The flux through this differential area is the dot product of the field vector and the differential area vector, and the total flux is found by integrating this product over the entire area.
What is the relationship between field strength and flux density?
-The field strength is the flux density, meaning that the density of field lines represents the strength of the field, and the number of field lines passing through an area represents the flux of the field through that area.
How does Gauss's Law demonstrate that the electric field flux does not depend on the shape or size of the Gaussian surface?
-Gauss's Law demonstrates that the electric field flux through a closed surface is proportional to the enclosed charge, regardless of the surface's shape or size. This is because the total number of field lines crossing the surface remains constant, regardless of the surface's geometry.
What is the significance of the permittivity of free space in Gauss's Law?
-The permittivity of free space, denoted as Epsilon 0, is a constant that relates the electric field flux to the enclosed charge in Gauss's Law. It is a fundamental property of the vacuum that determines the strength of the electric field produced by a given charge.
How is Coulomb's Law derived from Gauss's Law?
-Coulomb's Law can be derived from Gauss's Law by considering a spherical Gaussian surface around a point charge and calculating the electric field flux through the surface. The law states that the force between two charges is proportional to the product of the charges and inversely proportional to the square of the distance between them, with the constant of proportionality being related to the permittivity of free space.
Outlines
🌌 Introduction to Electromagnetism and Flux
The video script begins with an introduction to the fascinating phenomenon of electromagnetism, emphasizing the importance of understanding the concept of flux. The host uses the analogy of water flowing through a tube to explain flux, comparing it to the flow of an electric field. The density of the flow lines represents the strength of the field, and the number of lines passing through an area represents the flux. The script introduces Gauss's Law as a fundamental principle in electromagnetism, which will be explored in the video. The host also acknowledges a minor issue with the video lighting, but assures viewers of the colorful content to follow.
📚 Calculating Flux and Gauss's Law
This paragraph delves into the calculation of flux, starting with the representation of an area as a vector, perpendicular to the plane it encloses. The script explains how to calculate the flux of a uniform field through an area and how to adjust for non-perpendicular fields using the dot product of the field vector and the area vector. It also addresses more complex scenarios involving non-uniform fields or curved areas, using integration to find the total flux. The script then transitions to Gauss's Law, which relates the electric field flux through a closed surface to the enclosed charge, introducing the concept of permittivity and its role in the environment.
🔋 Gauss's Law and Its Implications
The script continues with a detailed explanation of Gauss's Law, stating that the electric field flux through a closed surface is proportional to the enclosed charge. It uses the analogy of a point charge generating an electric field to illustrate how the flux can be calculated by integrating the dot product of the electric field vector and the differential area vector over a spherical surface. The law implies that the flux is independent of the shape or size of the surface and is unaffected by charges outside the surface. The host provides a visual proof of this concept by drawing larger spheres and demonstrating that the flux remains constant, despite the change in field strength with distance from the charge.
🧲 Deriving Coulomb's Law from Gauss's Law
The final paragraph focuses on applying Gauss's Law to derive Coulomb's Law, which describes the force between two charges. The host sets up a scenario with a point charge and a spherical Gaussian surface, calculating the electric field strength at the surface and relating it to the enclosed charge. By placing a second charge on the surface, the force experienced by this charge is calculated, leading to the formulation of Coulomb's Law. The script concludes with a summary of the video's content and an invitation for viewers to like, subscribe, and stay tuned for the next video on inductance and magnetic fields.
Mindmap
Keywords
💡Electromagnetism
💡Flux
💡Gauss's Law
💡Electric Field
💡Field Lines
💡Area Vector
💡Dot Product
💡Differential Area
💡Permittivity
💡Coulomb's Law
Highlights
Introduction to the concept of flux as a fundamental principle in electromagnetism.
Illustration of flux using the water flow analogy to explain field lines and their density.
Explanation of how the density of field lines represents the strength of a velocity or electric field.
Introduction of Gauss's Law as a key principle in electrostatics and part of Maxwell's equations.
Description of how flux is calculated as the flow rate through a given area in a field.
The concept that flux is constant regardless of the cross-section size in a tube flow.
Discussion on the representation of area with a vector and its relation to calculating flux.
Clarification that the flux is the dot product of the field strength vector and the area vector.
Explanation of how to calculate flux when the field is not uniform or the area is curved.
The importance of understanding flux for grasping the significance of Gauss's Law in electrostatics.
Gauss's Law stated as the electric field flux through a closed surface being proportional to enclosed charges.
Derivation of Coulomb's Law from Gauss's Law, demonstrating the relationship between charge, distance, and force.
Illustration of how the electric field strength decreases with distance from a charge but the flux remains constant.
Demonstration that the flux does not depend on the shape or size of the Gaussian surface.
Explanation of how only enclosed charges contribute to the flux through a Gaussian surface.
The analogy of water flow to explain the concept of flux in electric fields, despite its limitations.
The upcoming topic of inductance and its relation to the understanding of magnetic fields.
Encouragement for viewers to like, subscribe, and engage with the content for more physics education.
Transcripts
Browse More Related Video
Electric Flux and Gauss’s Law | Electronics Basics #6
Gauss law of electricity | Electrostatics | Physics | Khan Academy
Electric Flux (part 1)
Gauss law logical proof (any closed surface) | Electric charges & fields | Physics | Khan Academy
Electric Flux
Physics 37.1 Gauss's Law Understood (3 of 29) What is Gauss' Law?
5.0 / 5 (0 votes)
Thanks for rating: